The Other Side of Zero – Complete Guide For Class 6th Math Chapter 10
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, The Other Side of Zero in Mathematics for Class 6th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
The chapter The Other Side of Zero explores the fascinating world of integers, which includes both positive and negative numbers. The chapter begins by introducing the concept of an ‘infinite lift’ to help visualize the addition and subtraction of integers. By imagining movements between floors, students learn how subtracting a negative number is equivalent to adding its positive counterpart. The chapter then transitions to the number line, showing that it extends infinitely in both directions from zero, accommodating negative numbers to the left and positive numbers to the right. This visual tool aids in understanding how to compare, add, and subtract integers. Through various examples and exercises, students develop a solid grasp of these concepts, preparing them for more complex mathematical operations involving integers.
The Other Side of Zero
More and More Numbers!
We began our mathematical journey with counting numbers: 1, 2, 3, 4, and so on. Soon, we discovered more numbers, such as zero, representing nothing. This number, zero, has a significant history in India and worldwide.
The Indian number system uses digits from 0 to 9, enabling us to write numbers, whether large or small, using these ten digits.
Next, we encountered fractions like 1/2, 3/2, and 13/6, which lie between whole numbers. But are there even more numbers? If zero is a number that comes before one and is less than one, are there numbers less than zero?
Consider the number line we know:
This line only shows numbers from zero to the right, making it a number ‘ray’. But what if there are numbers to the left of zero, turning this ray into a full number line? This is what we will explore in this chapter!
Bela’s Building of Fun
Let’s go further in this chapter – The Other Side Of Zero, Bela’s ice cream factory, famous for its delicious treats, now features a multistoried building filled with attractions called Bela’s Building of Fun.
Some floors are below ground, adding to the excitement. The building’s lift has two buttons: ‘+’ to go up and ‘–’ to go down.
For example, to reach the Art Centre from the Welcome Hall, you press the ‘+’ button twice (+2). Conversely, to go down two floors, you press the ‘–’ button twice (–2).

Numbering the Floors
Entry to the Building of Fun is on the ground floor, labeled Floor 0, the Welcome Hall.
Pressing ‘+1’ takes you to the Food Court (Floor +1), and pressing ‘+2’ takes you to the Art Centre (Floor +2).
To reach the Toy Store below ground, you press ‘–1’, making it Floor –1. Similarly, pressing ‘–2’ takes you to the Video Games shop on Floor –2.
Numbers with a ‘+’ sign are positive, while those with a ‘–’ sign are negative. Zero, the ground floor, is neither positive nor negative.
Movement with Lift Buttons
Further, on the other side of zero, we’ll understand movement with lift buttons. This involves-
- Combining button presses is also an addition
- Back to zero!
Using lift buttons, we can track movement between floors.
For instance, starting at the Food Court (+1) and pressing ‘+2’ takes you to the Book Store (+3): Starting Floor + Movement = Target Floor
(+1) + (+2) = (+3)
If you make a mistake, like pressing the ‘+’ button three times when you want to stay on the ground floor, you can cancel it by pressing the ‘–’ button three times. This is represented as:
(+3) + (–3) = 0
The inverse of +3 is –3, and vice versa.
Using inverses, if you press +4 and then –4, you return to the ground floor (0).
Comparing Numbers Using Floors
According to the chapter, The other side of zero, Comparing floors helps us understand the concept of greater and smaller numbers.
For example, Floor +3 is lower than Floor +4, so we write +3 < +4.
Similarly, Floor –4 is lower than Floor –3, so –4 < –3.
Subtraction to Find the Button Press
As stated in the chapter The other side of zero, subtraction helps determine the button press needed to move between floors.
For instance, if your starting floor is the Art Centre (+2) and your target is the Sports Centre (+5), you need to go up three floors: Target Floor – Starting Floor = Movement needed
(+5) – (+2) = (+3)
Similarly, moving from Floor –2 to Floor –1 requires pressing the ‘+1’ button:
(–1) – (–2) = (+1)
And moving from Floor +3 to Floor –1 requires pressing the ‘–4’ button:
(–1) – (+3) = (–4)
Adding and Subtracting Larger Numbers
Let’s go further in the chapter – The other side of zero and understand the concept of adding and subtracting large numbers. Imagine a mine where minerals are extracted. The mine has levels marked by positive and negative numbers, indicating how many meters above or below ground level they are. The same principles of addition and subtraction apply here.
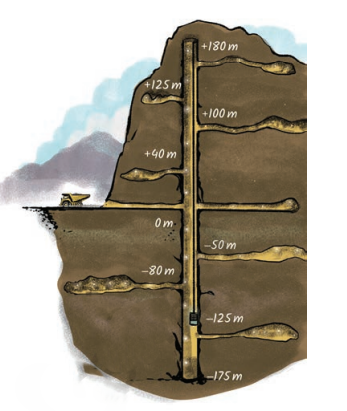
For instance:
- (+40) + (+60) = +100
- (–90) + (–55) = –145
Similarly, to find the movement needed:
(+40) – (–20) = (+60)
Using these concepts, we can navigate through both the Building of Fun and the mine, understanding the fascinating world of integers and their applications.
Integers: … – 4, – 3, – 2, – 1, 0, 1, 2, 3, 4, …
Adding, subtracting, and comparing any numbers
Let’s go further in the chapter – The other side of zero and understand the concept of adding, subtracting, and comparing numbers. To add and subtract even larger integers, imagine an ‘infinite lift’ that extends forever upwards and downwards, starting from Level 0.
This conceptual lift helps us visualize addition and subtraction of any integers.
Example:
Consider the subtraction +2000 – (–200).
Imagine a lift with 2000 levels above ground and 200 below ground:
Target Level−Starting Level = Movement Needed
To go from –200 to +2000:
Movement Needed = +2200
Thus, (+2000)−(–200)=+2200.
Notice that (+2000)+(+200) is also +2200
Number Line and Infinite Lift
According to the chapter – the other side of zero, the infinite lift can be rotated to resemble a number line, helping to visualize movements:
Starting Number + Movement = Target Number
Examples of the Number Line
- If, from 5 you wish to go over to 9, how far must you travel along the number line?
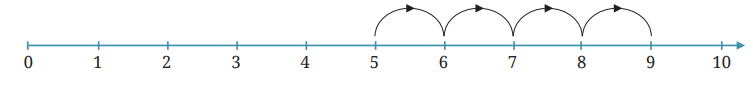
You must travel in 4 steps. That is why 5 + 4 = 9.
(Remember: Starting Number + Movement = Target Number)
The corresponding subtraction statement is 9 –5 = 4.
(Remember: Target Number – Starting Number = Movement needed)
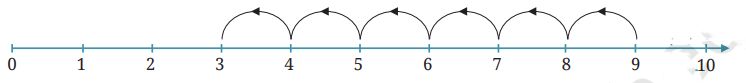
- Now, from 9, if you wish to go to 3, how much must you travel along the number line?

You must move 6 steps backward, i.e., you must move –6.
Hence, we write 9 + (–6) = 3.
(Remember again : Starting Number + Movement = Target Number)
The corresponding subtraction statement is 3–9=–6.
(Remember again: Target Number – Starting Number = Movement needed)
Using an Unmarked Number Line
As stated in the chapter the other side of zero, you can use an unmarked number line (UNL) for additions and subtractions:
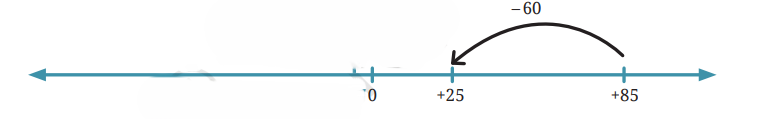
- Addition Problem: 85+(–60)=?
+85+(–60)=+25
- Subtraction Problem: (–100)–(+250)=?
(–100)–(+250)=? ?=–350
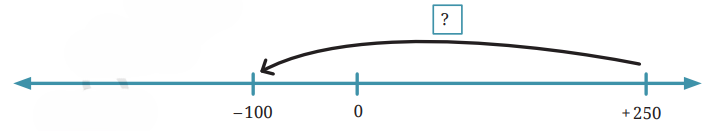
Converting Subtraction to Addition
To find the movement from 2 to –3:

- First Method: Looking at the number line, we see we need to move –5 (i.e., 5 in the backward direction). Therefore, – 3 – 2 = – 5. The movement needed is –5.
- Second Method: Break the journey from 2 to –3 into two parts.
- From 2 to 0, the movement is 0–2=–2.
- From 0 to –3, the movement is –3–0=–3. The total movement is the sum of the two movements: – 3 + (– 2) = – 5.
The Token Model
Using Tokens for Subtraction
Further in the chapter The other side of zero, we have seen how to perform the addition of integers with positive and negative tokens.
Similarly, we can perform subtraction using tokens!
Example 1: Subtracting (+5) – (+4)
From 5 positives, take away 4 positives:
+5−+4 = +1

Example 2: Subtracting (–7) – (–5)
Is (–7) – (–5) the same as (–7) + (+5)?
−7−(−5) = −7+5 = −2

Example 3: Subtracting +4 – (–6)
Start with 4 positives. We need to take out 6 negatives from these. By adding six zero pairs (6 positives and 6 negatives), we can then take away 6 negatives:
(+4−(−6) = +4+6 = +10

Integers in Other Places
Credits and Debits
Another topic to be covered in the chapter – the other size of zero is integers in other places. Consider opening a bank account with a balance of ₹100. The transactions in your account can be visualized as credits and debits.
Deposit ₹60 (credit):
New Balance: ₹100 + ₹60 = ₹160
Pay electric bill ₹30 (debit):
New Balance: ₹160 – ₹30 = ₹130
Major purchase ₹150 (debit):
New Balance: ₹130 – ₹150 = -₹20
Make ₹200 at your business (credit):
New Balance: -₹20 + ₹200 = ₹180
In banking terms, ‘credits’ are positive numbers, and ‘debits’ are negative numbers.
The total of all credits and debits determines your bank account balance, which can be positive or negative.
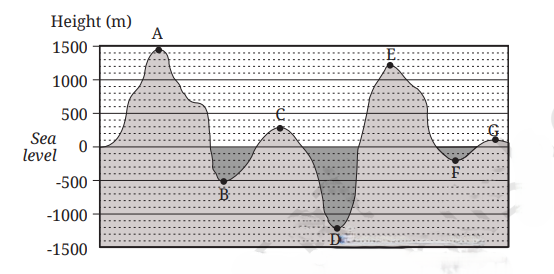
Geographical Cross Sections
As stated in the chapter – The other side of zero, Heights of geographical features such as mountains and plateaus are measured from sea level (0m).
Heights above sea level are positive, while those below are negative.
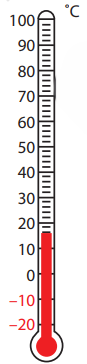
Temperature
Temperatures are measured in Celsius (°C).
For example, during summer, you might hear about a ‘heat wave’ with temperatures around 40°C, and in winter, temperatures could drop to around 15°C.
Explorations with Integers
A Hollow Integer Grid
Consider the following integer grid:
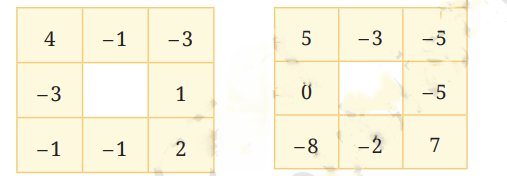
Top row sum: 4+(−1)+(−3) = 0
Bottom row sum: 5+(−3)+(−5) = −3
Left column sum: 4+(−3)+(−1) = 0
Right column sum: (−3)+1+2 = 0
In each grid, the numbers in each of the two rows (the top row and the bottom row) and the numbers in each of the two columns (the leftmost column and the rightmost column) add up to give the same number.
We shall call this sum the ‘border sum’. The border sum of the first grid is ‘0’. Now let’s understand the other topic from the chapter The Other Side of Zero named A Pinch of History.
A Pinch of History
Ancient Use of Integers
As stated in the chapter on the other side of zero, Ancient Asia was the first to use general fractions and integers, including zero and negative numbers.
In China, negative numbers were used in accounting and represented with red and black rods in “The Nine Chapters on Mathematical Art” (1st or 2nd century CE).
In India, Kautilya wrote about credits and debits in the “Arthaśhāstra” (c. 300 BCE), acknowledging negative balances.
The Bakśhālī manuscript (~300 CE) used special symbols for negative numbers.
Brahmagupta’s Contributions
As mentioned in the chapter The Other Side of Zero, Brahmagupta (628 CE) provided explicit rules for operations on positive numbers, negative numbers, and zero:
- The sum of two positives is positive.
- The sum of two negatives is negative.
- To add a positive and a negative, subtract the smaller from the larger and keep the sign of the larger.
- The sum of a number and its inverse is zero.
- The sum of any number and zero is the same number.
Rules for Subtraction
Based on the chapter – On the other side of zero, there are various rules of subtraction which include-
- Subtracting a smaller positive from a larger positive yields a positive.
- Subtracting a larger positive from a smaller positive yields a negative.
- Further, subtracting a negative is the same as adding the corresponding positive.
- Subtracting a number from itself yields zero.
- Subtracting zero from a number yields the same number.
- At last, Subtracting a number from zero yields its inverse.
These rules allow for addition and subtraction with any numbers: positive, negative, and zero. Brahmagupta’s contributions formed the foundation of modern arithmetic.
While Asia embraced these concepts early, Europe was slow to accept negative numbers, with resistance persisting into the 18th century.
However, the understanding and acceptance of these numbers eventually revolutionized mathematics globally.
In conclusion, Math CBSE Class 6th Math, Chapter 10 – The Other Side of Zero offers a fascinating exploration into the world of integers. From introducing the concept of negative numbers to demonstrating their practical applications through number lines and real-life scenarios, this chapter provides a comprehensive understanding of integers. Visual tools like the Infinite Lift make learning engaging and easy to grasp, whether adding, subtracting, or comparing integers. With iPrep Learning Super App’s resources for Class 6th Math, Chapter 10 – The Other Side of Zero, you can strengthen your math skills with clarity and confidence. Dive into this exciting chapter and master the concepts in Math CBSE Class 6th Math, Chapter 10 – The Other Side of Zero!
Practice questions on Chapter 10 - The Other Side of Zero
Get your free Chapter 10 - The Other Side of Zero practice quiz of 20+ questions & detailed solutions
Practice Now









Wow, good
thanks, please give notes for science as well
you guys explain it so well