Halves and Quarters – Complete Guide For Class 4 Math Chapter 9

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Halves and Quarters in Mathematics for Class 4th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.  

At iPrep, we believe that learning should be both fun and effective. That’s why our resources for CBSE Class 4th Math, Chapter 9 – Halves and Quarters are crafted with engaging examples, stories, and interactive exercises that make understanding fractions an enjoyable experience. By breaking down complex ideas into simple, relatable concepts, we ensure that every student feels confident in their learning journey. Now, we’ll understand what are halves and quarters.

What Are Halves And Quarters?

Students are introduced to the concept of fractions in the chapter “Halves and Quarters,” which focuses on how whole items can be divided into equal portions. The definitions of halves and quarters – two and four equal portions, respectively – are covered at the beginning of the chapter. By breaking up a pizza, a cake, or a piece of paper, for example, students can practice sharing things fairly and gain a visual understanding of fractions.

The chapter also looks at how to portray fractions with both numbers and drawings, and it highlights the value of equal sharing. Students are encouraged to recognize fractions in real-world scenarios as they are exposed to fraction notation, which includes symbols like ½ for halves and ¼ for quarters. The chapter also teaches students how to add and subtract basic fractions, which lays the groundwork for more difficult fractional computations in later grades.

By the end of the chapter, students have a solid understanding of how to divide things into halves and quarters as well as how to use fractions in practical situations. This practical, visual method helps in helping young students understand the fundamentals of fractions.

Introduction: The Story of Two Cats and a Monkey

The chapter Halves and Quarters can very well be explained with the story of the two cats and a monkey. The story goes like this-

Once upon a time, two cats were the closest of friends. One day, they stumbled upon a large loaf of bread. Excited by their find, they quickly began to argue over how to share it equally.

“I should get the bigger piece because I found it first!” declared the first cat.
“No! I should get the bigger piece because I’m more hungry!” objected the second cat.

Their argument grew louder and more heated, catching the attention of a clever monkey sitting in a nearby tree. Curious about the commotion, the monkey decided to intervene.

“I can help you divide the bread fairly so that neither of you feels cheated,” the monkey offered, descending from the tree. Desperate to find a resolution, the cats agreed to let the monkey assist them.

The monkey took the loaf and broke it into two pieces, but one was larger than the other. To balance the portions, he took a bite from the larger piece. However, this only made the smaller piece appear larger in comparison.

The monkey continued this process, taking bites from the larger portion to even things out. With each bite, the pieces seemed to shift until, before long, there was hardly any bread left.

As the two cats watched in disbelief, they realized that if they had divided the bread equally from the start, they wouldn’t have lost everything.

This tale teaches us a valuable lesson: the importance of sharing fairly. In this chapter, we will explore how to split large objects into halves and quarters, ensuring that everyone gets their fair share.

Key Terms and Concepts

1. Whole

Before going into the topic of halves and quarters, let’s clarify what a whole is. Something whole is said to be whole and undivided. A cake, a loaf of bread, or any other item that hasn’t been broken into pieces could be it.

Consider a pizza as an example. The pizza is whole before it is sliced. It is a single, whole thing.

2. Half (½)

A half is the term for each of the two equal pieces that we divide into. The fraction ½ represents this. The story of the cats and the monkey demonstrates the significance of splitting an object in half such that the two halves are the same size.

As an example, let’s say you wish to share a chocolate bar with your friend. You would split the chocolate bar into two equal portions, or halves so that you each receive the same amount. You would each receive a quarter of the chocolate.

3. Quarter (¼)

A quarter is the term for each of the four equal pieces that we split something into. The fraction ¼ represents this. Comparable to dividing anything in half, dividing it into quarters results in identical portions.

For example, you can cut a round cake into four equal pieces if you have four friends. Each piece would make up ¼ of the cake, or a quarter.

Understanding Fractions: One Half, Half of a Half, and Other Fractions

One Half (½)

To divide anything into two equal portions is to say it’s half done. It is called one-half, or ½ when you remove one portion out of those two. The fair division is usually represented by this fraction. The item is divided into two halves, as shown by the bottom number (denominator), 2, and the top number (numerator), 1, which represents one portion.

Example: If you have a pizza and you cut it into two equal slices, each slice is one-half, or ½, of the pizza. If you eat one slice, you’ve eaten ½ of the pizza.

Half of a Half

When we take half of a half, we divide something into two equal parts and then further divide one of those parts into half again. Mathematically, half of a half is represented as:

½×½ = ¼

This means that half of a half is a quarter, or ¼.

Example: If you have a pizza and cut it into two halves, and then you cut one of those halves into two equal parts again, each of those smaller pieces is one-quarter, or ¼, of the whole pizza.

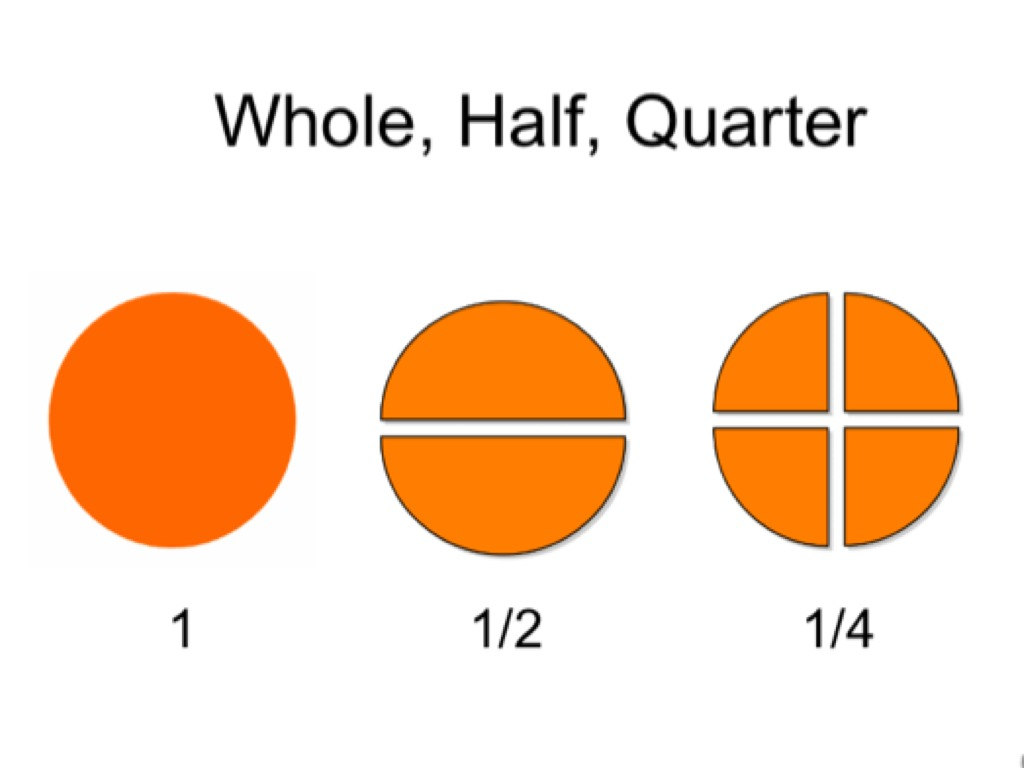
Meaning of ½ (One-Half)

The fraction ½ means dividing an object into two equal parts and taking one part. This is the simplest fraction and is frequently used in everyday situations like sharing food or measuring ingredients.

Example: If you have a chocolate bar and share it with a friend, you break it into two equal pieces. Each piece is one-half, or ½, of the chocolate bar.

Meaning of ¼ (One-Quarter)

The fraction ¼ represents one part of an object divided into four equal parts. The numerator (1) shows one part is taken, and the denominator (4) shows that the object has been divided into four parts.

Example: If a pizza is divided into four equal slices, each slice is one-quarter, or ¼, of the pizza.

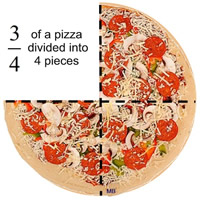
Meaning of ¾ (Three-Quarters)

The fraction ¾ means three parts out of four equal parts. The numerator (3) tells us how many parts are taken, and the denominator (4) indicates the total number of parts the object is divided into.

Example: If a pizza is cut into four equal slices, and you eat three slices, you have eaten ¾ of the pizza. The one slice left is ¼ of the pizza.

In summary, fractions like ½, ¼, and ¾ help us represent portions of a whole, making it easier to divide objects and quantities equally.

Equal Parts

For something to be divided into halves or quarters, the parts must be equal. This means that each part must be the same size. If the parts are not equal, it wouldn’t be a fair division.

Example: When cutting a pizza into two halves, both pieces must be of the same size, or one person will get more than the other, just like the cats and their loaf of bread.

Visualizing Halves and Quarters

Let’s go further in the chapter halves and quarters by visualizing them.

Drawing Fractions

One way to help students understand halves and quarters is by using drawings or objects they can cut or fold themselves. Let’s take a few examples:

Example: A Circle

• Draw a large circle on a piece of paper. This circle represents a whole pizza.
• Now, divide the circle into two equal parts. Each part is one-half, or ½, of the circle.
• Next, divide the circle into four equal parts. Each part is one-quarter, or ¼, of the circle.

By physically drawing or cutting shapes, students can see how fractions work.

Real-Life Examples of Halves and Quarters

1. Sharing Food

Imagine you have a sandwich, and you want to share it equally with your sibling. To do this, you cut the sandwich into two equal parts, which means each of you gets half of the sandwich. If there are three other friends with you, you can divide the sandwich into four equal parts. Each friend will get one-quarter of the sandwich.

2. Cutting a Pizza

When you order a pizza, it often comes sliced into equal parts. If the pizza is cut into two pieces, each piece is a half. If it’s cut into four pieces, each piece is a quarter. Now, if you have a pizza cut into 8 slices, each slice is one-eighth of the pizza.

3. Folding Paper

Let’s say you have a square piece of paper, and you want to fold it into halves. You fold the paper once, and you’ll have two equal parts. If you fold it again, you’ll have four equal parts, which are called quarters. This is a great hands-on way to show how whole objects can be divided into smaller, equal parts.

Addition and Subtraction of Fractions

Now that we understand what halves and quarters are, let’s learn how to work with them in math problems.

Adding Fractions

When adding fractions, it’s important to make sure the fractions have the same denominator (bottom number).

Example: If you have ½ of a chocolate bar and your friend gives you another ½, how much chocolate do you have in total?
½ + ½ = 1
You now have one whole chocolate bar.

Subtracting Fractions

Just like adding fractions, subtracting fractions works the same way, but instead, you take away parts.

Example: If you have a pizza and you eat ¼ of it, how much pizza do you have left?
1 − ¼  =  ¾
You have three-quarters of the pizza left.

Understanding Fair Sharing

The concept of halves and quarters is closely tied to the idea of fair sharing. When we divide something into equal parts, it ensures that everyone gets the same amount. This is important not only in math but also in everyday life.

Example: If you are playing a game with friends, and there are prizes to be distributed, dividing them into equal halves or quarters ensures that everyone feels they have a fair share.

Activities to Reinforce Learning

Activity 1: Fraction Hunt

Ask children to search the room or their own house for items that can be split into quarters and halves. They might discover, for instance, an apple, a sandwich, or even a piece of paper. After that, they can try applying the ideas of halves and quarters to divide these objects into equal pieces.

Activity 2: Cooking with Fractions

A wonderful method to practice fractions is through cooking. Students should be given a basic recipe to follow, requiring them to measure components in halves and quarters. They might require, for example, ½ cup sugar or ¼ teaspoon salt. This practical example reinforces the notion that fractions are utilized in daily life.

Conclusion

The “Halves and Quarters” chapter uses interesting, real-world examples to introduce students to the idea of fractions. Divide complete things into equal pieces to help students obtain practical mathematics skills and a feeling of justice. Understanding halves and quarters enables kids to make connections between mathematics and their surroundings, be it in sharing food, folding paper, or adding and subtracting fractions.

In conclusion, CBSE Class 4th Math, Chapter 9 – Halves and Quarters offers students an engaging introduction to fractions. By learning how to divide objects into equal halves and quarters, students not only strengthen their mathematical skills but also develop a sense of fairness in everyday situations. The chapter “Halves and Quarters” provides a solid foundation for understanding and applying fractions through real-world examples like sharing food or folding paper. Mastering these concepts prepares students for more advanced mathematical topics. As they explore Halves and Quarters, students can enjoy a fun and practical approach to learning fractions that will stay with them for years to come.

This chapter makes fractions understandable and enjoyable through stories, practical exercises, and visual aids, preparing students for more difficult concepts in subsequent classes. The concept of fair and equal division is an important lesson for both mathematics and life, as demonstrated by the stories of the two fighting cats, the intelligent monkey, and sharing pizza with friends.