Symmetry – Complete Guide For Class 6 Math Chapter 9

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Symmetry in Mathematics for Class 6th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Symmetry introduces students to the concept of balance and harmony in shapes and figures. It explains line symmetry, where a figure can be divided into two identical halves by a line, and rotational symmetry, where a figure looks the same after a certain degree of rotation around a central point. Practical activities such as paper folding, creating ink blot patterns, and identifying symmetrical objects in the environment are included to help students grasp these concepts. The chapter emphasizes the importance of symmetry in both natural and human-made designs, highlighting its aesthetic and mathematical significance. By understanding and applying the principles of symmetry, students learn to recognize and appreciate the balanced proportions in various shapes and patterns.

Symmetry 

Welcome to our exploration of symmetry in the beautiful world around us. Let’s dive into the fascinating concept of symmetry and discover how it adds balance and harmony to various objects and figures.

Symmetry in Everyday Objects

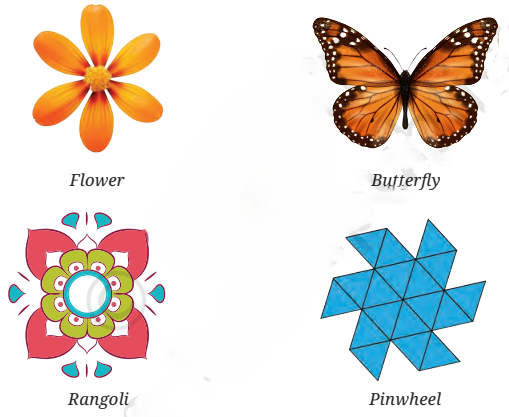
Take a look at objects around you like flowers, butterflies, rangolis, and pinwheels. 

Something is captivating about their appearance. 

The flower, for instance, looks the same from multiple angles. 

The butterfly, with its vibrant colors, also presents a symmetrical allure. 

In these examples, parts of the figures are repeated in a definite pattern, creating a pleasing sense of balance.

In the case of the rangoli, when rotated by 90 degrees around its center, the petals realign perfectly, showcasing rotational symmetry. 

The pinwheel, with its repeating shapes along each side of a hexagon, is another example of symmetry. 

In contrast, clouds, with their random formations, lack this repetitive pattern and are not symmetrical.

Definition: A symmetry refers to a part or parts of a figure that is repeated in some definite pattern. 

Line of Symmetry

A line of symmetry divides a figure into two mirror-image halves. 

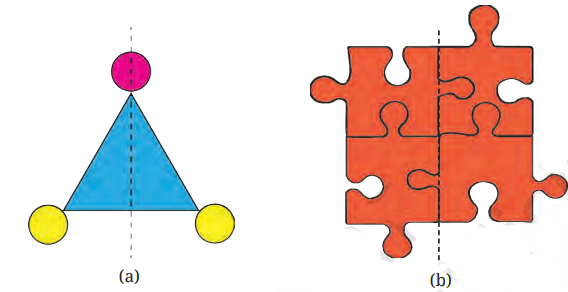
For instance, folding a triangle along its dotted line results in one half perfectly covering the other. These are called mirror halves! 

This concept extends to more complex shapes. 

For example, a square has multiple lines of symmetry—vertical, horizontal, and diagonal folds all produce symmetrical halves.

Definition: A line that cuts a figure into two parts that exactly overlap when folded along that line is called a line of symmetry of the figure.

Figures with more than One Line of Symmetry

Does a square have only one line of symmetry? 

Take a square piece of paper. By folding, find all its lines of symmetry. 

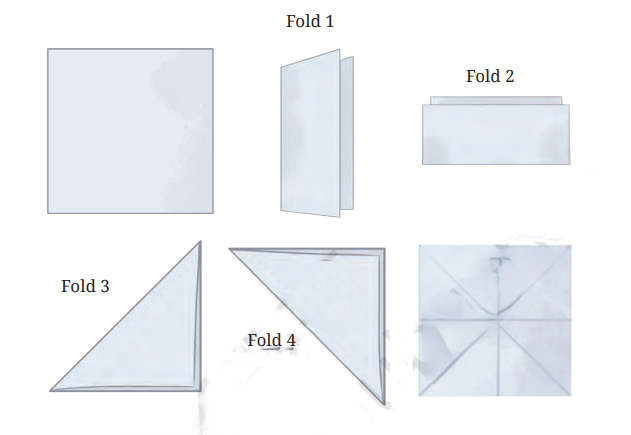
Here are the different folds giving different lines of symmetry. 

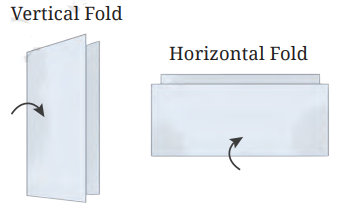
• Fold the paper in half vertically. 

• Fold it again into half horizontally (i.e., you have folded it twice). Now open out the folds.

The diagonal of a square is also a line of symmetry. 

Let’s consider a rectangle. Unlike a square, its diagonal fold does not create symmetrical halves. This highlights the unique symmetry properties of different shapes.

Reflection

When a figure is reflected along its line of symmetry, the parts on either side mirror each other. In other words, If we fold a figure along a line of symmetry, the two parts overlap completely. We could also say that the part of the figure on one side of the line of symmetry is reflected by the line to the other side; similarly, the part of the figure on the other side of the line of symmetry is reflected to the first side!

Definition: A figure that has a line or lines of symmetry is thus, also said to have reflection symmetry.

Imagine reflecting a square with labeled corners (A, B, C, D) along its vertical line of symmetry. Points B and C move to positions occupied by A and D, and vice versa. 

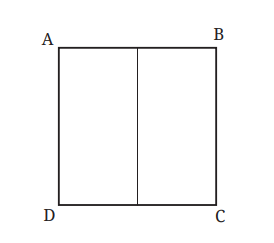
This reflection symmetry is a fundamental aspect of symmetrical figures.

Generating Symmetrical Shapes

Creating symmetrical figures can be a fun activity. 

One method involves ink blotting. 

Fold a piece of paper, spill a few drops of ink on one half, press the halves together, and then open it to reveal a symmetrical pattern. 

Similarly, paper folding and cutting techniques can produce intricate symmetrical designs, perfect for decorations and art projects.

a beautiful representation of symmetry in everyday objects from class 6 math chapter 9 – Symmetry

Rotational Symmetry

Rotational symmetry is observed when a figure looks the same after a certain angle of rotation. 

For example, a windmill rotated by 90 degrees appears unchanged, exhibiting rotational symmetry. 

The fixed point around which the object rotates is known as the center of rotation. 

Different figures have varying angles of rotational symmetry. 

An angle through which a figure can be rotated to look the same is called an angle of rotational symmetry.

A square, for instance, overlaps with itself after a 90-degree rotation, showcasing four angles of symmetry (90°, 180°, 270°, and 360°).

Example: 

Find the angles of symmetry of the following strip.

Solution: Let us rotate the strip in a clockwise direction about its center. 

A rotation of 180° results in the figure above.

Does this overlap with the original figure? No. Why? 

Another rotation through 180° from this position gives the original shape.

This figure comes back to its original shape only after one complete rotation through 360°. So, we say that this figure does not have rotational symmetry.

Exploring Radial Symmetry

Figures with radial arms, like the one with four radial arms, have distinct angles of symmetry. 

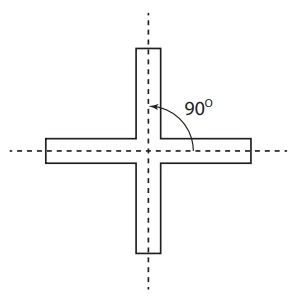
Adjusting the angles between these arms can modify the symmetry properties. 

For instance, If a figure with three radial arms should have rotational symmetry, then a rotated version of it should overlap with the original. Here are rough diagrams of both of them. If these two figures must overlap, what can you tell about the angles?

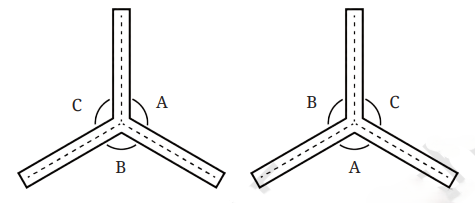
Observe that ∠A must overlap ∠B, ∠B must overlap ∠C and ∠C must overlap ∠A. 

So, ∠A = ∠B = ∠C. What must this angle be? 

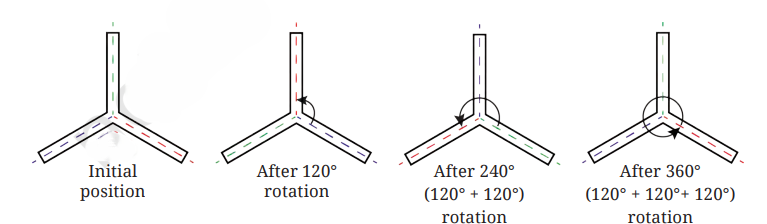
We know that a full turn has 360 degrees. This is equally distributed amongst these three angles. So each angle must be 360°/3 = 120°.

So, the radial arms figure with 3 arms shows rotational symmetry when the angle between the adjacent dotted lines is 120°. 

Symmetries of a Circle

A circle is unique in that it has infinite angles of symmetry. Any rotation around its center results in the circle coinciding with itself. Additionally, any diameter of the circle acts as a line of reflection symmetry, highlighting the perfect balance inherent in circular shapes.

Like wheels, we can find other objects around us having rotational symmetry. 

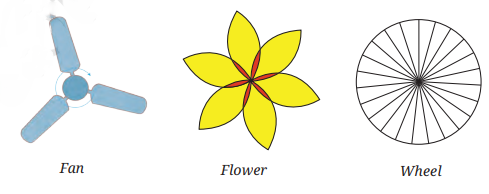
Summary

• When a figure is made up of parts that repeat in a definite pattern, we say that the figure has symmetry. We say that such a figure is symmetrical.  
• A line that cuts a plane figure into two parts that exactly overlap when folded along that line is called a line of symmetry or axis of symmetry of the figure.  
• A figure may have multiple lines of symmetry.  
• Sometimes a figure looks the same when it is rotated by an angle about a fixed point. Such an angle is called an angle of symmetry of the figure. A figure that has an angle of symmetry strictly between 0 and 360 degrees is said to have rotational symmetry. The point of the figure about which the rotation occurs is called the center of rotation.  
• A figure may have multiple angles of symmetry.  
• Some figures may have a line of symmetry but no angle of symmetry, while others may have angles of symmetry but no lines of symmetry. Some figures may have both lines of symmetry as well as angles of symmetry.  

Let’s Conclude

In conclusion, the chapter Symmetry in CBSE Class 6 Math, Chapter 9, offers an exciting exploration of balance and harmony in both natural and man-made designs. Through engaging activities and examples, students gain a deeper understanding of how symmetry manifests in various objects, from simple lines to complex shapes. Whether it’s through line symmetry or rotational symmetry, this chapter provides valuable insights into the aesthetic and mathematical importance of symmetry.

With the iPrep App, mastering the concept of symmetry in Chapter 9 is made fun and interactive, helping students strengthen their mathematical foundation while enjoying the learning journey. Embrace the beauty of symmetry in Symmetry – Complete Guide For Class 6 Math Chapter 9, and apply these concepts in both your academic and everyday life.