Fractions – Complete Guide For Math Class 6 Math Chapter 7

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Fractions in Mathematics for Class 6th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Fractions introduces the concept of fractions as a way to represent parts of a whole. It explains that a fraction consists of a numerator and a denominator that make up the whole. Students learn to differentiate between proper fractions, improper fractions, and mixed numbers, and practice converting between these forms. The chapter covers essential skills such as simplifying fractions and performing basic arithmetic operations like addition, subtraction, multiplication, and division with fractions. Visual aids like fraction strips and number lines help students understand these concepts better. By mastering fractions, students build a strong foundation for future mathematical topics and real-life applications, such as measuring quantities and understanding ratios.

Fractions

Fractions are a way to represent a part of a whole. Imagine sharing something equally among people—fractions tell us how much each person gets. 

For instance, if one roti is divided equally between two children, each child gets half a roti. This half is represented as the fraction ½. Similarly, if the roti is shared among four children, each child gets ¼ of the roti. It’s clear that the more people there are, the smaller the share each one receives, so ½ is larger than ¼.

i.e. 1/2 >1/4

Fractional Units and Equal Shares

Definition: When a whole is divided into several equal parts, each part is called a fractional unit. 

Understanding fractions involves grasping the concept of fractional units. 

Consider two fractions: 1/5 and 1/9. 

A common mistake is to think that 1/9 is larger because 9 is greater than 5. 

However, fractions represent division—1/5 means dividing a roti among five children, while 1/9 means dividing it among nine.

Naturally, each child gets a smaller share when there are more people to share with, so 1/5 is larger than 1/9. This idea can be extended to understand that 1/100 is larger than 1/200, and so on.

Definition: When one unit is divided into several equal parts, each part is called a fractional unit. 

These are all fractional units: 1/2 , 1/3 , 1/4 , 1/5 , 1/6 , …, 1/10 , …, 1/50 , …, 1/100 , etc. 

We also sometimes refer to fractional units as ‘unit fractions.’ 

Fractional Units as Parts of a Whole

Fractions also describe parts of a whole. 

Imagine a whole chikki (a traditional Indian sweet) divided into six equal pieces. Even if the pieces are different shapes, they represent equal parts of the whole—each piece is 1/6 of the chikki. 

If a larger piece contains three 1/4 pieces, it is 3/4 of the whole chikki. This shows how different fractional units can represent parts of the same whole, regardless of shape or arrangement.

Measuring Using Fractional Units

Fractional units are also useful for measuring. 

For example, if you take a strip of paper as one unit and fold it into two equal parts, each part is ½ of the original strip. Folding it again creates four equal parts, each ¼ of the strip. Continuing this process, you can create fractional measurements like 1/8, 1/16, and so on. 

By combining these fractional units, you can describe larger quantities—2 times 1/4 equals 2/4 or 1/2, and 4 times 1/8 equals 4/8 or 1/2, demonstrating how fractions can be used to measure and describe parts of a whole.

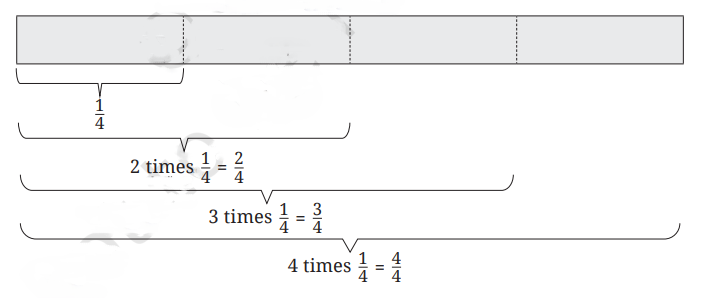
Marking Fraction Lengths on the Number Line

Marking fractions on a number line helps visualize their size. 

For example, the distance between 0 and 1 on a number line is one unit. If this distance is divided into two equal parts, each part is ½.

Similarly: Dividing the unit into three parts gives 1/3

Dividing into five parts gives 1/5, and so on.

Mixed Fractions

• Fractions greater than one

Mixed fractions, or mixed numbers, are fractions that are greater than one whole unit. 

In all the fractions that are less than 1 unit, the numerator is smaller than the denominator, while in the fractions that are more than 1 unit, the numerator is larger than the denominator.

For instance, 3/2 is greater than 1 and can be written as 

3/2 = 1/2 +1/2 +1/2 = 1 + 1/2

A mixed number consists of a whole number and a fraction less than 1.

            Similarly, 5/2  = 1/2 +1/2 +1/2 + 1/2 +1/2  = 2 + 1/2

• Writing fractions greater than one as mixed numbers

In above example , we saw 

3/2 = 1/2 +1/2 +1/2 = 1 + ½.

Similarly, 4/3 = 1/3 + 1/3 +1/3 +1/3  = 1 +1/3

A mixed number or mixed fraction contains a whole number (called the whole part) and a fraction that is less than 1 (called the fractional part).  

​

Equivalent Fractions

• Using a fraction wall to find equal fractional lengths!

Equivalent fractions are different fractions that represent the same value or portion of a whole. 

For example, 

These are ‘equivalent fractions’ that denote the same length, but they are expressed in terms of different fractional units.

• Understanding equivalent fractions using equal shares  

Example

​One roti was shared equally by four children. What fraction of the whole did each child get? 

The adjoining picture shows the division of a roti among four children. Fraction of roti each child got is 1/4 .

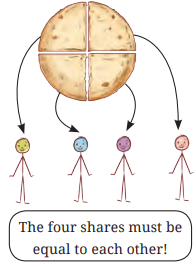
We can also express this event through division facts, addition facts, and multiplication facts. 

The division fact is 1 ÷ 4 = 1/4 . The addition fact is 1 = 1/4 + 1/4 + 1/4 + 1/4 . 

The multiplication fact is 1 = 4 × 1/4 . 

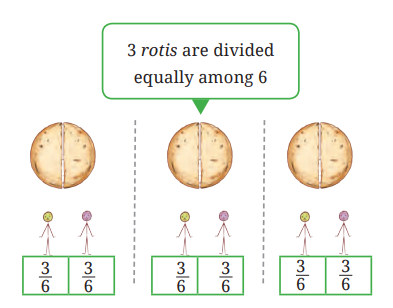
Fractions where the shares are equal are called ‘equivalent fractions’.

So, 1/2 and 3/6 are equivalent fractions.

• Expressing Fractions in Simplest Form

A fraction is in its simplest form when the numerator and denominator have no common factor other than 1. 

To simplify a fraction:

• Identify the common factors of the numerator and denominator.
• Divide both the numerator and denominator by their greatest common factor (GCF).

            Example: 

                    16/20 can be simplified by dividing both by 4: 16 ÷ 4 / 20 ÷ 4 = 4/5.

Now, there is no common factor between 4 and 5. Hence, 16/20 expressed in lowest terms is 4/5. So, 4/5 is called the simplest form of 16/20, since 4 and 5 have no common factor other than 1.

​Comparing Fractions

When comparing fractions like 4/5 and 7/9, it can be challenging to determine which one is greater. However, by converting these fractions to have the same denominator, we can easily compare them.

Convert 4/5 and 7/9 to equivalent fractions with a common denominator.

Let us see how we can use it:

4/5 = 4 x 9 / 5 x 9 = 36/45

7/9 = 7 x 5 / 9 x 5 = 35/45

Clearly, 36/45 > 35/45

We conclude that  4/5 > 7/9

Steps to Compare Fractions:

Step 1: Convert the fractions to equivalent fractions with the same denominator.

Step 2: Compare the numerators of these equivalent fractions to determine which fraction is greater.

Addition and Subtraction of Fractions

• Addition of Fractions 

    Example: 

If Meena ate 1/2 of a chikki and her brother ate 1/4 of it, together they ate: 1/2 + 1/4 =2/4 + 1/4 = 3/4

 So, they ate 3/4 of the chikki together.

• Adding fractions with the same fractional unit or denominator

              Example:

           Add 2/5 and 1/5 : 2/5 +1/5 = 3/5

• Adding fractions with different fractional units or denominators

             Example: 

           Add 1/4 and 1/3 : 1/4 +1/3

In this case, the common denominator can be made 3 × 4 = 12, i.e., we can find equivalent fractions with fractional unit 1/12.

1/4 = 1 x 3 /4 x 3 = 3/12

1/3 = 1 x 4/3 x 4 = 4/12

Now, 3/12  and 4/12 have the same fractional unit, i.e. 1/12.

Therefore, 1/4 +1/3 = 3/12 +4/12 = 7/12.

This method of addition, which works for adding any number of fractions, was first explicitly described in general by Brahmagupta in the year 628 CE! 

Brahmagupta’s Method For Adding Fractions

1. Find equivalent fractions so that the fractional unit is common for all fractions. This can be done by finding a common multiple of the denominators (e.g., the product of the denominators, or the smallest common multiple of the denominators). 

2. Add these equivalent fractions with the same fractional units. This can be done by adding the numerators and keeping the same denominator. 

3. Express the result in lowest terms if needed.

Example: 

Find the sum of 2/3 and 1/5.

The denominators of the given fractions are 3 and 5. The lowest common multiple of 3 and 5 is 15. Then we see that

2/3 = 2 × 5/3 x 5  = 10/15, 1/5 = 1 × 3 /5 × 3  = 3/15.

Therefore, 2/3 +1/5 = 10/15 + 3/15 = 13/15

• Subtraction of fractions with the same fractional unit or denominator

            Example: 6/7 – 4/7 = 2/7

• Subtraction of fractions with different fractional units or denominators

           Example: 3/4 – 2/3 

             3/4 = 3×3 /4 x 3 = 9/12 and similarly, 

             2/3 = 2 x 4 / 3 x 4 = 8/12.

Therefore,  3/4 – 2/3  = 9/12 – 8/12  = 1/12.

Brahmagupta’s method for subtracting two fractions

1. Convert the given fractions into equivalent fractions with the same fractional unit, i.e., the same denominator. 

2. Carry out the subtraction of fractions having the same fractional units. This can be done by subtracting the numerators and keeping the same denominator. 

3. Simplify the result into lowest terms if needed.

Puzzle!

It is easy to add up fractional units to obtain the sum 1, if one uses the same fractional unit, for example,

1/2 + 1/2 = 1, 1/3 + 1/3 + 1/3 = 1, 1/4 + 1/4 + 1/4 + 1/4 = 1, etc.