Lines and Angles – Complete Guide For Class 6 Math Chapter 2

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Lines and Angles in Mathematics for Class 6th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Lines and Angles introduces students to the fundamental concepts of geometry, which are essential for understanding more advanced topics. This chapter covers the basics, such as points, lines, rays, line segments, and angles, all of which form the foundation of plane geometry. Students learn how to identify and describe these geometric elements and explore the different types of angles, including acute, obtuse, right, straight, and reflex angles. The chapter also introduces methods for comparing and measuring angles using tools like protractors, as well as practical applications like creating rotating arms to visualize and compare angles. By mastering these basics, students gain the skills necessary to analyze and construct various geometric shapes, setting the stage for further exploration of geometry.

Lines and Angles

The fundamental concepts of geometry, including points, rays, line segments, lines, and angles, form the foundation for more advanced topics in geometry and help in the construction and analysis of various shapes.

Point

Imagine marking a dot on paper with the sharp tip of a pencil. This tiny dot represents a point in geometry. A point has no length, breadth, or height but signifies a precise location. Points are often labeled with capital letters such as A, B, or C. 

For instance, a dot labeled as Point A indicates a specific location on a plane.

Example: If you mark three points on a piece of paper, you may be required to distinguish these three points. 

For this purpose, each of the three points may be denoted by a single capital letter such as Z, P, and T. These points are read as ‘Point Z’, ‘Point P’, and ‘Point T’. Of course, the dots represent precise locations and must be imagined to be invisibly thin.

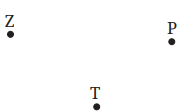
Line Segment

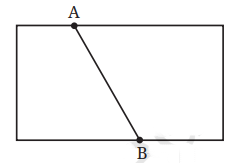
A line segment is the shortest path connecting two points, including both endpoints. If you mark two points, A and B, on a sheet of paper, the line connecting them is called the line segment AB (or BA). The points A and B are known as the endpoints of this line segment.

Line

A line extends infinitely in both directions. When you take a line segment AB and extend it beyond points A and B, it becomes a line. This line is represented as AB and can also be denoted by letters such as l or m. Any two distinct points determine a unique line that passes through both of them and extends endlessly.

Ray

As stated in the chapter Lines and Angles, a ray is a part of a line that starts at a specific point (the initial point) and continues infinitely in one direction. 

For example, a ray can be visualized as a beam of light from a lighthouse. 

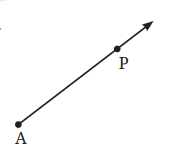
If you have a starting point A and another point P on the ray, it is denoted as ray AP and extends indefinitely from A through P.

Angle

According to the chapter Lines and Angles, an angle is formed by two rays that share a common starting point, known as the vertex of the angle. 

For instance, if rays BD and BE have B as their common starting point, an angle is formed. This angle can be named as ∠DBE or ∠EBD, with the vertex B always placed in the middle. 

The size of an angle is determined by the amount of rotation needed to move one ray to align with the other.

Real-life examples of angles include

The chapter Lines and Angles also covers a few real-life examples of angles including-

• The arms of a compass or divider form an angle where they are joined.

• The blades of a pair of scissors create an angle when opened.

• Spectacles and wallets also contain angles in their designs.

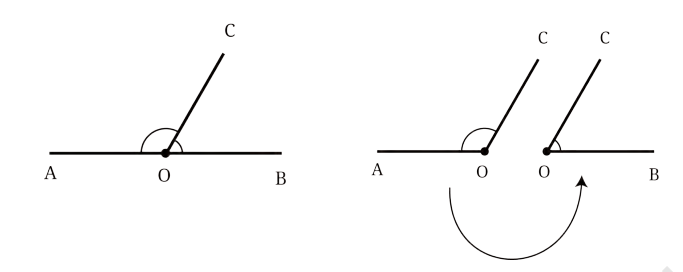
Comparing Angles by Superimposition

Comparing angles can be done through superimposition. Place one angle over another so that their vertices overlap. This method helps to see which angle is larger or smaller.

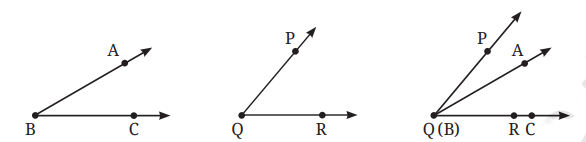
For instance, if we superimpose ∠PQR and ∠ABC, we can determine that ∠PQR is larger. 

Example

When comparing angles like ∠AOB and ∠XOY, if their arms and vertices align perfectly during superimposition, the angles are considered equal in size.

The corners of both of these angles match and the arms overlap with each other, i.e., OA ↔ OX and OB ↔ OY. So, the angles are equal in size.

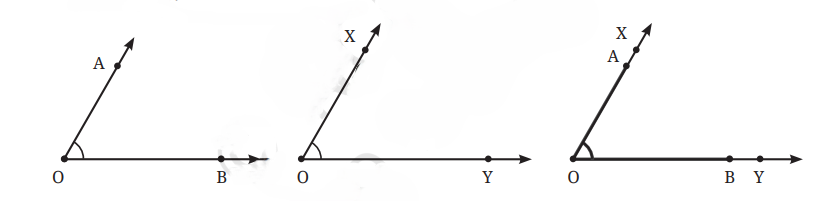
Comparing Angles without Superimposition

To compare angles without superimposition, you can use a transparent circular piece of paper. Place it on the angle with its center at the vertex and mark where the arms intersect the circle. Then, place the circle on another angle to see which is greater, equal, or smaller.

Let’s understand 

Let us place the circular paper on the angle made by the first crane. The circle is placed in such a way that its centre is on the vertex of the angle. Let us mark the points A and B on the edge circle at the points where the arms of the angle pass through the circle.

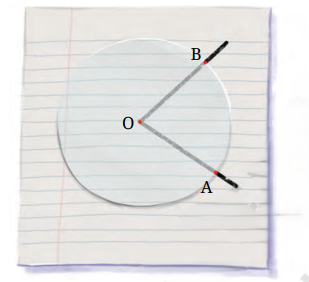
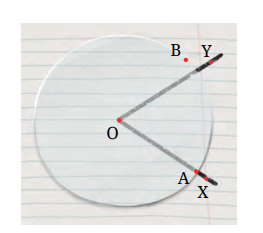
We can measure which crane makes a greater or smaller angle.

Exploring Angles: Rotating Arms and Special Types of Angles

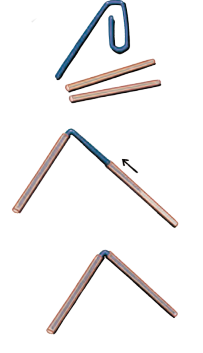
Making Rotating Arms

Activity: Let’s get hands-on with rotating arms using simple materials:

Materials Needed: Two paper straws, A paper clip

Steps to Create Rotating Arms

• Prepare the Materials: Take two paper straws and one paper clip.
• Assemble the Rotating Arm: Insert the paper straws into the arms of the paper clip. This creates a simple rotating arm mechanism.

Special Types of Angles

According to the chapter Lines and Angles, Angles can be categorized into various types based on their measurements. 

Let’s explore some special types:

1. Straight Angle:

A straight angle is formed when two rays or lines lie on a straight line. 

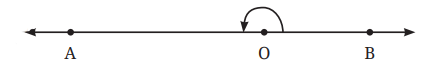
For example, when you open a book completely, the angle formed by the cover is a straight angle.

In a straight angle ∠AOB, if a ray OC is drawn, it divides the straight angle into two smaller angles, ∠AOC and ∠COB.

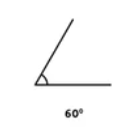
2. Acute Angle:

Acute angles are less than a right angle (less than a quarter turn). They are sharp and small, such as the angle between the hands of a clock at 10:10.

3. Obtuse Angle:

Obtuse angles are greater than a right angle but less than a straight angle (more than a quarter turn but less than a half turn). For instance, the angle made by a clock’s hands at 8:00 is an obtuse angle.

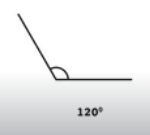
By understanding these special types of angles, you gain a clearer perspective on how angles vary and how they are classified based on their size.

Measuring Angles

Next in the chapter Lines And Angles, we’ll cover the concept of measuring angles. This involves-

Understanding Angle Measurement

To quantify how big an angle is, we use a unit called degrees (°). The idea is to divide a circle into 360 equal parts, each part being 1 degree. Here’s how you can understand angle measurements:

• Full Turn: A complete rotation around a circle is 360°, which is the measure of a full turn.
• Straight Angle: A straight line represents half a full turn, measuring 180°.
• Right Angle: A right angle, which forms a perfect corner, measures 90°. Two right angles make a straight angle.

Using a Protractor

According to the chapter Lines and Angles, a protractor is a tool designed to measure angles. It comes in two types:

• Full Protractor: A circle divided into 360 equal parts.
• Half Protractor: A semicircle divided into 180 equal parts.

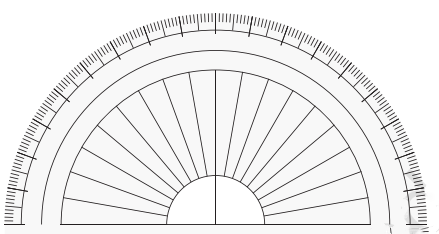
How to Read a Protractor

Further in the chapter Lines and Angles, we’ll cover how to read a protractor. It involves-

• Unlabelled Protractor: This protractor is divided into 180 units, but only part of the markings may be visible. Each long mark represents 10°, and each medium mark represents 5°.

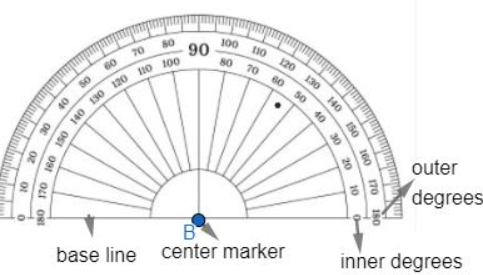
• Labelled Protractor: This one has two sets of numbers: one increasing from right to left and the other from left to right. This allows for easy reading of angles in either direction.

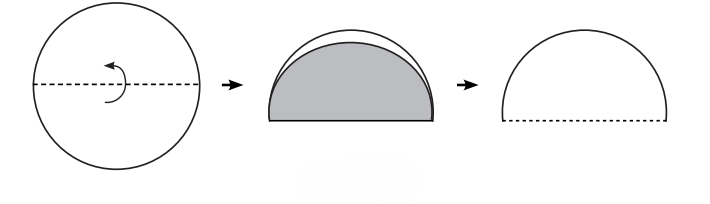
Creating Your Own Protractor

1. Draw and Cut: Draw a circle on paper and cut it out. Fold the circle to create a semicircle and mark the bottom right corner as ‘0°’.
2. Mark the Degrees: Use a ruler to evenly divide the semicircle into 180°.

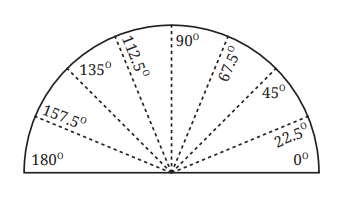
Common Mistakes with Protractors

• Ensure the protractor’s baseline is aligned correctly with the base of the angle.
• The center point of the protractor should be precisely on the vertex of the angle.

Now that we understand the common mistakes with protractors let’s go further in the chapter lines and angles and understand how to draw angles.

Drawing Angles

The chapter Lines and Angles also covers the concept of drawing angles which involves-

            Steps to Draw a 30° Angle:

1. Draw the Base Line: Start by drawing the base line, IN, for your angle.
2. Align the Protractor: Place the protractor’s center point on the vertex I and align the base line IN with the 0° line.
3. Mark the Angle: Count 30° from the 0° line on the protractor and mark this point as T.
4. Draw the Angle: Use a ruler to draw a line from I to T. The angle ∠TIN is now 30°.

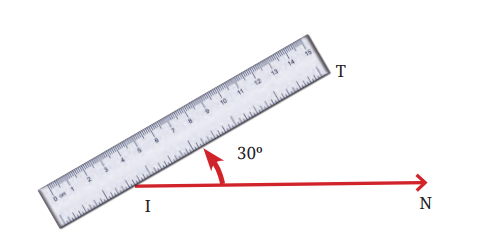
Types of Angles and Their Measures

The chapter lines and angles further cover types of angles and their measures. This includes-

1. Acute Angle

• Definition: An angle less than 90°.
• Measurement: Any angle between 0° and 90°.

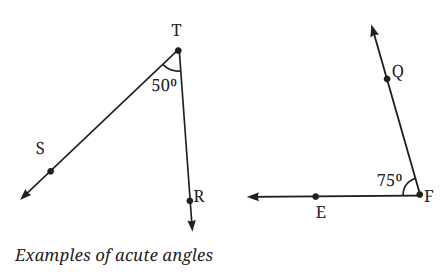
2. Obtuse Angle:

• Definition: An angle greater than 90° but less than 180°.
• Measurement: Any angle between 90° and 180°.

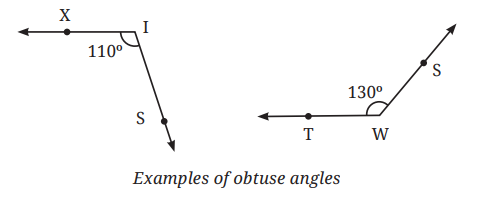
3. Reflex Angle:

• Definition: An angle greater than 180° but less than 360°.
• Measurement: Any angle between 180° and 360°.

By understanding these different types of angles and how to measure them, you gain a solid foundation in geometry. Practice measuring and drawing various angles to become proficient in this essential mathematical skill!

In conclusion, mastering the concepts in CBSE Class 6th Math, Chapter 2 – Lines and Angles provides students with the foundational knowledge of geometry. Understanding the different types of angles and how to measure and draw them equips students with skills that will be crucial in later mathematical studies. The concepts of points, lines, and angles introduced in Chapter 2 – Lines and Angles are not only important for academic success but also have practical applications in real-life scenarios. With iPrep Learning Super App’s engaging resources, students can practice and reinforce their understanding of Lines and Angles effectively. Make sure to revisit and practice the key concepts from Class 6th Math, Chapter 2 – Lines and Angles for a solid grasp of geometry!