Number Play – Complete Guide For Class 6 Math Chapter 3

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Number Play in Mathematics for Class 6th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The Chapter Number Play explores the intriguing world of numbers through various engaging activities. It highlights how numbers are used to organize our lives and solve problems, encouraging students to observe patterns and play with numbers in creative ways. The chapter covers concepts such as identifying numbers based on patterns, understanding supercells, exploring digit sums, palindromic numbers, and the famous Kaprekar’s magic number. It also introduces students to the Collatz Conjecture, a still-unsolved mathematical mystery, and emphasizes the importance of estimation and strategic thinking through games. This chapter is designed to make learning about numbers both fun and insightful.

Number Play

Numbers are everywhere. They help us organize our lives, solve problems, and understand the world around us. We’ve used numbers to count, add, subtract, multiply, and divide in our daily lives. But numbers are much more than that—they have patterns, mysteries, and games hidden within them. 

Numbers Can Tell Us Things

According to the chapter Number Play, numbers can communicate interesting things about the world. 

Imagine a group of children standing in a line in a park. Each child says a number. What do these numbers mean? Could their heights be playing a role?

• A child might say ‘1’ if only one taller child is standing next to them. 
• Another might say ‘2’ if both children next to them are taller. 
• A child says ‘0’ if neither of the children standing next to them is taller. 

In this way, each person says the number of taller neighbors they have. 

Numbers can tell us more than just a count—they can give us insight into relationships and arrangements.

Supercells: The Hidden Power of Numbers

Observe the numbers in the table below. 

Some numbers are colored—why?

43	79	75	63	10	29	28	34
200	577	626	345	790	694	109	198

A cell is colored if the number in it is larger than its adjacent cells. 

For example, 626 is colored because it is larger than 577 and 345. 

Similarly, 198 is colored as it is larger than the only adjacent cell, 109. 

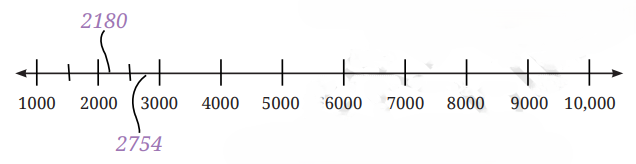
Patterns of Numbers on the Number Line

We are familiar with number lines, but according to the chapter Number Play, they can reveal surprising patterns when we place numbers on them. 

Let’s try placing these numbers on a number line: 

2180, 2754, 1500, 3600, 9950, 9590, 1050, 3050, 5030, 5300, and 8400.

Playing with Digits

We start writing numbers from 1, 2, 3, and so on. There are nine 1-digit numbers. But as stated in the chapter number play, how many numbers have two digits, three digits, four digits, and five digits?

• 1-digit numbers: 1–9 (9 numbers)
• 2-digit numbers: 10–99
• 3-digit numbers: 100–999
• 4-digit numbers: 1000–9999
• 5-digit numbers: 10000–99999

Digit Sums

Based on the chapter Number Play, when we add up the digits of certain numbers, the sum is the same. 

For example, the digit sum of 68 is the same as the digit sum of 176 or 545. 

 i.e.   6+8 = 14

        1+7+6 = 14

        5+4+5 = 14

Digit Detectives 

Further in the chapter number play, we’ll understand the concept of digit detectives. Let’s write numbers from 1 to 100, now understand how many times the digit ‘7’ appeared. 

• Counting the digit ‘7’ in the units place:

The numbers where ‘7’ appears in the units place are: 7, 17, 27, 37, 47, 57, 67, 77, 87, 97.

There are 10 numbers where ‘7’ appears in the units place.

• Counting the digit ‘7’ in the tens place:

The numbers where ‘7’ appears in the tens place are: 70, 71, 72, 73, 74, 75, 76, 77, 78, 79.

There are 10 numbers where ‘7’ appears in the tens place.

• Counting the digit ‘7’ in the number 77:

Note that the number 77 has ‘7’ in both the tens and units place, so it has been counted twice (once in the tens place and once in the units place). Now that we understand digit detectives lets understand another topic of the chapter number play named Total Count.

Total Count

Adding the appearances in both places gives us:

• 10 appearances in the units place
• 10 appearances in the tens place

So, the total number of times the digit ‘7’ appears from 1 to 100 is:

10+10=20 times10 + 10 = 20 times

Thus, the digit ‘7’ appears 20 times when writing the numbers from 1 to 100.

In the same way, we can find out the digit ‘7’ appeared among  the numbers 1–1000? The answers might surprise you!

Pretty Palindromic Patterns

What do these numbers have in common: 66, 848, 575, 797, 1111? They are palindromes, meaning they read the same from left to right and from right to left.

• All Palindromes Using 1, 2, 3: The numbers 121, 313, and 222 are some examples of palindromes using the digits ‘1’, ‘2’, and ‘3’. 
• Reverse-and-Add Palindromes: Start with a 2-digit number. Add this number to its reverse. If you get a palindrome, stop. If not, repeat the steps of reversing the digits and adding until you do.

For Instance, 48+84 = 132 +231 = 363

The Magic Number of Kaprekar

D.R. Kaprekar was a mathematics teacher who discovered a magical phenomenon with 4-digit numbers. Here’s how you can experience the magic yourself:

1. Pick any 4-digit number with at least two different digits, say 6382.
2. Make the largest number from these digits and call it A.
3. Make the smallest number from these digits and call it B.
4. Subtract B from A to get C .i.e C = A – B
5. Repeat the process with C.

You’ll notice that after a few steps, you will always reach the number 6174!, known as Kaprekar’s constant. No matter what 4-digit number you start with (as long as it has at least two different digits), you will eventually arrive at 6174!

Example

Take a 4-digit number like 3215. Rearranging to form the largest and smallest with these digits, we get 5321 and1235. Now, subtract them: 5321-1235 = 4086. Continue with the process of rearranging and subtracting:

• 8640−0468=8172
• 8721−1278=7443
• 7443−3447=3996
• 9963−3699=6264
• 6642−2466=4176
• 7641−1467=6174.

​Clock and Calendar Numbers

According to the chapter number play, on a 12-hour clock, certain times have interesting patterns, like 4:44, 10:10, and 12:21. 

Examples

• Manish’s birthday is on 20/12/2012, where the digits ‘2’, ‘0’, ‘1’, and ‘2’ repeat in that order. 
• Meghana’s birthday is on 11/02/2011, where the digits read the same from left to right and from right to left. 

Let’s understand

 Jeevan noticed that the calendar changes every year. But can a year’s calendar repeat after some years? Can you find out if any year’s calendar will match exactly with that of another year?

Yes, a year’s calendar can repeat after some years. The repetition of a calendar depends on two factors: the day of the week the year starts on and whether the year is a leap year or not.

When Does a Calendar Repeat?

• Same Day of the Week: For a calendar to repeat, the new year must start on the same day of the week.
• Same Type of Year: Both years must be either leap years or non-leap years.

General Rule

• A common year (non-leap year) calendar repeats after 6 or 11 years.
• A leap year calendar repeats every 28 years.

Mental Math

Fiurther in the chapter number play, we’ll be doing some mental math. Observe the figure below. What can you say about the numbers and the lines drawn? 

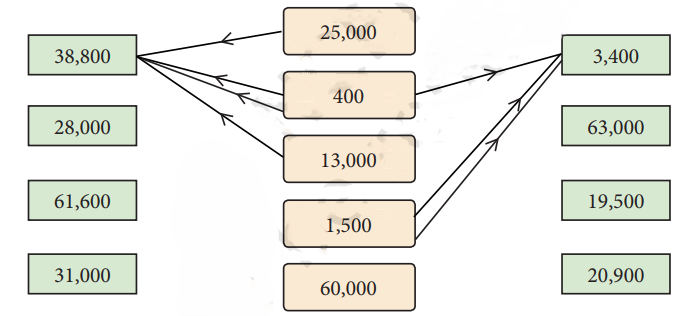
Numbers in the middle column are added in different ways to get the numbers on the sides. For example, 1500 + 1500 + 400 = 3400. The numbers in the middle can be used multiple times to achieve the desired sum. 

Two examples are given. It is simpler to do it mentally! 

• 38,800 = 25,000 + 400 × 2 + 13,000 
• 3400 = 1500 + 1500 + 400

Adding and Subtracting: Using the numbers in the boxes, you can use both addition and subtraction to get the required number. 

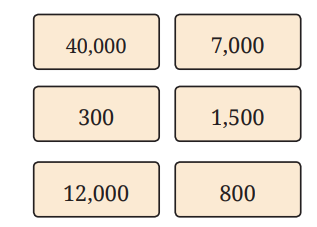
Example: 

39,800 = 40,000 – 800 + 300 + 300

Digits and Operations

• An example of adding two 5-digit numbers to get another 5-digit number is 12,350 + 24,545 = 36,895. 
• An example of subtracting two 5-digit numbers to get another 5-digit number is 48,952 – 24,547 = 24,405. 

Playing with Number Patterns

According to the chapter number play, numbers can form interesting patterns when arranged in specific ways. 

For example, adding numbers in certain figures can reveal a quicker method to find the sum rather than adding them one by one. 

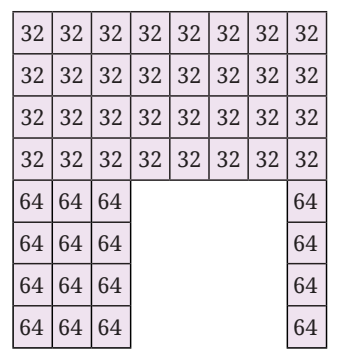
The pattern in the grid consists of two distinct numbers: 32 and 64.

• The number 32 occupies the majority of the grid, filling the top and left sections.
• The number 64 is located in a rectangular block at the bottom right and in a vertical column on the left side.

An Unsolved Mystery — The Collatz Conjecture!

As stated in the chapter number play, the Collatz Conjecture is a famous unsolved problem in mathematics. The rule is simple:

1. Start with any number.
2. If the number is even, take half of it.
3. If the number is odd, multiply it by 3 and add 1.
4. Repeat.

Eventually, all sequences will reach the number 1, regardless of the whole number you start with.

Examples: 

• 21, 64, 32, 16, 8, 4, 2, 1
•  22, 11, 34, 17, 52, 26, 13, 40, 20, 10, 5, 16, 8, 4, 2, 1

Simple Estimation

According to the chapter number play, Sometimes, an estimate is enough instead of an exact count. 

Example: 

Paromita’s class section has 32 children. The other 2 sections of her class have 29 and 35 children. So, she estimated the number of children in her class to be about 100. 

Along with Class 6, her school also has Classes 7–10 and each class has 3 sections each. She assumed a similar number in each class and estimated the number of students in her school to be around 500.

Games and Winning Strategies

Numbers can also be used to play games and develop winning strategies.

Game #1

• The first player says 1, 2, or 3.
• Players take turns adding 1, 2, or 3 to the previous number.
• The first player to reach 21 wins!

Play this game several times. Can you figure out the winning strategy? Which player can always win if they play correctly? There are many variations of this game

Game #2

• The first player says a number between 1 and 10.
• Players take turns adding a number between 1 and 10 to the previous number.
• The first player to reach 99 wins!

Try this variation of the game and discover the winning strategy. You can also create your own variations of these games. Decide how much one can add at each turn and what number is the winning number. Play your version several times and figure out the winning strategy. And that’s it for th chapter number play.

In conclusion, the chapter Number Play in Mathematics for Class 6th offers an exciting journey through the fascinating world of numbers. It allows students to explore patterns, play with digits, and dive into interesting mathematical mysteries like palindromes, Kaprekar’s magic number, and the Collatz Conjecture. Number Play encourages creative thinking and strategic problem-solving, making learning numbers fun and engaging. With its focus on activities and games, Number Play helps students see numbers as more than just tools for calculation but as gateways to understanding deeper mathematical concepts. Whether it’s estimation, digit sums, or playing with numbers on the number line, Number Play provides a comprehensive foundation for mastering mathematics.