Play With Patterns – Complete Guide For Class 4 Math Chapter 10

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Play with Patterns in Mathematics for Class 4th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.  

In this chapter named Play With Patterns, students will embark on a journey to discover the exciting world of patterns that exist all around them. Patterns can be found in everyday objects, nature, and even in art, making them a vital part of both mathematics and life. By recognizing and creating different types of patterns, learners will enhance their analytical skills and creativity. Through engaging activities and examples, they’ll see how patterns can be simple or complex, and how they play a crucial role in understanding mathematical concepts. Now, let’s understand what “Play with Patterns” actually means!

What is Play With Patterns?

“Play with Patterns” refers to the exploration and creation of various sequences and arrangements in numbers, shapes, and letters, helping students understand the concept of repetition and organization in mathematics. Students are introduced to the world of patterns interestingly and collaboratively in the chapter “Play with Patterns”. It covers numerous forms of patterns seen in numbers, shapes, and common items, helping youngsters understand the concepts of recurring and sequences.

The first thing that students do is study repeating patterns, which are collections of forms, colors, or numbers that are repeated in a specific order. Their capacity to predict what will happen next in the sequence grows as they discover and expand upon these patterns.

Growing patterns, in which a pattern’s steps are increasingly larger or smaller, are also covered in the chapter Play With Patterns. Children get an understanding of how patterns change over time and how mathematical concepts like addition and multiplication can be represented by them.

Learning to design one’s patterns is a key component of the chapter Play with Patterns. Students are encouraged to create patterns utilizing objects, numbers, or designs through exercises and activities, which strengthens their ability to think clearly and creatively.

The chapter also discusses mirror images and symmetry, where students investigate how patterns or objects might be mirrored while still seeming balanced. They are exposed to the concept of geometric symmetry through this.

By the end of the chapter Play with Patterns, students have a solid foundation on how patterns function in the real world, which improves their capacity for observation and develops an expanded understanding of mathematical ideas.

In Chapter 10, “Play with Patterns,” students explore various types of patterns that they encounter in their everyday lives. Patterns are an integral part of mathematics and help in understanding repetition, sequence, symmetry, and organization. The chapter teaches students how to recognize, create, and extend patterns using numbers, shapes, alphabets, and tiling designs. Let’s break down the important terms in this chapter and provide related examples to enhance understanding.

Let’s Play with Patterns

The concept of patterns lies at the center of the chapter Play with Patterns. A recurring arrangement of shapes, numbers, colors, objects, or designs is called a pattern. Patterns might be simple or complicated, but they are always predictable since they follow a set of rules or steps.

Example: Imagine you have a series of colors: red, blue, red, blue. This is a simple color pattern where red and blue repeat alternately. The next color in this sequence will be red, following the same rule.

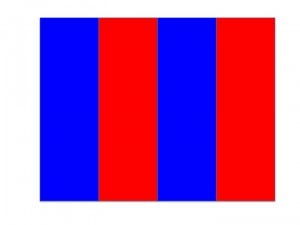
Block Patterns

Block patterns are composed of shapes or blocks that are repeated in a particular order. Children may be asked to make their designs with various blocks or to figure out what follows next in a sequence of blocks.

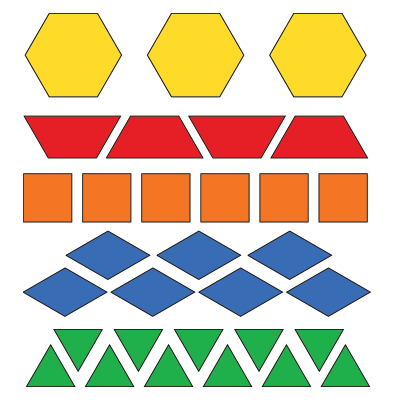
Example: Consider a row of blocks arranged as follows: square, circle, square, circle. The next block in this sequence will be a square because the pattern repeats. Block patterns can be extended to more complex sequences using different shapes, sizes, or colors.

This concept is important because it allows children to develop spatial reasoning and understand repetition in physical objects.

Number Patterns

Number patterns are collections of numbers arranged according to a specific guideline. These patterns teach students how addition, subtraction, and multiplication may be used to organize numbers in an ordered fashion.

• Increasing Number Patterns: These are patterns where each number is larger than the previous one. For example, if the pattern is 2, 4, 6, 8, 10, the rule is that each number is increasing by 2. The next number will be 12.

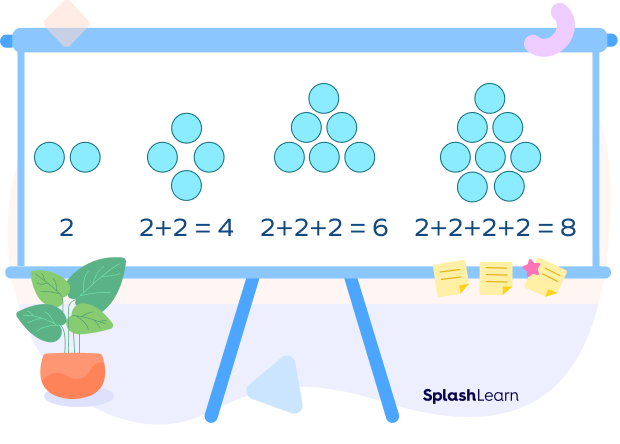
• Decreasing Number Patterns: These are patterns where each number is smaller than the previous one. For instance, in the patterns 20, 18, 16, and 14, the rule is that 2 is subtracted each time, so the next number will be 12.

• Multiplication-Based Number Patterns: Here, numbers increase based on multiplication. For example, 2, 4, 8, and 16 follow the rule of multiplying by 2. The next number will be 32.

Number patterns form the foundation of algebraic thinking, teaching students how to identify and follow mathematical rules.

Alphabet Patterns

In Alphabet Patterns, letters are placed in a particular sequence, often following the order of the alphabet or uniformly skipping letters.

Example: A pattern could be A, B, C, D, or E. In this case, the pattern follows the natural order of the alphabet. However, you can also create patterns like A, C, E, and G, where each letter skips one.

Such patterns help children learn alphabetical order and improve their ability to see sequences in non-numerical contexts.

Alphabet and Number Patterns

In alphabet and number patterns, letters and numbers are combined in a recurring order. Students must be able to identify the relationship between two distinct kinds of sequences to follow these patterns.

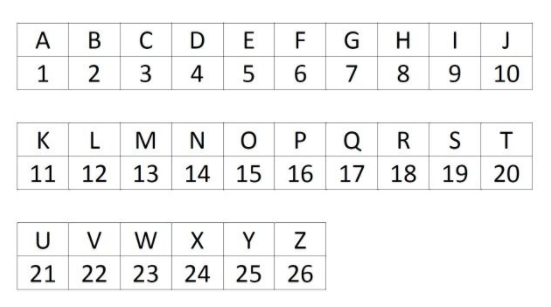
Example: A common example of this pattern is A1, B2, C3, D4, and E5, where both the letter and number are increasing by one. Alternatively, you can have patterns like A1, B3, and C5, where the numbers increase by two while the letters follow the regular alphabetical order.

This blending of alphabets and numbers enhances cognitive flexibility as children learn to track two types of sequences simultaneously.

Tiling Patterns

Repetitive shapes that seamlessly blend are known as tiling patterns. This idea is frequently seen in walls, floor tiles, and other artwork. The idea behind it is that you can utilize simple shapes like squares, triangles, or hexagons to create beautiful and intricate designs through repetition.

Example: A classic tiling pattern might involve squares arranged in a grid or triangles fitting together to create a hexagonal pattern. Students can explore different combinations of shapes that can tile a plane, such as using triangles to form hexagons or squares to create a checkerboard pattern.

Tiling patterns are important for understanding concepts of symmetry, spatial reasoning, and geometry.

Related Examples and Activities

There are various related examples and activities in the chapter Play with Patterns. These include-

1. Creating Block Patterns: Ask students to create a pattern using different colored blocks. They can start with a simple sequence, such as red, blue, red, and blue, and then increase the complexity by adding more colors or shapes, like red, blue, yellow, red, blue, and yellow.
2. Extending Number Patterns: Provide students with an incomplete sequence of numbers, such as 5, 10, 15, __, __, and ask them to identify the rule (adding 5) and extend the pattern to 20 and 25.
3. Alphabet Skip Patterns: Write a pattern like A, C, E, __, __, and ask students to fill in the blanks by identifying that the letters are skipping one each time. They should add G and I to the pattern.
4. Creating Tiling Designs: Provide students with triangular, square, and hexagonal shapes and ask them to create a tiling pattern. They can explore how these shapes fit together and try different combinations.

Importance of Patterns in Mathematics

Patterns are essential to mathematical thinking because they promote observation, reasoning, and prediction skills. Students can have a deeper comprehension of mathematical concepts like multiplication, division, and geometry by learning patterns.

In real life, patterns are also frequently utilized in art, architecture, and the natural world (see the symmetry in snowflakes or the spirals in sunflowers, for example). Students have greater awareness of their surroundings and can apply mathematical concepts in real-world scenarios by learning to recognize patterns.

Let’s Conclude

In conclusion, Chapter 10 – Play with Patterns not only introduces students to the fascinating world of patterns but also emphasizes their significance in mathematics and daily life. By exploring various types of patterns—whether in numbers, shapes, or even letters—students enhance their observational skills and develop critical thinking abilities essential for their academic journey. The engaging activities and examples in Play with Patterns encourage learners to create, extend, and analyze patterns, fostering a deeper understanding of mathematical concepts.

Ultimately, this chapter serves as a stepping stone for students, helping them recognize the role of patterns in the world around them and preparing them for more complex mathematical ideas in the future. As they continue their learning, the skills acquired from CBSE Class 4 Math Chapter 10 – Play with Patterns will be invaluable in their educational growth.