Inverse Trigonometric Functions – Complete Guide For Class 12 Math Chapter 2
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Inverse Trigonometric Functions in Mathematics for Class 12th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
The chapter “Inverse Trigonometric Functions” in Class 12th Math explores the concept of inverse functions applied to trigonometric functions. Students learn to define and use the inverses of the six trigonometric functions: sine, cosine, tangent, cosecant, secant, and cotangent. The chapter covers how to determine the domain and range of these inverse functions and how to solve equations involving them. Key concepts include the principal values of inverse trigonometric functions and their properties. Understanding these functions is essential for solving trigonometric equations and for applications in calculus and analytical geometry.
Inverse Trigonometric Functions
Inverse trigonometric functions are the inverses of the basic trigonometric functions (sine, cosine, tangent, cosecant, secant, and cotangent). They are used to find the angle that corresponds to a given trigonometric value.
Invertibility of Functions
A function is invertible if and only if it is both one-to-one (injective) and onto (surjective). In such cases, the inverse function, denoted by
𝑓⁻¹, exists. The domain of 𝑓⁻¹is the range of 𝑓, and the range of 𝑓⁻¹ is the domain of 𝑓
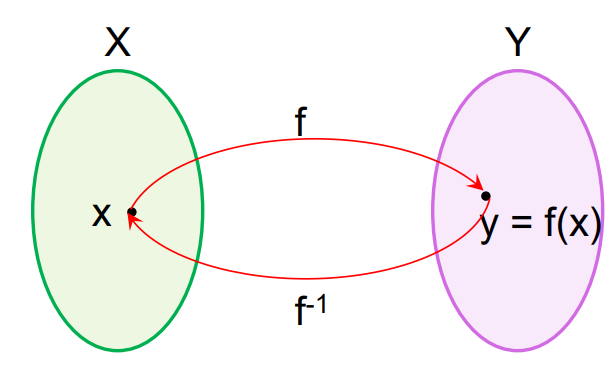
Graph of Inverse Functions
The graph of an inverse function can be obtained from the graph of the original function by reflecting it across the line y=x.
Inverse of Trigonometric Functions
Trigonometric functions such as sine, cosine, and tangent are periodic, which means they are not one-to-one over their entire domains. However, by restricting their domains, they can be made bijections, allowing us to define their inverse functions.
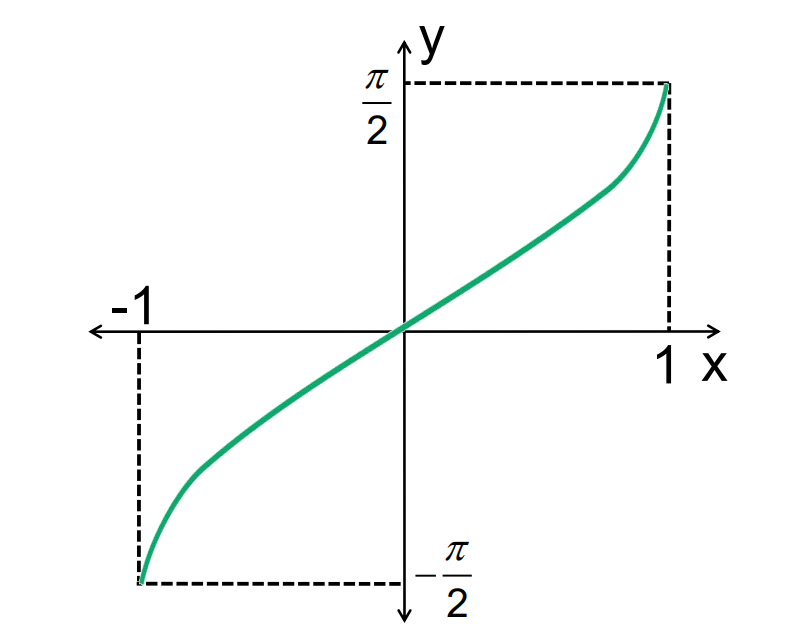
- Inverse Sine Function
The function y = sin(x) with domain [− π /2, π /2] and range [−1,1] has an inverse function called the inverse sine function or arcsine, denoted by sin⁻¹ (x). 𝑦 = sin⁻¹ (x) if and only if x = sin(y) and y ∈ [− π /2, π /2 ]
The principal value of sin⁻¹ (x)
The branch of the function sin⁻¹ (x) with range [− π /2, π /2 ] is called the principal value branch. The value of the inverse sine function within this interval is referred to as the principal value.
Example:
Find the principal value of sin⁻¹ (1/2)
Solution:
Let sin⁻¹ (1/2) = y. Then, sin(y) = 1/2
Since the range of the principal value branch of sin⁻¹ (x) is [ −𝜋/2, 𝜋/2], and sin (𝜋/6) = 1/2. The principal value of sin⁻¹ (1/2) is 𝜋/6.
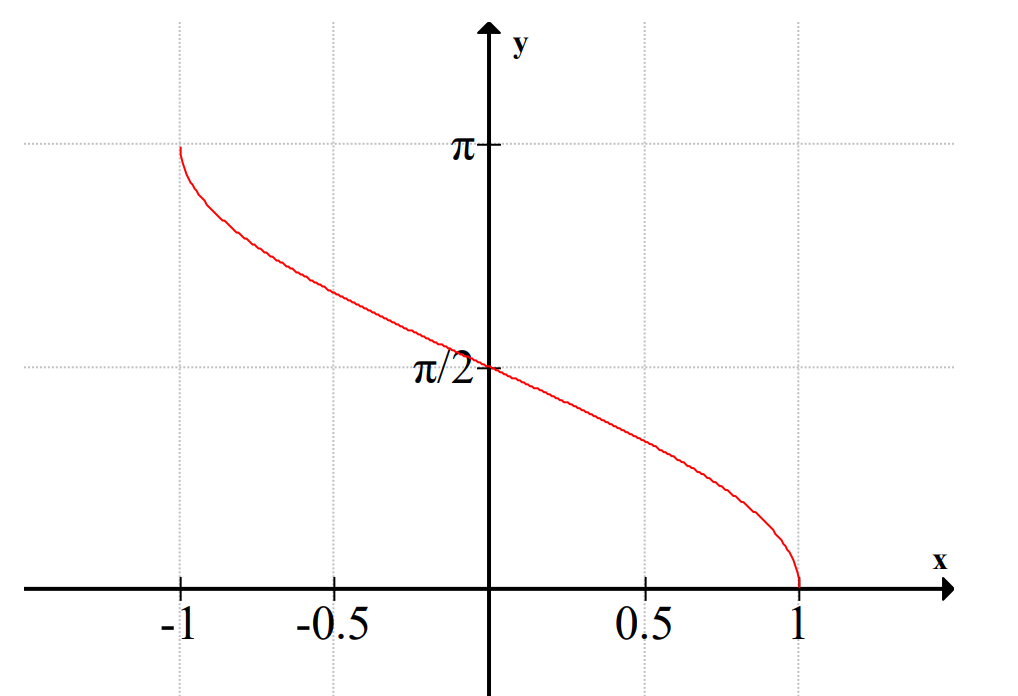
Inverse Cosine Function
Consider the cosine function f(x) = cos(x). Its domain is the set of all real numbers, and its range is [−1,1]. By restricting the domain to [0,π], the function becomes one-to-one and thus invertible, giving us the inverse cosine function or arccosine, denoted by cos⁻¹(x).
𝑦 = cos⁻¹(x) if and only if x = cos(y) and y∈[0,π]
The principal value of cos⁻¹(x)
The branch of cos⁻¹(x) with range [0,π] is called the principal value branch.
Example:
Find the principal value of cos⁻¹(√3/2)
Solution:
Let cos⁻¹(√3/2) = 𝑦. Then, cos(y)= √3/2.
Since the range of the principal value branch of cos⁻¹(x) is [0,π], and cos (𝜋/6) = √3/2. The principal value of cos⁻¹(√3/2) is 𝜋/6.
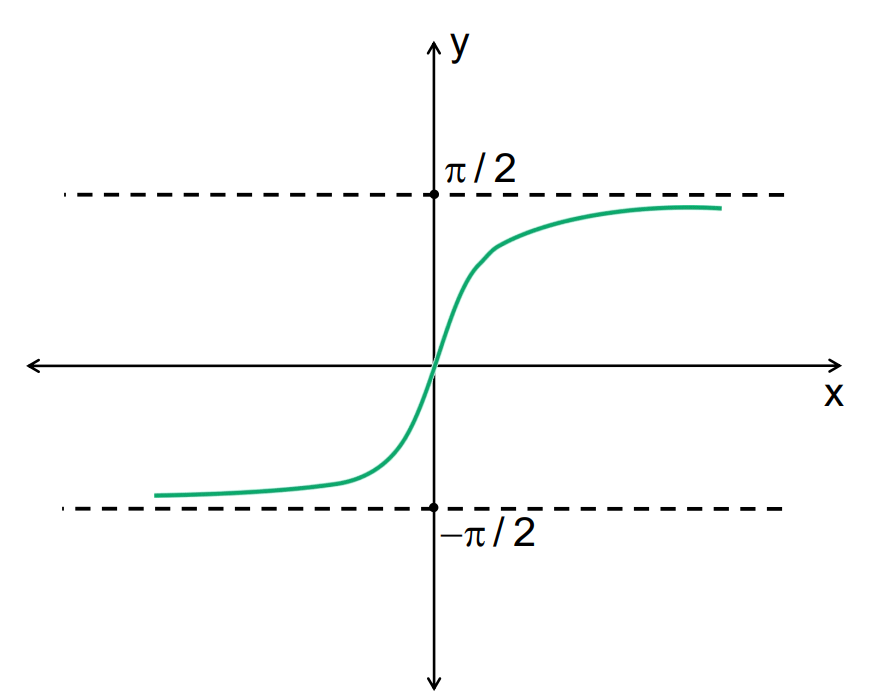
Inverse Tangent Function
The tangent function f(x) = tan(x) has a domain of {x: x ∈ R, x ≠ (2n+1)π/2, n ∈ Z} and a range of R. By restricting the domain to (−𝜋/2, 𝜋/2), the function becomes one-to-one, giving us the inverse tangent function or arctangent, denoted by tan⁻¹ (x).
𝑦 = tan⁻¹ (x) if and only if x = tan(y) and y ∈ (− π/2, π/2 )
The principal value of tan⁻¹ (x)
The principal value branch of tan⁻¹ (x) has a range of (−𝜋/2, 𝜋/2)

Example: Find the principal value of tan⁻¹ (-√3)
Solution:
Let tan⁻¹(-√3) = y. Then, tan(y) = − √3.
Since the range of the principal value branch of tan⁻¹(x) is (−𝜋/2, 𝜋/2), and
tan (− 𝜋/3) = −√3, The principal value of tan⁻¹(-√3) is − 𝜋/3.
Inverse Cotangent Function
The cotangent function f(x) = cot(x) has a domain of {x: x ∈ R, x ≠ nπ, n ∈ Z} and a range of R. By restricting the domain to (0,π), the function becomes one-to-one, allowing us to define the inverse cotangent function or arccotangent, denoted by cot⁻¹(x).
y = cot⁻¹(x) if and only if x = cot(y) and y ∈ (0,π)
The principal value of cot⁻¹ (x)
The principal value branch of cot⁻¹(x) has a range of (0,π).
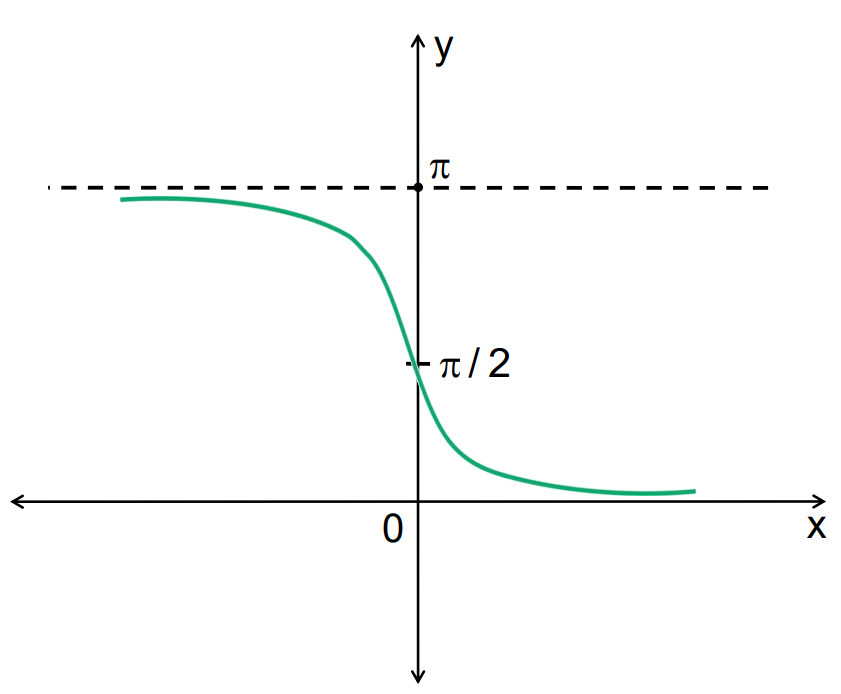
Example:
Find the principal value of cot⁻¹ (√3).
Solution:
Let cot⁻¹ (√3) = y. Then, cot(y) = √3.
Since the range of the principal value branch of cot⁻¹ (x) is (0,π), and cot (𝜋/6) = √3,
The principal value of cot⁻¹ (√3) is 𝜋/6
Inverse Secant Function
The secant function f(x) = sec(x) has a domain of 𝑅−{x: x = (2n+1)π/2, n ∈ Z} and a range of R−(−1,1). By restricting the domain to [0,π]−{ π/2}, the function becomes one-to-one, leading to the inverse secant function or arcsecant, denoted by sec⁻¹(x).
y=sec⁻¹ (x) if and only if x = sec(y) and y ∈ [0,π]−{π/2}
The principal value of sec⁻¹ (x)
The principal value branch of sec⁻¹ (x) has a range of [0,π]−{π/2]
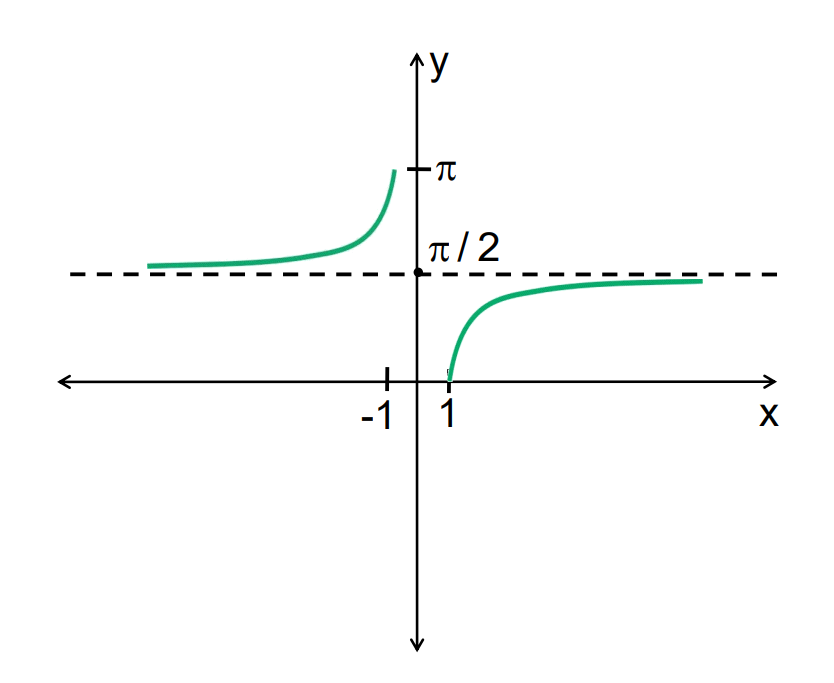
Example:
Find the principal value of sec⁻¹(2/√3).
Solution: Let sec⁻¹(2/√3) = y. Then sec y = 2 /√3.
The range of the principal value branch of sec⁻¹ is 0,π]−{π/2} and sec (π/6) = 2 /√3.
Therefore, The principal value of sec⁻¹(2/√3) is π/6.
Inverse Cosecant Function
The inverse cosecant function, denoted as cosec⁻¹(x), is defined for x in the ranges where cosec (x) is valid. The range of the principal value branch of cosec⁻¹(x) is given by [−𝜋/2, 𝜋/2] – {0}
Principal value of cosec⁻¹(x): The principal value of cosec⁻¹(x) is the value that lies within this interval.

Example: Find the principal value of cosec⁻¹(2).
Solution:
Let cosec⁻¹(2) = y. Then, cosec (y) = 2.
The range of the principal value branch for cosec⁻¹(x) is [− 𝜋/2, 𝜋/2] – {0}.
Thus, the principal value of cosec⁻¹(2) is 𝜋/6.
Table of Domains and Ranges of Inverse Trigonometric Functions
| Inverse Function | Domain | Range |
| sin⁻¹ | [-1, 1] | [− 𝜋/2, 𝜋/2] |
| cos⁻¹ | [-1, 1] | [0, 𝜋] |
| tan⁻¹ | R | [− 𝜋/2, 𝜋/2] |
| cosec⁻¹ | R – (-1, 1) | [− 𝜋/2, 𝜋/2] – {0} |
| sec⁻¹ | R – (-1, 1) | [0, 𝜋] – {𝜋/2} |
| cot⁻¹ | R | (0, 𝜋) |
Properties of Inverse Trigonometric Functions:
Property I:
(i) sin(sin⁻¹x) = x, for x∈[−1,1]
(ii) cos(cos⁻¹x) = x, for x∈[−1,1]
(iii) tan(tan⁻¹x) = x, for x∈R
(iv) cosec(cosec⁻¹x) = x, for x∈(−∞,−1]∪[1,∞)
(v) sec(sec⁻¹x) = x, for x∈(−∞,−1]∪[1,∞)
(vi) cot(cot⁻¹x) = x, for x∈R
Property II:
(i) sin⁻¹(sinx) = x, for x∈[− π/2, π/2]
(ii) cos⁻¹(cosx) = x, for x∈[0,π]
(iii) tan⁻¹(tanx) = x, for x∈(− π/2, π/2)
(iv) cosec⁻¹(cosecx) = x, for x∈[−π/2, π/2] -{0}
(v) sec⁻¹(secx) = x, for x∈[0,π] – {π/2}
(vi) cot⁻¹(cotx) = x, for x∈(0,π)
Property III:
(i) sin⁻¹(1/x) = cosec⁻¹x, for x≤−1 or x≥1
(ii) cos⁻¹(1/x) = sec⁻¹x, for x≤−1 or x≥1
(iii) tan⁻¹(1/x) = cot⁻¹x, for x>0
(iv) tan⁻¹(1/x) = −π+cot⁻¹x, for x<0
Property IV:
(i) sin⁻¹(−x) = −sin⁻¹ x, for x∈[−1,1]
(ii) tan⁻¹(−x) = −tan⁻¹x, for x∈R
(iii) cosec⁻¹(−x) = −cosec⁻¹x, for x≥1
Property V:
(i) cos⁻¹(−x) = π−cos⁻¹x, for x∈[−1,1]
(ii) sec⁻¹(−x)= π−sec⁻¹x, for x≥1
(iii) cot⁻¹(−x) = π−cot⁻¹x, for x∈R
Property VI:
(i) sin⁻¹x + cos⁻¹x = π/2, for x∈[−1,1]
(ii) tan⁻¹x + cot⁻¹x = π/2, for x∈R
(iii) cosec⁻¹x + sec⁻¹x = π/2, for x≥1
Property VII:
(i) tan⁻¹x + tan⁻¹y = tan⁻¹(1−xy/x+y), for xy<1
(ii) tan⁻¹x − tan⁻¹y = tan⁻¹(1+xy/x−y), for xy>−1
Property VIII:
(i) 2tan⁻¹x = sin⁻¹(2x/1+x²), for x≤1
(ii) 2tan⁻¹x = cos⁻¹(1 + x²/ 1−x²), for x≥0
(iii) 2tan⁻¹x = tan⁻¹((2x/1-x²), for −1<x<1
Example:
Express tan⁻¹ (√1+x² -1/x) in the simplest form.
Solution: Let x = tan y. Then y = tan⁻¹x with −π/2 < y < π/2.
Therefore, tan⁻¹ (√1+x² -1/x) = tan⁻¹ (√1+tan² y -1/tan y) = tan⁻¹ (sec y – 1/tan y) = tan⁻¹(1 – cos y/sin y) = tan⁻¹(2 sin² y/2 / 2 sin y/2 cos y/2)
= tan⁻¹ (tan y/2) = y/2 = 1/2 tan⁻¹x.
Let’s Conclude
In conclusion, Chapter 2 – Inverse Trigonometric Functions in CBSE Class 12th Math plays a crucial role in developing a deeper understanding of trigonometry and its inverse operations. Mastering the properties, domain, and range of inverse trigonometric functions equips students with the tools needed to solve complex mathematical problems, especially in calculus and analytical geometry. With iPrep’s engaging learning resources, including videos and practice questions, students can strengthen their grasp of Inverse Trigonometric Functions and confidently tackle exam challenges. Don’t miss the opportunity to explore every aspect of Inverse Trigonometric Functions and enhance your math skills with ease!
Practice questions on Chapter 2 - Inverse Trigonometric Functions
Get your free Chapter 2 - Inverse Trigonometric Functions practice quiz of 20+ questions & detailed solutions
Practice Now








