Application of Derivatives – Complete Guide For Class 12 Math Chapter 6

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Application of Derivatives in Mathematics for Class 12th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Applications of Derivatives explores the practical uses of derivatives in various fields. It covers essential concepts such as finding the rate of change of quantities, determining the equations of tangents and normals to curves, and identifying turning points in a graph to locate maximum and minimum values of functions. The chapter also delves into the analysis of intervals where functions are increasing or decreasing, and it introduces the use of derivatives to approximate values of certain quantities. Overall, this chapter highlights the powerful tools that derivatives provide in analyzing and solving real-world problems, making it a crucial part of higher mathematics.

Application of Derivatives

The chapter on applications of derivatives gives a thorough understanding of the various scenarios where derivatives can be applied including-

• To determine the rate of change in quantities  
• To find the equation of tangent and normal to a curve at a point.  
• To find turning points on the graph of a function which in turn will help us to locate points at which the largest or smallest value(locally) of a function occurs.  
• To find intervals on which a function is increasing or decreasing.  
• To find the approximate value of certain quantities. Introduction 

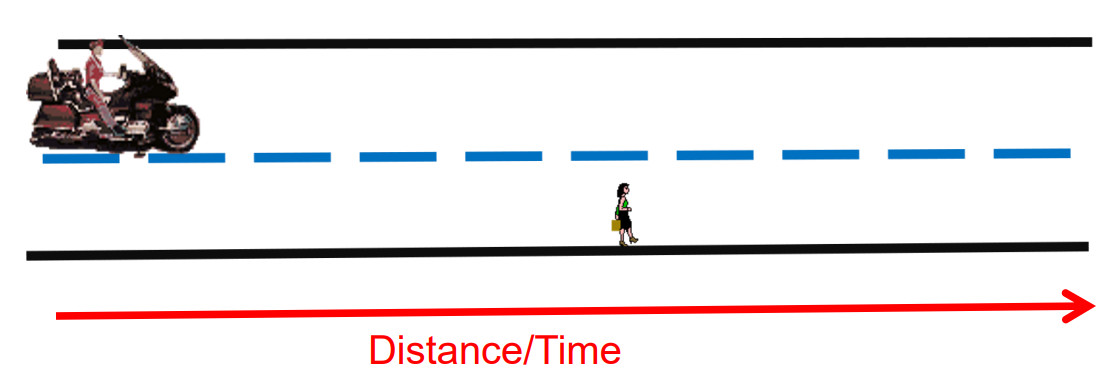
Application of derivatives is used to represent and interpret the rate at which one quantity/Variable, distance (say) changes concerning another variable, time (say). Most of the changes are considered in terms of independent variable time, as shown in the above case. But there is no restriction that the changes are considered concerning time only. 

Derivative as a Rate Measure

The chapter Application of Derivatives also covers the concept of a derivative as a measure. Let’s see how-

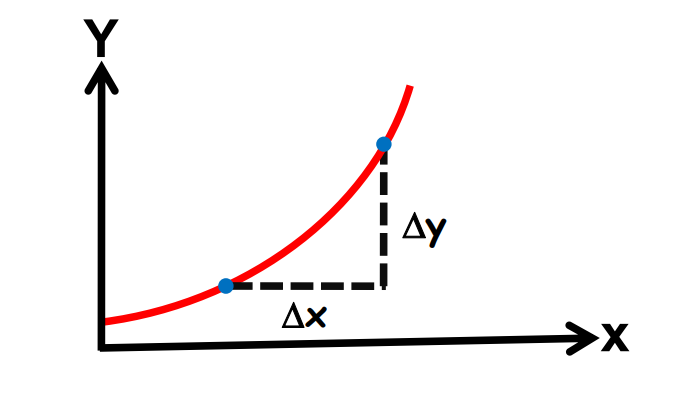
• Δy/Δx → Average rate of change of y w.r.t. x 
• dx/dy → Instantaneous rate of change of y w.r.t. x 

If one quantity y varies with another quantity x,  satisfying some rule y = f(x), then dy/dx represents the rate of change of y concerning x. 

At x = a, f´(a) represents the rate of change of y concerning x at x=a. 

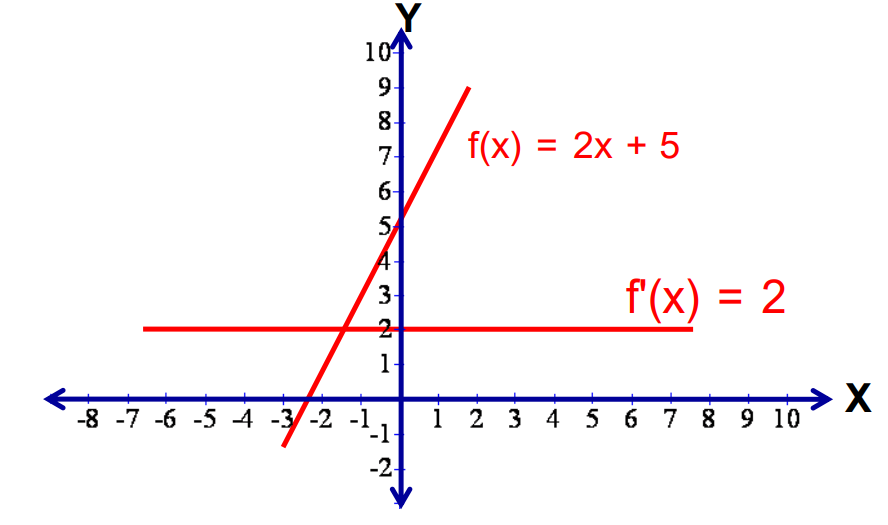
F (x) is the same at all points of the function. 

The rate of change of function is the same at all points. 

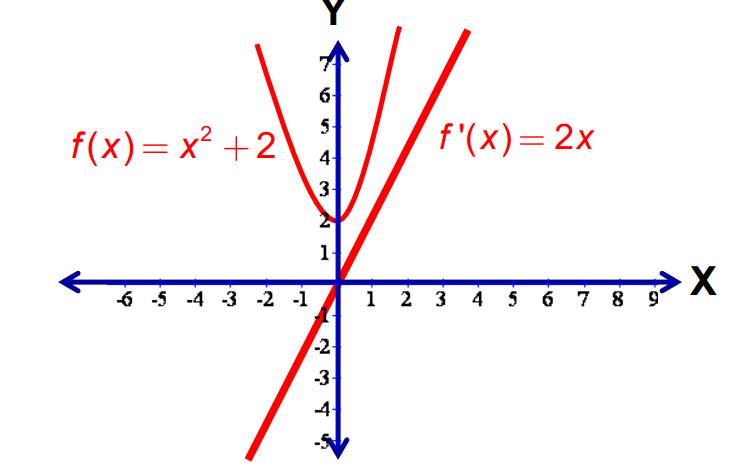
f ‘(x)is different at all points of the function. 

The rate of change of function is different at all points. 

Point to be remembered

As stated in the chapter Application of Derivatives, If two variables x and y vary concerning another variable (say t) i.e. If y = f(t) and x = g(t), 

then dy/dx  = dy/dt /dx/dt, provided that dx/dt ≠ 0

Remarks :  

• If y increases as x increases, then dy/dx is positive.  
• If y decreases as x increases, then dy/dx is negative.   

Example: Find the rate of change of the area of the circle concerning its radius r when r = 5 cm. 

Solution: A = (π) × r²

                  dA/dr = 2π x r

                 dA/dr = 2π x 5

                 dA/dr = 10 π 10 cm²/cm

Hence, the area of the circle is changing at the rate of 10 cm²/cm concerning radius. 

Increasing Function  

As stated in the chapter Application of Derivatives, there are a few points to be considered for an increasing function. These include-

• A function is said to be increasing on an interval ( a, b) if x₁ < x₂ ⇒ f(x₁) ≤ f (x₂) for all x₁, x₂ ∈(a, b ) 
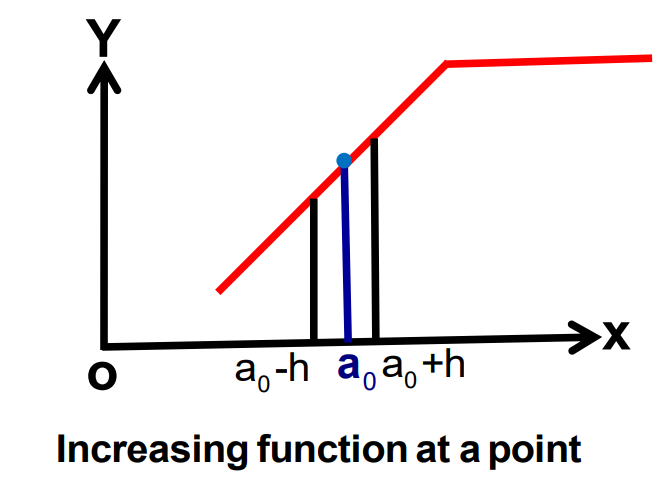
• A function is said to be increasing at a point if there exists an interval (a₀ – h, a₀ + h), h > 0

            x₁ < x₂ ⇒ f(x₁)≤ f (x₂) for all x₁, x₂ ∈ (a₀ – h , a₀ + h)

Strictly increasing function 

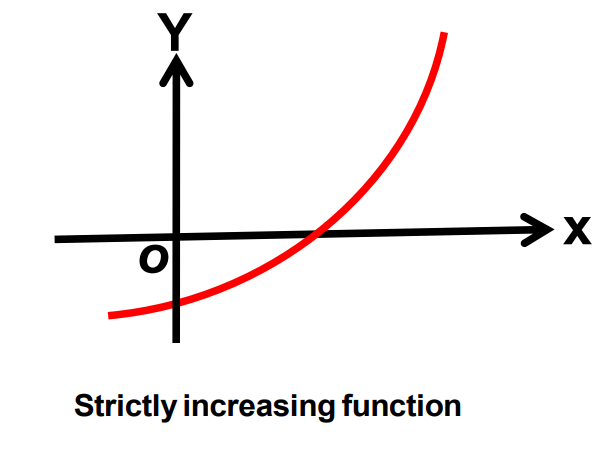
A function f(x) is said to be strictly increasing in an interval I, if

x₁ < x₂ ⇒ f(x₁) < f (x₂) for all x₁,x₂ ∈ I

Remember: If a function f(x) is continuous on [a,b] and differentiable on (a, b), then f(x) is strictly increasing in (a, b) if f´(x) > 0 for all x in (a, b). 

Example: 

Show that the function given by f(x) = 5x – 2 is strictly increasing on R.

Solution:  Let x₁ and x₂ be any two numbers in R. 

Such that x₁ < x₂. Then

⇒ 5x₁ < 5x₂

⇒5x₁ – 2 < 5x₂ – 2

⇒ f(x₁) < f(x₂)

Therefore, f is strictly increasing on R

Decreasing Function

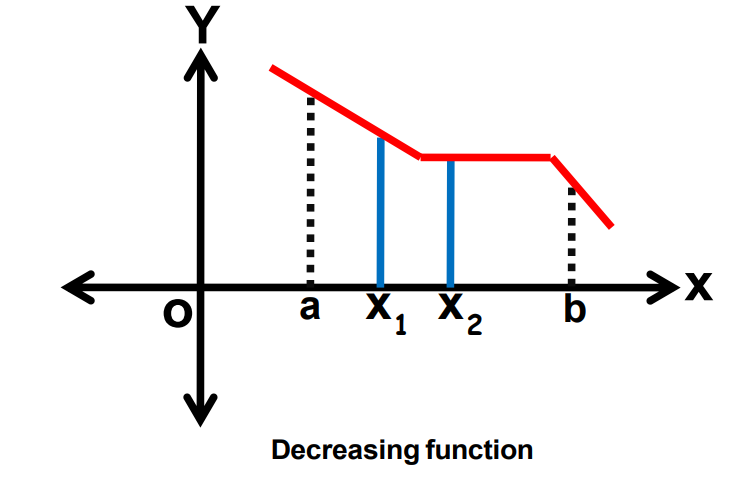
A function is said to be decreasing on an interval (a, b) if 

x₁ < x₂ ⇒ f(x₁) ≥ f (x₂) for all x₁,x₂ ∈ (a, b)

Example: 

Show that the function is an increasing function f(x) = 9x + 2

Method 1: x₁ < x₂ 

⇒ 9x₁ < 9x₂

⇒9x₁ + 2 < 9x₂ + 2

⇒ f(x₁) < f(x₂)

x₁ < x₂  ⇒ f(x₁) < f(x₂)

Thus f(x) = 9x + 2 is an increasing function.

Method 2:

f(x) = 9x + 2

⇒ f’(x) = 9 ⇾ +ve for all values of x ⇾ Function is increasing.

Function Decreasing at a Point

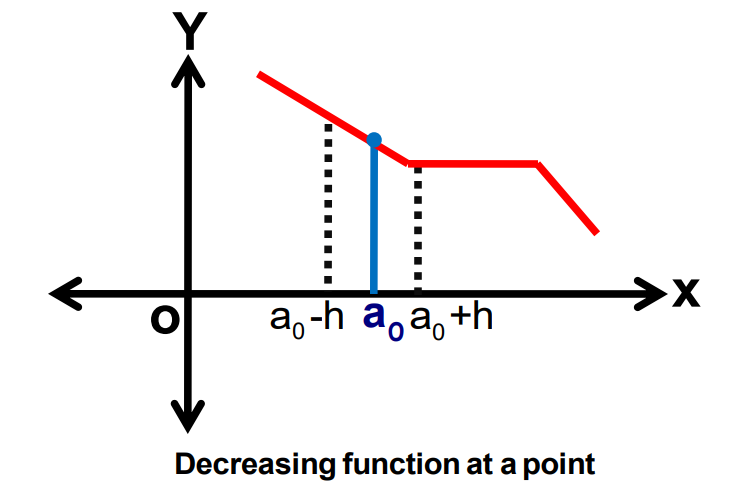
A function f is said to be decreasing at a point a₀ if there exists an interval ( a₀ – h, a₀ + h ), h > 0 such that x₁ < x₂ ⇒ f(x₁)≥ f (x₂) for all x₁, x₂ ∈ (a₀ -h, a₀ +h).

Strictly increasing function 

A function f(x) is said to be strictly decreasing on an interval I, if

x₁ < x₂ ⇒ f(x₁) > f (x₂) for all x₁, x₂ ∈ I

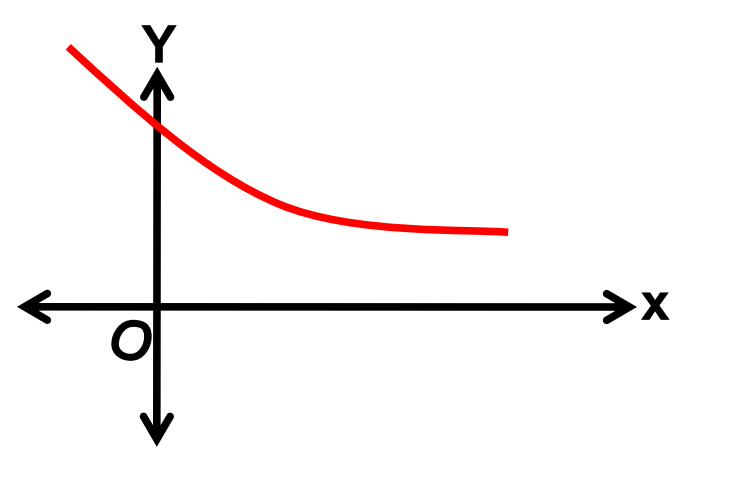
Remember: If a function f(x) is continuous on [a,b] and differentiable on (a, b), then f(x) is strictly decreasing in (a, b) if f´(x) < 0 for all x in (a, b).

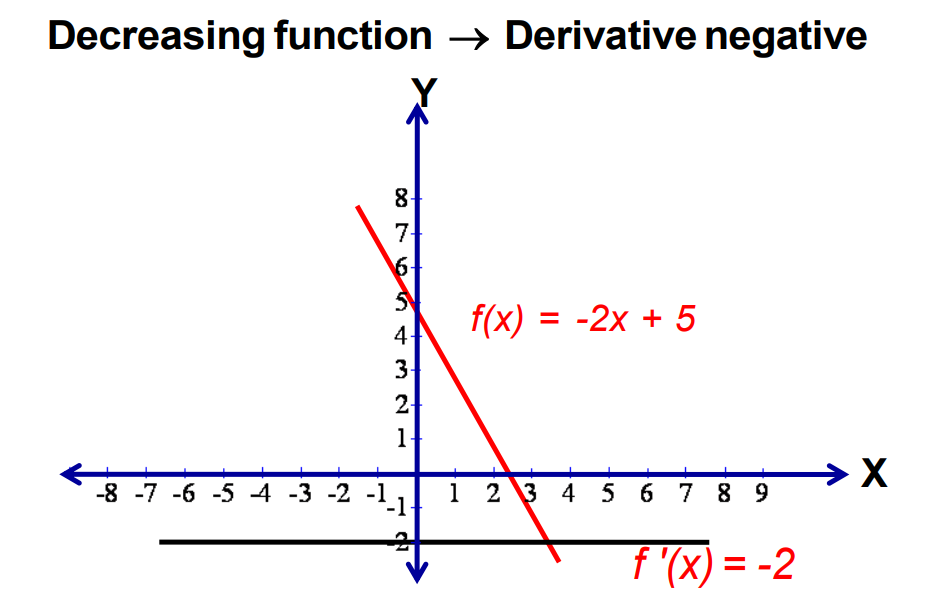
Example: 

Show that the function given by f(x) = -2x + 5 is strictly decreasing on R. 

Let x₁ and x₂ be any two numbers in R. 

Such that x₁ < x₂ Then

⇒ -2x₁ < -2x₂

⇒ – 2x₁ + 5 < – 2x₂ + 5

⇒ f(x₁) > f(x₂)

Therefore, f is strictly decreasing on R

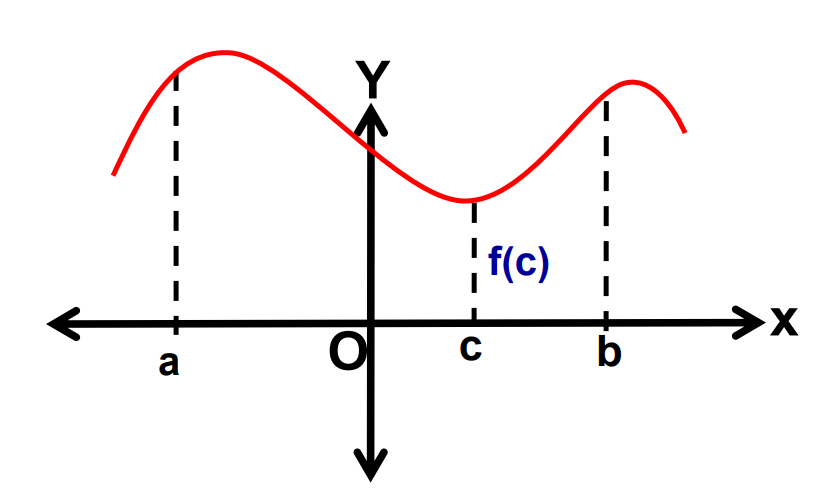
Neither Increasing or Decreasing: 

• Is it possible that a function may neither increase nor decrease in a given interval? For this let’s observe the figure.

We observe the given function f(x) is neither increasing nor decreasing in a given interval (a, b). 

• Is it possible that a function may neither increase nor decrease in a given interval?

For this look at the figure and observe … x 

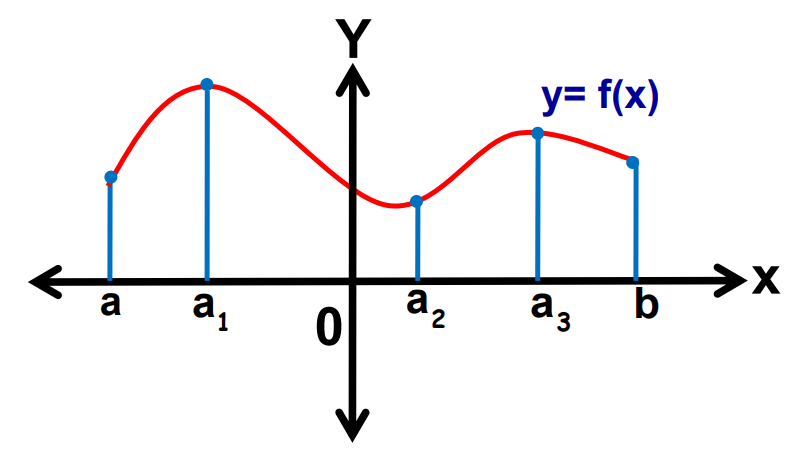
However, it is increasing in the subintervals (a, a₁), (a₂, a₃) and decreasing in subintervals (a₁, a₂), (a₃, b).

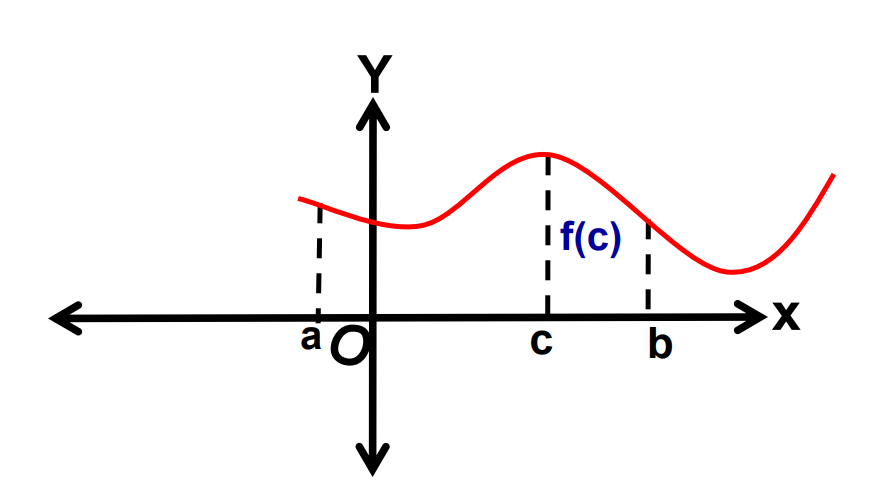
Maximum Value of a Function

Let f be a real-valued function defined on D = [a,b] then f is said to have a minimum value in D if there exists a point x = c in D such that f(c) ≥ f(x) for all x ∈ D. The number f(c) is called the maximum value of f in D and the point c is called the point of maxima of f in D. 

Minimum Value of a Function

Let f be a real-valued function defined on D = [a,b] then f is said to have a minimum value in D if there exists a point x = c in D such that f(c) ≤ f(x) for all x ∈ D. The number f(c) is called the minimum value of f in D and the point c is called the point of minima of f in D.

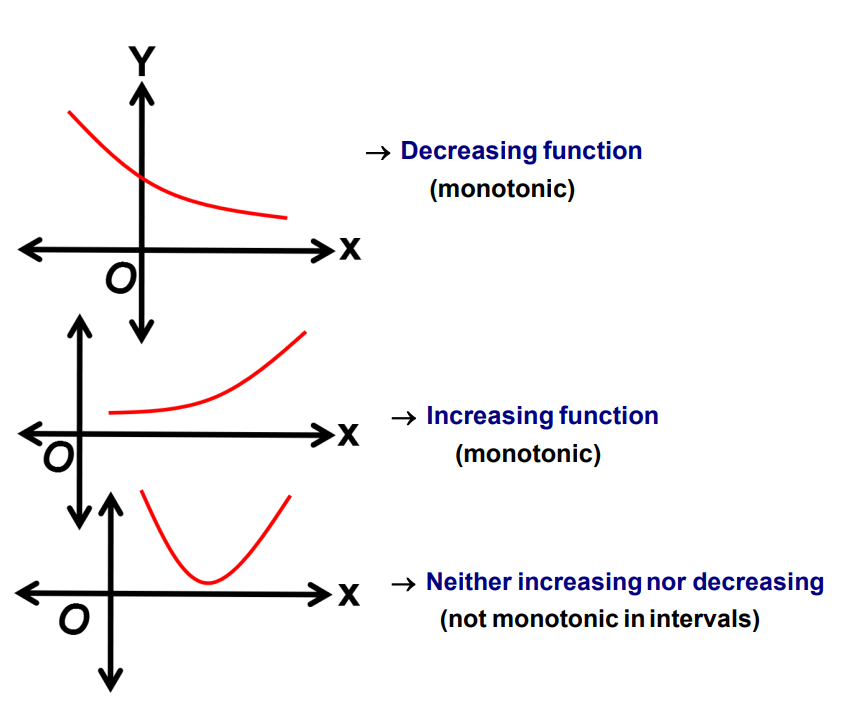
Monotonic Function

• A monotonic function f in an interval I is a function that is either increasing in I or decreasing in I. 
• Every monotonic function assumes its maximum and minimum values at the endpoints of the domain of the function.

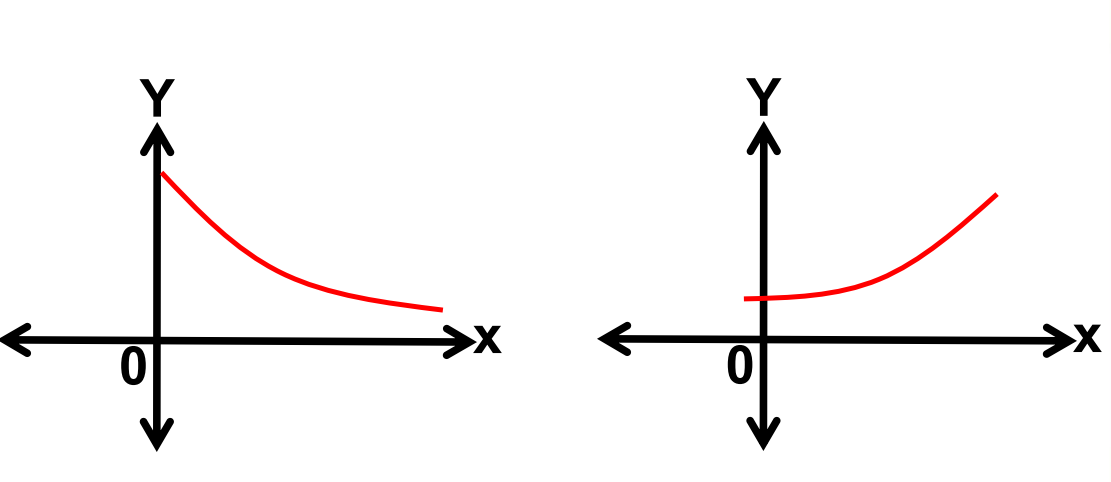
• Monotonic Function is either increasing or decreasing, but not both in the interval. 
• The sign of the derivative does not change in the case of a monotonic function.

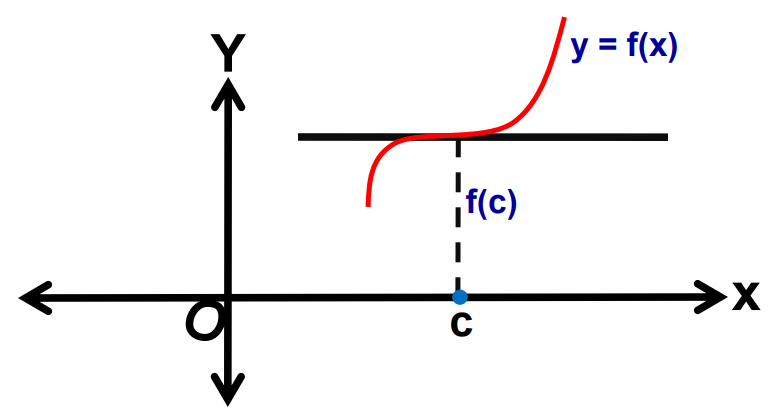
Local Maxima

Let f be a real-valued function and let c be an interior point in the domain of f. Then c is called a point of local maxima if there is an h > 0 such that f (c) > f (x), for all x in (c – h, c + h), x ≠ c

The value f (c) is called the local maximum value of f.

Local Minima

Let f be a real-valued function and let c be an interior point in the domain of f. Then c is called a point of local minima if there is an h > 0 such that f (c) < f (x), for all x in (c – h, c + h), x ≠ c

The value f (c) is called the local minimum value of f. 

First Derivative Test

Let f be a function defined on an open interval I and continuous at a critical point c in I. Then 

(i) If f ′(x) changes sign from positive to negative as x increases through c, then c is a point of local maxima. 

(ii) If f ′(x) changes sign from negative to positive as x increases through c, then c is a point of local minima. 

(iii) If f ′(x) does not change sign as x increases through c, then c is neither a point of local maxima nor a point of local minima. Such a point is called a point of inflection. 

Example: 

Find all points of local maxima and local minima of the function f given by f(x) = x³ – 3x + 3. 

Solution: We have f(x) = x³ – 3x + 3

                 f ‘(x) = 3x² – 3 

                          = 3(x² – 1) 

                          = 3(x – 1) (x + 1).

Therefore f ‘(x) = 0 at x = 1 and x = -1. Thus, x = ±1 are the only critical points that could be the points of local maxima and/or local minima of f. 

• Let us first examine the point x = 1. For values close to 1 and to the right of 1, f’(x) > 0, and for values close to 1 and to the left of 1, f’(x) < 0. Therefore, by the first derivative test, x = 1 is a point of local minima and the local minimum value is f(1) = 1. 
• In the case of x = -1, f’(x) > 0, for values close to and to the left of -1, and f’(x) < 0, for values close to and to the right of -1. Therefore, by the first derivative test, x = -1 is a point of local maxima and the local maximum value is f(-1)= 5.

Second Derivative Test

Let f be a function defined on an interval I and c ∈ I. Let f be twice differentiable at c. Then

(i) x = c is a point of local maxima if f ′(c) = 0 and f ″(c) < 0 The value f (c) is the local maximum value of f. 

(ii) x = c is a point of local minima if f′(c) = 0 and f ″(c) > 0 In this case, f (c) is the local minimum value of f. 

(iii) If f ′(c) = 0 and f ″(c) = 0 then test fails.

In this case, try the first derivative test

Example: 

Find the maximum and minimum values, if any, of the function f given by f(x) = 9x² + 12x + 2

We have f(x) = 9x² + 12x + 2

f ‘(x) = 18x + 12 

          = 6(3x + 2) 

Therefore f ‘(x) = 0 at x = – 2/3

f”(x) = 18 > 0

Therefore, by the second derivative test, x = -2/3 is a point of local minima and the local minimum value of f at x = -2/3 is f(-2/3) = -2. 

Maximum and Minimum Values of a Function in a Closed Interval 

Theorem 1: Let f be a continuous function on an interval I = [a, b]. Then f has the absolute maximum value and f attains it at least once in I. Also, f has the absolute minimum value and attains it at least once in I. 

Theorem 2: Let f be a differentiable function on a closed interval I and let c be any interior point of I. Then 

(i) f’(c)= 0 if f attains its absolute maximum value at c. 

(ii) f’(c)= 0 if f attains its absolute minimum value at c.

Working Rules for Finding Absolute Maximum and Absolute Minimum

• Step 1: Find all the points x where either f′(x) =0 or f is not differentiable. 
• Step 2: At all these points (listed in Step 1) and also at the endpoints of the interval, calculate the values of f. 
• Step 3: Identify the maximum and minimum values of f out of the values calculated in Step 2 This maximum value will be the absolute maximum (greatest) value of f and the minimum value will be the absolute minimum (least) value of f.

Let’s Conclude

In conclusion, the Application of Derivatives is a vital concept in CBSE Class 12 Math that provides powerful tools for solving real-world problems. This chapter covers essential topics like calculating the rate of change, determining tangents and normals to curves, and analyzing functions to find their maximum and minimum values. The Application of Derivatives also helps in understanding whether a function is increasing or decreasing over a given interval, making it a crucial aspect of mathematical analysis. With the comprehensive learning resources available on iPrep, mastering the chapter Application of Derivatives becomes a seamless and engaging experience.