Work Energy and Power – Complete Guide For Class 11 Physics Chapter 6
Welcome to iPrep, your Learning Super App. Our learning resources for Chapter 6, “Work, Energy, and Power,” in Class 11 Physics are meticulously designed to ensure students gain a comprehensive understanding of this essential topic. These resources include detailed notes on fundamental concepts such as work, kinetic and potential energy, and power. We also provide clear explanations of the work-energy theorem and the concept of conservation of mechanical energy, helping students understand how energy transforms within a system.
Additionally, our resources feature illustrative examples to demonstrate real-world applications, such as how power is calculated for machines or how energy is conserved in different types of collisions. Practice exercises and problem-solving strategies are also provided to help students apply these concepts effectively in exams and practical scenarios.
What Is Work Energy And Power?
The concept of “Work Energy and Power” in Class 11 Physics delves into the foundational principles of science by exploring the methods and standards used to quantify and describe physical phenomena. This chapter introduces students to the definitions of work energy and power, establishing how they are related through mathematical expressions. Students learn how to calculate work done by a force and explore different types of energy, such as kinetic energy (energy due to motion) and potential energy (energy due to position or configuration).
The chapter also explains the work-energy theorem, which links work done on an object to changes in its kinetic energy. Additionally, students gain insights into the concept of conservation of mechanical energy, where total energy in a system remains constant in the absence of non-conservative forces like friction. Finally, students are introduced to power, which measures the rate at which work is done or energy is transferred.
- Work: Defined as the force applied on an object resulting in its displacement.
- Energy: The capacity to do work.
- Power: The rate at which work is done.
The Work-Energy Theorem states that the change in the kinetic energy of an object is equal to the work done by the net force acting on the object.
Scalar Product
The scalar product (or dot product) of two vectors A and B is expressed as:

A⋅B=ABcosθ
Where:
- A and B are the magnitudes of the vectors,
- θ is the angle between them.
This product represents the projection of one vector along the direction of another.
Scalar Product of Unit Vectors
For unit vectors along the X, Y, and Z axes:

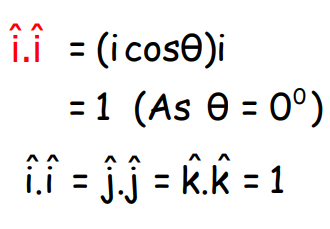

For two general vectors the scalar product is given by:
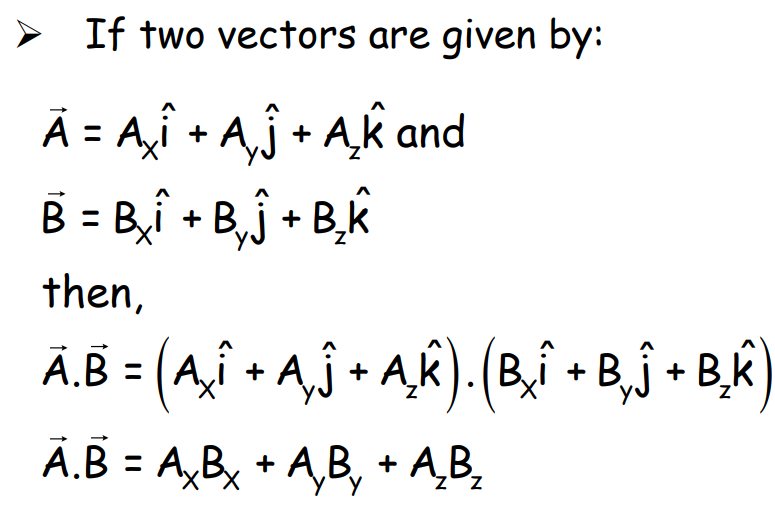
Properties of the Dot Product
Work
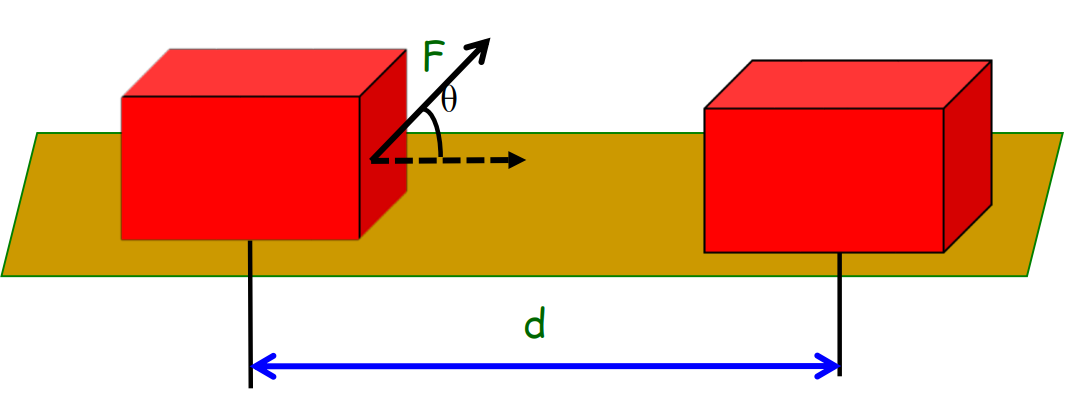
Work is said to be done when a force acts on a body and displaces it. The formula for work is:
W=F⋅d=Fdcosθ
Where:
- F is the force applied,
- d is the displacement,
- θ is the angle between the force and displacement.
Work is a scalar quantity, and its SI unit is the joule.
Examples of Work
- Kicking a ball: The ball moves due to the force applied, resulting in work.

- Throwing a basketball: Work is done when the ball is thrown upwards.
Nature of Work
Work can be classified as:
Type of Work Description Example Positive Work Force and displacement are in the same direction. Work done by gravity on a falling object. Negative Work Force and displacement are in opposite directions. Work done by gravity on an object moving upwards. Zero Work No displacement, or force and displacement are perpendicular. A coolie carrying a load horizontally (force perpendicular to displacement).
Zero Work Done
Zero work is done in the following cases:
- No displacement: If a force is applied but there is no movement (e.g., pushing against a wall).

- No force: A block moving on a frictionless surface undergoes displacement, but no work is done as there is no frictional force.
- Perpendicular forces: When the force and displacement are perpendicular, such as carrying a load horizontally.
Work and Energy
The Work-Energy Theorem states that the work done by the net force on a body is equal to the change in its kinetic energy:
From third equation of Kinematics : v2+u2 = 2as……..(1)
Where u and v are the initial and final speeds respectively s distance traversed by the body. An acceleration of the body.
On multiplying both sides of equation(1) by m / 2,we get,
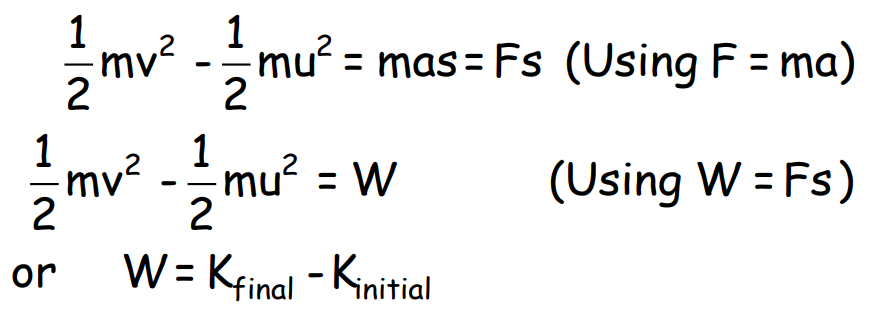
The above relation is known as work – the energy theorem.
Kinetic Energy

Kinetic energy (K) is the energy possessed by a body due to its motion:
K=1/2mv2
Examples of Kinetic Energy
- A hammer driving a nail into wood.

- Water powering a water mill.
Work Done by a Variable Force
When force varies with displacement, the work done is represented as the area under the force-displacement graph:
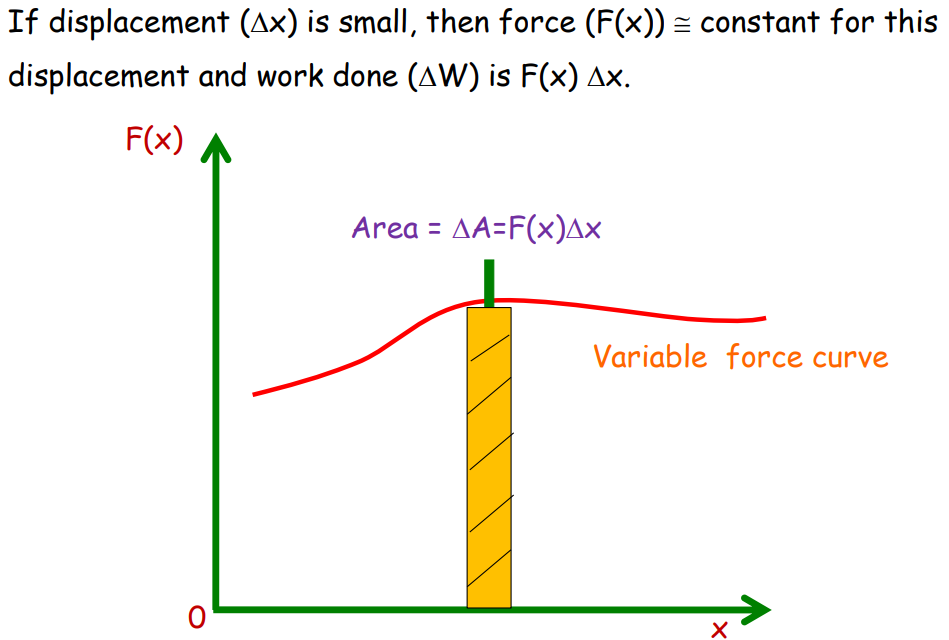
This area represents the total work done by the variable force.
Potential Energy
Potential Energy (P.E.) is the energy stored in a body due to its position or configuration. Two common forms of potential energy are:
- Gravitational Potential Energy: U=mgh
- Elastic Potential Energy: Energy stored in a stretched or compressed spring, following Hooke’s Law: Fs=−kx
Gravitational Potential Energy
The gravitational potential energy of an object, at a point, is the work done in raising it from the reference level to that point, against gravity.
V(h) = Gravitational potential energy of an object as a function of height, h.
V(h) = mg
When ‘h’ is variable, then the gravitational force is
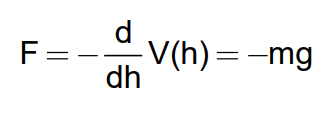
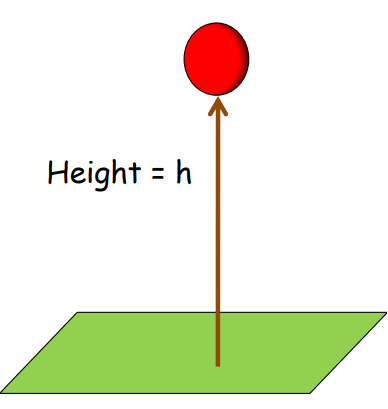
The speed of the ball just before it hits the ground is
v2 = 2gh. Or 1/2 mv2 = mgh
So, the gravitational energy of an object at a height (h) changes into the kinetic energy of the object just before it hits the ground.
Conservative and Non-Conservative Forces
- Conservative Forces: Work done depends only on the initial and final positions (e.g., gravitational, spring forces).
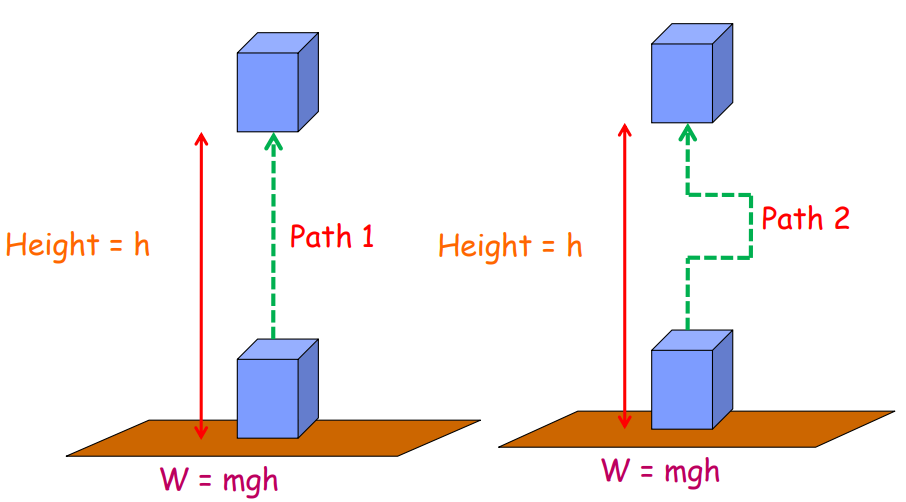
- Non-Conservative Forces: Work done depends on the path taken (e.g., friction).

Mechanical Energy Conservation
In a system where only conservative forces are acting, the total mechanical energy (kinetic + potential) is conserved. For a free-falling object:
E total=K.E.+P.E
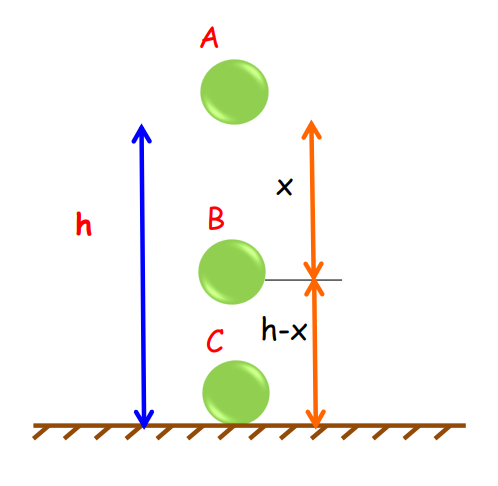
Mechanical Energy Conservation in Free Fall
Let’s consider a body of mass m held at a height h above the ground:
- At point A, the body is at rest, so:
- Kinetic Energy (K.E.) = 0
- Potential Energy (P.E.) = mgh
- Total energy at A = E1=K.E+P.E=0+mgh=mgh
- As the body falls freely under gravity, it reaches the ground (point C) with a velocity v. Using the equation.
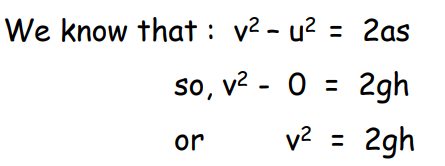
- Kinetic Energy at C is
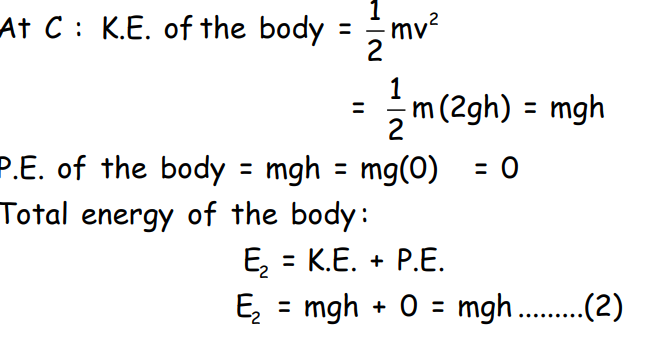
- Potential Energy at C = 0
- Total energy at C = E2=mgh
Thus, the total mechanical energy E1=E2=mgh, proving the conservation of mechanical energy.
Potential Energy of a Spring
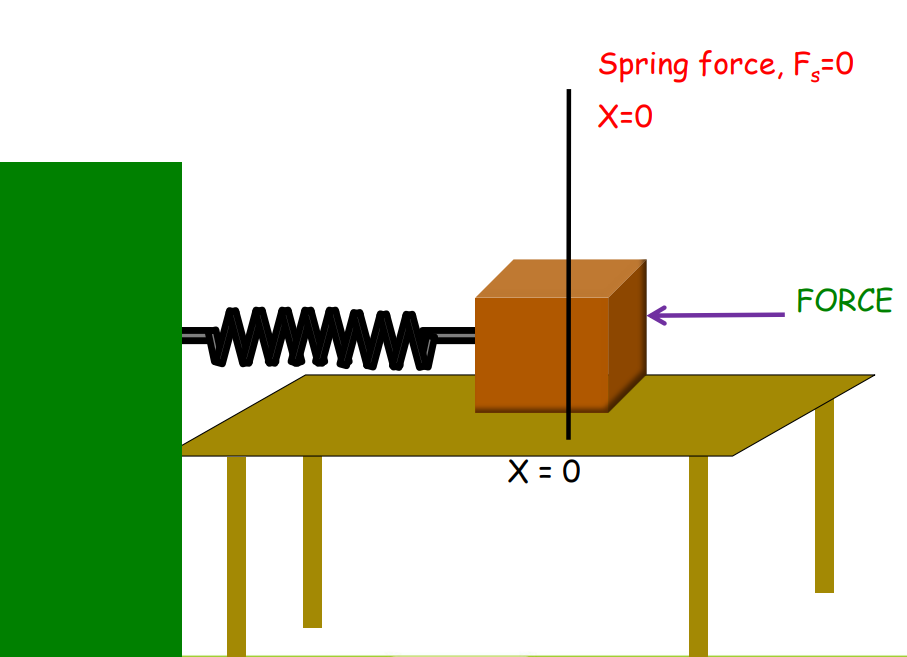
The potential energy stored in a spring is proportional to the square of the displacement from its equilibrium position. This relationship is described by Hooke’s Law:
Fs=−kx
Where:
- Fs is the spring force,
- k is the spring constant,
- x is the displacement from the equilibrium position.
Work Done by Spring Force
The work done by the spring force is calculated as:
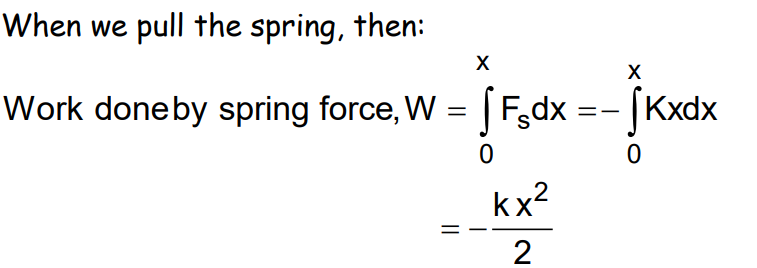

This formula shows that the potential energy stored in the spring is dependent only on the displacement and the spring constant.
Work done by the spring force in moving the block from initial displacement (xi ) to final displacement (xf ) only depends on the endpoints and is given by:
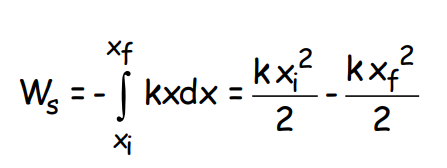
Various Forms of Energy
Energy exists in different forms, each playing a crucial role in physical systems:
- Heat Energy: Energy possessed by a body due to the random motion of its molecules. Heat can be transferred via conduction, convection, or radiation.
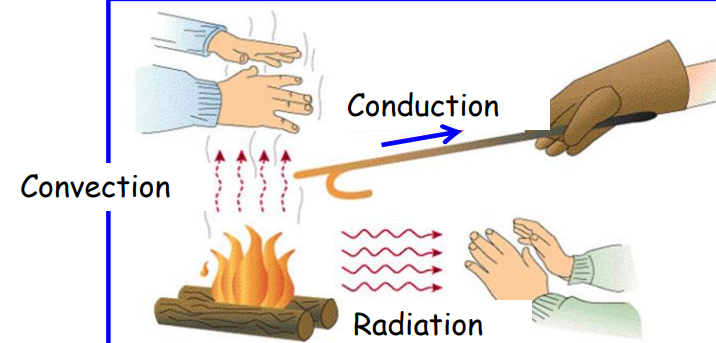
- Chemical Energy: Stored in the bonds of molecules and released during chemical reactions.

- Nuclear Energy: Released during nuclear fusion (combining lighter nuclei) or fission (splitting heavier nuclei). This energy is associated with the atomic nucleus. Radioactive decay involves the spontaneous emission of radiation and ionizing particles by an unstable atomic nucleus.
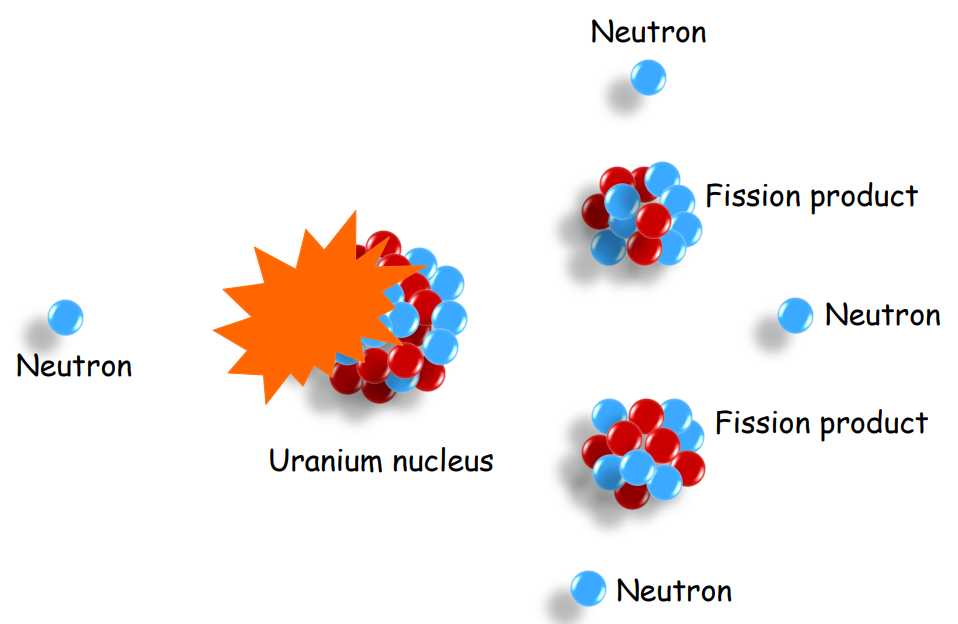

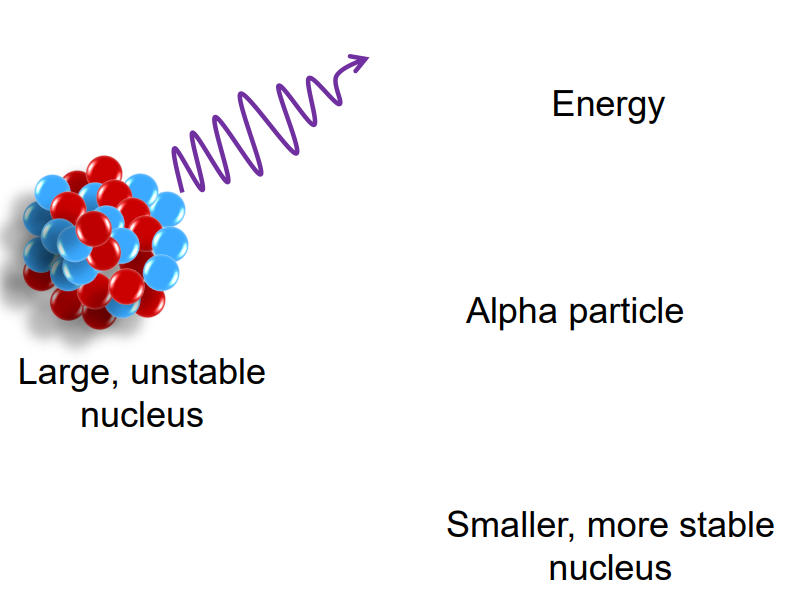
Nuclear Fission Nuclear Fusion Radioactive Decay
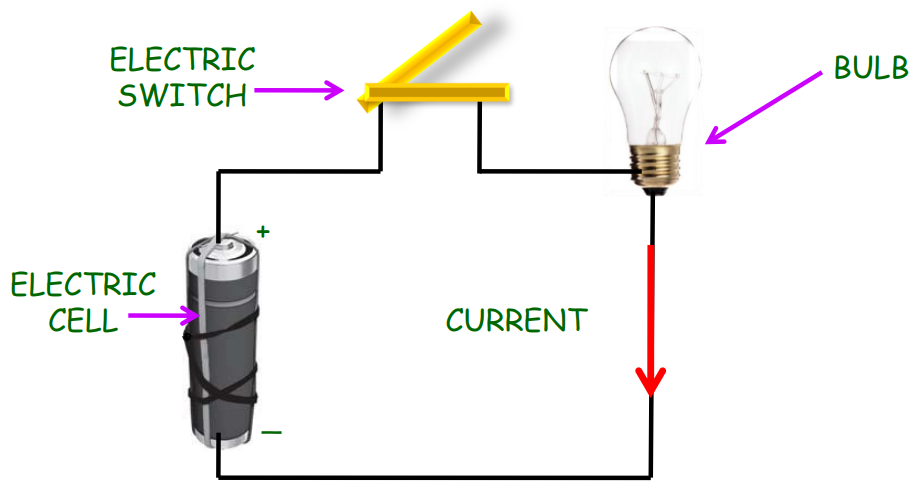
- Electrical Energy: Energy available from the flow of electric charge through a conductor.
- Mass-Energy Equivalence: According to Einstein’s famous equation E=mc2, mass can be converted into energy, indicating the relationship between mass and energy.
Principle of Conservation of Energy
The Principle of Conservation of Energy states that energy cannot be created or destroyed but can only be transformed from one form to another. For example:
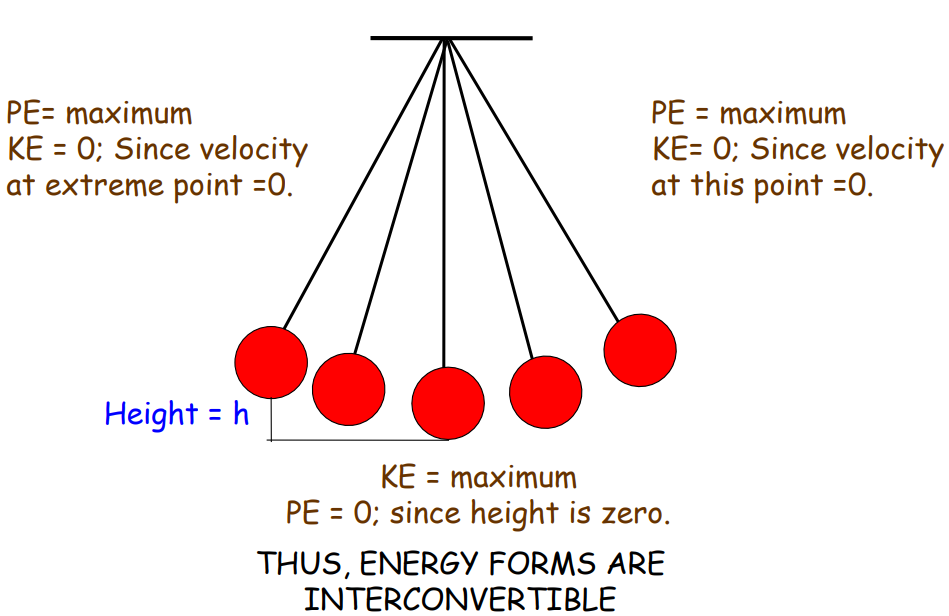
- Potential energy transforms into kinetic energy as a body falls, and vice versa when the body is thrown upward.
- At the highest point of motion, potential energy is maximum, and kinetic energy is zero, while at the lowest point, kinetic energy is maximum, and potential energy is zero.
Power
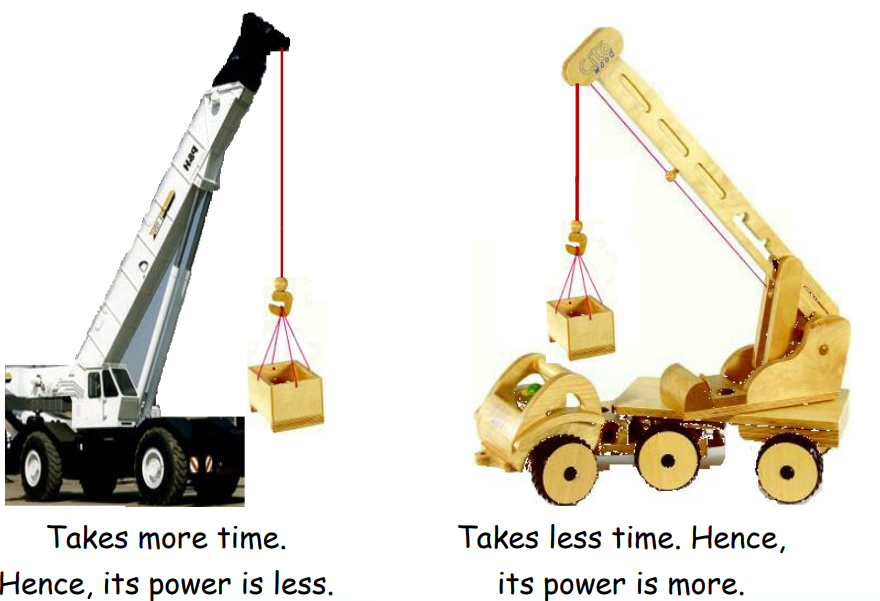
Power is defined as the rate at which work is done or energy is transferred. It is given by:
P=dW/dt
Where:
- P is power,
- W is work,
- t is time.
The SI unit of power is the watt (W), and its dimensional formula is
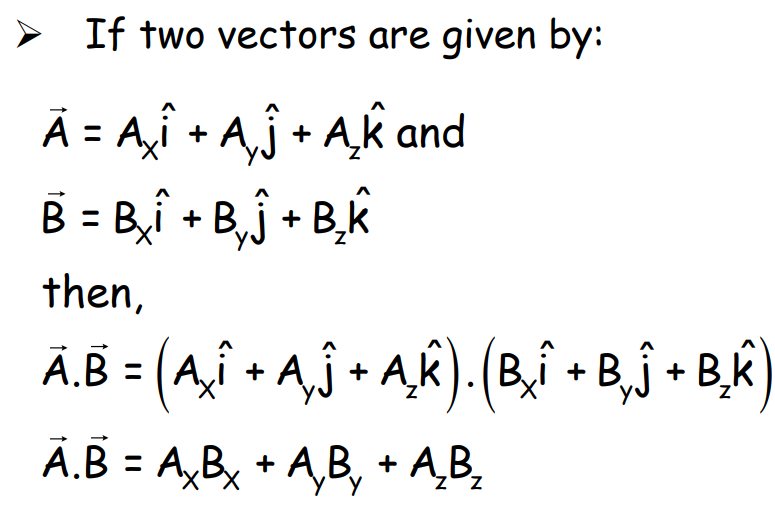
Collisions

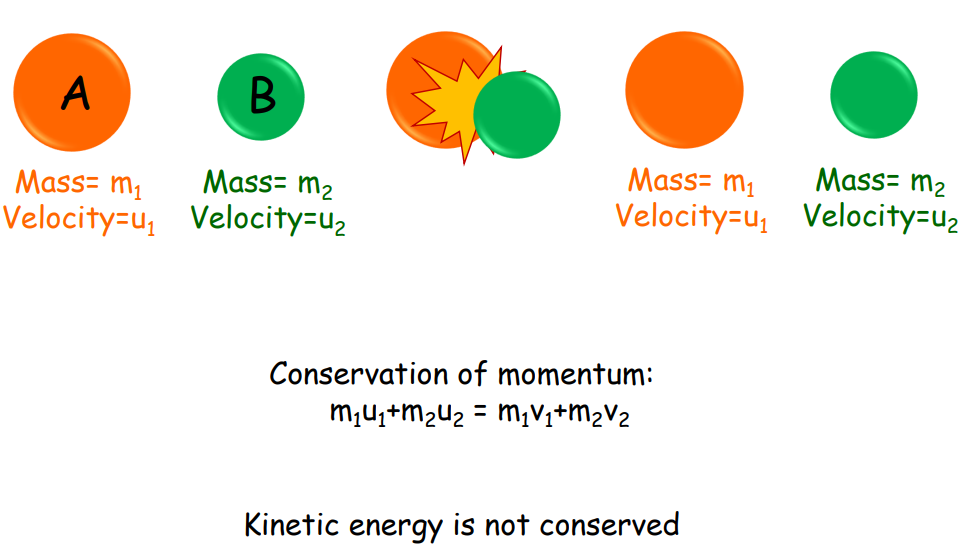
A collision is an event where two or more bodies exert forces on each other over a short time interval. Collisions are classified into two main types:
- Elastic Collisions:
- Both momentum and kinetic energy are conserved.
- Example: Billiard balls colliding.

- Inelastic Collisions:
- Only momentum is conserved, while kinetic energy is not.
- In a perfectly inelastic collision, the bodies stick together and move with a common velocity after the collision.
Elastic Collision in One Dimension
In one-dimensional elastic collisions, both momentum and kinetic energy are conserved:
Inelastic Collision
In an inelastic collision, some of the kinetic energy is converted into other forms of energy, such as heat or deformation. The momentum remains conserved, but kinetic energy does not.
In a perfectly inelastic collision, the colliding bodies stick together and move with a common velocity:

Conclusion
This comprehensive guide on “Work Energy and Power” provides an in-depth exploration of the fundamental aspects of physics as outlined in Class 11. It covers the core concepts of units, measurements, and the importance of standardization in scientific studies. The guide explains work as the product of force and displacement and introduces energy as the ability to perform work with detailed discussions on kinetic and potential energy. It delves into the work-energy theorem, which links the work done by a force to changes in an object’s energy.
Furthermore, the guide explores the principle of conservation of energy, which states that energy cannot be created or destroyed but only transformed from one form to another. It also includes a thorough explanation of power, defined as the rate at which work is done or energy is transferred, helping students understand its applications in real-world scenarios like machines and engines.
Practice questions on Chapter 6 - Work Energy and Power
Get your free Chapter 6 - Work Energy and Power practice quiz of 20+ questions & detailed solutions
Practice Now








