Kinetic Theory – Complete Guide For Class 11 Physics Chapter 13
Welcome to iPrep, your Learning Super App. Our learning resources for Chapter 13, “Kinetic Theory,” in Class 11 Physics are meticulously designed to ensure students gain a comprehensive understanding of this essential topic. These resources include detailed notes that explain the behavior of gases based on molecular motion, delve into the derivation of gas laws, and explore concepts such as pressure, temperature, and heat. Through illustrative diagrams and step-by-step problem-solving techniques, students can connect theoretical knowledge with real-world applications. The focus is on building a strong foundation for advanced topics while making learning engaging and interactive.
The concept of “Kinetic Theory” in Class 11 Physics delves into the foundational principles of science by exploring the methods and standards used to quantify and describe physical phenomena. This chapter introduces students to the microscopic model of gases, where gas molecules are in constant random motion. It explains the relation between molecular movement and macroscopic properties like pressure and temperature. The theory connects the behavior of gas molecules with thermodynamic laws and derives important equations such as the ideal gas law. Understanding kinetic theory lays the groundwork for concepts like heat transfer and the nature of gases.
Kinetic Theory
Just like solids and liquids, gases are also composed of atoms or molecules. However, unlike solids and liquids, the interatomic or intermolecular forces in gases are negligible, allowing the particles to move freely and rapidly.
The kinetic theory of gases explains the behavior of gases by considering these rapid movements. It aligns with Avogadro’s hypothesis and various gas laws, interpreting properties like viscosity, specific heat capacity, conduction, and diffusion of gases.

Molecular Nature of Matter
According to Dalton’s Atomic Theory, all matter is made up of the smallest particles called atoms. Here’s a quick breakdown:
- Atoms of a single element are identical but differ from those of other elements.
- Atoms combine to form molecules of compounds.
Dalton’s Atomic Theory supports two important laws:
- Law of Definite Proportions: In any given compound, its constituents are in a fixed proportion by mass.
- Law of Multiple Proportions: When two elements form more than one compound, the masses of one element that combine with a fixed mass of the other are in ratios of small integers.
Supporting Theories:
- Gay Lussac’s Law: When gases combine chemically to form another gas, their volumes are in small integer ratios.
- Avogadro’s Law: Equal volumes of all gases at the same temperature and pressure contain the same number of molecules.
- Recent Observations: Molecules make up matter, and the size of an atom is around an angstrom (A).
Comparison Between Solids, Liquids, and Gases:
| State of Matter | Structure | Interatomic Distance | Characteristics |
| Solids | Atoms are tightly packed | About 2 A | Strong interatomic forces, fixed size, shape, and volume |
| Liquids | Atoms are not fixed, can move around | About 2 A | Weaker forces, definite volume but no definite shape |
| Gases | Atoms are far apart | Tens of angstroms | Negligible forces, no fixed shape or volume |
Gas Laws
The behavior of gases is governed by certain laws, including:
Boyle’s Law
- Statement: At constant temperature, the pressure of a given mass of gas is inversely proportional to its volume.
P∝1 or PV=constant

Charles’ Law
- Statement: At constant pressure, the volume of a given mass of gas is directly proportional to its temperature.
V∝T or V/T=constant
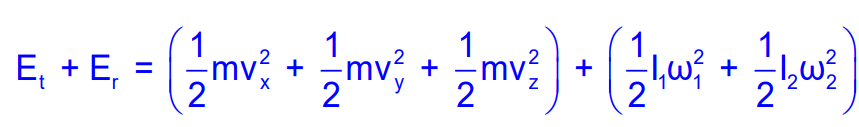
Dalton’s Law of Partial Pressure
- Statement: The total pressure exerted by a mixture of non-reactive gases is the sum of the partial pressures exerted by each gas.
Ptotal=P1+P2+P3+⋯+Pn
Ideal Gas or Perfect Gas
An ideal gas perfectly obeys the gas laws (Boyle’s Law, Charles’ Law, etc.). While no real gas is completely ideal, gases at low pressure and high temperature come close to ideal behavior.
Characteristics of an Ideal Gas:
- The size of gas molecules is negligible.
- There are no intermolecular forces (attraction or repulsion).
The behavior of Ideal Gases:
At low pressure and high temperature, a gas follows the simple relation:
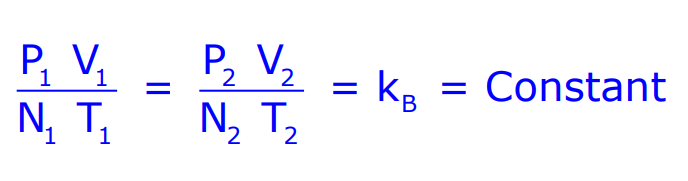
Where N is the number of gas molecules, and kB is the Boltzmann constant.
Avogadro’s Hypothesis
According to Avogadro’s Hypothesis, equal volumes of all gases under identical conditions of pressure and temperature contain the same number of molecules.
Avogadro’s Number
- Value: 6.023× 10^{23}molecules in 22.4 liters of any gas at NTP (Normal Temperature and Pressure).
Using Avogadro’s number, the perfect gas equation is written as:
PV=μRT
Where μ is the number of moles, and R is the universal gas constant.
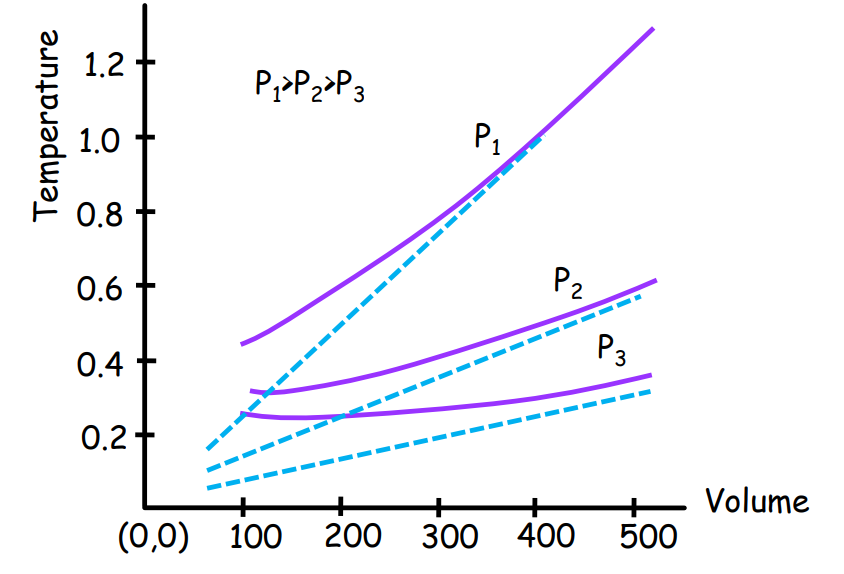
Real vs Ideal Gases
At high temperatures and low pressures, gases approach ideal behavior. However, real gases deviate from ideality at higher pressures and lower temperatures due to molecular interactions.
Deviation from Boyle’s Law:
Graphical representation shows the deviation of real gases from ideal behavior at various temperatures.
Kinetic Theory of an Ideal Gas
This theory is based on several key assumptions:
- A gas consists of a large number of molecules in continuous random motion.
- The intermolecular distances are much larger than the size of the molecules.
- The collisions between molecules (and between molecules and container walls) are perfectly elastic.

Pressure in an Ideal Gas:
The pressure of an ideal gas is due to the continuous bombardment of gas molecules against the walls of the container. The pressure is related to the kinetic energy of the molecules, which is proportional to the temperature of the gas.

Kinetic Interpretation of Temperature
The temperature of an ideal gas is a measure of the average kinetic energy of its molecules. It is given by:
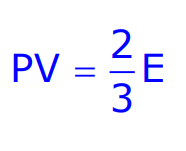
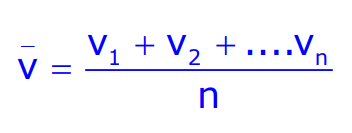
Where E is the average kinetic energy of a gas molecule, and T is the absolute temperature.
Kinetic Interpretation of Temperature
For a mixture of non-reactive ideal gases, the total pressure contributes from each gas. The average kinetic energy of the molecules of different gases in a mixture is equal at equilibrium.
Pressure Equation
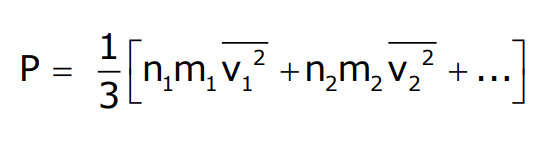
This relationship leads to Dalton’s law of partial pressure:
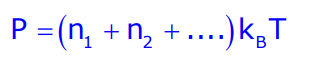
Where:
- P: Pressure
- n: Number of moles
- kB: Boltzmann constant
- T: Absolute temperature
Different Forms of Speeds
In a given mass of gas, the velocities of all molecules vary. Collisions constantly change their direction and speed.
Types of Molecular Speeds
- Most Probable Speed (VM. p)
- Mean Speed (v)
- Root Mean Square Speed
Maxwell Speed Distribution
The distribution of molecular speeds is given by:
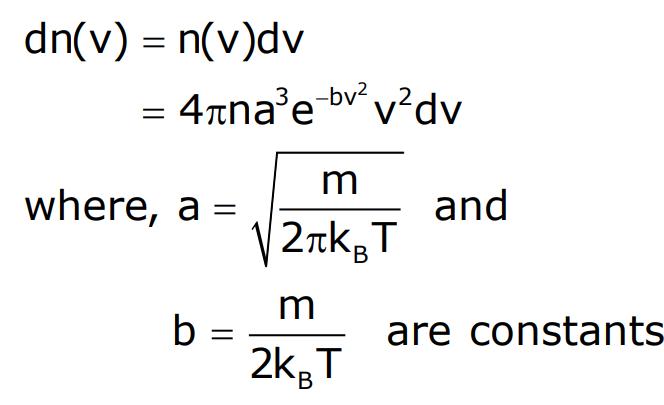
Where a and b are constants.
Most Probable Speed
The most probable speed is defined as the speed possessed by the maximum fraction of the total number of molecules:

Mean Speed
The mean speed of n individual molecules is given by:
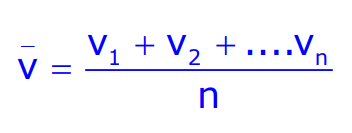
From Maxwell’s distribution, it can be expressed as:
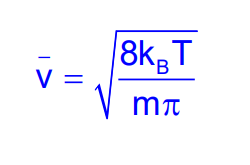
Root Mean Square Speed
The root mean square speed is the square root of the mean of the squares of the speeds:
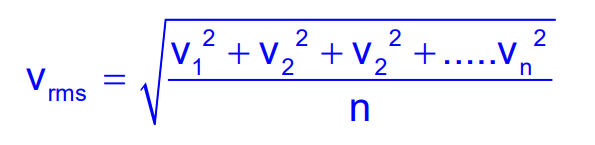
From Maxwell’s distribution, it can be expressed as:
Speed Ratios
The ratios of these speeds can be expressed as follows:
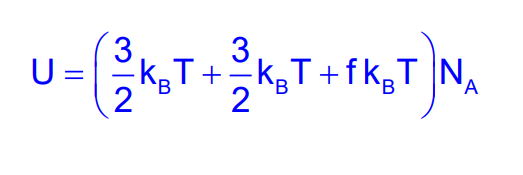
Graham’s Law of Diffusion
According to Graham’s law, the rate of diffusion of gases is inversely proportional to the square roots of their densities or masses:
v1/v2=m2/m1
Degrees of Freedom
The degrees of freedom of a dynamical system refers to the number of independent coordinates needed to describe its position and configuration.
Degrees of Freedom Examples
- A point particle moving in a straight line: 1 degree of freedom.
- A point particle in a plane: 2 degrees of freedom.
- A point particle in space: 3 degrees of freedom.
For a system with NP particles and R-independent relations, the degrees of freedom (ND) can be calculated as:
ND=3NP−R
Example: Two Particles
For two particles at constant motion in space, ND=3NP−R = 3×2−1=5
Law of Equipartition of Energy
In thermal equilibrium, energy is equally distributed among all possible modes, with each mode having an average energy of (½)kBT.
Kinetic Energy Expression
For translational kinetic energy:
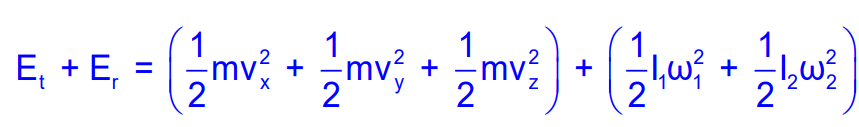
Average translational energy is:
Et=3/2kBT
Diatomic Molecules
For diatomic molecules, there are additional rotational degrees of freedom. The total energy can be expressed as:
E=Et+Er
Specific Heat Capacity
The law of equipartition of energy helps predict the specific heat of gases.
Monatomic Gases
Monatomic gases have three degrees of freedom:
Diatomic Gases
Diatomic gases have five degrees of freedom:
CV=5/2R
Triatomic Gases
- Linear Triatomic Gases: Seven degrees of freedom, CV=7/2R
- Non-linear Triatomic Gases: Six degrees of freedom, CV=3R
Polyatomic Gases
For polyatomic gases, the total energy is given by:
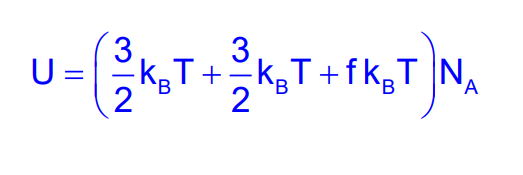
Specific Heat Capacities Without Vibrational ModesMean Free Path
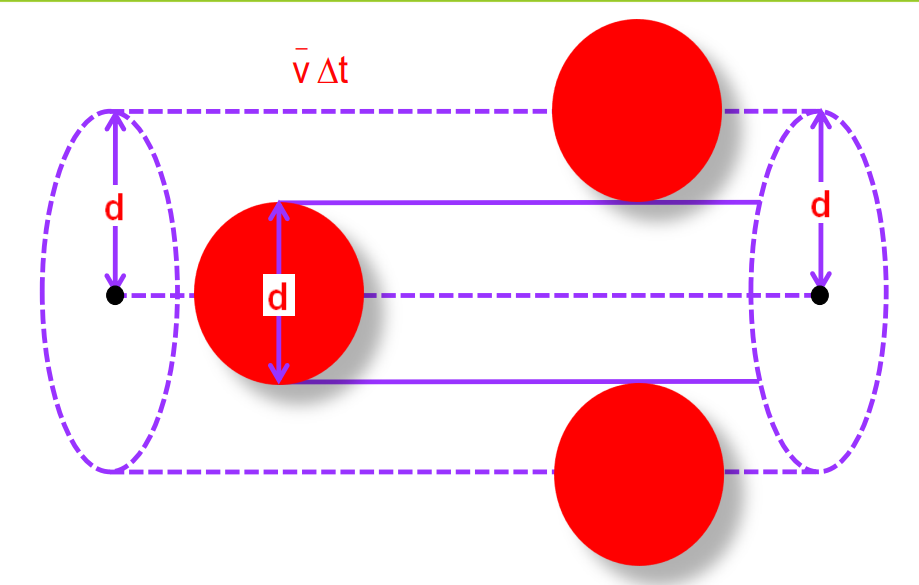
The mean free path is the average distance between two successive collisions of gas molecules.
Calculation of Mean Free Path

- Molecules of gas are treated as spheres of diameter d.
- The average distance is defined as:
λ=v/nd2
where n is the number of molecules per unit volume.
Conclusion
This comprehensive guide on “Kinetic Theory” provides an in-depth exploration of the fundamental aspects of physics as outlined in Class 11. It covers core concepts such as the behavior of gas molecules, the relationship between temperature and kinetic energy, and how molecular collisions result in measurable pressure. The chapter further explains the assumptions of the ideal gas model and derives key equations, including the ideal gas law and root mean square velocity. These concepts form the basis for understanding thermodynamic processes, heat transfer, and the laws governing gases.
Practice questions on Chapter 13 - Kinetic Theory
Get your free Chapter 13 - Kinetic Theory practice quiz of 20+ questions & detailed solutions
Practice Now








