Gravitation – Complete Guide For Class 11 Physics Chapter 8
Welcome to iPrep, your Learning Super App. Our learning resources for Chapter 7, “Gravitation,” in Class 11 Physics are meticulously designed to ensure students gain a comprehensive understanding of this essential topic. These resources include detailed notes on the universal law of gravitation, Kepler’s laws of planetary motion, and the mathematical derivations of gravitational forces. They also cover important concepts such as gravitational potential energy, orbital velocity, and the behavior of satellites. Additionally, practical examples, diagrams, and solved problems are provided to enhance conceptual clarity and ensure students are well-prepared for exams and real-world applications of gravitation.
What is Gravitation?
The concept of “Gravitation” in Class 11 Physics delves into the foundational principles of science by exploring the methods and standards used to quantify and describe physical phenomena. This chapter introduces students to the universal law of gravitation, explaining how every mass in the universe exerts an attractive force on others. Students are guided through Kepler’s laws, which describe planetary motion, and are taught how to calculate gravitational force, potential energy, and escape velocity. Furthermore, the chapter emphasizes the role of gravitational constants and their experimental determination. Through these concepts, students develop a deep understanding of the forces that govern planetary systems and everyday gravitational interactions.
Newton, building upon this understanding, proposed that a net force must be acting on the Moon to prevent it from moving in a straight line. He identified this force as the gravitational attraction exerted by the Earth on the Moon.

In this chapter, we will explore:
- The law of universal gravitation.
- The laws of planetary motion as formulated by Johannes Kepler.
- Gravitational potential energy and the motion of planets and satellites.
Kepler’s Laws of Planetary Motion

1. Kepler’s First Law – Law of Orbits
All planets move in elliptical orbits with the Sun at one of the two foci.
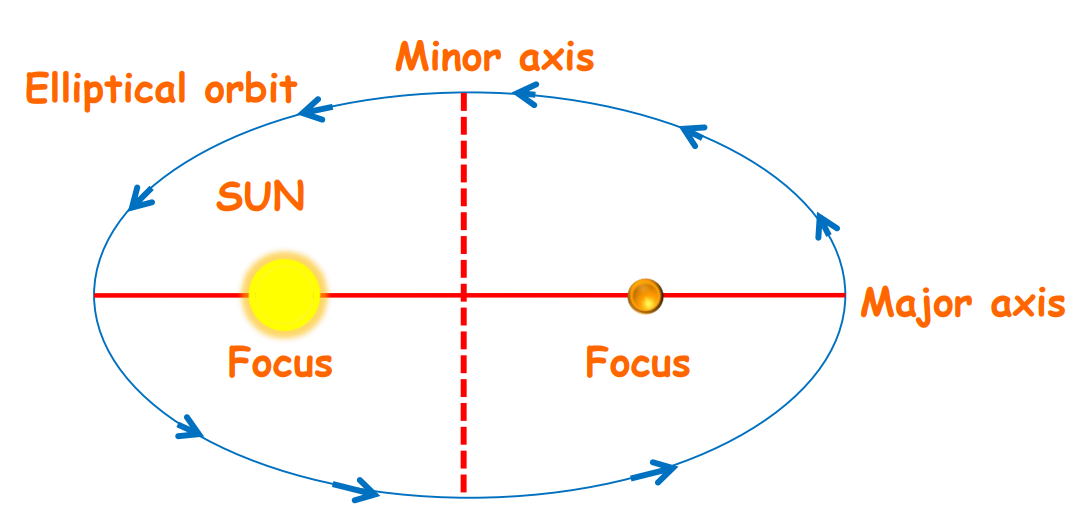
Orbit Characteristics Description Major Axis Longest diameter of the ellipse Minor Axis Shorter diameter of the ellipse Focus The point where the Sun is located
2. Kepler’s Second Law – Law of Areas
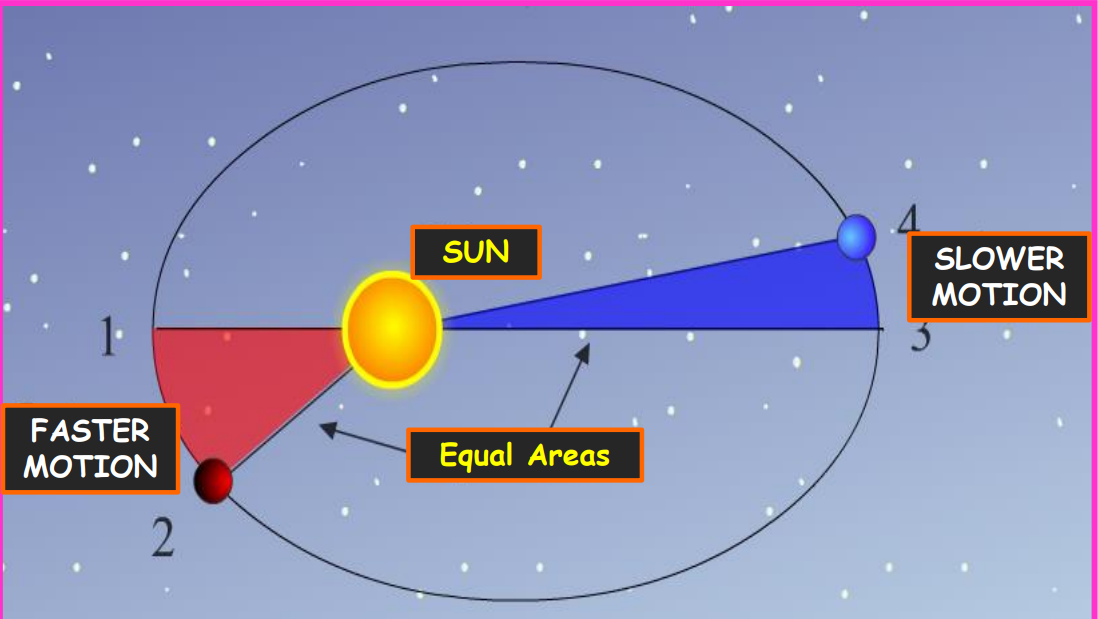
The line joining a planet to the Sun sweeps out equal areas in equal intervals of time.
- Areal velocity: The rate at which area is swept by a planet is constant.
- The linear speed of a planet is greater when it is closer to the Sun and slower when farther away.
Kepler’s second law is derived from the law of conservation of angular momentum.
3. Kepler’s Third Law – Law of Periods
The square of the time period of revolution of a planet around the Sun is directly proportional to the cube of the semi-major axis of the planet’s orbit.
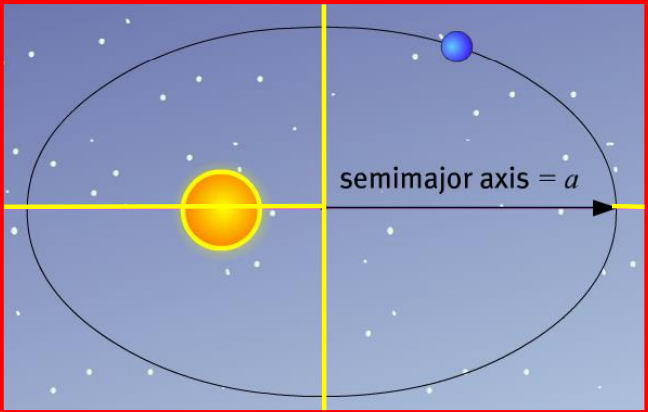
T2∝r3
Where:
- T = Time period of the planet
- r = Semi-major axis of the orbit
Universal Law of Gravitation
Newton’s law of gravitation states that any two bodies in the universe attract each other with a force directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
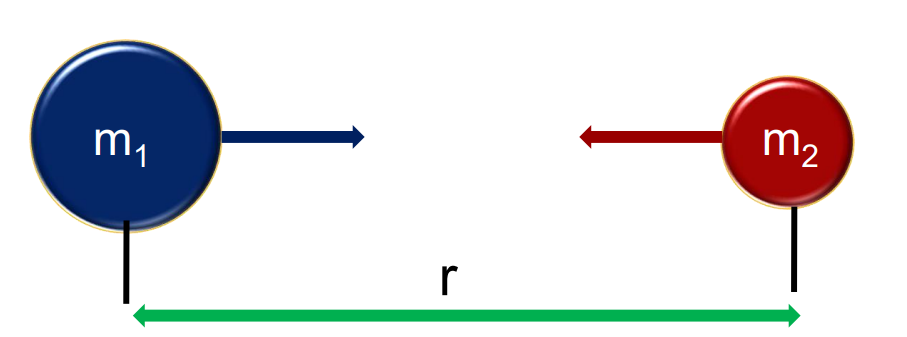
F∝m1m2/r2
F=G(m1m2/r2)
Where:
- F = Gravitational force between two masses
- G = Universal gravitational constant
- m1,m2 = Masses of the two bodies
- r = Distance between the two bodies
Universal Gravitational Constant (G)

- The universal gravitational constant is equal to the force of attraction acting between two bodies of unit masses at unit distance apart from each other.
The value of G was experimentally determined by Henry Cavendish in 1798. The accepted value is:

- A and B are two small lead spheres each of mass m attached with a bar of length l.
- S1 and S2, two big, heavy spheres each having mass M are kept on either side of A and B.
- The bigger spheres attract the smaller spheres with equal forces but in opposite directions. As a result, a gravitational torque acts on the bar.
- Let θ be the angle by which the bar is rotated by the gravitational torque. The restoring torque tends to bring back the bar to its initial position.
- The restoring torque is proportional to angle of rotation (θ) and is equal to 𝜏θ , where 𝜏 = restoring couple per unit angle of twist. Gravitation torque can be given as the product of gravitation force (F) between small and big spheres and length of the bar (l). In equilibrium, gravitational torque is equal to the restoring torque. Hence, we have
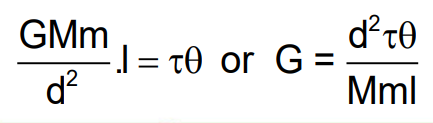
- The experiment is repeated by placing the bigger spheres at ‘S1’ and ‘S2’ and the mean value gives the true value of ‘G’.
- The accepted value of G is 6.67 × 10–11 Nm2/kg2.
Acceleration Due to Gravity (g)
The acceleration experienced by a body falling freely under Earth’s gravity is called the acceleration due to gravity, denoted by g.
F=mg (Newton’s second law)
g=F/m
- If m=1 unit, then g = F. Thus, the acceleration due to gravity is the gravitational force acting on an object of unit mass.
- ‘g’ is a vector quantity and its direction is always towards the centre of the earth.
- Its value is independent of factors like shape, size and mass of object.
Relation Between g and G
The force of attraction between the Earth and an object of mass mmm is given by:
F=GMmR2F = \frac{GMm}{R^2}F=R2GMm
By Newton’s second law:
F=mgF = mgF=mg
Equating both expressions:
g=GMR2g = \frac{GM}{R^2}g=R2GM
Variation of g with Height
At a height hhh above the Earth’s surface:
g′=g(RR+h)2g’ = g \left( \frac{R}{R+h} \right)^2g′=g(R+hR)2
This shows that the value of ggg decreases with height.
Variation of g with Depth
At a depth ddd below the Earth’s surface:
g′=g(1−dR)g’ = g \left( 1 – \frac{d}{R} \right)g′=g(1−Rd)
This implies that ggg decreases with depth as well.
Gravitational Field
The region around a material body where its gravitational force can be experienced is called its gravitational field.
Gravitational Potential
Gravitational potential at a point is the amount of work done in bringing a body of unit mass from infinity to that point without acceleration. Mathematically, it is given by:
P=WmP = \frac{W}{m}P=mW
Gravitational Potential Energy
Gravitational potential energy of a body at a point in the gravitational field is the work done in bringing the body from infinity to that point without acceleration.
U=−GMmrU = – \frac{GMm}{r}U=−rGMm
Escape Speed
The escape speed is the minimum speed needed for an object to escape Earth’s gravitational field without any further propulsion. It is given by:
ve=2GMRv_e = \sqrt{\frac{2GM}{R}}ve=R2GM
Where:
- vev_eve = Escape speed
- GGG, MMM, RRR have their usual meanings.
Satellites
Orbital Speed
The orbital speed of a satellite is the minimum speed required to keep it in orbit around the Earth. It is given by:
v=GMrv = \sqrt{\frac{GM}{r}}v=rGM
Where rrr is the radius of the orbit.
Time Period of a Satellite
The time taken by a satellite to complete one revolution around the Earth is:
T=2π(R+h)3gR2T = 2\pi \sqrt{\frac{(R+h)^3}{gR^2}}T=2πgR2(R+h)3
Energy of an Orbiting Satellite
The total mechanical energy of an orbiting satellite is the sum of its kinetic and potential energy:
E=−GMm2rE = \frac{-GMm}{2r}E=2r−GMm
Types of Satellites
Geostationary Satellites
- These satellites appear stationary to an observer on Earth.
- They are used for communication purposes.
- Time period: 24 hours.
Polar Satellites
- Polar satellites revolve in polar orbits around the Earth.
- They have a 90º angle of inclination with the equatorial plane.
- Used for mapping, weather observation, and environmental monitoring.
Weightlessness
Weightlessness is the condition in which an object experiences no net gravitational force, such as when it is in free fall or in a satellite orbiting the Earth.
Conclusion
This comprehensive guide on “Gravitation” provides an in-depth exploration of the fundamental aspects of physics as outlined in Class 11. It covers core concepts of gravitational forces, laws of planetary motion, and the relationship between mass, distance, and gravitational pull. The guide explains the significance of Newton’s law of universal gravitation, alongside its application to real-world scenarios like satellite motion and planetary orbits. Additionally, it delves into key topics such as acceleration due to gravity, gravitational potential energy, and escape velocity. Through detailed discussions, it ensures a strong grasp of both theoretical and practical aspects of gravitation in everyday phenomena and celestial mechanics.
Practice questions on Chapter 8 - Gravitation
Get your free Chapter 8 - Gravitation practice quiz of 20+ questions & detailed solutions
Practice Now








