Mechanical Properties of Fluids – Complete Guide For Class 11 Physics Chapter 10

Welcome to iPrep, your Learning Super App. Our learning resources for Chapter 9, “Mechanical Properties of Fluids,” in Class 11 Physics are meticulously designed to ensure students gain a comprehensive understanding of this essential topic. These resources include detailed notes on fluid pressure, Pascal’s Law, buoyancy, and the behavior of fluids in motion, ensuring a thorough grasp of fundamental principles. Topics such as Bernoulli’s Principle, streamline flow, and the equation of continuity are explained with real-life examples and practical applications. Additionally, visual aids like diagrams and tables are used to enhance conceptual clarity. This structured approach helps students build a solid foundation in fluid mechanics, crucial for higher studies.

What Are the Mechanical Properties of Fluids?

The concept of “Mechanical Properties of Fluids” in Class 11 Physics delves into the foundational principles of science by exploring the methods and standards used to quantify and describe physical phenomena. This chapter introduces students to the behavior of liquids and gases under various conditions, including pressure, flow, and viscosity. Key laws such as Pascal’s Law and Bernoulli’s Principle are examined in detail, providing a clear understanding of how fluids behave in static and dynamic scenarios. The chapter also highlights the practical applications of these principles in real-world systems like hydraulic machines and fluid measurement devices. Through a mix of theory and practical insights, students gain a deeper appreciation for the mechanics of fluids in nature and technology.

Shearing Stress in Fluids

• The shearing stress in fluids is significantly lower than that in solids, roughly a million times smaller.
• Even a small shear stress causes the shape of a liquid to change. 

Pressure

Everyday Example

When a sharp needle pierces the skin, it succeeds because of its small contact area, unlike a spoon’s back which does not pierce the skin even when pressed with the same force. This demonstrates the concept of pressure.

• Pressure is the amount of force exerted per unit area.

Fluid at Rest

A fluid at rest exerts a force that is always perpendicular to any surface immersed in it. If a force F acts on an area A, the pressure PPP is defined as:

P=F/A 

• Pressure is a scalar quantity with SI units of N/m2.
• The dimension of pressure is [ML−1T−2].
• Atmospheric pressure at sea level is approximately 1 atm=1.013×105 Pa 

Pascal’s Law

Pascal’s Law states that the pressure in a fluid at rest is the same at all points on a horizontal plane. Consider a prismatic element submerged in a liquid. The pressure at three different faces of the prism, each at the same depth, will be the same. This can be expressed as:

Pa=Fa/Aa, Pb=Fb/Bb=Pc=Fc/Cc

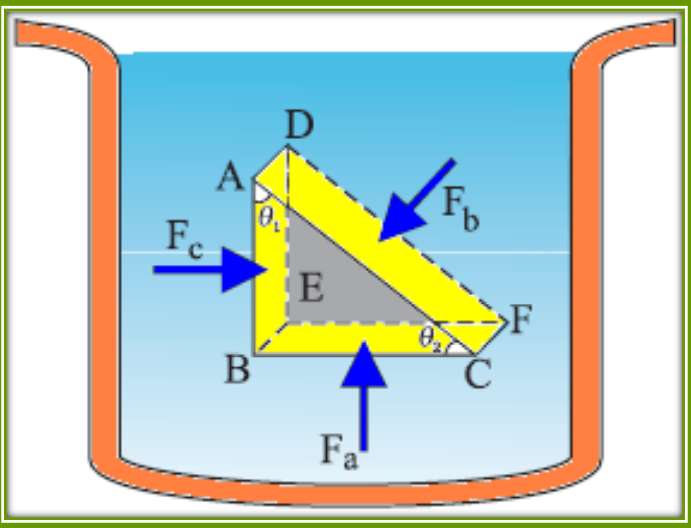
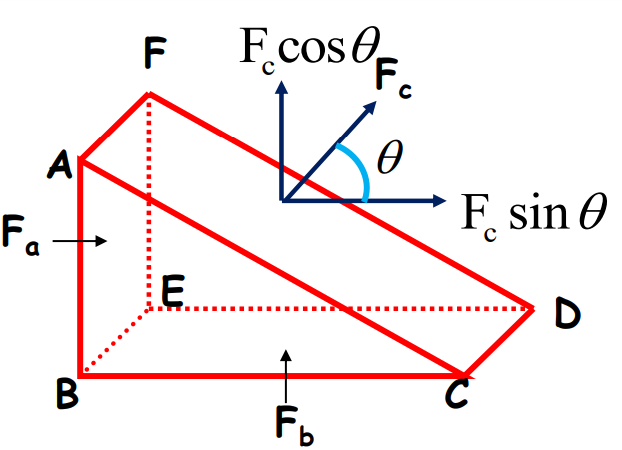
Where Pa, Pb, and Pc are the pressures on different faces of the prism.

Pascal’s Law for Transmission of Fluid Pressure

External pressure applied on a fluid in a confined space is transmitted equally in all directions without loss. This principle is utilized in hydraulic lifts and brakes.

For example, if a force F1​ is applied on a small piston with cross-sectional area A1​, the pressure is transmitted to a larger piston of area A2, generating a larger force F2. This relationship is given by:

F2=F1×A2/A1 

This is the principle behind mechanical advantage in hydraulic systems. 

Pressure Variation with Depth

Consider a cylindrical element of fluid of height h and base area A, with pressures P1 at the top and P2​ at the bottom. The difference in pressure between these two points is given by:

P2−P1=ρgh 

Where ρ is the density of the fluid and g is the acceleration due to gravity. This equation shows that pressure increases with depth in a fluid. 

Fluid Dynamics

Fluid dynamics deals with the behavior of fluids in motion. Steady flow occurs when the velocity of fluid particles at any point remains constant over time. However, if the velocity at any point varies with time, the flow becomes unsteady or turbulent.

Streamline Flow

A streamline represents the path taken by a fluid particle in a steady flow. The tangent to the streamline at any point gives the direction of the fluid velocity at that point. No two streamlines can intersect.

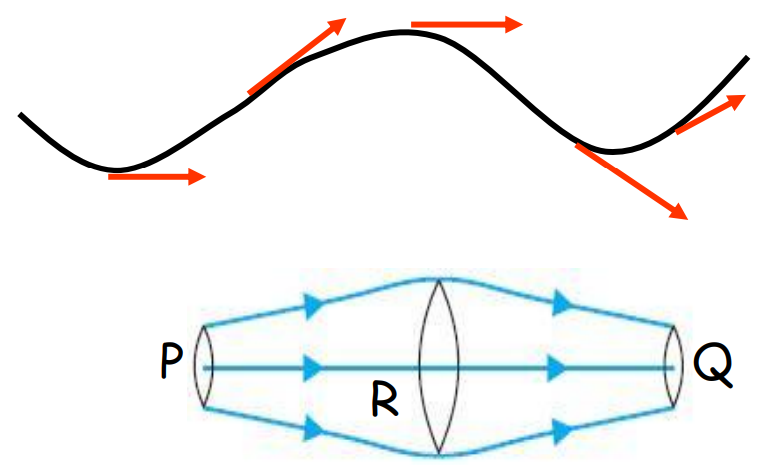
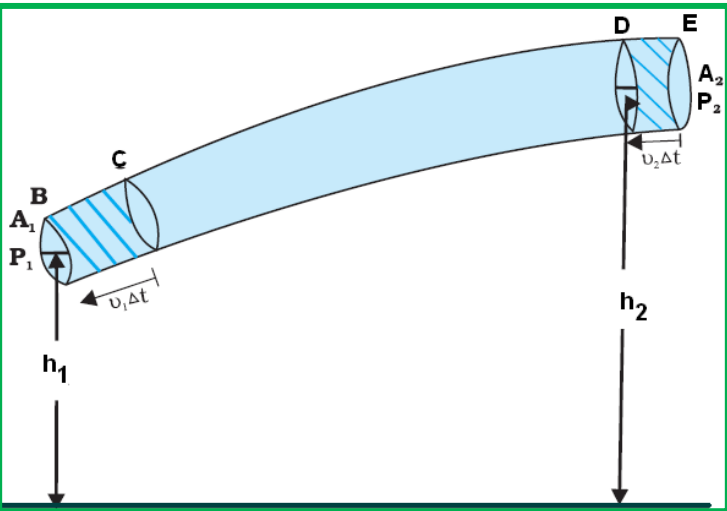
Equation of Continuity

For an incompressible fluid in streamline flow, the flow rate (volume of fluid passing through a section per unit time) remains constant. The equation of continuity is given by:

A1v1=A2v2 

Where A1 and A2​ are the cross-sectional areas at two points along the flow, and v1 and v2 are the respective velocities. 

Turbulent Flow

At higher velocities, fluid flow becomes irregular and turbulent, characterized by unpredictable changes in velocity and pressure. The critical velocity is the threshold at which flow transitions from streamline to turbulent. 

Bernoulli’s Principle

Bernoulli’s Principle is a manifestation of the conservation of energy in fluid dynamics. For an ideal fluid in motion (incompressible and non-viscous), the total mechanical energy remains constant throughout the flow:

P+ρgh+1/2ρv2=constant 

Where P is the pressure, ρ is the fluid density, g is the acceleration due to gravity, hhh is the height, and v is the velocity. 

Applications of Bernoulli’s Principle

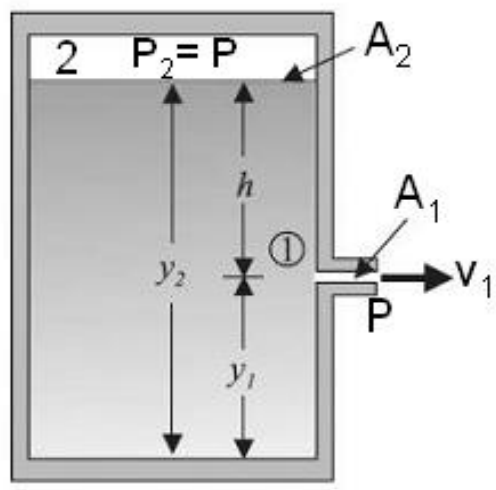
Torricelli’s Law

The velocity of efflux of a liquid from an orifice is equivalent to the velocity a freely falling object would acquire after falling from a height equal to the depth of the orifice below the liquid surface. The velocity v is given by:

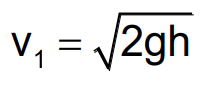
Where h is the height of the liquid column.

Venturi Meter

A Venturi meter is used to measure the flow speed of a fluid by taking advantage of Bernoulli’s principle. The meter consists of a tube with a constricted section. The pressure difference between the wide and narrow sections allows for the determination of fluid velocity.

Using the equation of continuity and Bernoulli’s theorem, the velocity in the wider section of the tube can be derived from:

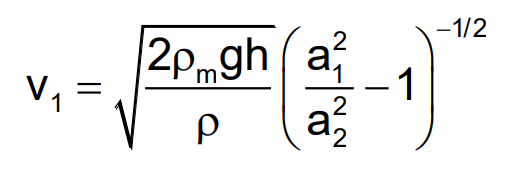
Where A1 and A2 are the cross-sectional areas at the wide and narrow sections, respectively.

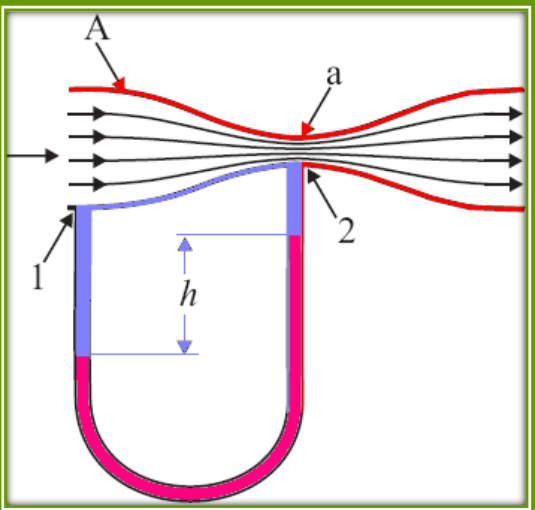
Applications of Venturi Meter

The Venturi meter is widely used in various applications:

• Spray Gun: The Venturi principle is applied in spray guns, where the venturi channel allows air to flow at high speed, creating suction for the fluid.

• Carburetors: In automobiles, the carburetor uses a venturi channel to regulate the air-fuel mixture.
• Other Applications: Filter pumps, Bunsen burners, atomizers, and sprayers also operate on the Venturi principle. 

Heart Attack and Fluid Dynamics

Artery Constriction

Arteries, responsible for carrying blood away from the heart, can become constricted due to the accumulation of plaque on their inner walls. This buildup increases blood flow pressure in the affected area, potentially causing the artery to collapse.

Mechanism Leading to Heart Attack

As the heart works to open the constricted artery, it exerts additional pressure. This process causes the internal pressure to drop, which may lead to the collapse of the artery. Continuous collapse can result in a heart attack. 

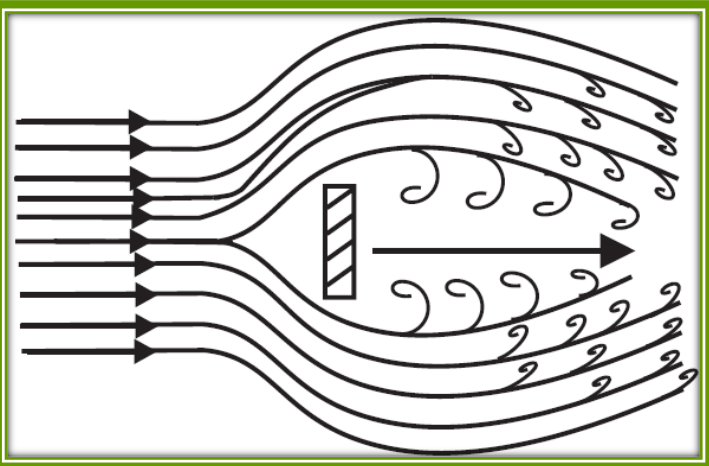
Dynamic Lift

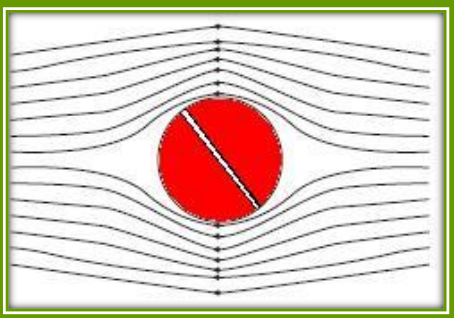
Streamlines Around a Non-Spinning Ball

• When a ball moves through the air without spinning, the velocity of the fluid (air) above and below the ball is equal.
• There is no vertical force acting on the ball.

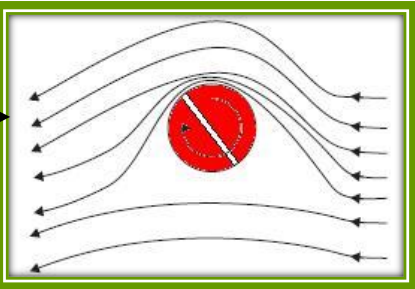
Streamlines for a Spinning Ball (Magnus Effect)

• When a ball spins, it drags air with it. The velocity of air above the ball is higher than below.
• This difference in velocity creates a dynamic lift called the Magnus effect, which explains the curved trajectory of a spinning ball.

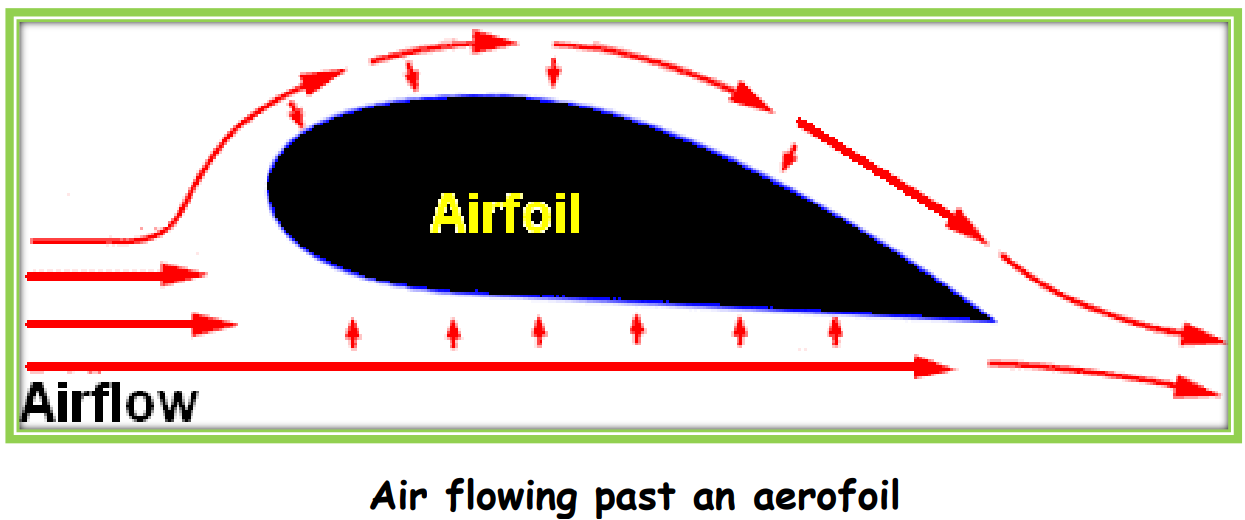
Air Flowing Past an Aerofoil

• An aerofoil provides an upward dynamic lift as it moves through the air.
• The streamlines are more crowded above the wing than below it, causing the air to move faster on top, generating an upward force that lifts the wing. 

Viscosity

Viscosity is the resistive force that comes into play when a fluid is in motion. It arises due to the relative motion between the fluid’s layers.

• Dependence on Temperature: The viscosity of liquids decreases with increasing temperature, while for gases, it increases.
• Units: The SI unit of viscosity is the poiseuille (pl), and it also has dimensions of [ML^-1T^-1].

Coefficient of Viscosity

The coefficient of viscosity is defined as the ratio of shearing stress to the strain rate. In mathematical form:

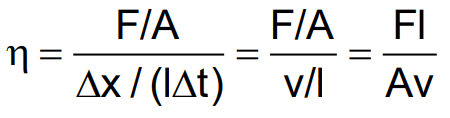
Stokes’ Law

When a spherical body moves through a viscous medium, a backward dragging force acts on it. According to Stokes’ law, this force is:

F=6πηrv 

Where:

• η is the coefficient of viscosity,
• r is the radius of the spherical body,
• v is the velocity of the body. 

Reynolds Number

The Reynolds number (Re) determines the flow nature of a liquid through a pipe:

Re=ρvd/η 

Where ρ is the density, v is the flow velocity, and d is the pipe diameter.

• 0 to 1000: Laminar or streamline flow.
• Above 2000: Turbulent flow.
• 1000 to 2000: Transition between laminar and turbulent flow. 

Surface Tension

Surface tension is a property of liquids that causes the liquid surface to behave like a stretched elastic membrane, minimizing its area.

• Formula: Surface tension (S) is given by:

S=F/l

Where F is the force, and lll is the length along which it acts.

Phenomena Related to Surface Tension

• Liquids form spherical drops.
• Mercury does not wet glass, while water does.
• Paintbrush hairs cling together when wet. 

Surface Energy

Surface energy is the work required to increase the surface area of a liquid. If a liquid’s free surface area is expanded, the work done against surface tension becomes the potential energy of the increased surface.

• Formula: Surface tension (S) is related to surface energy (E) by:

S=E/2ld

Where ld represents the increase in surface area. 

Determination of Surface Tension

A glass plate balanced horizontally near a liquid surface experiences a downward pull due to surface tension. By measuring the force required to lift the plate, the surface tension of the liquid can be determined using the formula:

S=W/2l 

Where W is the weight pulling the plate, and lll is the length of the plate. 

Angle of Contact

The angle of contact is the angle between the tangent to the liquid surface at the point of contact with a solid surface and the solid’s surface inside the liquid.

• Formula:

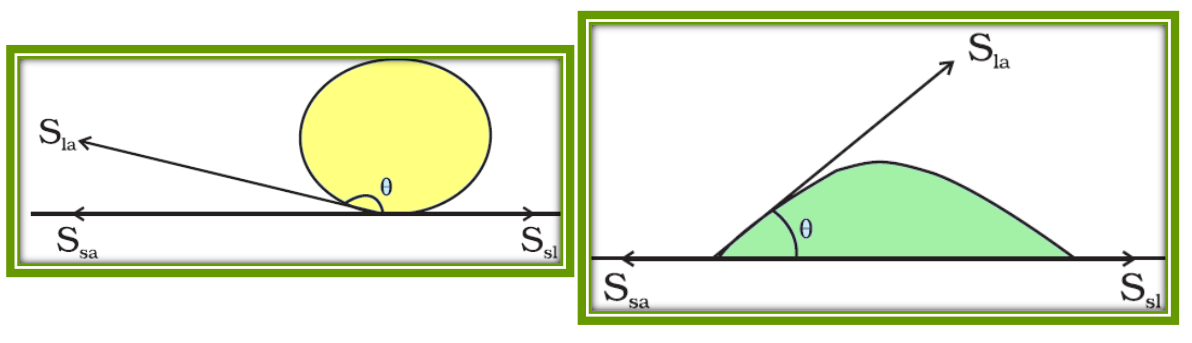
Where Ssa​, Ssl​, and Sla​ are the surface tensions of the solid-air, solid-liquid, and liquid-air interfaces, respectively. 

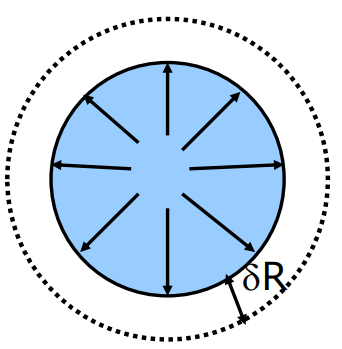
Excess Pressure Inside Liquid Drop and Bubble

For a liquid drop of radius R, the excess pressure inside is:

p=2S/R 

For a soap bubble with two surfaces, the excess pressure is:

p=4S/R 

Capillary Rise

The rise of liquid in a capillary tube is due to the pressure difference across the curved liquid-air interface. The height hhh to which the liquid rises is given by:

h=2Scos⁡θ/ρga 

Where S is surface tension, θ is the contact angle, ρ is the liquid density, ggg is the acceleration due to gravity, and aaa is the tube radius. 

Detergents

 The dirty greasy stains on clothes can not be cleared by simply washing them in water.  This is so because water does not find its contact with greasy dirt and hence, cannot wet such surfaces. Detergents contain molecules that are hairpin-shaped. The pointed ends attach to greasy stains, enabling water to interact with and a water-greasy-dirt interface is formed which finally wash away the dirt.

Conclusion

This comprehensive guide on “Mechanical Properties of Fluids” provides an in-depth exploration of the fundamental aspects of physics as outlined in Class 11. It covers the core concepts of fluid dynamics, pressure, Pascal’s Law, and Bernoulli’s Principle, helping students grasp how fluids behave under various conditions. Key topics such as pressure variation with depth, streamlined flow, turbulent flow, and practical applications like hydraulic lifts and Venturi meters are explained with clarity. The detailed explanation of these principles forms the foundation for understanding advanced topics in fluid mechanics. This guide is an essential resource for students aiming to excel in this chapter.