Motion in a Straight Line – Complete Guide For Class 11 Physics Chapter 2
Welcome to iPrep, your Learning Super App. Our learning resources for Chapter 2, “Motion in a Straight Line,” in Class 11 Physics are meticulously designed to ensure students gain a comprehensive understanding of this essential topic. These resources include detailed notes on fundamental concepts such as displacement, velocity, and acceleration, with a focus on motion along a straight path. Additionally, students will find step-by-step examples that illustrate the application of these concepts in various scenarios. The chapter also provides exercises and problem sets designed to reinforce understanding and encourage critical thinking. With these resources, students can build a solid foundation in kinematics, preparing them for more advanced topics in physics.
The concept of “Motion in a Straight Line” in Class 11 Physics delves into the foundational principles of science by exploring the methods and standards used to quantify and describe physical phenomena. This chapter introduces students to the fundamental aspects of kinematics, focusing on how objects move along a straight path. It covers critical topics such as displacement, velocity, and acceleration, providing a thorough understanding of how these quantities are measured and analyzed. The chapter also emphasizes the use of mathematical equations to describe motion, helping students develop analytical skills necessary for solving problems related to linear motion.
Motion in a Straight Line
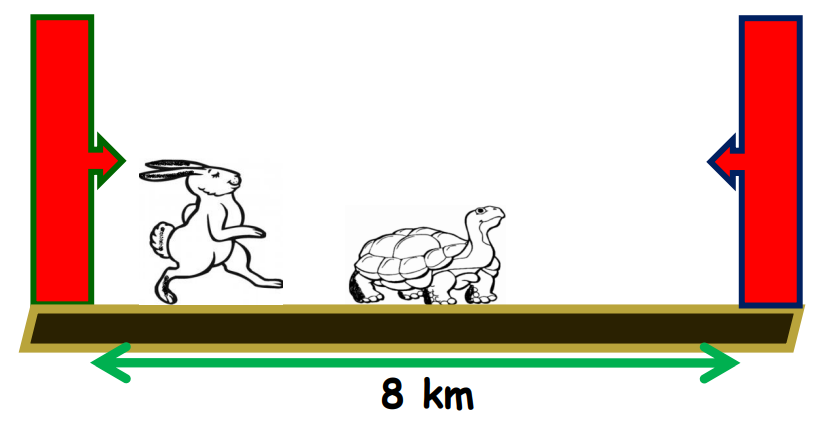
To illustrate the concept of motion, consider the classic race between a tortoise and a hare over a distance of 8.0 km. The hare runs 0.500 km and then stops for a 90.0-minute nap. Upon awakening, it runs twice as fast and finishes the course in a total time of 1.80 hours, winning the race. Let’s calculate the average speed of the hare and its average speed before it stopped for a nap.
Hint:
Average speed = total distance / total time
Explanation:
- Overall Average Speed:
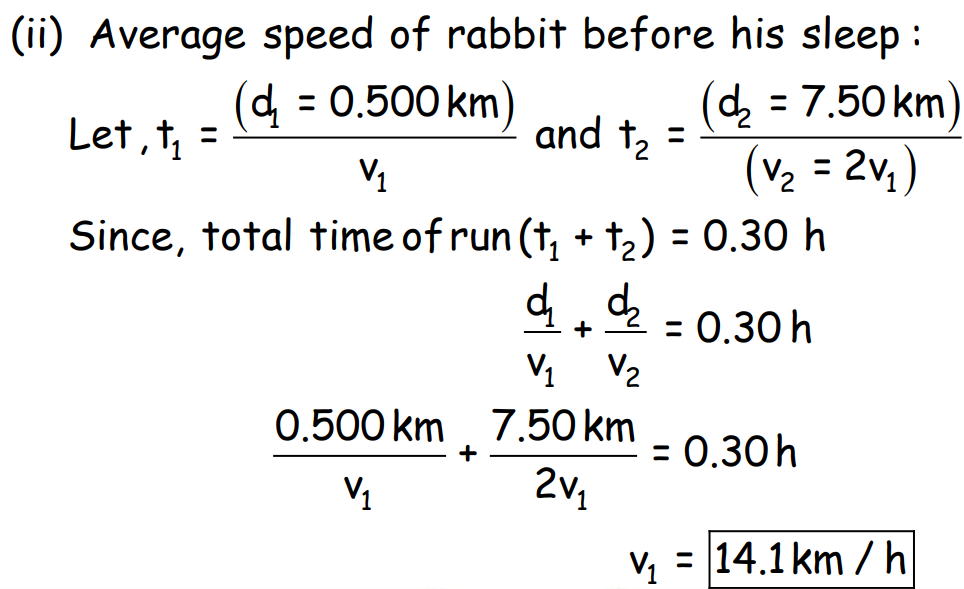
Average speed=total distance/total time=8.0 km/1.80 h=4.44 km/h - Average Speed of Hare Before Sleep:
- Distance before sleep, d1=0.500 km
- Distance after sleep, d2=7.50 km
Calculating Average Velocity
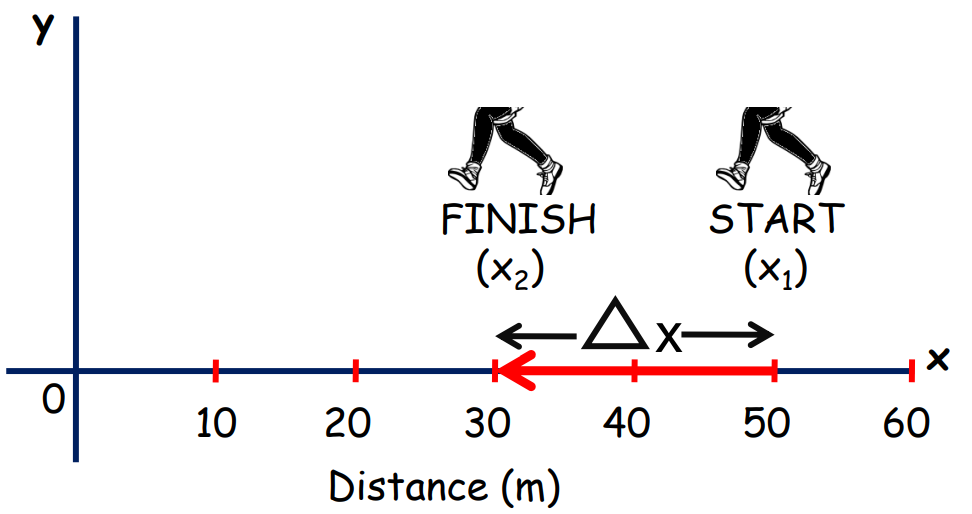
Consider an athlete moving along the x-axis of a coordinate system. Over 3.0 seconds, the athlete’s position changes from x1=50.0 m to x2=30.0 m. How do we find the athlete’s average velocity?
Hint:
Average velocity is the displacement divided by the elapsed time.
Explanation:
- Displacement (Δx):
Δx=x2−x1=30.0 m−50.0 m=−20.0 m - Time Interval (Δt\Delta tΔt):
Δt=3.0 s - Average Velocity (vvv):
v=ΔxΔt=−20.0 m3.0 s=−6.66 m/sv
Understanding Velocity and Acceleration
Suhael poses an intriguing question: “If the velocity of an object is zero, does it mean that the acceleration is zero? Conversely, if the acceleration is zero, does it mean the velocity is zero?” Let’s explore this concept with examples.
Hint:
Rate of change in velocity implies acceleration.
Explanation:
A zero velocity does not necessarily imply zero acceleration, nor does zero acceleration imply zero velocity. For example:
- Case 1: If a car at rest starts moving when the driver presses the accelerator, its initial velocity is zero, but its acceleration is not zero as the velocity changes.
- Case 2: If a person travels along a straight highway at a constant velocity of 50.0 km/h, the acceleration is zero because the velocity is constant.
Calculating Average Acceleration
Stanley’s car moves to the right along a straight highway (considered the positive x-axis). He applies the brakes. If the initial velocity is 10.0 m/s and it takes 5.0 seconds to slow down to 5.0 m/s, what will the average acceleration of his car be?
Hint:
Average acceleration is the change in velocity divided by the elapsed time.
Explanation:
- Initial and Final Velocities:
- Initial velocity, v1=10.0 m/s
- Final velocity, v2=5.0 m/s
- Time Interval (t2−t1):
- t1=0
- t2=5.0 s
- Average Acceleration (a):
Motion at Constant and Changing Velocities
Let’s consider three scenarios:
- A stone thrown vertically upwards rises to its highest point and falls back into the thrower’s hand.
- A bus starts from rest and speeds up to 10.0 m/s.
- A spacecraft drifts through space at a constant velocity.

Do any of these motions have points where the instantaneous velocity equals the average velocity over the entire motion?
Hint:
Explanation:
- Stone: The average velocity is zero because it returns to the starting point (displacement is zero). At the top, the instantaneous velocity is also zero.
- Bus: It will have every instantaneous velocity between 0 and 10.0 m/s. At some point, the instantaneous velocity equals the average velocity.
- Spacecraft: Since its instantaneous velocity is constant, the instantaneous velocity at any time equals the average velocity over any interval.
Estimating Average Acceleration
A baseball pitcher throws a fastball with a speed of 10.0 m/s. The average acceleration during the throw can be estimated if the pitcher accelerates the ball through a displacement of about 3.0 m from behind the body to the release point.
Hint:
v2=u2+2as
Explanation:
- Initial and Final Velocities:
- Initial velocity, u=0
- Final velocity, v=10.0 m/s
- Displacement (s):
- s=3.0 m
- Acceleration (a):
v2−u2=2as ⟹ a=(v2−u2)/ 2s
{ (10.0 m / s)2 – (0 m / s)2}/2(3.0 m) = a = 16.7 m / s2
Designing an Airbag System
Harry is designing an airbag system for a car that can protect the driver in a head-on collision at 27.0 m/s. How quickly must the airbag inflate to protect the driver, assuming the car crumples over a distance of about 1.0 m?


Hint:
v=u+at
Explanation:
- Initial and Final Velocities:
- Initial velocity, u=27.0 m/s
- Final velocity, v=0.0 m/s
- Distance (sss):
- s=1.0 m
- Deceleration (a):
a=-u2/2s=-(27.0 m/s)2/2(1.0 m)=-364.5 m/s2 - Time to Decelerate (t):
t=(v−u)/a=0−27.0 m/s/−364.5 m/s2=0.074 s
To be effective, the airbag must inflate faster than this.
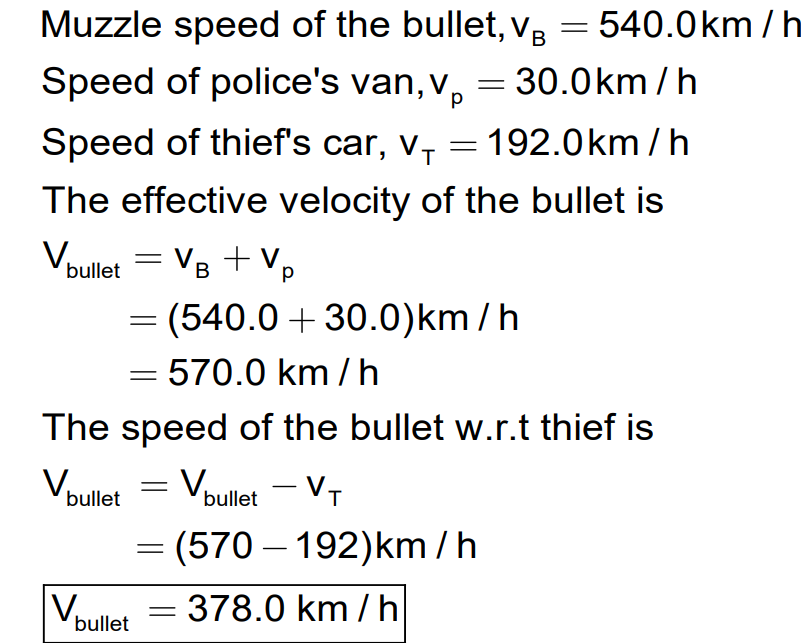
Catching the Thief
Sam, a detective, was sitting in a police van trying to catch a thief on a highway. He observed the speed of the van to be 30.0 km/h, while the thief’s car was moving at a speed of 192.0 km/h. To frighten the thief, a bullet was fired with a muzzle speed of 540.0 km/h. What should be the speed of the bullet to hit the thief’s car?
Given Data:
- Speed of police van, vp = 30.0 km/h
- Speed of thief’s car, vT = 192.0 km/h
- Muzzle speed of bullet, vB = 540.0 km/h
Solution:
To find the speed of the bullet relative to the thief’s car:
Stopping the Bus
Four friends were traveling in a bus moving at a speed of 35.0 m/s. Suddenly, the driver stopped the bus after covering a distance of 200.0 m. Calculate the retardation of the bus and the time taken to stop.
Given Data:
- Initial velocity, u = 35.0 m/s
- Final velocity, v = 0 m/s (since the bus stops)
- Distance covered, s = 200.0 m
Solution:
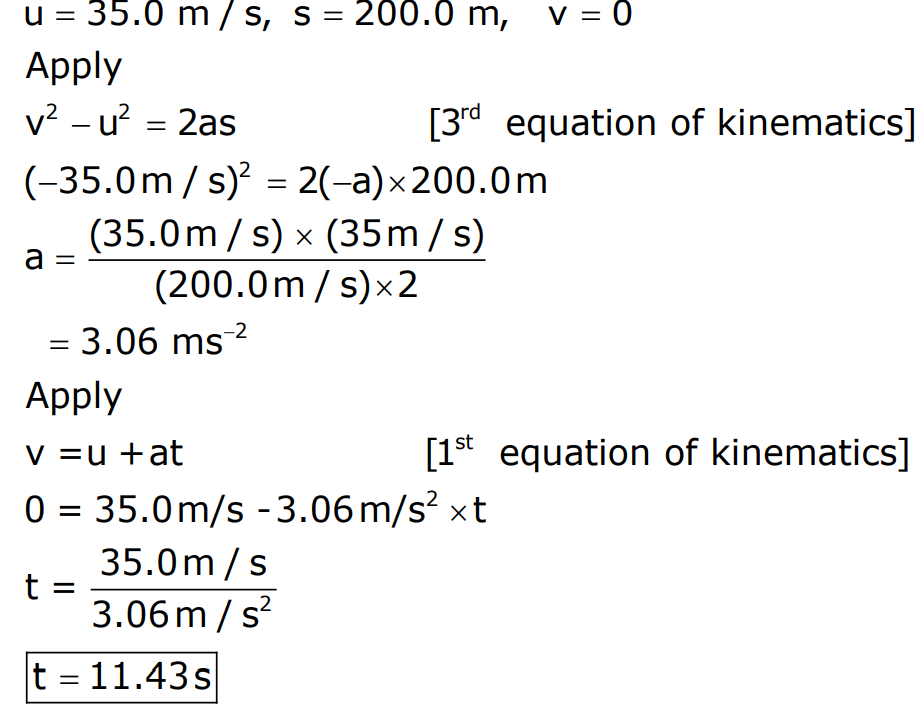
So, the retardation is 3.06 m/s² and the time taken to stop the bus is 11.43 seconds.
Acceleration of a Train
Shivani observed from the window of a train that the telegraph poles were spaced at regular intervals of 90.0 m. From a reference pole, she noticed that the next two successive poles were crossed at time intervals of 3.0 seconds and 2.0 seconds, respectively. Find the acceleration of the train assuming it to be constant.
Given Data:
- Distance between poles = 90.0 m
- Time for first interval, t1 = 3.0 s
- Time for second interval, t2 = 2.0 s
Solution:
- Using the second equation of kinematics:
- Solving the equations:

Average Velocity
Celina traveled to Mumbai in her car. She observed that she had traveled one-third of the distance with a velocity of 10.0 km/h, the next one-third with a velocity of 20.0 km/h, and 60.0 km/h in the last portion of the journey. Find the average velocity of the total journey.

Given Data:
- Velocity for the first part, v1 = 10.0 km/h
- Velocity for the second part, v2 = 20.0 km/h
- Velocity for the third part, v3 = 60.0 km/h
Solution:
- Calculating total time taken:
- Calculating average velocity:
Distance Covered After Braking
Mahima was driving her car at a speed of 20.0 m/s. Suddenly, she applied brakes, and the car came to rest in 25.0 seconds. Find the distance covered by the car during the time the brakes were applied.

Given Data:
- Initial velocity, u = 20.0 m/s
- Final velocity, v = 0 m/s
- Time taken, t = 25.0 s
Solution:
- Using the first equation of kinematics:
- Using the third equation of kinematics to find distance:
So, the distance covered by the car during braking is 250 meters.
Distance Covered by Sam
Sam went for a long drive with his family. He drove the car at a speed of 60.0 km/h with an acceleration of 10.0 km/h². Calculate the distance covered by Sam in 3.0 hours.
Given Data:
- Initial velocity, u = 60.0 km/h
- Acceleration, a = 10.0 km/h²
- Time, t = 3.0 h
Solution:
Using the second equation of kinematics:
So, the distance covered by Sam in 3 hours is 225 kilometers.
Relative Velocity of a Boat
Aditya was rowing a boat heading due north across a wide river with a speed of 12.0 km/h relative to the water. The water in the river had a uniform speed of 4.0 km/h due east relative to the Earth. How can we determine the velocity of the boat relative to an observer standing on either bank?
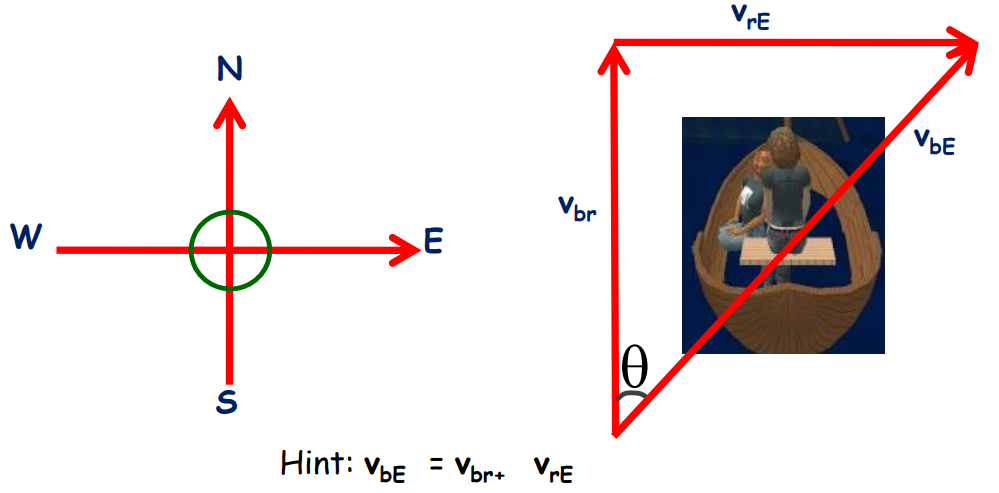
Solution:
Finding the velocity of the boat relative to the Earth:

Thus, the velocity of the boat relative to an observer on the bank is 12.65 km/h.
Conclusion
This comprehensive guide on “Motion in a Straight Line” provides an in-depth exploration of the fundamental aspects of physics as outlined in Class 11. It covers the core concepts of kinematics, including average speed, velocity, acceleration, and displacement, all in the context of motion along a straight path. Through various real-life examples and problem-solving techniques, the chapter helps students grasp these concepts intuitively. With detailed explanations, step-by-step calculations, and practical scenarios, this guide aims to build a solid foundation for understanding motion, an essential topic in physics. Let’s dive into each section to uncover the intricacies of motion in a straight line!
In conclusion, Chapter 2: Motion in a Straight Line provides a crucial foundation for understanding the principles of kinematics, an essential topic in Class 11 Physics. This chapter focuses on the key concepts of displacement, velocity, and acceleration, helping students comprehend how objects move along a straight path. By engaging with real-life examples, problem-solving techniques, and practical scenarios, students will strengthen their grasp of these important principles. As you delve deeper into Chapter 2: Motion in a Straight Line, you’ll gain valuable insights that will prepare you for more advanced topics in physics. Make sure to revisit the notes, practice problems, and exercises to master Chapter 2: Motion in a Straight Line fully.
Practice questions on Chapter 2 - Motion in a Straight Line
Get your free Chapter 2 - Motion in a Straight Line practice quiz of 20+ questions & detailed solutions
Practice Now








