Oscillations – Complete Guide For Class 11 Physics Chapter 14

Welcome to iPrep, your Learning Super App. Our learning resources for Chapter 14, “Oscillations,” in Class 11 Physics are meticulously designed to ensure students gain a comprehensive understanding of this essential topic. These resources include detailed notes on the principles of simple harmonic motion (SHM), periodic motion, and oscillatory systems, providing step-by-step explanations of key concepts. Students will find in-depth discussions on topics like the mathematical representation of SHM, energy exchange in oscillations, and the relationship between displacement, velocity, and acceleration. Additionally, our resources feature diagrams, graphs, and solved examples to enhance visual understanding. Practice problems and conceptual questions are also included to help students reinforce their knowledge and prepare for exams effectively.

What Are Oscillations?

The concept of “Oscillations” in Class 11 Physics delves into the foundational principles of science by exploring the methods and standards used to quantify and describe physical phenomena. This chapter introduces students to the nature of periodic motion, focusing on how oscillatory systems behave under various forces. Students learn about simple harmonic motion (SHM), the mathematical representation of oscillations, and the role of restoring forces in oscillatory systems. The chapter also covers energy transformations between kinetic and potential energy during oscillations, along with real-world applications such as pendulums and spring-mass systems. Additionally, it examines advanced topics like damped and forced oscillations, along with the concept of resonance in mechanical systems.

Non-Repetitive Motion

When we throw a ball into the air, it goes up and then comes down due to gravity, but this motion does not repeat after it lands on the ground.

Repetitive Motion

If we bounce a ball between the ground and our palms, the motion repeats over and over, making it a repetitive motion.

Periodic Motion

Periodic motion refers to a motion that repeats itself at regular intervals of time.

Examples of Periodic Motion

• Motion of the hands of a clock
• Revolution of a planet around the sun
• Swinging of a pendulum
• Vibration of a drum membrane
• Blades of a fan

Visual Example of Periodic Motion

The hands of a clock exhibit periodic motion.

Oscillatory Motion

Oscillatory motion is a to-and-fro motion about a mean position at regular intervals. An example is the movement of the bob of a simple pendulum.

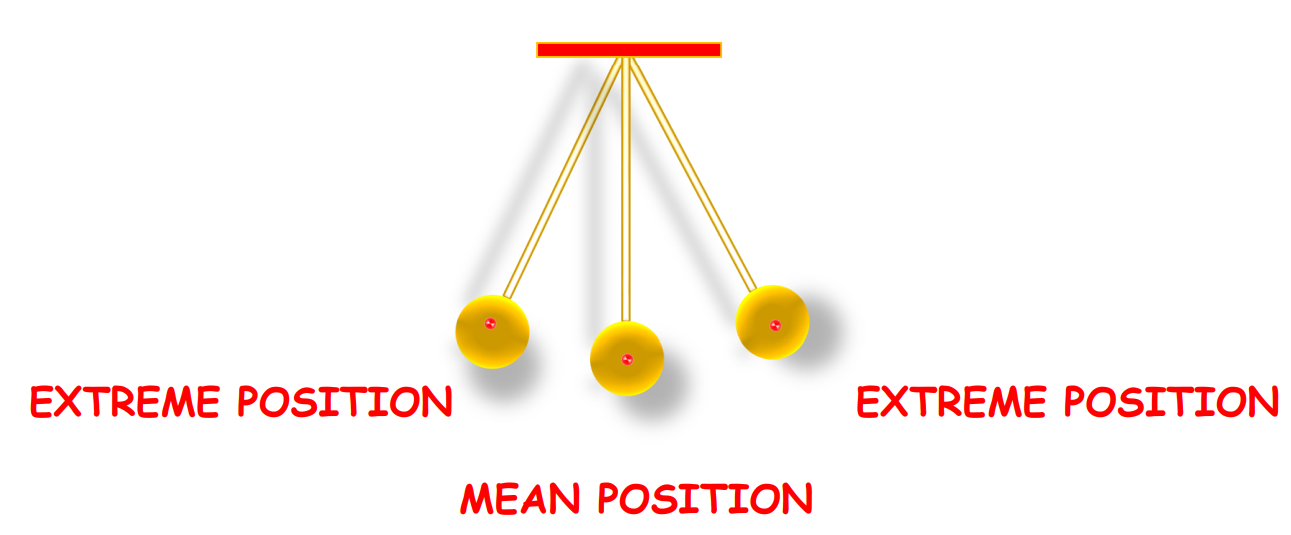
Difference Between Periodic and Oscillatory Motion

• Cause of Oscillations and Vibrations: If a body is displaced from its mean position, a force tries to return it to equilibrium, causing oscillations or vibrations.

• Periodic vs Oscillatory: Every oscillatory motion is periodic, but not all periodic motions are oscillatory. For example, the revolution of the Earth around the Sun is periodic but not oscillatory.

Oscillations vs Vibrations

• Oscillations: To-and-fro motion with low frequency (e.g., oscillation of a tree branch).
• Vibrations: To-and-fro motion with high frequency (e.g., vibration of a string in a musical instrument).

Simple Harmonic Motion (SHM)

Simple Harmonic Motion (SHM) is an oscillatory motion in which the restoring force is directly proportional to the displacement from the mean position and always directed towards it.

Period and Frequency

• Period (T): The time taken for one complete oscillation, measured in seconds (S).
• Frequency (f): The number of oscillations per second, measured in Hertz (Hz).

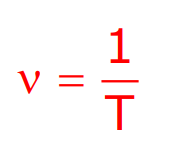
Displacement and Characteristics of SHM

• Displacement: The change in the position vector of a body with time, measured from the equilibrium position.
• Key Characteristics: In SHM, the body moves back and forth around the mean position with acceleration proportional to displacement.

Mathematical Representation of SHM

x(t)=Acos⁡(ωt+ϕ) 

Where:

• x(t) = displacement
• A = amplitude
• ω = angular frequency
• Φ = phase constant

Graphical Representation of SHM

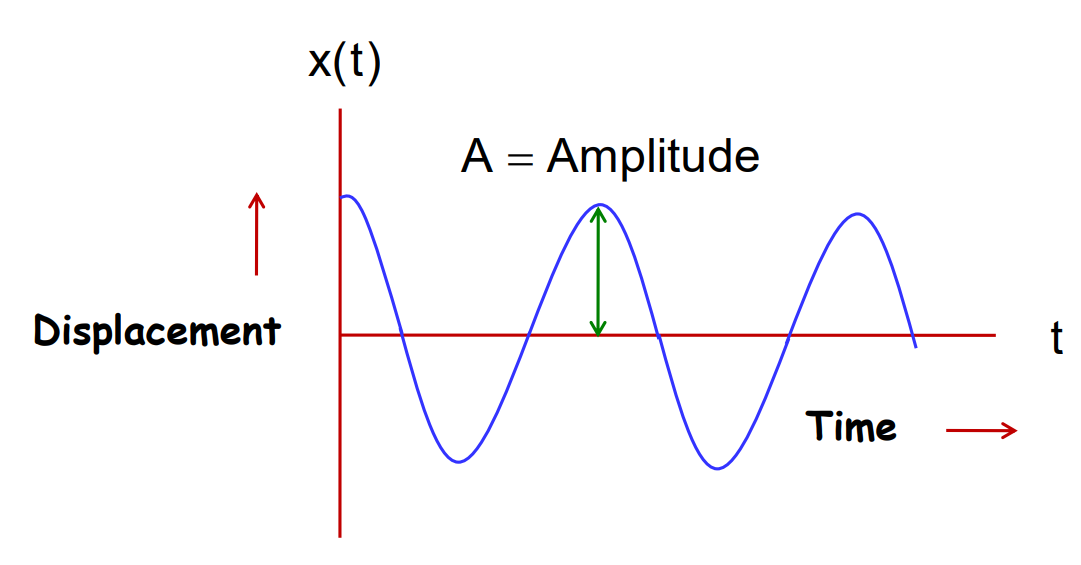
• The graph drawn between the displacement and time is a cosine wave.

SHM and Uniform Circular Motion

• Consider a particle executing uniform circular motion. 
• At any time, the angular position of the particle is represented by ωt+ϕ. The projection of this particle on the X-axis (denoted as P’) traces out a simple harmonic motion (SHM) along the diameter of the circle.

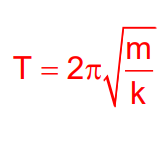
SHM and Uniform Circular Motion

A particle undergoing uniform circular motion projects a simple harmonic motion (SHM) when viewed along the diameter of the circle. The projection of the circular motion along a diameter represents SHM.

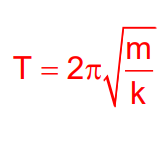
Velocity and Acceleration in SHM

• Velocity: The instantaneous velocity of a particle in SHM is given by:

• Acceleration: The acceleration is:

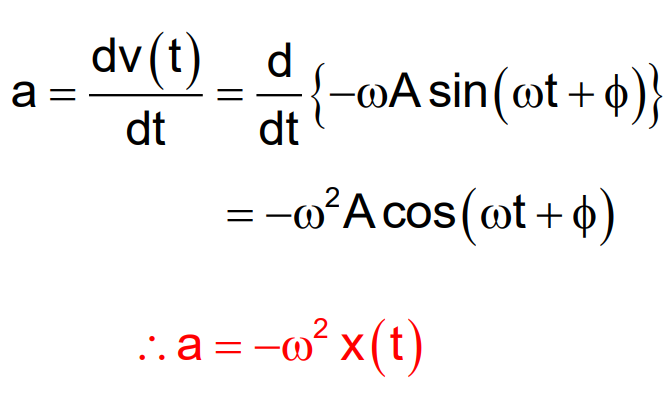
Thus, the acceleration is directly proportional to displacement and directed towards the mean position.

Graphical Representation of Displacement, Velocity, and Acceleration

The graphical representation of SHM for displacement, velocity, and acceleration shows the following relations:

Force Law for SHM

According to Newton’s second law:

F=ma=−kx(t) 

Where k is the force constant and F is the restoring force, which is time-dependent.

Linear and Non-Linear Harmonic Motion

• Linear Harmonic Oscillator: Restoring force is a linear function of displacement (Hooke’s Law).
• Non-Linear Harmonic Oscillator: Restoring force is a non-linear function of displacement.

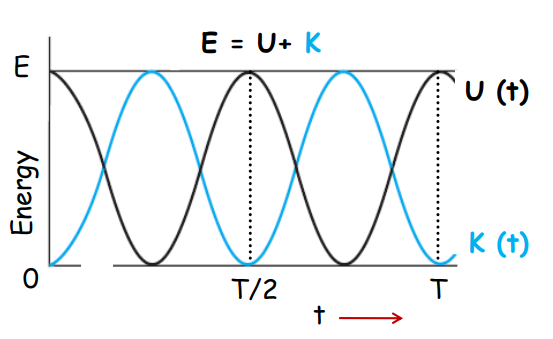
Energy in SHM

During SHM, there is a continuous exchange of kinetic energy (K.E.) and potential energy (P.E.).

Kinetic Energy

Potential Energy

It is maximum at the extreme positions and minimum at the mean position.

Total Energy

The total energy remains constant:

A graph of total energy versus displacement shows a constant value throughout the motion.

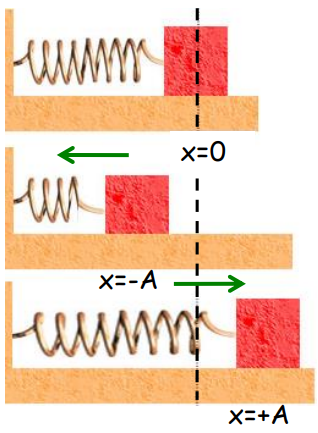
Oscillations Due to a Spring

In the case of a block attached to a spring, the restoring force follows Hooke’s law:

F(x)=−kx(t) 

Angular Velocity

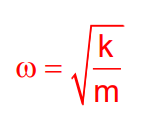
Period

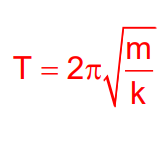
The system executes simple harmonic motion with:

Simple Pendulum

A simple pendulum consists of a point mass suspended by a weightless string in a uniform gravitational field. The restoring torque acting on the bob is proportional to the angular displacement, resulting in SHM for small angular displacements.

The period of the simple pendulum is:

T=2πLg Where L is the length of the pendulum and g is the acceleration due to gravity.

Damped and Forced Oscillations

Damped Harmonic Motion

In reality, oscillating systems experience damping due to frictional forces, causing the amplitude to decrease over time. The displacement is given by:

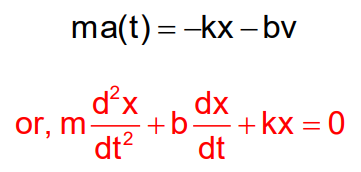
Forced Oscillations and Resonance

Forced Oscillations and Resonance

When an external periodic force acts on an oscillator, it undergoes forced oscillations. The amplitude of forced oscillations depends on the natural frequency and the driving frequency.

Resonance

Resonance occurs when the driving frequency matches the natural frequency, resulting in maximum amplitude. The condition for resonance is:

ωd=ω0; ωd/ω0 = 1

Resonance Curve

A resonance curve shows the variation in amplitude with frequency. Less damping results in a taller and narrower resonance peak.

Examples of Resonance

• Soldiers break steps while crossing bridges to avoid resonance.
• Earthquakes cause significant damage to buildings due to resonance effects.

Conclusion

This comprehensive guide on “Oscillations” provides an in-depth exploration of the fundamental aspects of physics as outlined in Class 11. It covers core concepts such as simple harmonic motion (SHM), the relationship between displacement, velocity, and acceleration, and how energy is conserved during oscillations. The guide also delves into more complex topics like damped and forced oscillations, explaining how external forces and resistive mediums affect motion. Additionally, the concept of resonance is thoroughly explored, highlighting its significance in mechanical systems. With detailed explanations and practical examples, this guide ensures a deep understanding of oscillatory motion and its applications in real-world scenarios.