Waves – Complete Guide For Class 11 Physics Chapter 15

Welcome to iPrep, your Learning Super App. Our learning resources for Chapter 5, “Waves,” in Class 11 Physics are meticulously designed to ensure students gain a comprehensive understanding of this essential topic. These resources include detailed notes on the various types of waves, such as transverse and longitudinal waves, and their distinct characteristics. The resources explain crucial concepts like wave speed, frequency, wavelength, and the mathematical representation of wave motion. Additionally, students will find in-depth explanations of wave phenomena, such as reflection, superposition, and the Doppler effect. With illustrative diagrams and real-world examples, these resources ensure clarity and a practical understanding of wave mechanics.

The concept of “Waves” in Class 11 Physics delves into the foundational principles of science by exploring the methods and standards used to quantify and describe physical phenomena. This chapter introduces students to the various types of waves, including mechanical, electromagnetic, and matter waves, and explains how they propagate through different mediums. It covers the mathematical representation of wave motion, focusing on important parameters like amplitude, wavelength, frequency, and velocity. Additionally, students learn about the principles of superposition, interference, and standing waves, which play crucial roles in understanding wave behavior. The chapter also discusses real-world phenomena such as the Doppler effect and beats, offering practical insights into the application of wave theory.

Introduction to Waves

Energy can be transported from one place to another in two ways:

1. By moving the matter carries kinetic energy.
2. By the vibration of particles in a medium, transferring energy from one particle to another without the matter moving itself. 

Types of Waves

Waves can be classified into three main types:

1. Mechanical Waves: Require a medium to propagate.
2. Electromagnetic Waves: Can travel through a vacuum.
3. Matter Waves: Associated with particles.

Types of Mechanical Waves

Mechanical waves are further classified as:

• Transverse Waves: The particles move perpendicular to the wave direction.
• Longitudinal Waves: The particles move parallel to the wave direction.

Transverse Waves

In transverse wave motion, particles of the medium vibrate perpendicular to the direction of the wave. These waves travel through a medium in the form of crests and troughs.

Crest and Trough

• Crest: The point where the medium is raised above its equilibrium position.
• Trough: The point where the medium is lowered below its equilibrium position.

Longitudinal Waves

In longitudinal wave motion, particles of the medium vibrate parallel to the wave direction.

These waves travel through a medium in the form of compressions (C) and rarefactions (R).

Compression and Rarefaction

• Compression: Region where particles are closer together.

• Rarefaction: Region where particles are farther apart, increasing volume and decreasing density.

Wave Function and Displacement Relation

A wave function describes the motion of a wave. For a progressive wave, the displacement relation is:

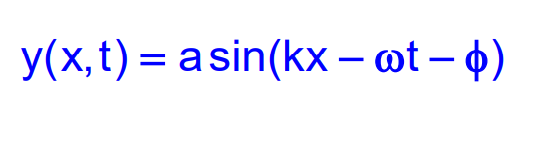
Where:

• y(x,t): Displacement at a point
• a: Amplitude
• k: Angular wavenumber
• ω: Angular frequency 

Key Terms in Wave Motion

• Amplitude: Maximum displacement of particles from their equilibrium position.

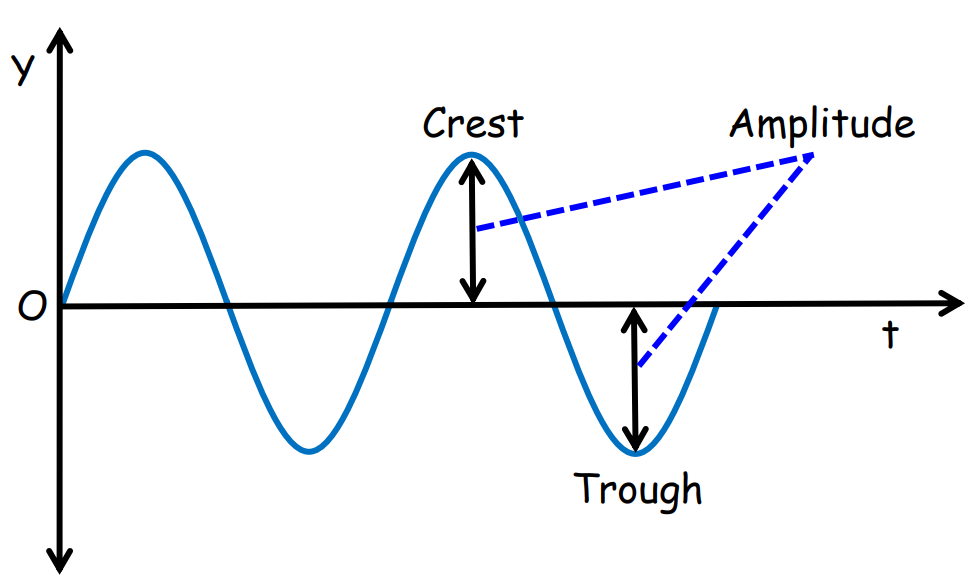
• Wavelength: The distance between two consecutive crests or troughs in a transverse wave, or compressions and rarefactions in a longitudinal wave.

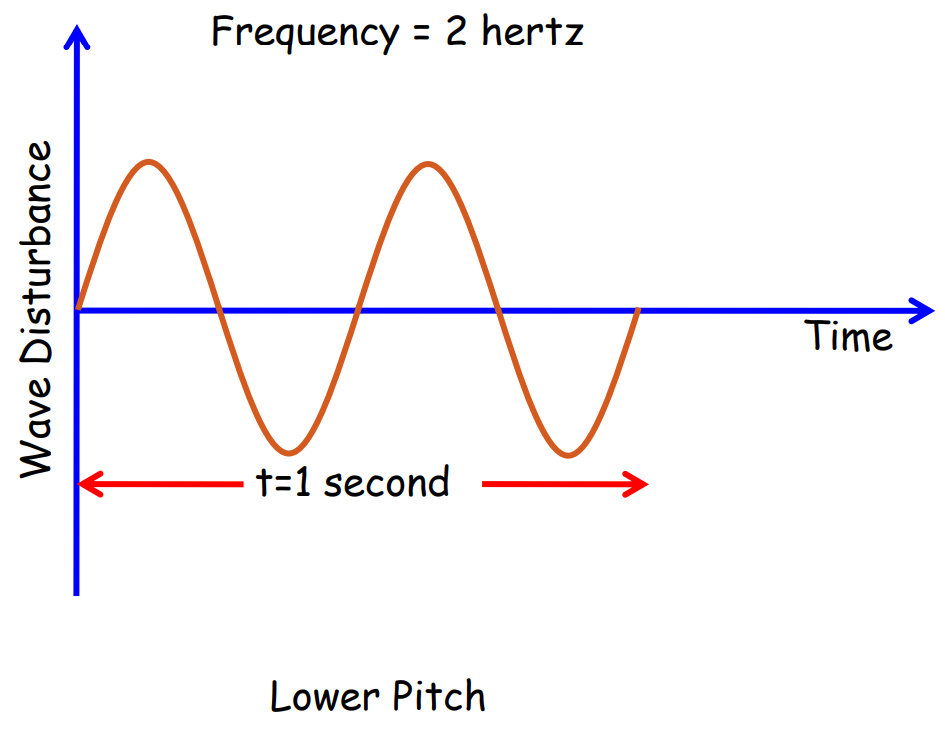
• Frequency: The number of complete cycles per second, measured in Hertz (Hz).

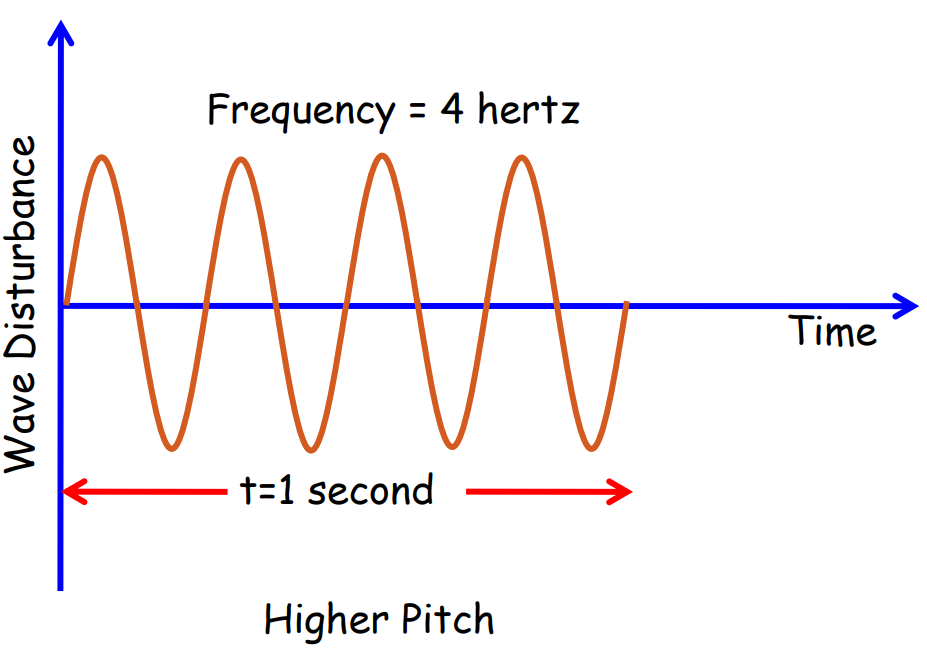
• Angular Frequency: The angular frequency of the wave is 2 times the frequency of the wave. It is represented by. 

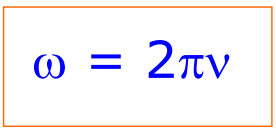
• It is measured in rad/s.
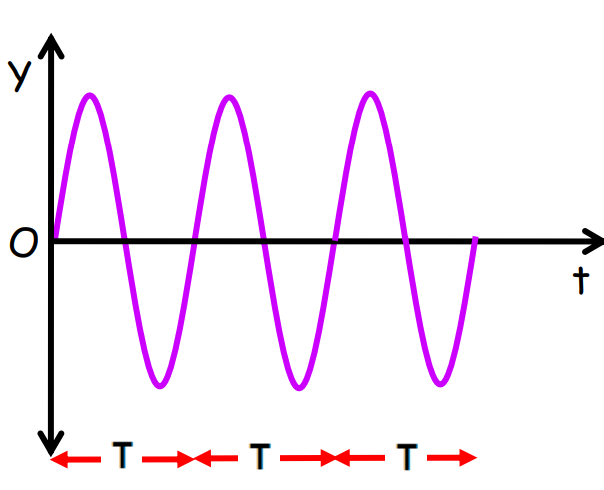
• Period: The time taken for one complete vibration, T=1f

Speed of a Travelling Wave

The speed of a wave is the product of its frequency and wavelength:

v=fλ 

For a transverse wave on a stretched string, the speed depends on the tension (T) and linear mass density (µ):

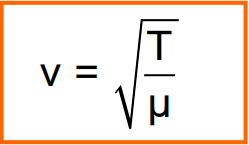
For a longitudinal wave, the speed depends on the bulk modulus (B) and density (ρ) of the medium:

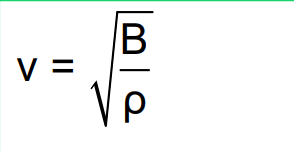
Newton’s Formula for Speed of Sound

• According to Newton, the compressions and rarefactions are formed slowly and there is hardly any change in the temperature of the system. 
• The heat produced during compressions is immediately lost to the surroundings. 
• The heat lost during rarefactions is gained from the surroundings immediately. 
• He concluded that the propagation of sound waves is an isothermal process. 
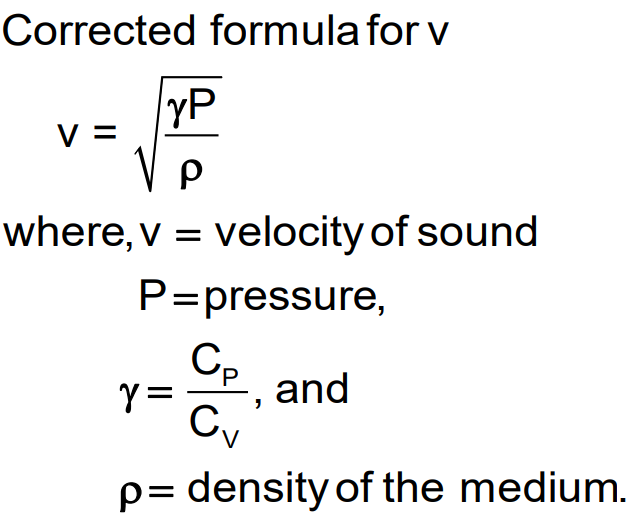
• Newton’s formula of sound is given by

• The value calculated based on Newton’s formula was less than the experimental value by 15%. Such a large error could not be taken as an experimental error.

Laplace’s Correction

According to Laplace, 

• The compressions and rarefactions are formed so rapidly that there is no exchange of heat between the surroundings and the system. 
• When sound waves propagate through gas, the change in pressure and volume of the gas is not isothermal, but adiabatic. Thus, Boyle’s law does not apply in this case. 
• The value of the speed of sound waves calculated is fairly close to the experimental value at N.T.P.

Superposition of Waves

The principle of superposition states that when two or more waves overlap, the resultant displacement at any point is the algebraic sum of the displacements due to each wave.

Mathematical Form of Superposition

If two waves y1(x,t) and y2(x,t) overlap, the resultant displacement (y(x,t)) is given by:

y(x,t)=y1(x,t)+y2(x,t) 

Suppose two harmonic waves are traveling in a positive x direction and have the same angular frequency (ω), wavelength (or same angular wave number, k), and amplitude(a), but differ in phase. Then, the resultant displacement is: 

Special Cases of Superposition

• In-phase: The resultant wave has double the amplitude.

• Out-of-phase: The resultant wave has zero amplitude. 

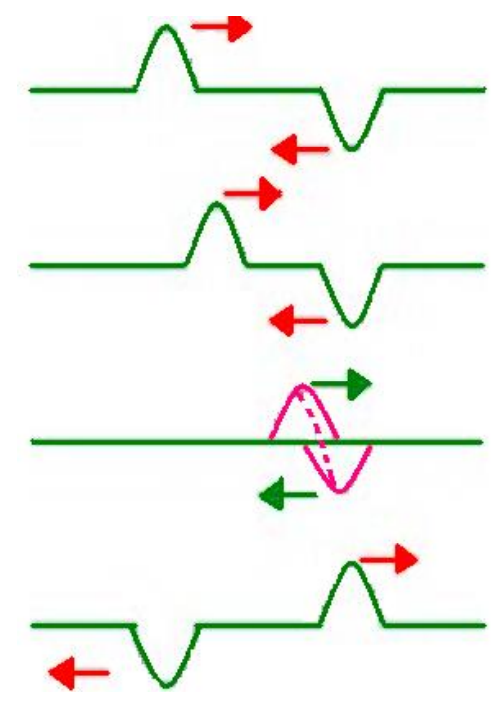
Reflection of Waves

Reflection at a Closed End

When a wave encounters a rigid boundary (such as a closed-end), it is reflected with an inversion. A crest is reflected as a trough and vice versa. It is represented as

Reflection at an Open End

At an open boundary, the wave is reflected without inversion, meaning a crest remains a crest, and a trough remains a trough. It is represented as

Standing Waves

Standing waves occur when two waves of the same frequency and amplitude travel in opposite directions, resulting in points of no motion (nodes) and points of maximum motion (antinodes).

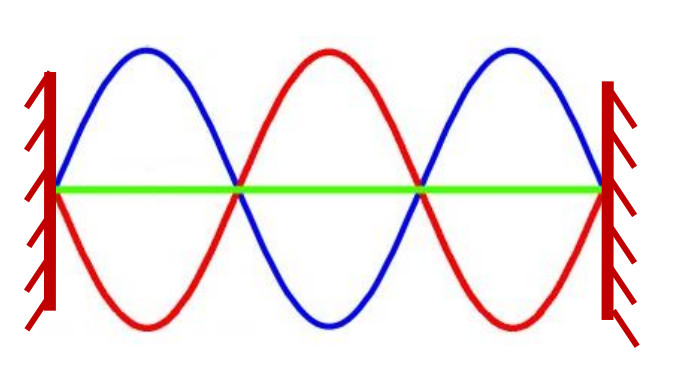
Normal Modes in Fixed Ends

The allowed frequencies for standing waves in a string fixed at both ends are:

Where v is the speed of the wave and L is the length of the string. 

Beats

Beats occur when two waves of slightly different frequencies interfere, causing an alternating increase and decrease in the intensity of sound. The beat frequency is given by:

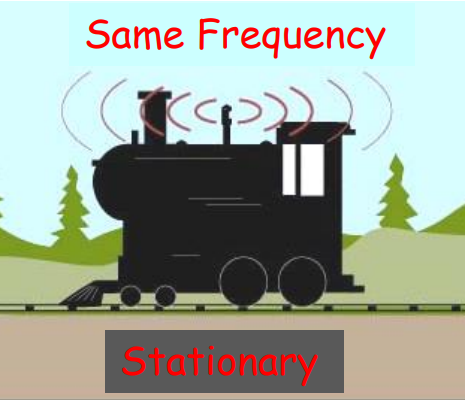
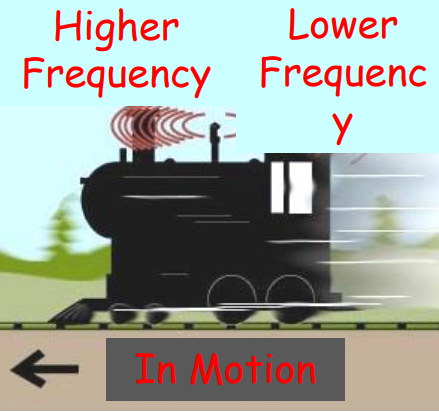
Doppler Effect

The Doppler Effect refers to the change in the frequency of a wave when there is relative motion between the source and the observer. 

The apparent frequency f′′ is given by:

Where:

• v: Speed of sound
• vo​: Speed of the observer
• vs: Speed of the source
• v: Actual frequency of the source

Special Cases of Doppler Effect

• When the source moves toward a stationary observer, the frequency increases.
• When the source moves away from the observer, the frequency decreases.

Conclusion

This comprehensive guide on “Waves” provides an in-depth exploration of the fundamental aspects of physics as outlined in Class 11. It covers the core concepts of units, measurements, and the importance of standardization in scientific studies. The guide explains the different types of waves, including mechanical and electromagnetic waves, and their characteristics. It delves into the mathematical representation of wave functions, displacement relations, and the principles behind wave motion. Additionally, the guide thoroughly discusses key phenomena like the Doppler effect, the superposition of waves, and the reflection of waves. With detailed examples and illustrations, it serves as a valuable resource for understanding wave mechanics in various contexts.