Application of Integrals – Complete Guide For Class 12 Math Chapter 8
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Application of Integrals in Mathematics for Class 12th are designed to ensure you grasp this concept with clarity and perfection. Whether studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best-integrated learning with interesting explanations and examples.
The chapter Application of Integrals explores the practical uses of definite integrals in various fields. It covers essential concepts such as calculating the area under curves and the area between two curves. The chapter explains how to determine the area of regions bounded by curves, the x-axis, and given limits, and introduces the area function to represent these regions. Additionally, students learn to use integrals to find the area enclosed by complex shapes that cannot be addressed by simple geometrical formulae. Overall, this chapter emphasizes the significant role of integrals in solving real-world problems in mathematics, physics, and engineering, making it a crucial component of advanced mathematical studies.
Application of Integrals
The Application of Integrals section delves into the practical uses of definite integrals, enabling students to calculate areas under curves and between boundaries, essential for solving real-world mathematical problems. As stated in the chapter Application of Integrals, the area of many simple geometrical figures can be calculated by using elementary formulae.
However, these formulae are inadequate for calculating the areas enclosed by
different curves. For this, we need some concept of integral calculus.
Definite Integral
Let ‘f’ be a function defined in the closed interval [a,b] and ‘φ’ be another function such that, φ'(x) = f(x) for all ‘x’ in the domain of ‘f’
Then
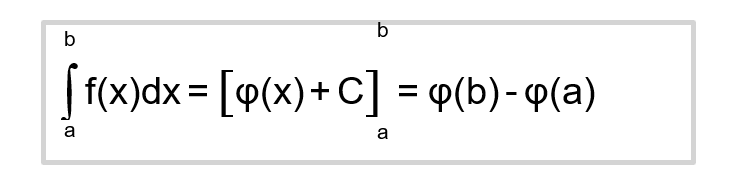
is called the definite integral of the function f(x) over the interval [a, b].
Area Function
Let us define abf(x)dx as the area of the region bounded by the curve y = f(x), the ordinates x = a and x = b and x-axis, where x is a point in [a,b].
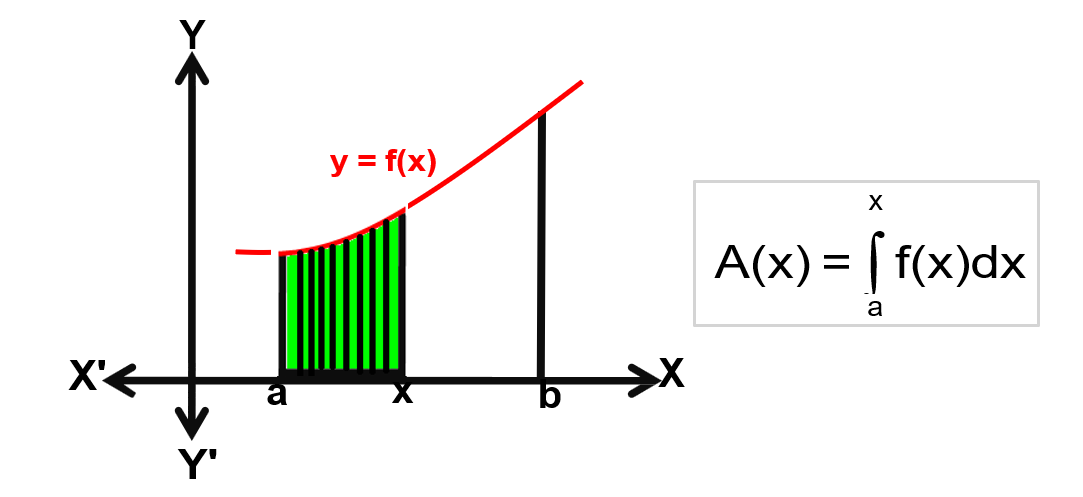
Then ax f(x)dx represents the area of the shaded region as shown in the figure and it depends on the value of x. We call this function of x an area function and denote it by A(x).
The area under Simple Curves
- Consider an arbitrary strip of height y and width dx, then
- dA (Area of the elementary strip) = ydx, where y = f(x).
Let f(x) be a continuous function defined on [a, b]. Then, the area bounded by the curve y = f(x), the x-axis and the ordinates x = a and x = b can be thought to be composed of a large number of very thin vertical strips.
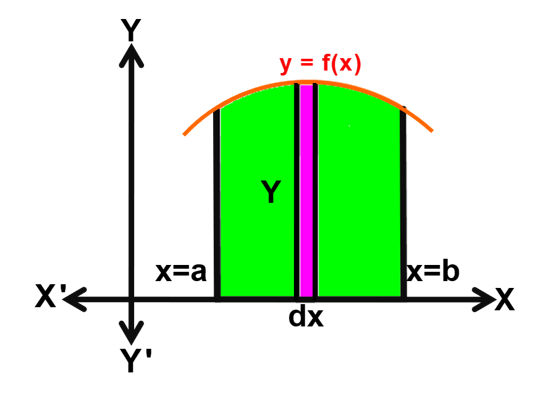
We can think of the total area A of the region between the x-axis, ordinates x = a, x = b, and the curve y = f (x) as the result of adding up the elementary areas of thin strips across the region.
Symbolically, we express it as
The area which is located at an arbitrary position within the region, that is specified by some value of x between a and b is called the elementary area.
A = ab y dx = ab f(x)dx
Remember:
If the curve y = f(x) lies below the x-axis, then the area bounded by the curve y = f(x), the x-axis, and the ordinates x = a and x = b are negative.
So, the area is given by | ab y dx |
Let g(y) be a continuous function defined on [c, d]. Then, the area bounded
by the curve x = g(y), the y-axis and the lines y = c and y = d is given by
cd g (y) dy or cd x dy
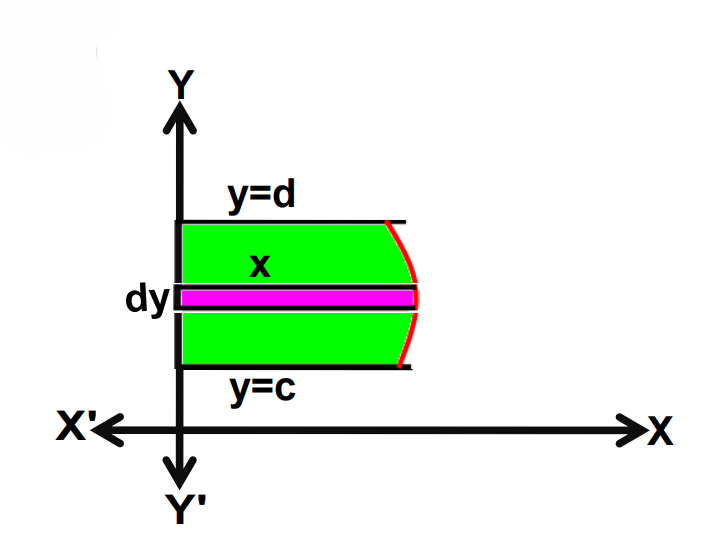
Remember:
Case I:- If the strip is parallel to the y–axis, then its width is taken as dx.
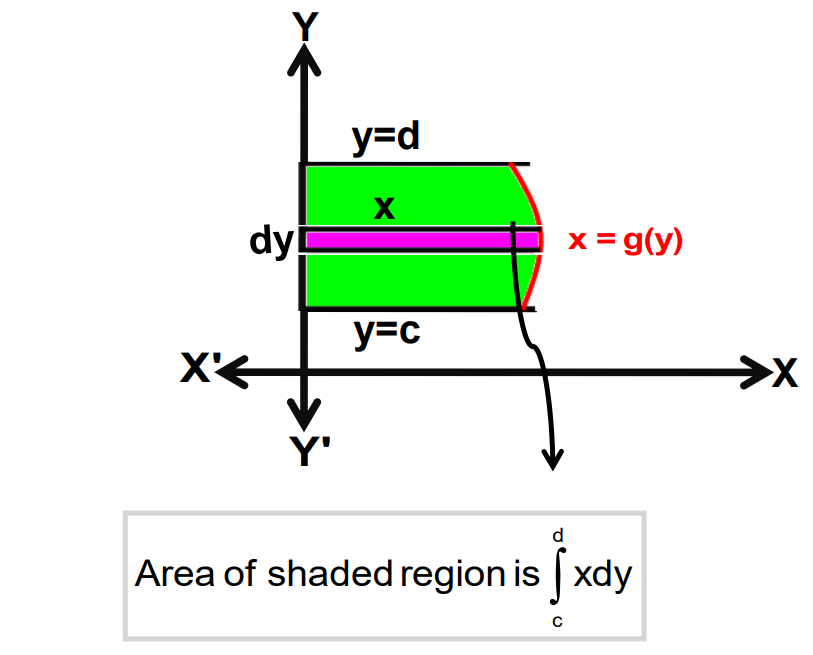
In this case, the approximating rectangle can move from x = a to x = b.
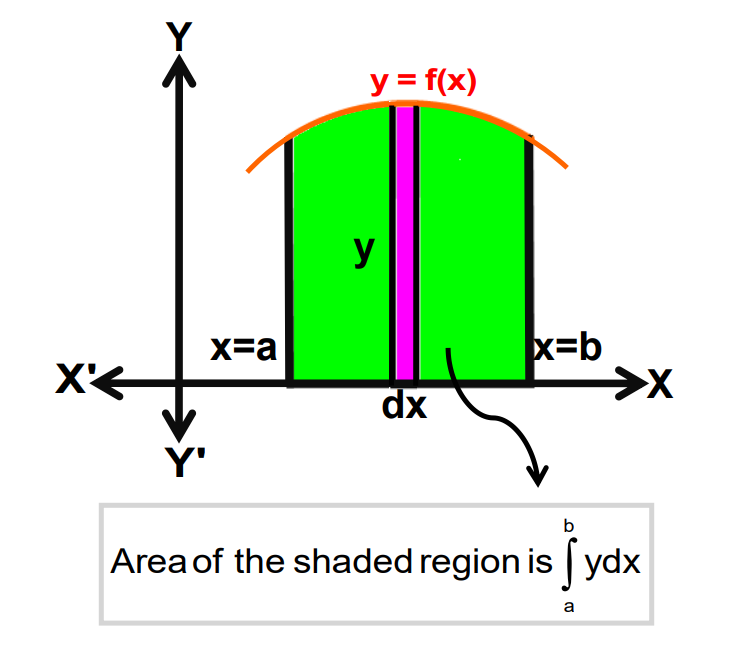
Case II:- If the strip is parallel to the x-axis, then its width is taken as dy.
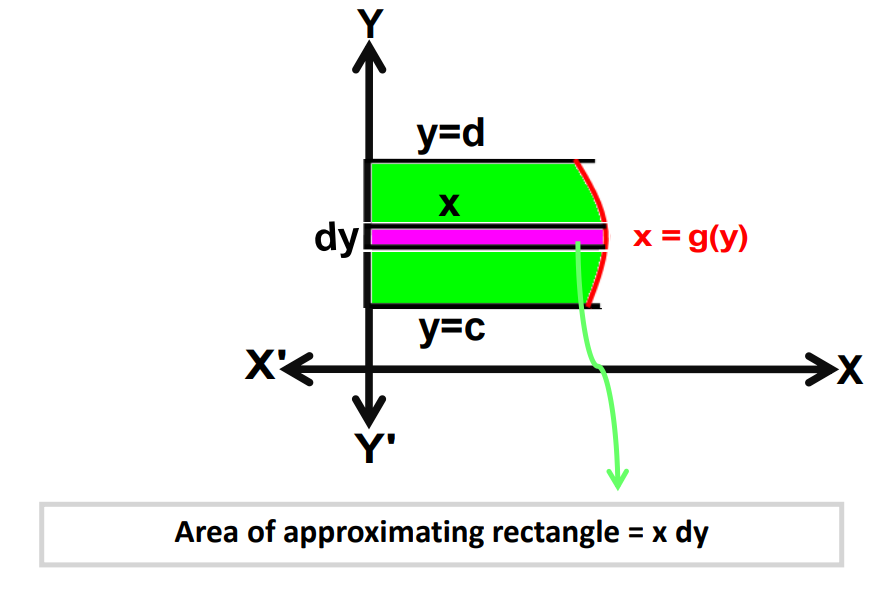
Remember:
In this case, the approximating rectangle can move from y = c to y = d.

Area Bounded by a Curve and a Line
Further in the chapter application of integrals, we’ll cover areas bounded by a curve and a line. Let’s find the area bounded by the parabola y² = 4ax and its latus rectum.

The equation of the latus rectum (for the parabola y² = 4ax) is given by x = a.
Refer to the figure. We have to find the area of the shaded region.
Since y² = 4ax is symmetrical about the x–axis,
Required area LOL’SL = 2(Area of LOSL)
We slice the area LOSL into vertical strips. Hence, the required area (say A) is
given by A = 2 0a y dy
Also, y² = 4ax y = ± √4ax
As the region LOSL lies in the first quadrant, y is taken as
positive. So,
A = 2 0a √4ax dx = 4 √a 0a √x dx
A = 4 √a [x³/₂ /3/2]
A = 4 √a x 2/3 a³/₂ = 8/3 a² square units.
The Area between Two Curves
The chapter application of integrals also covers the concept of area between two curves. Suppose two curves y = f(x) and y = g(x) as shown in the figure given below :
are given, where f(x)³g(x) in[a, b]

Here the point of intersection of these curves is given by x = a and x = b, obtained by common values of y from the given equations of curves.
The elementary strip has height f(x) – g(x) and width dx, so that the elementary area, dA = [f(x) – g(x)]dx and total area, A = 0a [f(x) – g(x)] dx.
Let’s Conclude
In conclusion, Chapter – Application of Integrals serves as a vital component of CBSE Class 12 Mathematics, equipping students with the tools necessary to tackle complex problems involving areas under curves. Through our engaging resources, including animated videos and practice questions, learners can grasp the intricacies of this chapter with ease. The practical applications of integrals, highlighted in Application of Integrals, showcase its relevance in various fields, from mathematics to physics and engineering. As you dive deeper into Chapter – Application of Integrals, remember that mastering these concepts not only prepares you for exams but also builds a solid foundation for future studies. Embrace the journey of learning, and let iPrep be your trusted guide in achieving excellence in mathematics!
Practice questions on Chapter 8 - Application of Integrals
Get your free Chapter 8 - Application of Integrals practice quiz of 20+ questions & detailed solutions
Practice Now








