Continuity and Differentiability – Complete Guide For Class 12 Math Chapter 5
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Continuity and Differentiability in Mathematics for Class 12th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
The chapter Continuity and Differentiability explores two fundamental concepts in calculus. Continuity of a function is discussed as the property where a function has no breaks, jumps, or holes in its graph, meaning it can be drawn without lifting the pen. A function is continuous at a point if the limit of the function as it approaches the point is equal to the function’s value at that point. Differentiability, on the other hand, is the measure of how a function changes as its input changes, defined through the derivative.
A function that is differentiable at a point is also continuous at that point, but the converse is not always true. The chapter also delves into important theorems like Rolle’s Theorem and the Mean Value Theorem, which provide conditions under which derivatives exist, and explores various rules for differentiation including the product, quotient, and chain rules, as well as derivatives of common algebraic, trigonometric, exponential, and logarithmic function.
Continuity and Differentiability
In mathematics, continuity and differentiability are fundamental concepts that help us understand the behavior of functions. Let’s start with a simple observation of graphs to illustrate these concepts.
Consider two different graphs:
Graph 1: This graph can be drawn without lifting the pen.
Graph 2: This graph cannot be drawn without lifting the pen.
- The first graph represents a continuous function.
- The second graph represents a discontinuous function.
In simpler terms, if you can draw the graph of a function without lifting your pen, the function is continuous. If you need to lift your pen, the function is discontinuous.
Continuity at a Point
As stated in the chapter Continuity and Differentiability, A function f is said to be continuous at x = a. if ‘f’ is defined in some neighborhood around a.
The limit of f(x) as x approaches a exists and is equal to f(a):
caf(x) = f(a)
i.e. xa⁻f(x) = xa⁺f(x) = f(a)
In other words, the function f is continuous at x = a if it has no breaks or jumps at that point. If a function is not continuous at a, then a is called a point of discontinuity.
Continuous Function: A function ‘f’ is said to be continuous function iff it is Continuous at each point of its domain. (i.e. wherever it is defined.)
Domain of Continuity: The set of all points where the function is continuous, is called its domain of continuity.
Discontinuous Functions
As mentioned in the chapter Continuity and Differentiability, A function may be discontinuous in a domain for several reasons:
- The limit does not exist at x = a.
- The left-hand limit and right-hand limit exist but are not equal:
xa⁻f(x) ≠ xa⁺ f(x)
Algebra of Continuous Functions
Let f and g be real-valued continuous functions defined in a neighborhood of a point ‘a’. Then :
(i) kf is continuous at ‘a’ for all k ∈ R
(ii) f±g is continuous at ‘a’
(iii) fg is continuous at ‘a’
(iv) f/g is continuous at ‘a’ , provided g(a) ≠ 0.
In other words, theorems about continuous functions include:
- Sum and Difference Rule: If f and g are continuous at x = a, then f±g is continuous at x = a.
- Product Rule: If f and g are continuous at x = a, then fg is continuous at x = a.
- Quotient Rule: If f and g are continuous at x = a, and g(a) ≠ 0, then f / g is continuous at x = a.
Continuous Functions
There are several key points around the continuous function mentioned in the chapter continuity and differentiability. These include-
Key Points:
- A polynomial function is continuous at each point of its domain.
- A rational function is continuous at each point of its domain.
- The exponential function ex is continuous at each point of its domain.
- The sine and cosine function is continuous at each point of its domain.
Example:
Find all points of discontinuity of f, where f is defined by
f(x) = { 2x+3, if x≤2,
2x−3, if x>2.
Its graph is:

To determine points of discontinuity
Evaluate limits from both sides at x = 2:
h0f(2+h) = h02(2+h) – 3 = 1
h0f(2-h) = h02(2-h) – 3 = 7
and f(2) = 2(2) + 3 = 7
Here the value of RHL ≠ LHL = f(2), therefore, the function is continuous at x = 2.
Theorem: Let ‘f’ and ‘g’ be real functions such that ‘fog’ is defined at a. If ‘g’ is continuous at a point ‘a’ and if ‘f’ is continuous at g (a), then (fog) is continuous at ‘a’.
Example: The function defined by f(x) = cos (x²) is a continuous function. Consider g(x) = cos x and h(x) = x².
Since both g and h are continuous functions, it can be deduced that f(x)= goh(x) is a continuous function.
Differentiability
The chapter Continuity and Differentiability gives a thorough understanding of the concept of Differentiability. Let’s understand with an example.
Consider the function f(x) whose graph is given below:
Now how will you determine the change in a particular interval? What is the behavior of this function?
- The small change at a point can be calculated by differentiation.
- A derivative is a measure of ‘How a function changes as its inputs change’.
In other words, if we find the slope of the graph at a given point then it is said to be the derivative of the function at that point.
So, Differentiability is concerned with how a function changes as you move from one point to another. The derivative of a function at a point c is defined as:
f’(c ) = h0f(c + h) − f(c) /h
If this limit exists, the function is said to be differentiable at c. Differentiability implies continuity, but continuity does not always imply differentiability.
Let’s understand
Suppose f is a real function and c is a point in its domain. The derivatives of f at c is defined by:
dy/dx = h0f(c + h) − f(c) /h
provided the limit exists. Derivative of f at c is denoted by f’(c) or d f(x)/dx Ic . The function defined by
dy/dx = h0f(x + h) − f(x) /h
wherever the limit exists is defined to be the derivative of f.
Theorem: If a function f is differentiable at a point c, then it is also continuous at that point.
The meaning of this theorem is that every derivable function is continuous but every continuous function may or may not be derivable.
Rules of Derivatives
If U and V are two functions then:
- Sum and Difference Rule:
(U±V)’ = U’±V’
- Product Rule:
(UV)’ = U′V + UV’
′
- Quotient Rule:
(U/V)′ = U′V−UV′ / V², where V ≠ 0
Derivatives of Common Functions
- Algebraic Functions:
- Constant k has a derivative of 0.
- xⁿ has a derivative of nxⁿ⁻¹
.
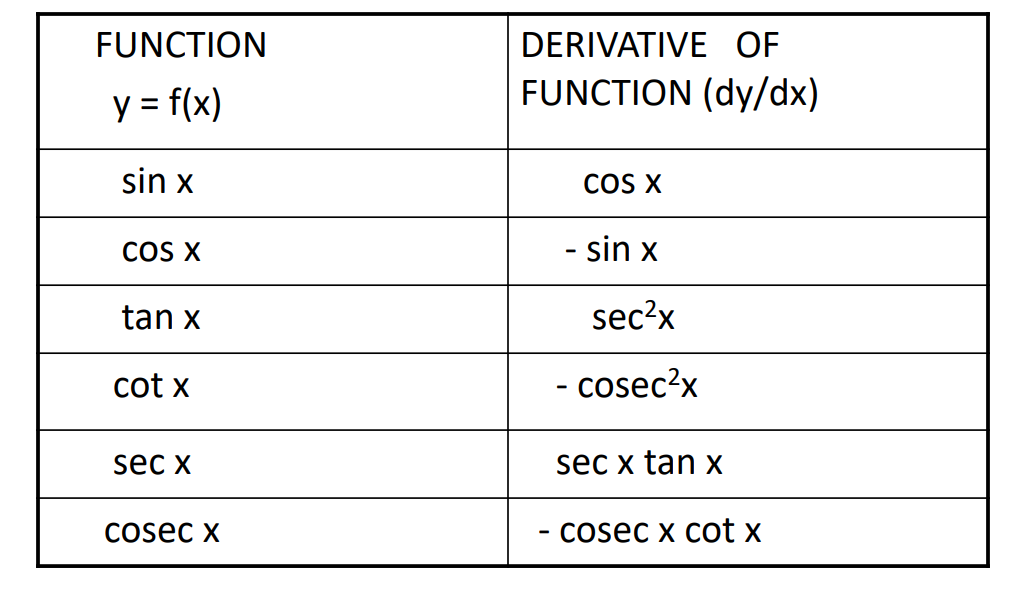
- Trigonometric Functions:
- Sin x has a derivative of cos x.
- cos x has a derivative of −sin x.
- tan x has a derivative of sec² x.
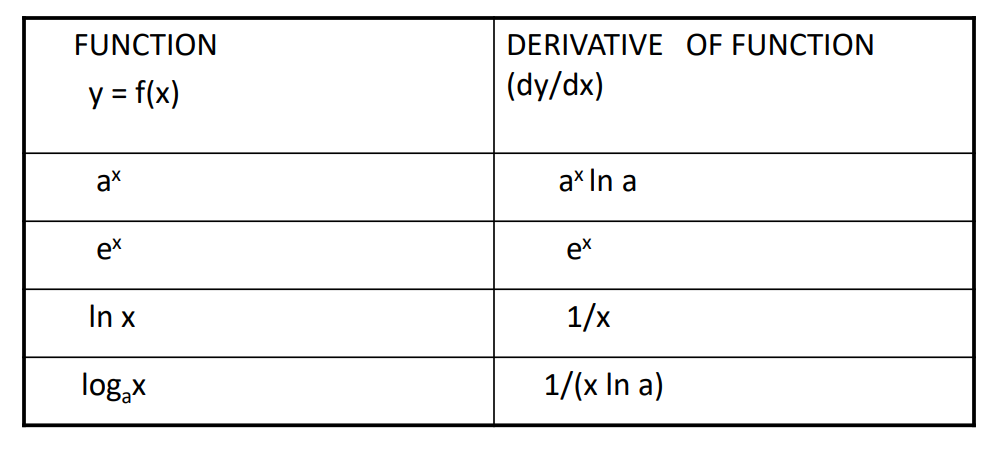
- Exponential and Logarithmic Functions:
- eˣhas a derivative of eˣ
- ln x has a derivative of 1/x
.
The Chain Rule
Given a composite function y = f(x), i.e. a function represented by y = f (u), u = g (x)
or y = f [g (x)], then y´ = dy/dx = (df/du) (du/dx)
This is called the chain rule.
The rule can be extended to any number of composite functions. e.g. If y = f (u (v (x))), then y´ = dy/dx = (df/du) (du/dv) (dv/dx)
Example: Find dy/dx when y = sin x²
Solution: Let u = x² y = sin u
dy/dx = (dy/du) (du/dx) = (cos u)(2x) = 2x cos x²
Explicit Differentiation
Definition: A variable y is said to be an explicit function of another variable x when the value of y is directly expressed in terms of x. If y = 3x² + 4x + 6, then y is an explicit function of x.
Implicit Differentiation
Definition: When the variables x and y are expressed in a functional relation, then either variable is an implicit function of the other. If x² + y² + 2axy + 2bx = 0, then x is an implicit function of y and vice-versa.
For functions defined implicitly, like
x² + y² = a²
Differentiate concerning x
2x+2y dx/dy = 0
or dx/dy =− x/y
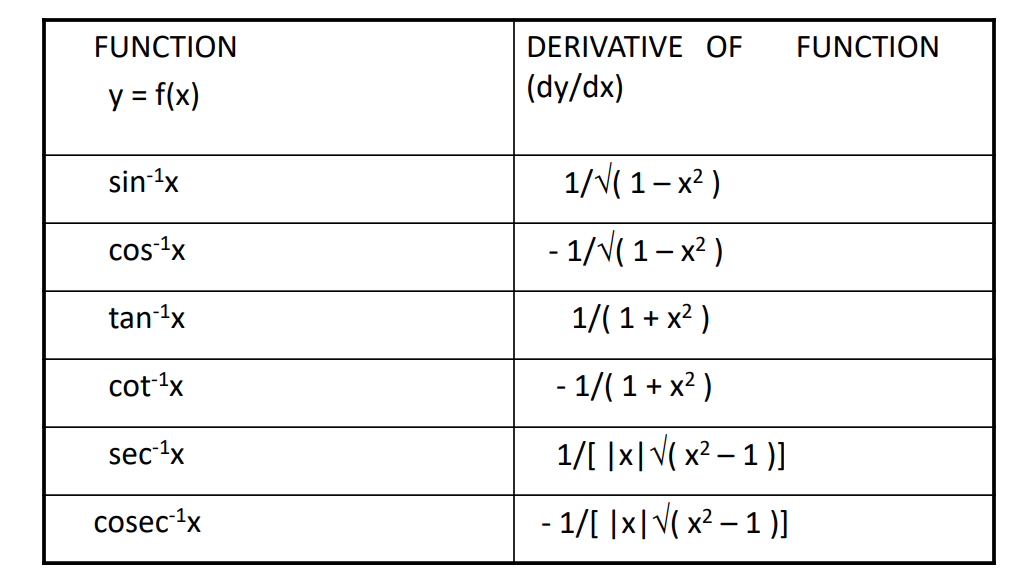
Derivatives of Inverse Trigonometric Functions
Exponential and Logarithmic Differentiation
Definition: The function y = f(x) = bˣ is called the exponential function with positive base b >1. y
Definition: For real numbers b >1, if bˣ = a, then the logarithm of a to the base b is x.
Thus logba = x if bˣ = a.
Thus logarithmic function is defined as logb: R⁺ → R. x → logbx = y if by = x.
As before if the base b = 10, we say it is common logarithms. Often natural logarithm is denoted by ln. But generally, we represent ln x as log x.
Property of log function
logbpq = logbp + logbq
logbpⁿ = n logbp
logb(x/y) = logbx – logby
Example:
Find dy/dx when y = esin x
Solution: dy/dx = d(esin x)/dx = esin x cos x.
Logarithmic Differentiation
If y = uv , where u and v are the functions of x.
Taking logs on both sides and differentiating w.r.t x.
d(log y)/dx = d (v log u)/dx
or (1/y)(dy/dx) = log u dv/dx + v d(logu)/dx
that implies
dy/dx = y [log u dv/dx + v/u du/dx]
dy/dx = uv [ log u dv/dx + v/u du/dx ]
Derivative of Parametric Function
If x = (t), y = (t),
then dy/dx is given by dy/dx = (dy /dt) / (dx/dt) = (d /dt) / (d/dt) = ’ (t)/ ’(t)
Example:
The function y of x is given by x = a cos t, y = a sin t. Find the derivative of y w.r.t. x at t = π /4.
Solution: dy/dx = [d(a sint)/dt] / [d(a cost)/dt]
= (cos t) / (- sin t) = (- cot t )
If we want to compute dy/dx at a particular point t, say at t = π /4, then [dy/dx]ₓ ₌ π/₄ = ( – cot π/4 ) = (-1).
Second Order Derivative
Definition: If a function f is differentiable at a point x, then its derivative f´ is called the first derivative or derivative of the first order of the function f.
If f´ is further differentiable at the same point x, then its derivative is called the second derivative or derivative of the second order of f at that point and is denoted by f´´ (read as an f-double dash).
Example: Find the second derivative of: y = sin x.
Solution: Given y = sin x
Therefore, dy/dx = cos x
And d²y/dx² = d/dx (dy/dx) = d(cosx)/dx = (-sin x ).
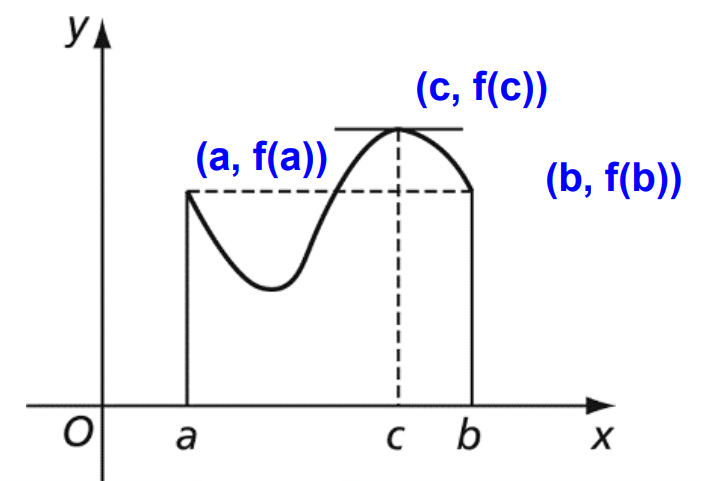
Mean Value Theorem
Rolle’s Theorem: If a function f is:
(i) continuous in the closed interval [a, b]
(ii) derivable in the open interval (a, b ) and
(iii) f(a) = f(b), then there exists at least one real number c in (a, b)such that f´(c) = 0.

Mean Value Theorem: If a function f is:
(i) continuous in the closed interval [a, b] and
(ii) derivable in the open interval (a, b) then there exists at least one real number c in (a, b) such that f´(c) = [f(b) – f(a)] /(b – a)

Example: Verify the Mean Value Theorem for f(x) = x²+2x+3 in the interval [4,6]
Solution: Given f(x) = x² + 2x + 3
(i) f(x) being a polynomial function is continuous in [4, 6].
(ii) f(x) being a polynomial function is derivable in (4, 6). Thus, both the conditions of the mean value theorem are satisfied.
Therefore, there exists at least one real number c in (4, 6) such that f´(c) = [f(b)-f(a)] /(b-a)
or 2c + 2 = [f(6)-f(4)] /(6-4) (since f’(x) = 2x + 2)
2c + 2 = [51 – 27] /(6-4)
2c + 2 = 24/2 = 12
2c = 12 – 2 = 10 or c = 5.
Thus there exists c = 5 in ( 4, 6 ).
Hence Mean Value theorem is verified and c = 5.
Let’s Conclude
In conclusion, CBSE Class 12th Math, Chapter 5 – Continuity and Differentiability provides a foundational understanding of two critical concepts in calculus. Mastering the principles of continuity ensures that you can identify the behavior of functions without interruptions, while differentiability equips you with the tools to analyze how these functions change. By studying this chapter thoroughly, you will grasp important theorems such as Rolle’s Theorem and the Mean Value Theorem, which play a crucial role in connecting the concepts of continuity and differentiability.
At iPrep, we strive to offer engaging resources to help you navigate CBSE Class 12th Math, Chapter 5 – Continuity and Differentiability with confidence. Remember, the journey of mastering mathematics is not just about memorizing formulas; it’s about understanding the underlying principles that govern the behavior of functions. So, as you continue your studies, keep revisiting the key concepts in Continuity and Differentiability, and make use of our animated videos, practice questions, and notes to solidify your learning. Embrace the challenge, and let iPrep be your partner in achieving excellence in your mathematics journey!
Practice questions on Chapter 5 - Continuity and Differentiability
Get your free Chapter 5 - Continuity and Differentiability practice quiz of 20+ questions & detailed solutions
Practice Now








