Vector Algebra – Complete Guide For Class 12 Math Chapter 10

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Vector Algebra in Mathematics for Class 12th are designed to ensure you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Vector Algebra introduces students to the essential concepts of vectors, which are quantities with both magnitude and direction. The chapter covers fundamental topics such as directed line segments, where vectors are represented, and their various types, including zero vectors, unit vectors, and collinear vectors. Key operations include vector addition, scalar multiplication, and the computation of scalar (dot) and vector (cross) products.

The chapter also explores the properties and applications of these operations, such as the triangle and parallelogram laws for vector addition and the use of dot and cross products to find projections and areas of parallelograms and triangles. Vector Algebra is crucial for understanding spatial relationships and solving problems in physics, engineering, and computer graphics, making it a vital component of advanced mathematics education.

Vector Algebra

This chapter on vector algebra explores the world of Vector Algebra, a fundamental aspect of mathematics that bridges the gap between abstract concepts and their practical applications. 

Let’s understand 

Scalars and Vectors

In our daily lives, we encounter quantities that have only magnitude, such as time, volume, and mass. These are known as scalars. For instance, as mentioned in the chapter on vector algebra, the time it takes to watch a football match or the volume of water in a bottle are scalar quantities. However, in certain situations, we deal with quantities that have both magnitude and direction. These quantities are known as vectors. An example is the force applied by a player when hitting a football.

Directed Line Segment

Let us observe the following line segments.

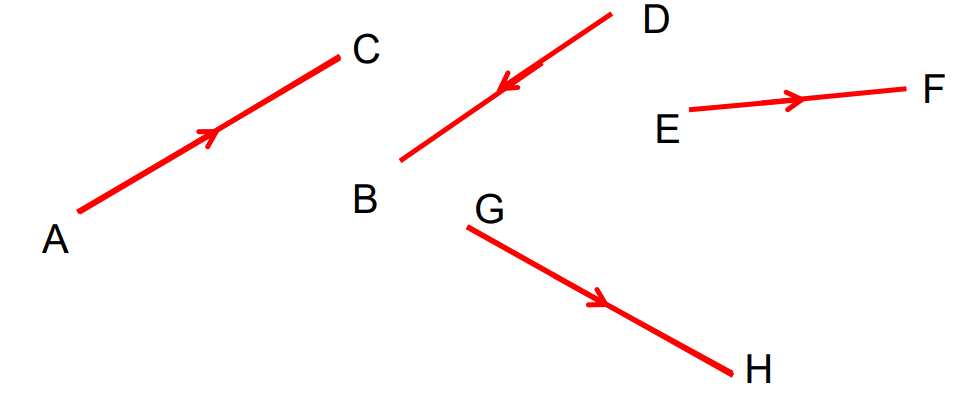
A line segment that has a particular direction is called a directed line segment.

Vector

A vector is a directed line segment. 

Therefore, DE = a is a vector.

The distance between the initial point and the terminal point is called the length ( or magnitude) of the vector.

The magnitude of the vector DE or a is denoted as |DE| or |a| 

Position Vector

As per the chapter Vector Algebra, OP is called the position vector of P concerning O.

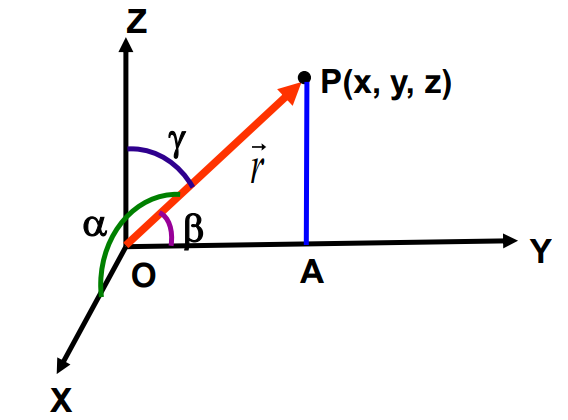
Direction Cosines

Suppose a vector r makes the angles with α,β, γ the positive direction of the x, y, and z -axes respectively. cos α, cos β, and cos γ are called direction cosines of the vector r and are denoted by

 l, m,n respectively.

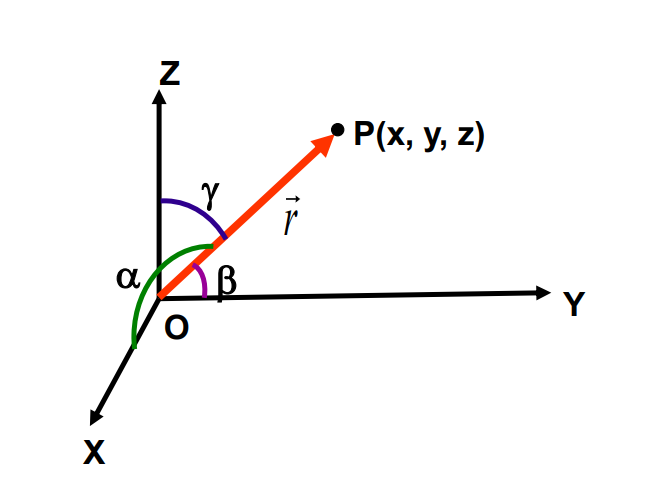
Direction Ratios

OP = |r|. Then cos β = OA/OP = y/|r| = y = m|r|.

Similarly, x = l|r| and z = n|r|.

The numbers l|r|, m|r|, n|r|, proportional to the direction cosines are called direction ratios of vector r.

Types of Vectors

In the chapter Vector Algebra, There are various types of vectors mentioned. These include-

Zero or Null Vector

A vector whose initial and terminal points are coincident is called the zero or null vector. Thus, the magnitude of the null vector is zero but its direction is not definite, it may be regarded in any direction.

Coinitial Vectors

Vectors having the same initial point are called coinitial vectors.

Collinear Vectors 

Vectors having the same or parallel supports, irrespective of their magnitude and direction, are called collinear vectors.

Unit Vector

A vector whose magnitude is unity is called a unit vector. The unit vector in the direction of a is denoted by a    

Thus |a| = 1 

Equal Vectors

Vectors having the same magnitude and direction, regardless of the positions of their initial points, are said to be equal.

Negative of a Vector

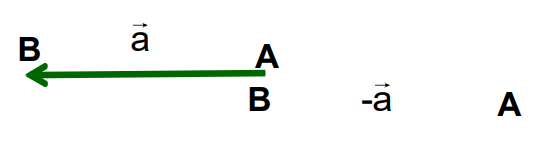
A vector that has the same magnitude as the given vector but the direction is opposite to that is called the negative of the given vector. 

The negative of AB = a is written as BA = – a 

Let’s now go further in the chapter on vector algebra and understand the addition of vectors.

Addition of Vectors

In the chapter – Vector Algebra, There are various key points mentioned about the addition of vectors. These include-

Key Points:

•  When vectors are in the same direction:

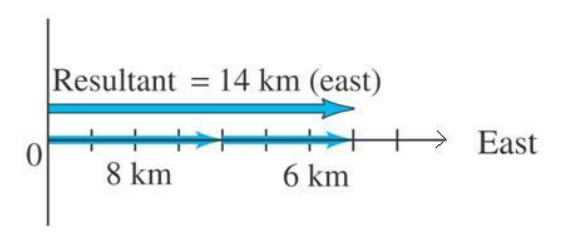
• When vectors are in opposite directions:

Triangle Law of Vector Addition

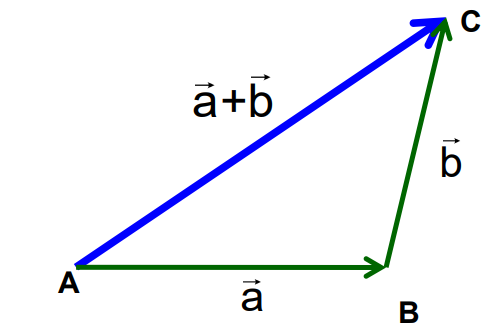
If a particle moves from A to B then B to C, its net displacement is given by                           

                                   AC = AB+ BC

This is the triangle law of vector addition.

We have, 

     AC = AB+ BC

⇒ AB + BC – AC =  0

⇒ AB + BC +CA = 0

This means sides of the triangles taken in order, lead to zero resultant. 

Parallelogram Law of Vector Addition 

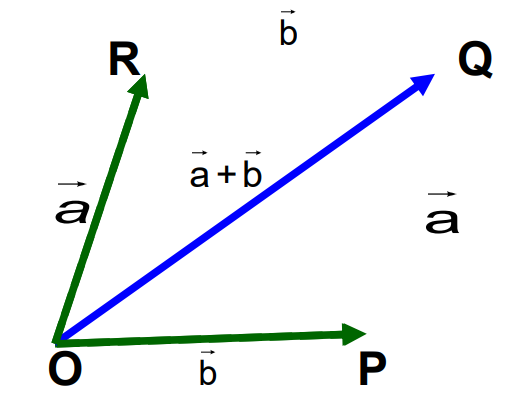
Thus, the parallelogram law of vector addition states: 

According to Vector Algebra, If two vectors a and b are represented in magnitude and direction by two adjacent sides of a parallelogram, then their sum a + b is represented by the diagonal of the parallelogram which is coinitial with the given vectors.

Properties of Vector Addition

In the chapter Vector Algebra, There are various properties of vector addition mentioned. These include-

1. Vector addition is commutative:

If a and b are any two vectors, then a + b  = b  + a.

2. Vector addition is associative: 

If a, b, c are any two vectors, then a +( b  + c ) =  (a + b) + c.

3. Additive identity for vector addition: 

Zero vector 0 is the additive identity for vector addition i.e., for any vector a,  a + 0 = a = 0 +  a

4. Additive inverse of a vector :

For every vector a, there exists a vector – a 

            such that a + (-a ) = 0

           Here -a  is called the additive inverse of a.

           Proof: Let OA = a, then -a  = – OA = AO.

          Therefore, a  + (-a ) = OA +  AO =  OO = 0.

Multiplication of a Vector by a Scalar

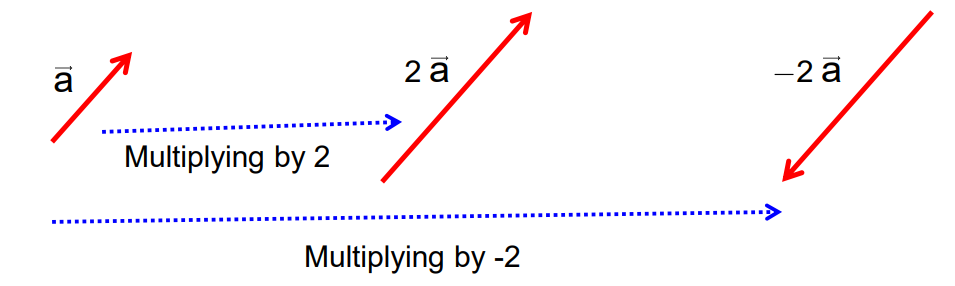
Let m be a scalar and a  be a vector, then ma is defined as a vector having the same support as that of a such that its magnitude is |m| times the magnitude of a and its direction is the same as or opposite to the direction of an according as m is positive or negative.

Components of a Vector in Two Dimensions  

In the chapter Vector Algebra, There is also the mention of the components of a vector in two dimensions. Let P(x, y) be a point in the XOY plane, and let i and j be unit vectors along OX and OY respectively. Then, OP  = xi  + yj and |OP|= √x² + y²

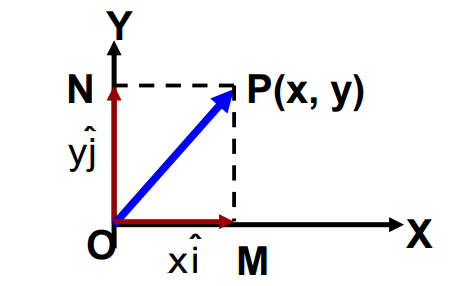
The vectors xi and yj are known as the component vectors of  OP along x and y-axes respectively.

Components of a Vector in Three Dimensions

In the chapter Vector Algebra, There is also the mention of the components of a vector in three dimensions. If a point P in space has coordinates (x, y, z), then its position vector r is xi, yj, and zk, and | r | = √ x² + y² + z²

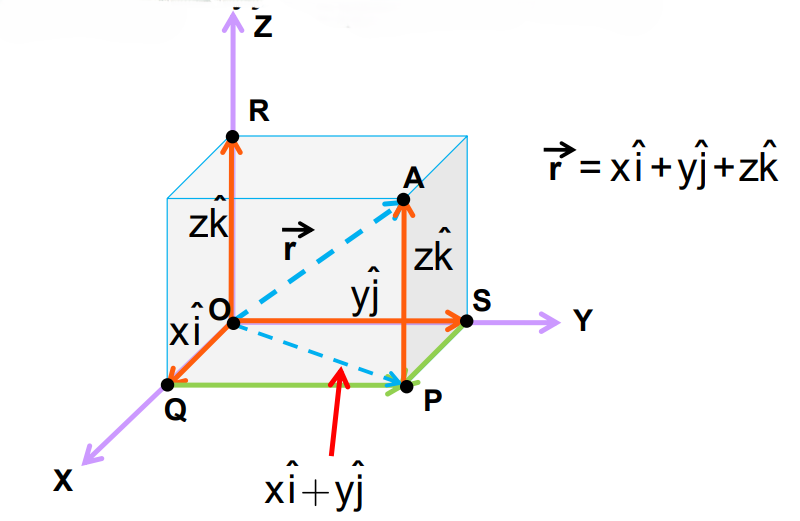
The vectors xi, yj, and zk are known as the component vectors of r along the x, y, and z-axes respectively.

Vector Joining Two Points  

Let P(x₁, y₁, z₁) and Q (x₂, y₂, z₂) two points and O be the origin.

PQ = OQ – OP

⇒ PQ = (x₂i, y₂j, z₂k) – (x₁i, y₁j, z₁k)

⇒ PQ = (x₂ – x₁) i + (y₂ – y₁) j + (z₂ – z₁) k

⇒ |PQ| = √(x₂ – x₁)² + (y₂ – y₁)² + (z₂ – z₁)²

Section Formula 

• Internal Division: Let A and B be two points with position vectors a and respectively, and let C be a point dividing AB internally in the ratio m: n. Then, the position vector of C is given by

                            c  = m b + n a / (m + n)

• External division: Let A and B be two points with position vectors respectively, and let C be a point dividing AB externally in the ratio m: n. Then, the position vector of C is given by

                          c  = m b – n a / (m + n)

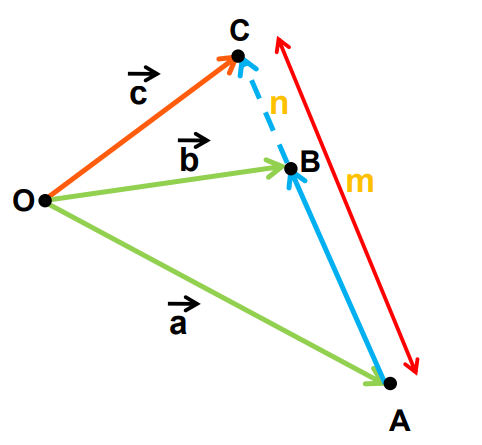
Scalar (or Dot) Product of Two Vectors 

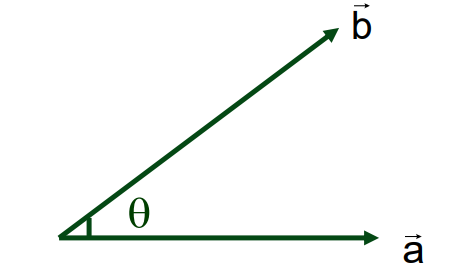
Let a be b two nonzero vectors inclined at an angle θ (0 ≤ θ ≤ π). Then, the scalar product is denoted by and defined by a . b and defined as 

a . b = |a || b | cos θ

Projection of a Vector on a Line

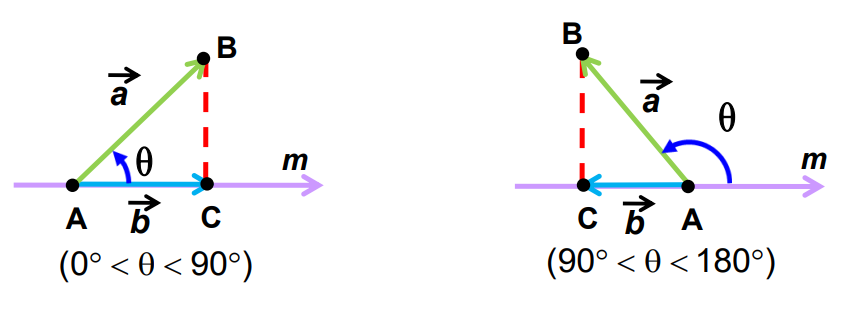
AC is called the projection of the vector AB on the directed line m.

|AC| = | AB| cos θ

Projection of a Vector on a Line

AC is called the projection of the vector AB on the directed line m.

|AC| = | AB| cos θ

Important Point:

Projection of a vector a on vector b is given by a . b  or a . b / |b |

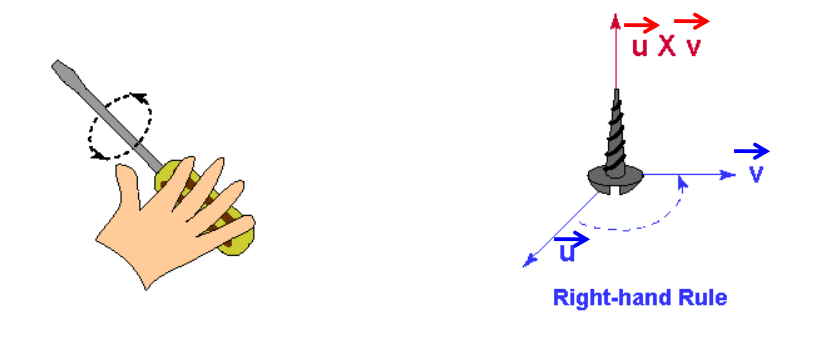
Right-Hand Rule for Vector Product 

As mentioned in the chapter on vector algebra, the cross product of two vectors is perpendicular to both. The diagrams show the right-hand rule. The order of operands is important. The result u x v   is in the same direction as a screw must point if twisting from u to v drives it into the wood. 

Or, think of the direction your thumb points when your fingers curl around from to u to v .

Vector (or Cross) Product of Two Vectors 

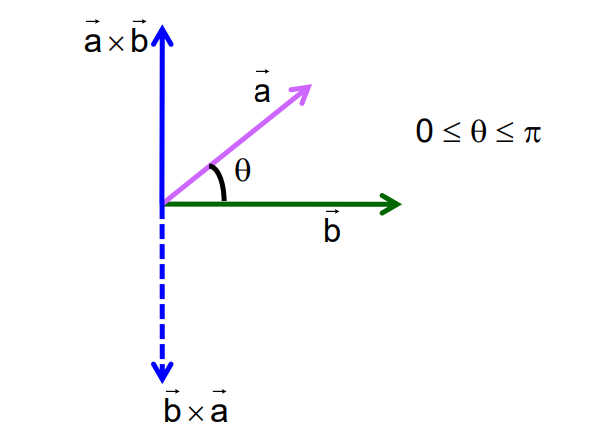
Let a and b are two nonzero vectors then their vector product is defined as a x b =  |a||b|sin θ n, where θ is the angle between a and b, 0 ≤ θ ≤ π and n is a unit vector perpendicular to both a and b such that a, b, n form a right-handed system rotated from a to b  moves in the direction of n. 

Vector Product in Terms of Components 

Let a = (a₁i, a₂j, a₃k) and b =  (b₁i, b₂j, b₃k). Then-

a x b  = 

	i	j	k
	a₁	a₂	a₃
	b₁	b₂	b₃

For example,  a = 3i + k and b =  i + j + k gives

a x b  = 

	i	j	k
	3	0	1
	1	1	1

             = – i – 2 j + 3 k

Areas in Terms of Vector Product

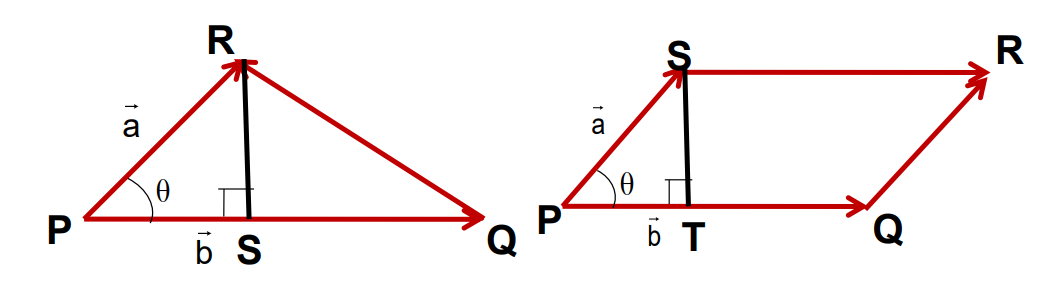
Area of triangle PQR = 1/2 |PQ||RS| = 1/2  |b|. |a|  sin θ = 1/2 |a  x  b|

Area of parallelogram PQRS =  |PQ||RS| =  |b|. |a|  sin θ = 1/2 |a  x  b|

Areas in Terms of Vector Product

If a  and b represents the adjacent sides of a triangle then its area is 1/2 |a x  b|

If a  and b represent the adjacent sides of a parallelogram then its area is |a x  b|

Let’s Conclude

In conclusion, Vector Algebra is a vital chapter in CBSE Class 12 Mathematics, as it builds a strong foundation for understanding spatial relationships and their practical applications in various fields such as physics, engineering, and computer graphics. Through the concepts of vectors, operations like vector addition, scalar multiplication, and the computation of dot and cross products, you gain a comprehensive understanding of how to represent and manipulate vectors in both two and three dimensions. Mastering Vector Algebra will not only prepare you for exams but also enhance your problem-solving skills for advanced topics. With iPrep’s engaging resources, you’re set to conquer every aspect of Vector Algebra with ease. Happy learning!