Current Electricity – Complete Guide For Class 12 Physics Chapter 3
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Current Electricity in Physics for Class 12th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
The chapter Current Electricity explores the fundamental principles governing the flow of electric charge in conductors. It delves into key concepts such as electric current, resistance, and Ohm’s Law, which describes the relationship between voltage, current, and resistance in a conductor. The chapter further explains the drift velocity of electrons, resistivity, and the factors affecting resistance. It introduces Kirchhoff’s Laws, crucial for analyzing complex electrical circuits, and discusses the internal resistance and electromotive force (EMF) of cells.
Additionally, it covers practical concepts like electrical power, energy dissipation in resistors, and the Wheatstone Bridge for measuring unknown resistances. The temperature dependence of resistivity and the limitations of Ohm’s Law are also examined, providing students with a comprehensive understanding of the behavior of electric currents in various materials and circuits. This chapter lays the groundwork for more advanced studies in electromagnetism and electronics.
Current Electricity
Current electricity is one of the essential building blocks of our modern technological world. From the moment we turn on a light switch to use various gadgets, the flow of electric current underpins numerous devices that make life convenient.
This chapter focuses on the fundamentals of current electricity, covering topics such as electric current, Ohm’s Law, resistance, and various electrical phenomena.
Current electricity deals with the movement of electric charges, which constitutes an electric current. Unlike the static charges discussed in earlier chapters, current electricity involves dynamic charges.
The chapter begins by introducing scenarios where electric currents naturally occur, such as lightning, and moves on to more controlled and steady forms of current used in devices like flashlights and clocks.
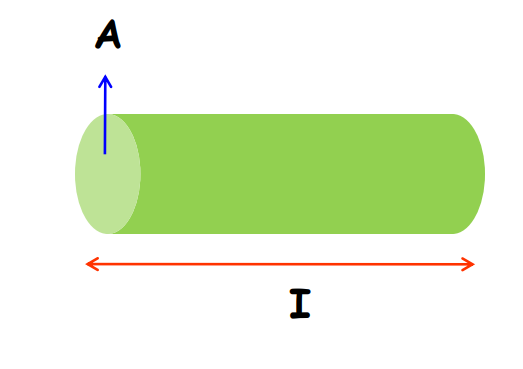
Key Concept: Electric current refers to the flow of charges across a given cross-sectional area in a conductor. The SI unit of current is the ampere (A).
Electric Current
As per the chapter “Current Electricity” from class 12 physics, Electric current is defined by the amount of charge flowing through a conductor’s cross-section over time. The formula is:
I (Electric Current) = q (Total Charge Flowing)/t (Time Taken)
Where:
- I is the current in amperes,
- q is the charge in coulombs,
- t is the time in seconds.
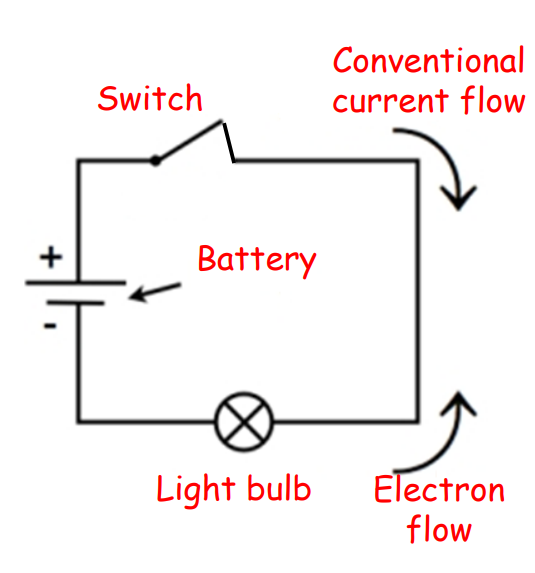
For conductors like metals, current flows primarily due to the movement of electrons, and the current direction is taken as opposite to the direction of electron flow.
Electric Currents in Conductors
Conductors are materials that allow the free flow of electric charges due to their loosely bound outer electrons. As mentioned in the chapter on current electricity from class 12 Physics, Metals are typical examples where electrons can move freely under the influence of an electric field, contributing to a steady current. The chapter explains that in the absence of an electric field, electrons exhibit random motion with no net current. However, when a field is applied, the electrons experience a force that results in a net movement, thereby producing a current.
Mobility
It is defined as the magnitude of the drift velocity per unit electric field and is denoted byi.e., = |vd|/ E
or = e /m
Where is the average collision time?
Its SI unit is m²/Vs.
Current Density
Current density J (current per unit area) is given by: J = nevd
or J = ne² x E/m
In vector form, J = ne² x E/m
Ohm’s Law
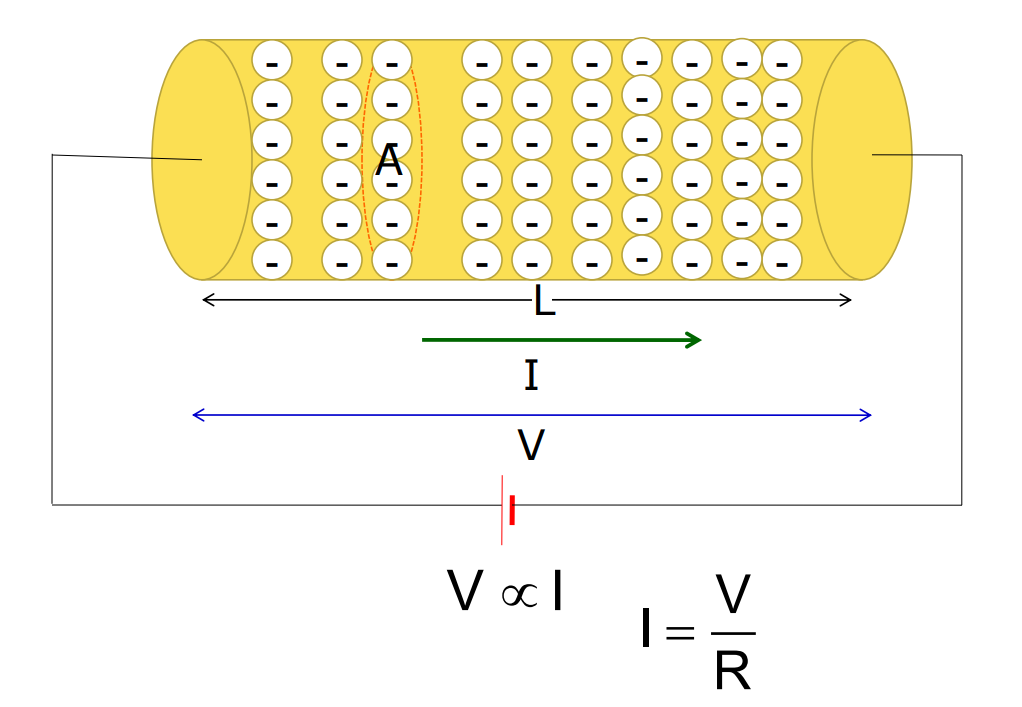
As discussed in the chapter on current electricity, one of the fundamental laws of electricity is Ohm’s Law, which states the relationship between voltage (V), current (I), and resistance (R):
V = IR
Where:
- V is the voltage across the conductor,
- I is the current flowing through the conductor,
- R is the resistance of the conductor.
Let’s now go further in the chapter “Current Electricity” and understand the concept of drift velocity.
Drift Velocity
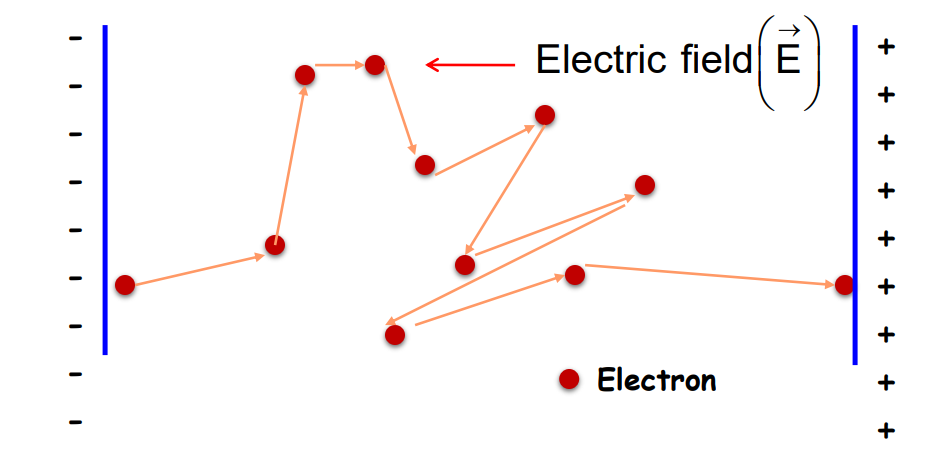
The average velocity with which electrons drift towards the positive end of the conductor is called drift velocity.
The drift velocity is given by:
vd = – eT/m x E
Where,
- m = mass of the electron
- e = electronic charge
- T = relaxation time
- E = electric field applied
Drift of Electrons and Resistivity
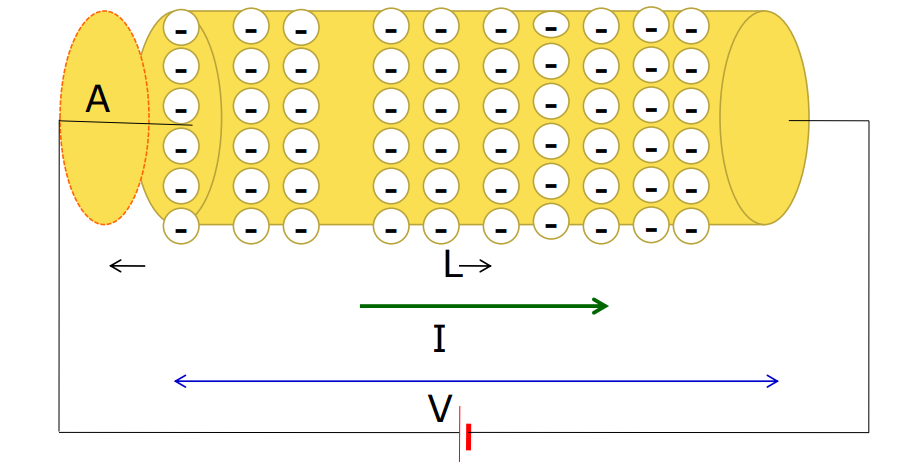
The concept of drift velocity explains how electrons move under an electric field. Drift velocity is the average velocity of electrons due to the electric field and is much smaller compared to the random thermal velocities of electrons. Despite this, drift velocity is responsible for the flow of current.
The chapter introduces the formula for current in terms of drift velocity: I = nAevd
Where:
- I is the current,
- n is the number density of electrons,
- A is the cross-sectional area of the conductor,
- e is the charge of an electron,
- vd is the drift velocity.
Limitations of Ohm’s Law
There are various limitations of Ohm’s Law mentioned in the chapter on current electricity. These involve-
Key Points:
- According to Ohm’s law, the graph between potential difference and current should always be a straight line. But, practically it is not always true.
- Ohm’s law does not apply to semiconductor devices. The relation between V & I depends on the sign of V.
- According to Ohm’s law, there should be one value of V for the same current I. But, practically the relation between V & I is not unique in some cases.
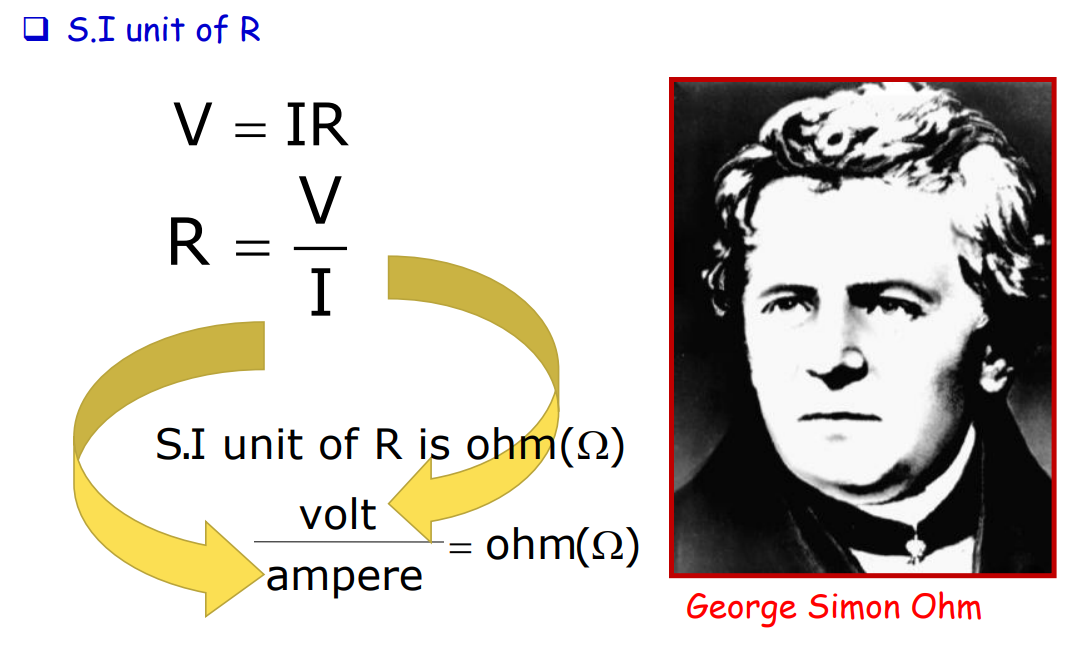
- It is the property of a conductor due to which it opposes the flow of current through it.
- Its SI unit is Ohm () and it is denoted by R.
R = Potential Difference/Current
⇒ R = V/I
1ohm = 1 volt/1 ampere
While Ohm’s Law is widely applicable, it does not hold for all materials or situations.
For instance:
- Non-ohmic materials: Devices like diodes and transistors exhibit nonlinear relationships between voltage and current.
- Temperature effects: The resistance of materials such as semiconductors can change with temperature, deviating from Ohm’s Law.
Understanding Resistance
The chapter on current electricity in class 12 physics also gives you a detailed understanding of the concept of resistance. Let’s begin-
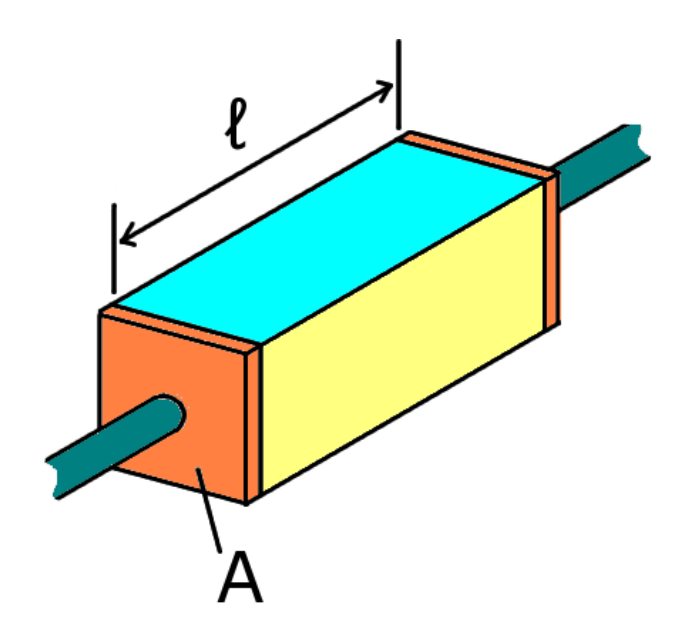
Resistance is a property of a material that opposes the flow of electric current. It depends on the material’s properties and dimensions (length and cross-sectional area). It also discusses the concept of resistivity (ρ), which is an intrinsic property of materials:
R = l/ρ
Where:
- ρ is the resistivity of the material,
- l is the length of the conductor,
- A is the cross-sectional area.
Resistivity
Let’s go further in class 12 Physics Chapter 3 – Current Electricity and understand the concept of resistivity.
The specific resistance (resistivity) of a material is equal to the resistance of a wire of unit length and unit area of a cross-section of the given material of conduct.
⇒ρ = RA /I (R = ρ x I/A)
If A = 1m², I = 1m
Therefore ρ = R
Its SI unit is ohm-metre.
R ∝ L ……….(i)
R ∝ 1/A ……….(ii)
R ∝ L/A ……….(iii)
R = ρL/A (Resistivity or specific resistance)
Unit of Resistivity
SI Unit ρ = R x A/L = ohm x m²/m = ohm-metre = m
Electric Field and Resistivity
V = IR………Ohm’s Law
V = Iρ x L/A
V/L = ρ x I/A
Conductivity () = 1 /ρ (resistivity)
SI Unit of Resistivity = 1 /ohm m = (ohm m)⁻¹
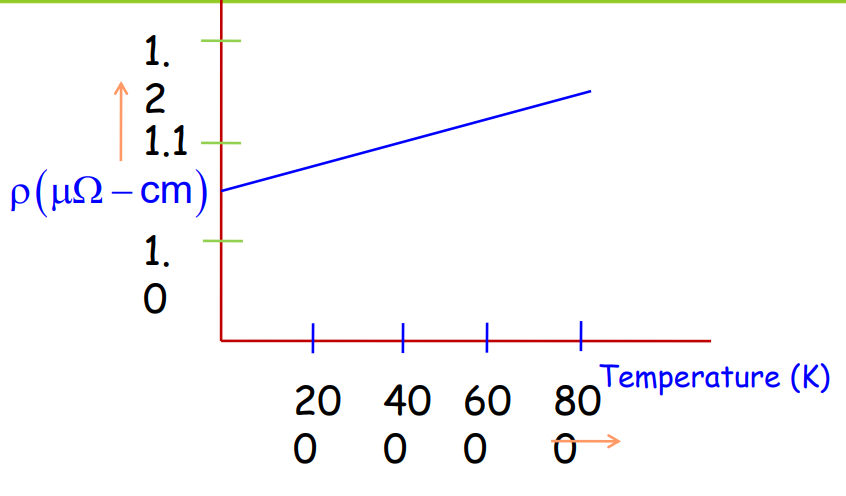
Temperature Dependence of Resistivity
Resistivity varies with temperature, and for most conductors, it increases as temperature rises. The relation is:
ρT = ρ₀ [1 + α(T−T₀)]
Where:
- α is the temperature coefficient of resistivity.
- ρT is the resistivity at a temperature T.
- ρ₀ is the resistivity at a temperature T₀.
Electrical Power
The chapter Current Electricity from class 12 physics also covers the concept of Electric Power. It is defined as the rate at which electrical energy is consumed.
Power = Work Done/TimeTaken.
It is denoted by ‘P’ and its SI unit is watt (W).
Mathematically, P = IV = I² R = V²/R
Electrical Energy
It is the energy supplied by the source of e.m.f. for maintaining the current in an electric circuit for a given time.
Electrical energy = Electric power x time
Its SI unit is joule(J), while its commercial unit is kilowatt hour(KWh).
1 KWh = 3.6 x 10⁶ J
Resistances in Series

Key Points:
- Resistors are said to be connected in series,
- if the current flowing through each resistor is the same.
- The potential difference developed across each resistor is different.
The equivalent resistance in series combination is given by:
Rₑq = R₁ + R₂ + R₃
For n number of resistors,
Rₑq = R₁ + R₂ + R₃+ ……….+Rₙ.
Resistances in Parallel
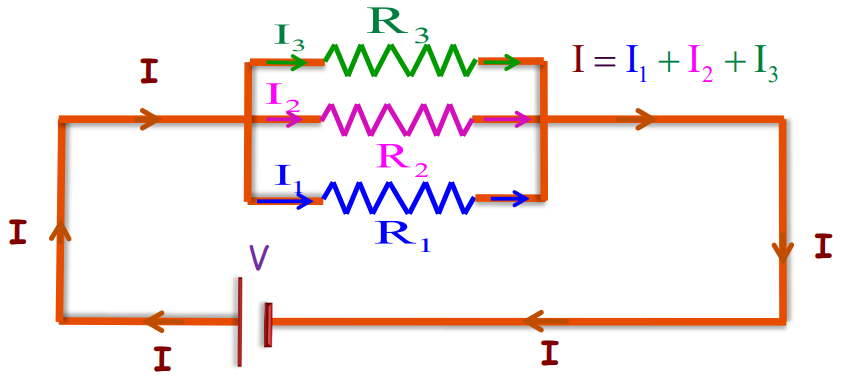
Resistors are said to be connected in parallel if the potential difference across each of them is the same and the currents flowing through them are different.
The equivalent resistance when connected in parallel is given by:
1/Rₑq = 1/R₁+1/R₂+1/R₃
For n number of resistors,
1/Rₑq = 1/R₁+1/R₂+1/R₃+…………..+ 1/Rₙ.
Cells and EMF (Electromotive Force)
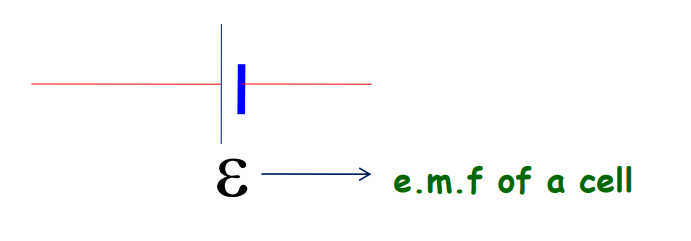
Cells are a common source of electric current. The electromotive force (EMF) of a cell is the potential difference between its terminals when no current is flowing. When a current flows, the terminal voltage of a cell is less than the EMF due to the internal resistance of the cell. The relationship between EMF (ϵ), terminal voltage (V), and internal resistance (r) is V = ϵ−Ir.
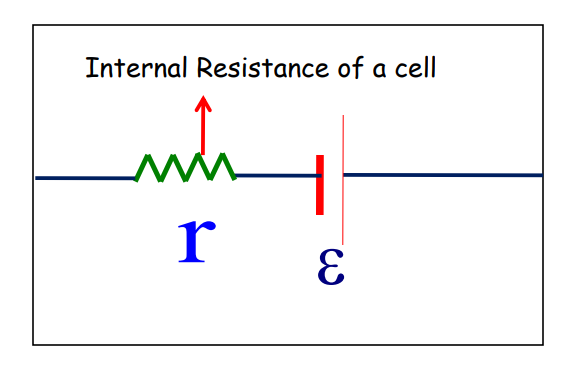
Internal Resistance
It is defined as the resistance offered by the electrolyte and electrodes of a cell when the electric current flows through it. It is denoted by r.
Series Combination
Next in class 12 Physics Chapter 3 – Current Electricity we have Series Combination. This involves-
- Two cells are said to be connected in series if one terminal of each cell is joined together and the other terminals of both cells are free.
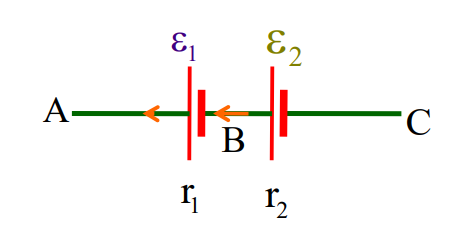
- In the series combination of cells, the equivalent e.m.f ϵₑq and equivalent internal resistance rₑq are
ϵₑq = ϵ₁ + ϵ₂
rₑq = r₁ + r₂
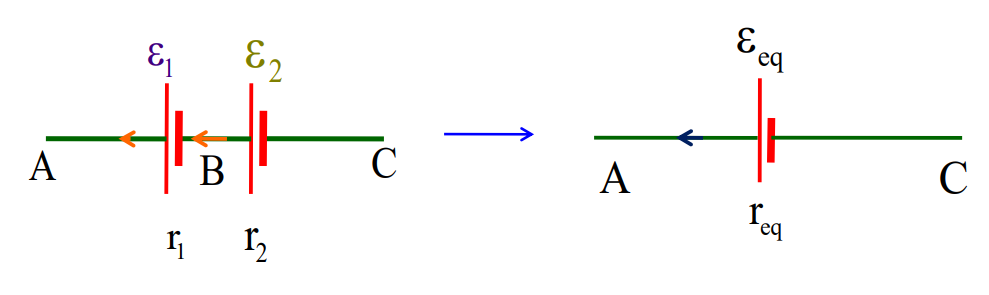
Parallel Combination
After the Series combination, the chapter Current Electricity from class 12 Physics also covers Parallel combination. This covers-
- Two cells are said to be connected in parallel if the positive terminals of each cell are connected to one point and the negative terminals of each cell to the other end.
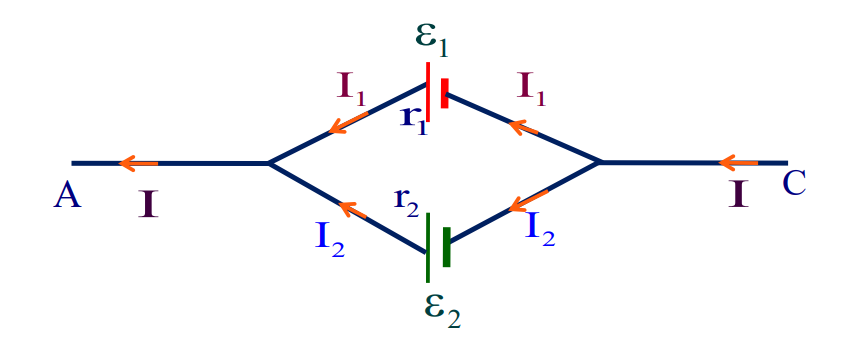
- In the parallel combination of cells, the equivalent e.m.f ϵₑq and equivalent internal resistance rₑq are
ϵₑq /rₑq = ϵ₁/r₁ + ϵ₂/r₂ and
rₑq = 1/r₁ + 1/ r₂
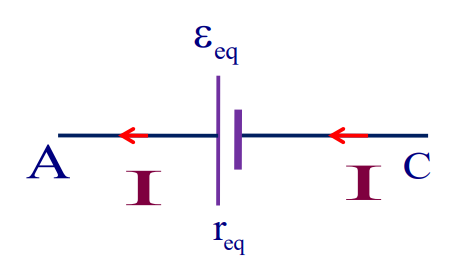
- If the parallel combination of cells is replaced by a single cell between B₁ and B₂ of e.m.f ϵₑq and internal resistance rₑq, then
rₑq = 1/r₁ + 1/ r₂
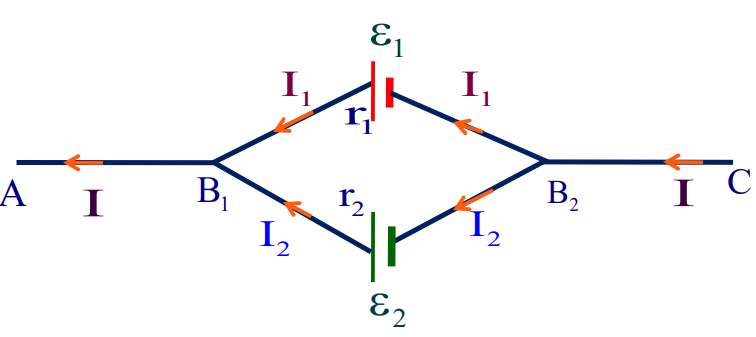
and ϵₑq /rₑq = ϵ₁/r₁ + ϵ₂/r₂
Kirchhoff’s Rules
As mentioned in the chapter “Current Electricity” from class 12 Physics, Kirchhoff’s Laws are critical for analyzing complex circuits with multiple loops and junctions:
- Junction Rule: The total current entering a junction equals the total current leaving the junction.
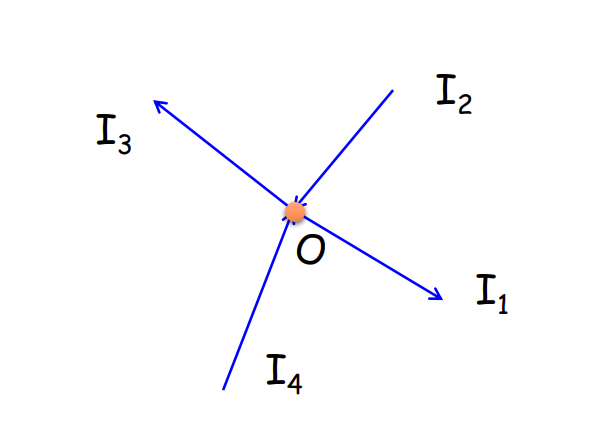
– I₁ – I₃ + I₂ + I₄ = 0
i = 1n Iₙ = 0
- Loop Rule (Kirchhoff’s Voltage Law): The sum of the potential differences around any closed loop in a circuit is zero.
These rules are fundamental for solving circuits that cannot be simplified into series or parallel combinations.
Wheatstone Bridge
The Wheatstone Bridge is a special circuit used to measure unknown resistances. When the bridge is balanced, the ratio of resistances in one pair of branches equals the ratio in the other pair.
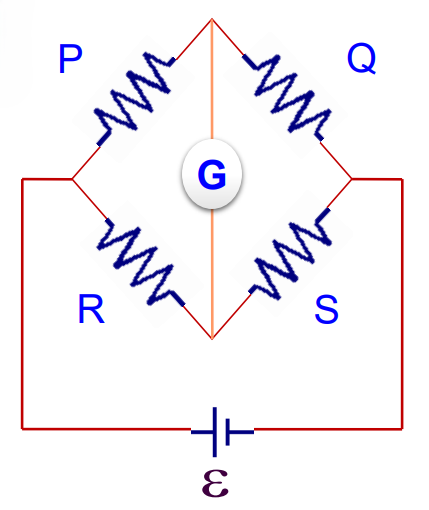
P/Q = R/S
Meter Bridge
It is a practical form of wheat stone bridge and is also known as a slide wire bridge.
Applying the Wheatstone bridge at the balanced point, the unknown resistance R is given by:
R = S x I₁/(100 – I₁)
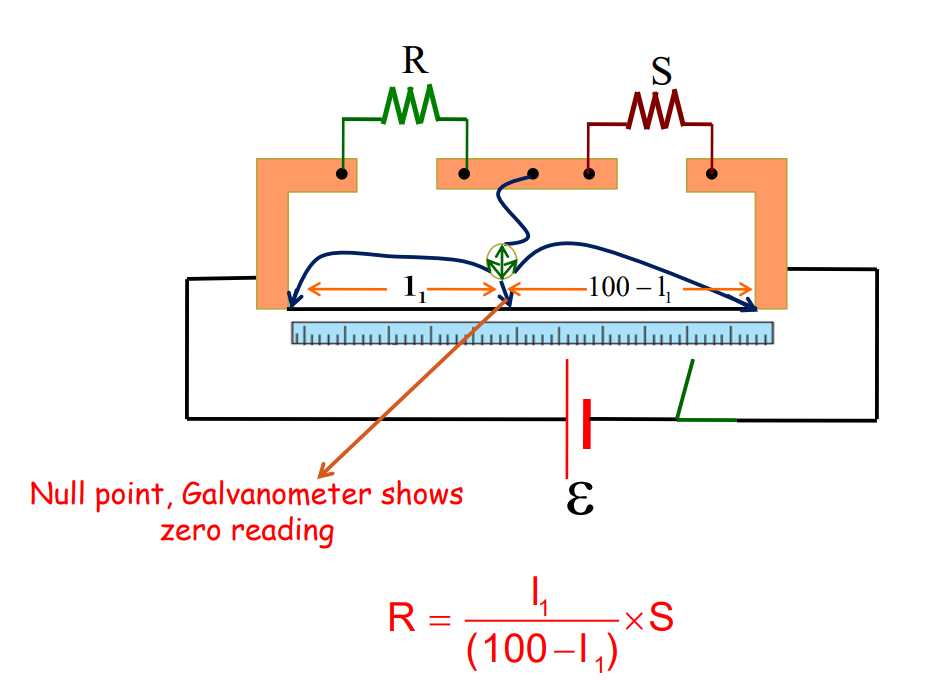
Measurement of Internal Resistance of Cell

The internal resistance of the cell is given by:
r = R (I₁/I₂ -1)
Where
- R = external resistance connected with the cel.
- I₁ = length of the wire between the balanced point N1 and A when the key is open.
- I₂ = length of the wire between the balanced point N2 and A when the key is closed.
Conclusion
In conclusion, Current Electricity, Chapter 3 of CBSE Class 12 Physics, offers a deep dive into the essential principles that govern electric current. By understanding the behavior of charges, the role of resistance, and the application of fundamental laws such as Ohm’s Law and Kirchhoff’s Laws, students can build a solid foundation in electrical circuits. This chapter also covers advanced topics like the Wheatstone Bridge, internal resistance, and the temperature dependence of resistivity, making it crucial for anyone aspiring to pursue higher studies in physics or electronics.
With iPrep, your Learning Super App, mastering the concepts of Current Electricity from Class 12 Physics becomes an engaging and effective experience. Whether through animated videos, practice questions, or notes, iPrep provides comprehensive resources to help you excel in your studies. So, dive into Current Electricity and strengthen your understanding of one of the most fundamental topics in Physics. Stay connected with iPrep to make learning fun, interactive, and rewarding!
Practice questions on Chapter 3 - Current Electricity
Get your free Chapter 3 - Current Electricity practice quiz of 20+ questions & detailed solutions
Practice Now








