Magnetism and Matter – Complete Guide For Class 12 Physics Chapter 5

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Magnetism and Matter in Physics for Class 12th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Magnetism and Matter explores the interaction between magnetic fields and materials, explaining the origin and behavior of magnetism in matter. It covers key concepts such as magnetic dipoles, magnetic field lines, and the earth’s magnetism, providing insight into the geomagnetic field and its components. The chapter explains the properties of different types of magnetic materials, including diamagnetic, paramagnetic, and ferromagnetic substances, based on their response to external magnetic fields. It delves into the concepts of magnetization, magnetic susceptibility, and permeability, which are essential in understanding how materials behave in magnetic environments. Overall, the chapter Magnetism and Matter lays the foundation for understanding the principles of magnetism and its applications in modern technology.

Magnetism and Matter: A Comprehensive Overview

Magnetic phenomena have been integral to our understanding of the universe. The study of magnetism and matter delves deep into how magnetic fields, both natural and artificial, interact with materials around us. From ancient discoveries to modern technological applications, the chapter Magnetism and Matter covers the essential concepts of magnetism.

Introduction to Magnetism

• The word magnet is derived from the name of an island in Greece called Magnesia.  
• Magnet is a substance that attracts substances like iron, nickel, cobalt, etc. and this phenomenon of attraction is known as MAGNETISM. 
•  A natural magnet has both attractive and directive properties.
• The naturally occurring iron ore that shows magnetism is magnetite (Fe₃O₄).
• This kind of material is known as a natural magnet.

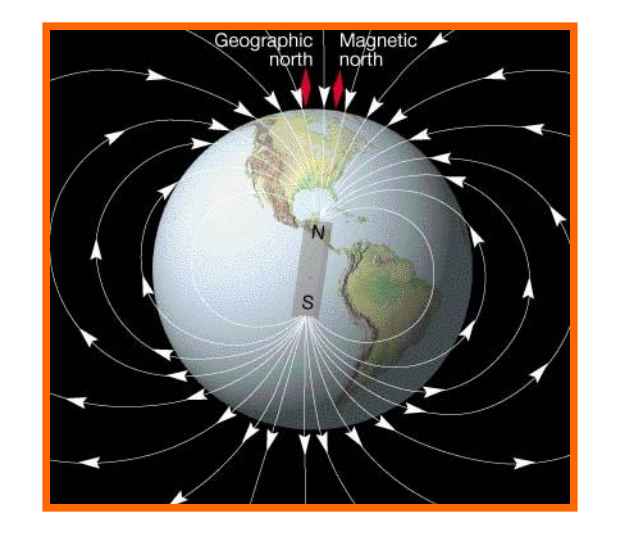
The earth itself acts as a magnet with magnetic fields originating from the geographic south and pointing towards the north. 

Bar Magnets and Magnetic Field Lines

The simplest example of a magnet as mentioned in the chapter Magnetism and Matter from Class 12 Physics, is the bar magnet, which exhibits two poles: a north pole and a south pole.

Properties of Bar Magnets

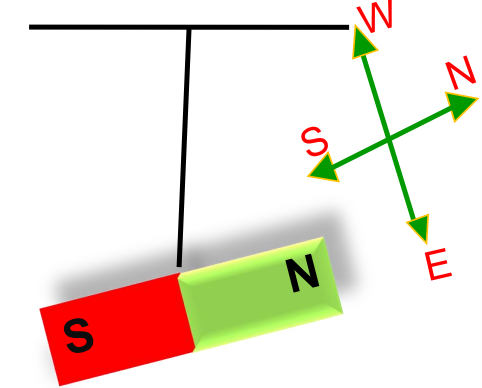
• A magnet attracts magnetic materials like iron, steel, cobalt, nickel, etc. The attraction is maximum at the two ends of the magnet called POLES.
• When a magnet is suspended freely, it comes to rest along the geographic N-S direction approximately.
• Magnetic monopoles do not exist.
• Like poles repel and unlike poles attract each other.

Magnetic Field Lines

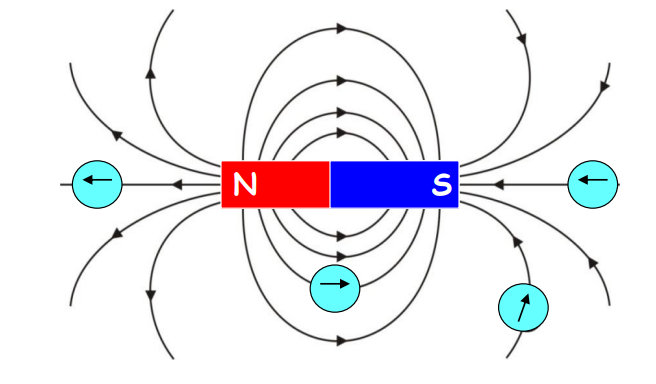
As mentioned in the chapter Magnetism and Matter,  These magnetic field lines are the lines drawn in a magnetic field, along which a hypothetical north pole would move if it were free to do so.

To Draw Magnetic Field Lines with a Compass Needle:

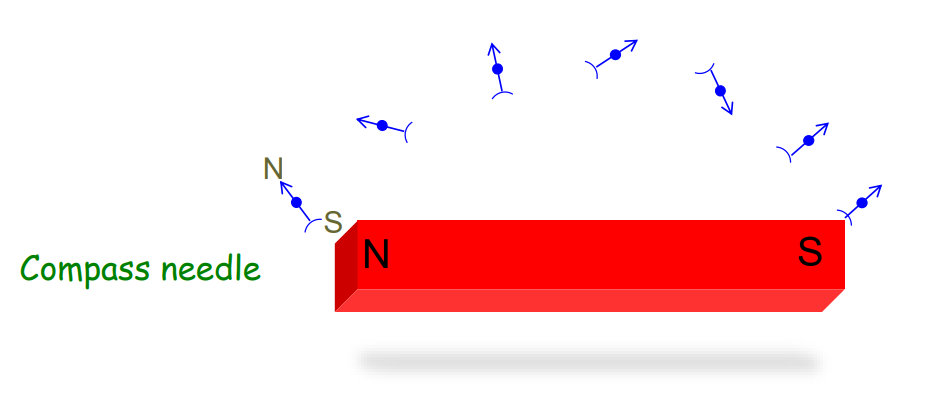
Properties of Magnetic Field Lines

The class 12 physics chapter 5 – Magnetism and Matter also discusses the concept of Properties of Magnetic Field Lines. These include- 

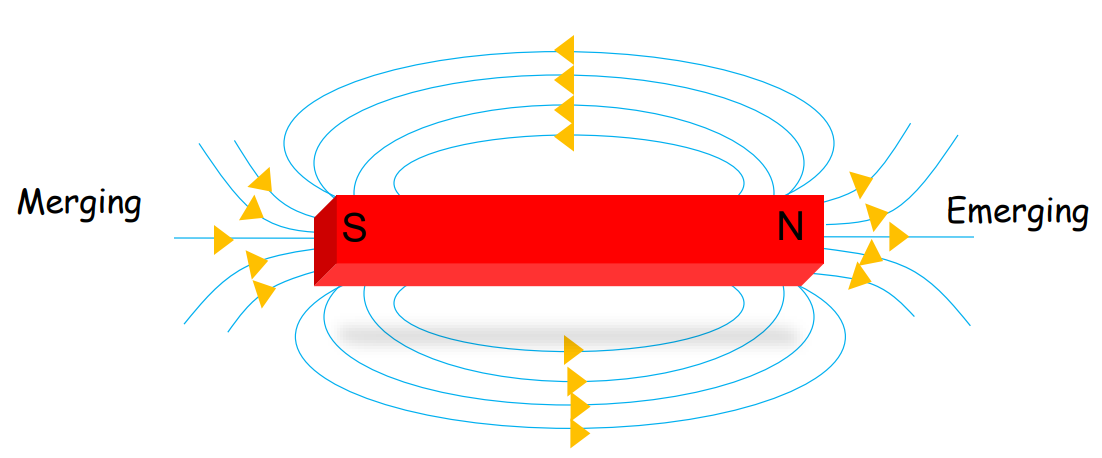
• Outside a magnet, magnetic lines of force emerge from the north pole of a magnet and merge at its south pole.  
• They form continuous closed loops.
• The magnitude of the magnetic field is large where the number of field lines crossing per unit area is large.
• The tangent drawn at any point on the field line gives the direction of the net magnetic field at that point. 
• Magnetic field lines do not intersect. If they happen to intersect at a point, the magnetic field at that point will have two directions, which is impossible.

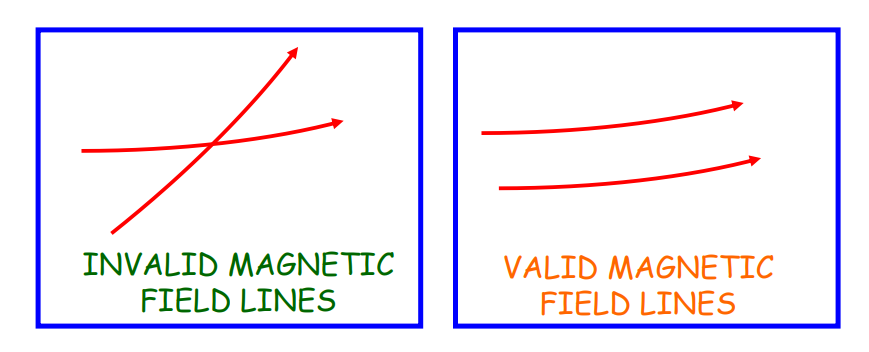
Bar Magnet as an Equivalent Solenoid

The chapter Magnetism and Matter mentions that the resemblance of magnetic field lines for a bar magnet and a solenoid suggests that a bar magnet can be thought of as a large number of circulating currents in analogy with a solenoid.

• Consider a solenoid of length ‘2L’, radius ‘a’, and number of turns per unit length ‘n’.  
• Let ‘I’ be the current flowing through it.
• Consider a small element of thickness (dx) at a distance ‘x’ from ‘O’.  
• The magnitude of the field at ‘P’ due to the circular element is:

                             dB = ₀Ia²(ndx) / 2[ (r-x)² + a²]³/₂

                               If r>>a & r>>L, then

                             dB =  ₀Ia²ndx/2r³………(i)

• Integrating eq.(1) by taking the range of ‘x’ from –L to +L, we get:

B  =   ₀Ia²n/2r³x = -Lx = +Ldx

or B =  ₀Ia²n(2L)/2r³

• The magnitude of the magnetic moment of a solenoid is given by the product of the total number of turns(n2L), current(I), and the cross-sectional area(A).

                                m = n(2L) I A………(ii)

                               or, m= n(2L) I(a²)

• The magnitude of the magnetic field of the solenoid is 

                                        B = ₀2m/4r³

• Substituting the value of ‘m’ from eq.(ii) we find that a bar magnet and a solenoid produce similar magnetic fields.  
• The magnetic moment of a bar magnet is equal to the magnetic moment of an equivalent solenoid that produces the same magnetic field.

Dipole in a Uniform Magnetic Field

Further in the chapter Magnetism and Matter, we’ll understand the topic of Dipole in a Uniform Magnetic Field. This will cover-

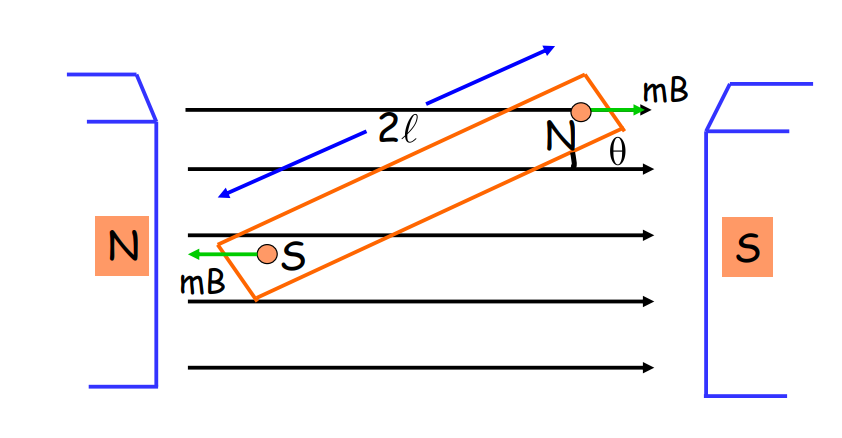
• Let a magnetic dipole of the magnetic moment ‘m’ be held, subtending an angle ‘q’ with the direction of a uniform magnetic field ‘B’.
• The torque acting on the dipole is given by: = m x B
• The magnitude of the torque acting on the dipole is given by:       

                                    = mB sin

Magnetic Potential Energy

As mentioned in the chapter magnetism and matter, magnetic potential energy due to the dipole is given by:

                                       Uₘ = d

                                        = mB sin d

                                        = – mB cos

                                       Uₘ = – m . B

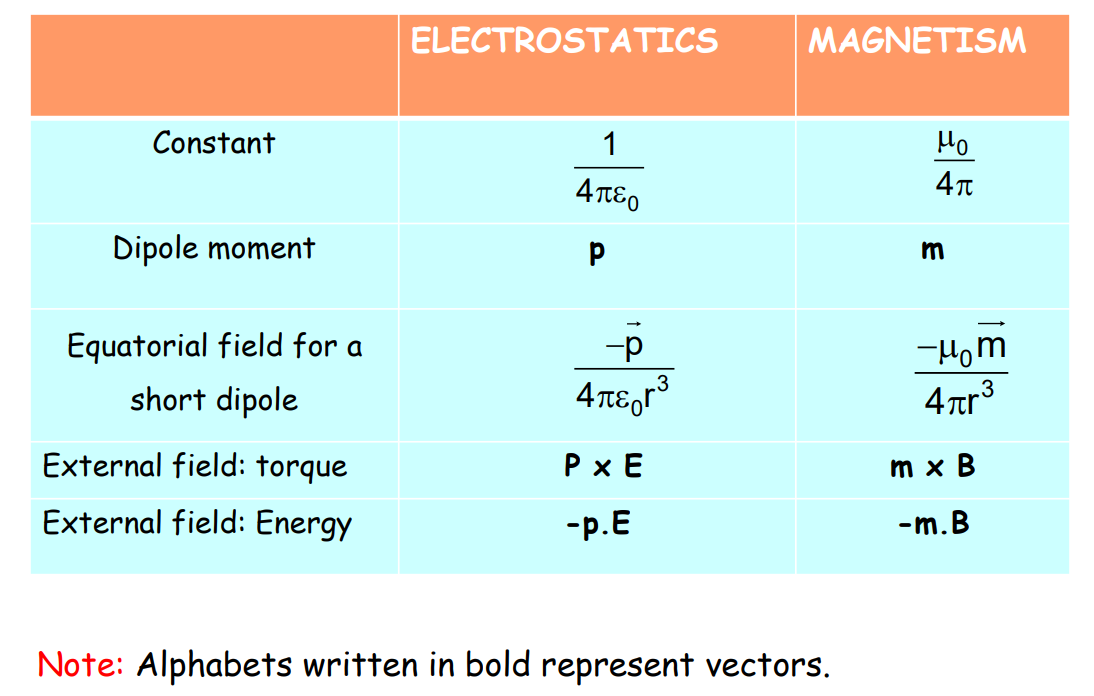
Electrostatic Analog

As discussed in the chapter magnetism and matter, the equation of magnetic field at large distances due to a bar magnet of magnetic moment ‘m’ can be obtained from that of electric field due to the electric dipole of dipole moment ‘p’ by making the following replacements:

1.  E→ B,  p→ m
2. 1/4 ₀ → ₀/4

Gauss’s Law for Magnetism

The chapter Magnetism and Matter also discusses Gauss’s law of magnetism. This covers-

• The law states that the net magnetic flux through any closed surface is zero.  
• It is the direct consequence of the nonexistence of magnetic monopoles.
• It can be expressed as:

_B  = ALLB. S = 0

Gauss’s Laws of Electrostatics and Magnetism

•  The electric flux through a closed surface is 1/₀ times the total charge ‘Q’ enclosed by the surface. 

_E  = E. S = Q/₀

• The net magnetic flux through any closed surface is zero.

              _B  = B. S = 0

• The basic difference between the two laws lies in the fact that isolated magnetic poles do not exist.  
• The simplest magnetic element is a dipole or a current loop. But, in the case of electrostatics, mono-charge exists.

Magnetization and Magnetic Intensity

Further in the chapter Magnetism and Matter, we uncover the topic of Magnetization and Magnetic Intensity. This will cover-

Magnetization

• The magnetization of a substance is defined as the net magnetic moment per unit volume.

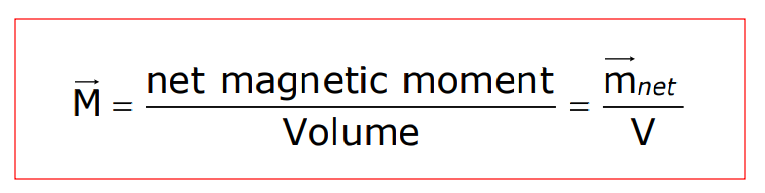
• It is a vector quantity having dimension [L⁻¹A].  
• The SI unit of magnetization is ampere per meter (A/m). 

Magnetic Intensity

• Magnetic intensity is defined by:

H = B/ ₀  – M

• It is a vector quantity.  
• The SI unit of magnetic intensity is ampere per meter (A/m). 

Magnetic Susceptibility (c)

• It measures how a magnetic material responds to an external field.
• Mathematically, it can be expressed in terms of M and H as:

                       x = M/H 

• It is a dimensionless quantity. 

Relation between B and H

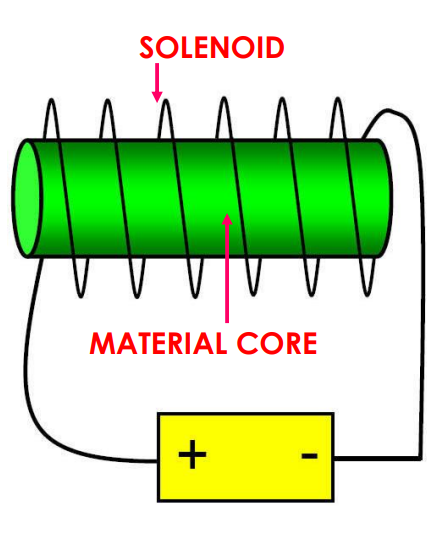
The magnetic field in the interior of the solenoid is given by:

                             B₀ = ₀nI

The magnetic field due to the magnetization of the material core Bₘ is given by: Bₘ = ₀ M  

The net field in the interior of the solenoid is the sum of the fields B₀ and Bₘ.

                               B =  B₀ + Bₘ = ₀ (H + M)

                                            = ₀ (H + H) = ₀ H (1 + )

 Therefore,  B  = _0r H

Here, _r = (1 + ) is known as the relative permeability. It is a dimensionless quantity. It is the analog of the dielectric constant in electrostatics.

Diamagnetic Substances

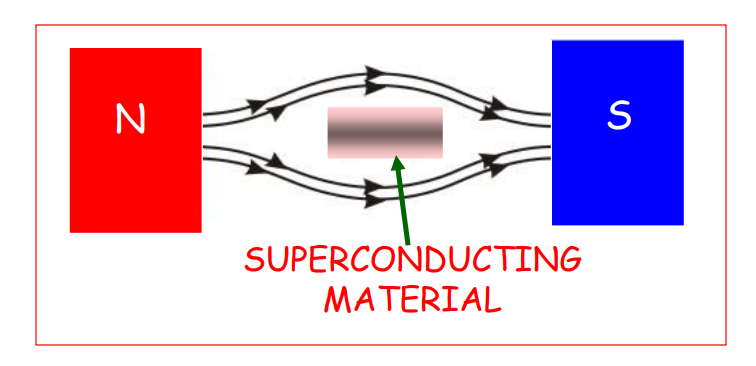
These are weakly repelled by magnets and have a small negative magnetic susceptibility. Bismuth, copper, water, superconductors, etc. are some such examples.

Molecular Explanation of Diamagnetism

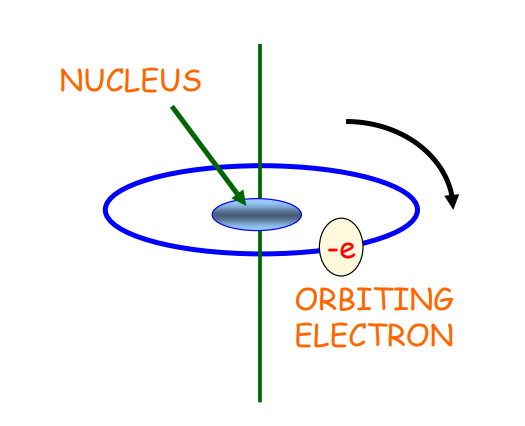
• Electrons orbiting around the nucleus act like a current-carrying loop, possessing orbital angular momentum and an orbital magnetic moment. 

• Diamagnetic substances have no unpaired electrons, resulting in zero net magnetic moment. When an external magnetic field is applied, electrons with magnetic moments aligned with the field slow down, while those opposite speed up. This causes the substance to develop a magnetic moment in the direction opposite to the applied field, leading to repulsion.

Superconductors

• These are the materials that have zero resistance at very low temperatures.  
• They possess perfect conductivity, zero permeability, and negative susceptibility and hence show perfect diamagnetism.

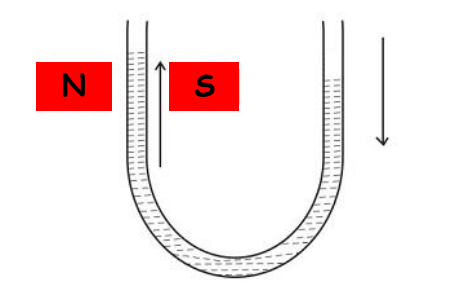
Meissner Effect 

• When placed in an external magnetic field, the field lines are expelled by the superconductors.
• This phenomenon is known as MEISSNER EFFECT.

Basic Properties of Diamagnetic Substances

• When suspended in a uniform magnetic field, they set their longest axis at a right angle to the direction of the field. 
• In such substances, the resultant magnetic moment of an atom is zero.
• When a diamagnetic liquid contained in a watch glass is placed on the closely spaced pole pieces of a magnet, it gets depressed in the middle. While, if the poles are moved apart, the liquid rises in the middle.

• Thus, in a non-uniform magnetic field, diamagnetic substances tend to move from stronger parts of the field to the weaker. 
• When placed in a magnetic field, diamagnetic substances develop a net magnetic moment in a direction opposite to that of the applied field.

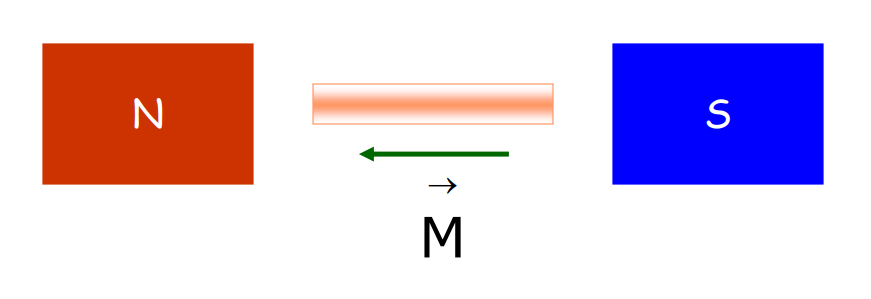
• The field lines prefer not to pass through the diamagnetic specimen, which is placed in a magnetizing field.
• The level of a diamagnetic liquid in a U tube is depressed when subjected to a magnetic field.

• Relative magnetic permeability is always less than unity.

                               Therefore,   _r< 1

• The susceptibility of diamagnetic substances is negative and doesn’t change with temperature. 

                                          Therefore, < 0

Paramagnetic Substances

As mentioned in the chapter Magnetism and Matter, These materials are weakly attracted by magnets and possess a small positive magnetic susceptibility.

Basic Properties of Paramagnetic Substances

The chapter Magnetism and Matter also discusses the Basic Properties of Paramagnetic Substances. These involve

• When suspended in a uniform magnetic field, they set their longest axis along the direction of the field.
• When paramagnetic substances are kept in a uniform magnetic field, the individual atomic dipole moment of the atoms aligns them in the direction of the magnetic field.
• When a paramagnetic liquid contained in a watch glass is placed on the closely spaced pole pieces of a magnet, the liquid rises in the middle. On the other hand, if the poles are moved apart, it gets depressed in the middle.

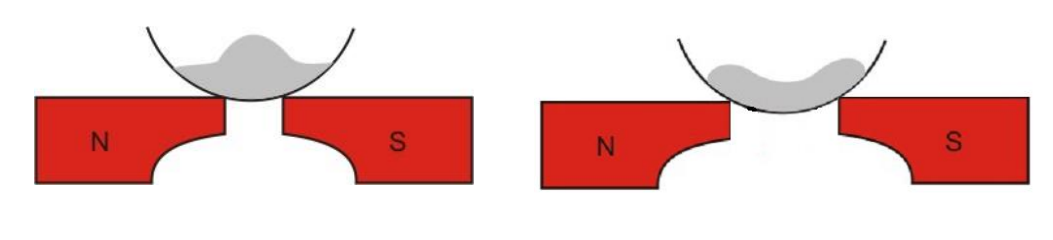
• Thus, in a non-uniform magnetic field, paramagnetic substances move from the weaker parts of the field to the stronger parts.
• The field lines pass through the paramagnetic specimen when it is kept in a magnetic field.
• The level of a paramagnetic liquid in a U tube rises when subjected to a magnetic field.

• Relative magnetic permeability is always more than unity.

                                 Therefore,   _r >1

• The susceptibility of paramagnetic substances is positive. 

Ferromagnetic Substances

These are strongly attracted by magnets and have a large positive magnetic susceptibility. Examples include iron, cobalt, and nickel. The magnetization of ferromagnetic materials is much larger than that of paramagnetic substances. 

Molecular Explanation of Diamagnetism

• In ferromagnetic materials, the individual atoms possess dipole moments. These dipole moments interact with one another and spontaneously align themselves in a common direction in a domain. Each domain has a net magnetism.

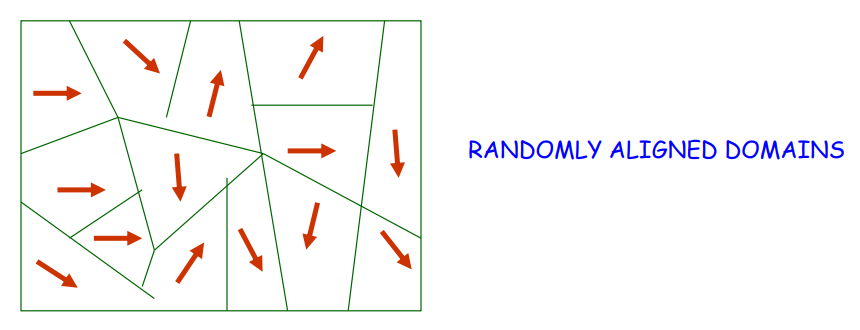
• When the external magnetic field is applied, the domains orient themselves in the direction of the magnetic field (B)and grow in size.  
• Thus, a ferromagnetic material gets strongly magnetized in the direction of the applied field.

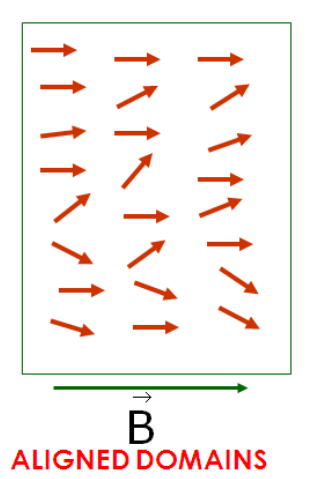
Hard Ferromagnets

• These are the ferromagnetic materials in which magnetization persists even after the external field is removed.  Alnico, an alloy of iron, aluminum, nickel, cobalt, and copper is one of the examples.  
• They are used for making permanent magnets.

Soft Ferromagnets

• These are the magnetic materials in which magnetization disappears after the external field is removed.  
• They are often used for making electromagnets.
• Soft iron is an example. 

Basic Properties of Ferromagnetic Substances

The chapter Magnetism and Matter also discusses the Basic Properties of Ferromagnetic Substances. These cover-

• They are strongly attracted by the magnets. When placed in a non-uniform magnetic field, a ferromagnetic substance shows a strong tendency to move from the weaker to the stronger part of the field.  
• Each atom of such substance is a magnetic dipole having a resultant magnetic moment.
• A specimen of ferromagnetic substance gets strongly magnetized even when it is placed in a weak external field. Even after the removal of an external field, it does not lose magnetism completely.  
• At a certain high temperature, a ferromagnetic substance is converted into a paramagnetic substance.

Permanent Magnets

Permanent magnets are those substances that retain their ferromagnetic properties for a long time at room temperature. 

Conclusion

In conclusion, CBSE Class 12th Physics, Chapter 5 – Magnetism and Matter offers a deep dive into the fascinating world of magnetic fields and their interactions with materials. It explains the fundamental principles of magnetism, from the behavior of magnetic dipoles to the intricate properties of different magnetic materials. The chapter provides essential insights into how natural and artificial magnetic fields operate, setting the stage for understanding real-world applications in modern technology.

As you work through this chapter on Magnetism and Matter in Class 12th Physics, the detailed explanations of magnetic susceptibility, permeability, and the earth’s magnetism will help you build a solid foundation. Whether you’re preparing for an exam or reinforcing concepts, iPrep’s resources, including animated videos, practice questions, and notes, are designed to make your learning experience comprehensive and engaging.

By the end of Chapter 5, Magnetism and Matter, you will have a clear understanding of how magnetic fields shape the world around us, both in nature and in technology. With iPrep’s integrated learning tools, mastering Magnetism and Matter in Class 12th Physics has never been easier. Happy learning!