Moving Charges and Magnetism – Complete Guide For Class 12 Physics Chapter 4
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Moving Charges and Magnetism in Physics for Class 12th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
The moving Charges and Magnetism chapter explores the fundamental relationship between electric currents and magnetic fields. It introduces the concept of the magnetic field created by a moving charge or current-carrying conductor, described by Biot-Savart’s law. The chapter Moving Charges and Magnetism, delves into the force experienced by a charged particle moving in a magnetic field (Lorentz force), the motion of charges in uniform magnetic fields, and the interaction between two parallel current-carrying conductors. Additionally, it covers Ampere’s circuital law and its applications in calculating the magnetic field due to different current configurations. The chapter also highlights the principle of torque on a current-carrying loop in a magnetic field, laying the foundation for understanding devices like electric motors and galvanometers.
Moving Charges and Magnetism
In this chapter, Moving Charges and Magnetism, we explore the fascinating relationship between electricity and magnetism, discovering how moving charges produce magnetic fields and how these magnetic fields interact with electric currents. This connection forms the basis for a wide range of physical phenomena and technological advancements, including motors, generators, and electromagnetic waves.
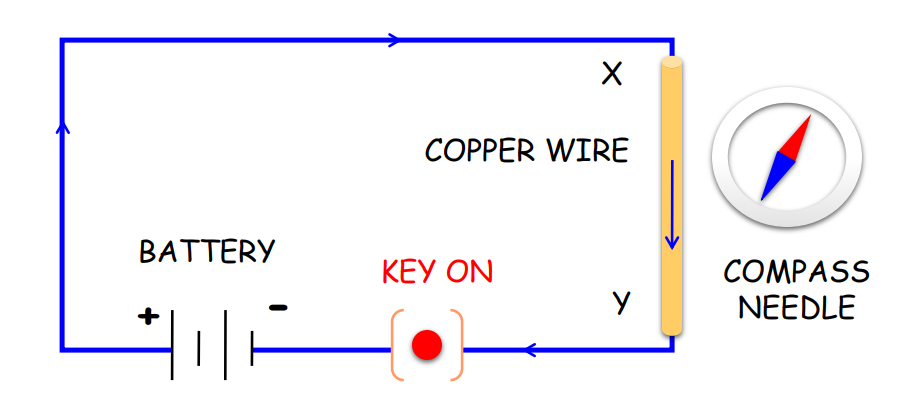
- When a magnetic needle is kept near a current-carrying conductor, it shows deflection.
- The alignment of the compass needle is tangential to an imaginary circle.
- To ignore the Earth’s magnetic field, the magnitude of current flow should be large and the needle should be kept close to the wire.
- On reversing the direction of the current, the orientation of the compass needle also gets reversed.
- The deflection increases by increasing the strength of the current flowing through a wire or by bringing the compass needle close to the wire.
- It is concluded that current or moving charges produce a magnetic field in the surrounding space.
Let’s now go further in the chapter Moving Charges And Magnetism from class 12 physics and understand the concept of Magnetic Field and Its properties.
Magnetic Field & Its Properties
The chapter on Moving Charges and Magnetism from class 12 physics covers the magnetic field and its properties thoroughly. This involves-
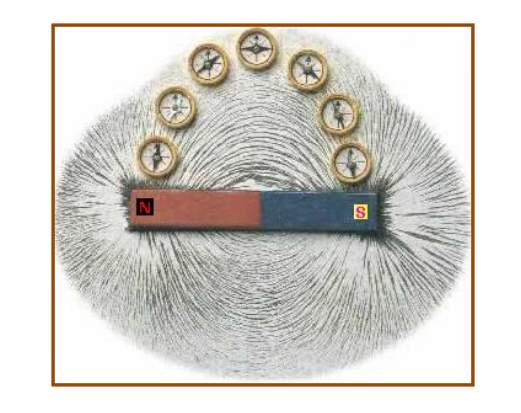
- It is the region or space around a current-carrying conductor or magnet where its magnetic effect can be felt.
- The S.I. unit of the magnetic field is the tesla (T).
- Static charge produces an electric field and moving charge produces a magnetic field in addition to the electric field.
- It is a vector quantity denoted by B.
- It has some basic properties identical to the electric field.
- It follows the principle of superposition.
- According to this principle, the magnetic field of several sources is the vector addition of the magnetic field of each source.
Lorentz Force
Another very important topic from class 12 physics chapter 4 -Moving Charges and Magnetism is the Lorentz force.
One of the key principles in this chapter is the Lorentz force. A charged particle moving through both electric and magnetic fields experiences a force, given by: F = q (E + v × B ).
This equation shows how the force on the charge depends on the charge’s velocity, the electric field E, and the magnetic field B.
In a magnetic field, we observe that:
- Lorentz force depends upon q (charge), v (velocity), and B (magnetic field).
- The force acting on a negative charge is opposite to that acting on a positive charge.
- The force due to the magnetic field, F= q(v × B)
- It includes the vector product of velocity and the magnetic field itself.
- If velocity and magnetic field are parallel or anti-parallel, the magnetic force becomes zero.
- The magnetic force acts in the direction perpendicular to velocity and magnetic field.
- The direction of magnetic force can be found by using the Right-hand screw rule.
Lorentz Magnetic Force on a Current-Carrying Conductor
Let us now understand the topic Lorentz Magnetic Force on a Current-Carrying Conductor from class 12 physics chapter 4. with the help of the image given below.
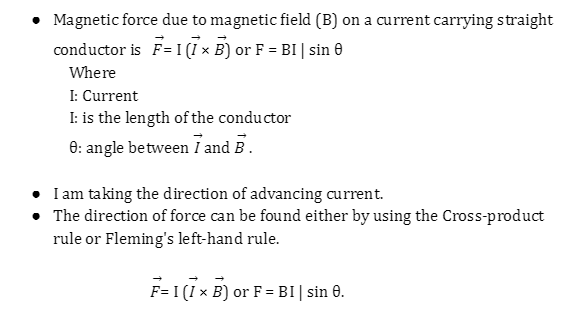
- When a charged particle moves at right angles to the field, the work done on a charged particle is zero.
- There is no change in the magnitude of velocity.
- No change occurs in the kinetic energy as well.
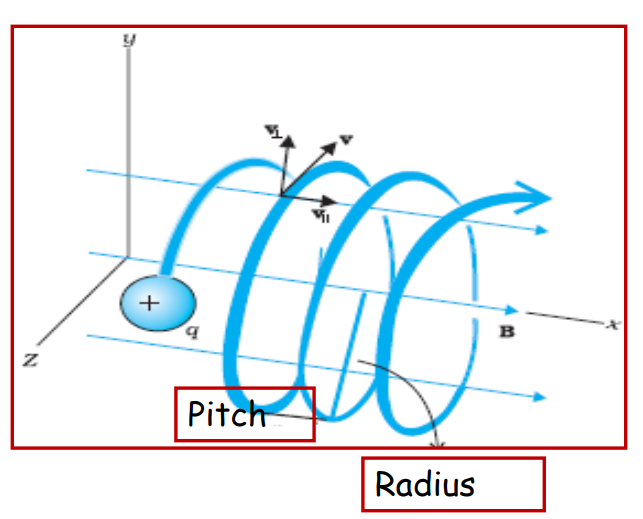
Motion of Charged Particles in Magnetic Fields
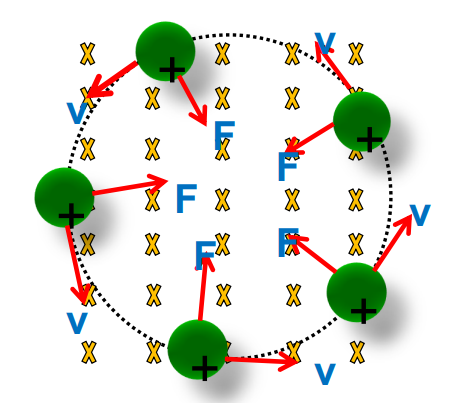
- When a charged particle moves perpendicular to the magnetic field, the force acting on the particle is perpendicular to both its velocity and the magnetic field, causing the particle to move in a circular path.
- The radius of this path depends on the velocity of the particle and the magnetic field strength. If the particle’s velocity has a component parallel to the field, it follows a helical path.
- The period of rotation of a charged particle in a circular motion is T = 2m/qB.
- The radius of the circle on which the charged particle revolves in a circular path is r = mv sin /qB.
Cyclotron: Accelerating Particles
Another highly important topic from the chapter Moving Charges and Magnetism, is Cyclotron.
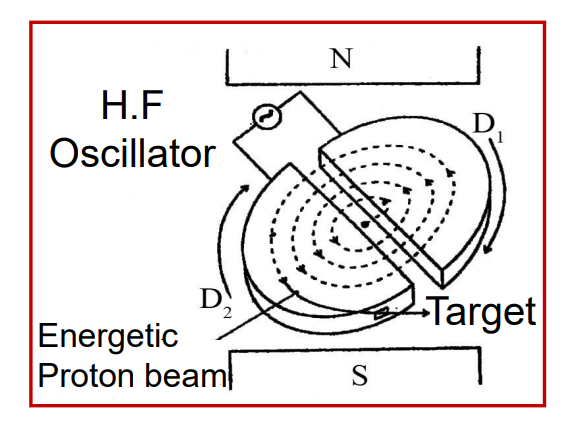
- The cyclotron is a machine used to accelerate charged particles to high speeds using both electric and magnetic fields.
- Particles move inside semicircular discs called “dees” under the influence of a magnetic field, which bends their paths into circular motion.
- An alternating electric field between the dees accelerates the particles, increasing their energy with each pass.
- The cyclotron frequency is vc = qB/2m.
- The kinetic energy of the charged particle is K.E = q²B²r²/2m
Biot-Savart Law
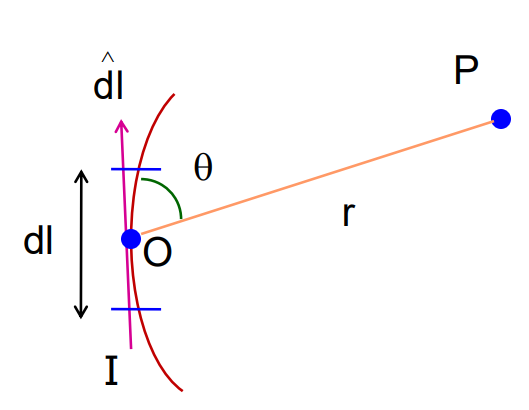
- This gives the relationship between magnetic field intensity at a point and the current which produces the magnetic field at that point
- The magnetic induction produced at a distance (r) from the current element is |dB| = 0 /4 x Idl sin /r²
Where:
- I am the current passing through a small element dl of a conductor.
- B is the magnetic field intensity at a distance r from the current carrying element.
- This law is crucial for calculating the magnetic field produced by various current configurations.
Magnetic Field on the Axis of a Circular Current Loop

- The magnetic field due to the circular coil along its axis is
B = 0 IR²/2(x² + R²)³/₂
or, B = 0 IR²x i/2(x² + R²)³/₂
- Putting x=0, we will get the field at the center as
B = 0 I/2R
Ampere’s Circuital Law
- Ampere’s Circuital Law provides a way to calculate the magnetic field in systems with high symmetry.
- It states that the line integral of the magnetic field around a closed path is proportional to the total current threading through the path:
∮ B⋅dI = 0 I
This law is particularly useful for calculating the magnetic fields inside solenoids and toroids.
Solenoid
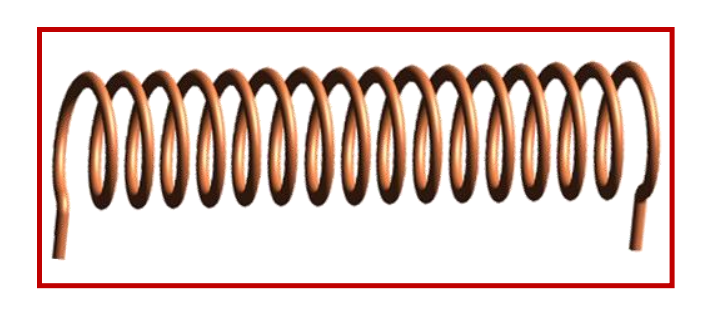
- It is a long cylindrical coil wound over a hollow insulating cylinder.
- The field outside the solenoid approaches zero.
- The field inside the solenoid is parallel to the axis.
- The magnetic field inside the solenoid is B = 0 nI
- The magnetic field induction near the end of the solenoid is B = 0 nI/2
Toroid

- It is a hollow circular ring on which a large number of insulating turns of a metallic wire are closely wound.
- It is an endless solenoid in the form of a ring.
- The field inside the toroid is given by: B = 0 NI/2r.
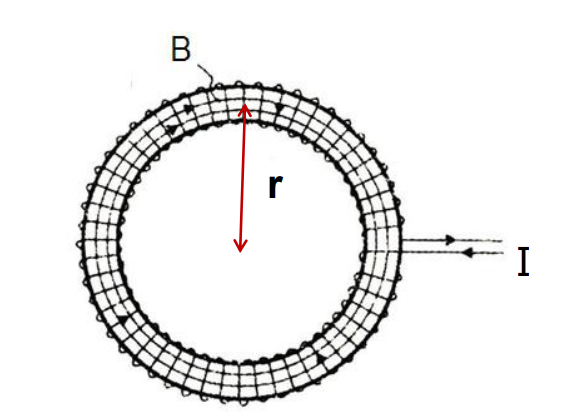
Where:
- N is the number of turns of the toroidal coil.
- r is the average radius of the toroid
The force between Two Parallel Currents
As mentioned in the chapter Moving Charges and Magnetism, two long parallel conductors of length ‘l’ separated by a distance ‘r’ experience a force due to the currents flowing through them.
- Same Direction of Current: When currents in both conductors flow in the same direction, they attract each other.
- Opposite Direction of Current: When currents in both conductors flow in opposite directions, they repel each other.
This interaction depends on the direction of the current and whether it results in attraction or repulsion between the conductors.
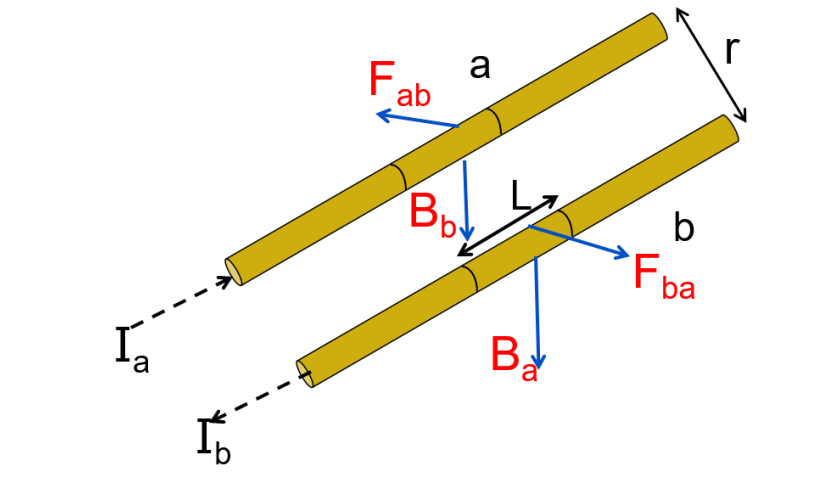
The magnitude of the force is F = 0 Iₐ Ib L/2r.
Torque on a Rectangular Current Loop in a Uniform Magnetic Field
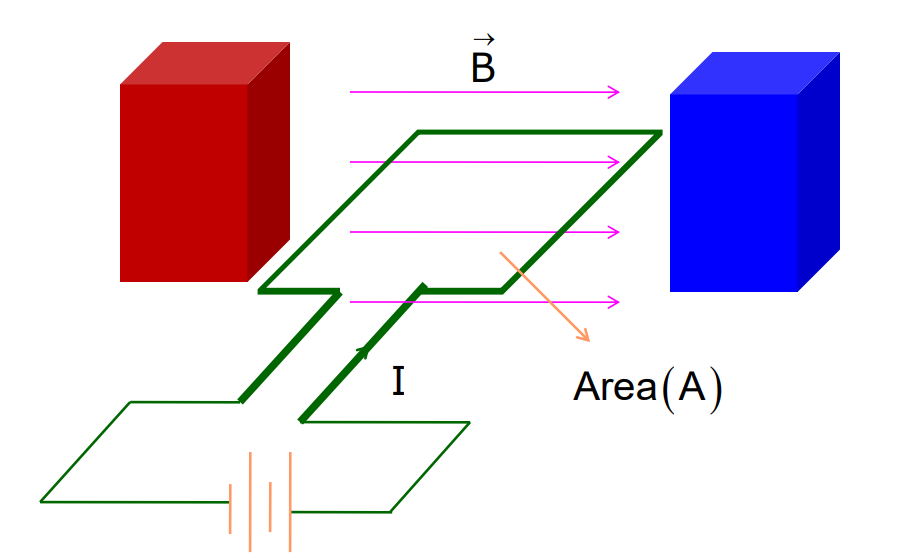
When a current-carrying loop is placed in a uniform magnetic field, it experiences a torque that tends to rotate the loop. The torque is given by:
τ = IABsinθ.
Where:
- I is the current
- A is the area of the loop
- B is the magnetic field
The magnetic Moment of the current loop is m = IA or m = IA.
The torque acting on the current loop is
τ = m x B
If the loop has N closely wound turns, then torque will be equal to
τ = N m x B
or τ = NIABsinθ
This principle is used in electric motors, where the interaction between the magnetic field and current produces rotational motion.
Circular Current Loop as a Magnetic Dipole
The magnetic field on the axis of the circular loop of radius R and carrying steady current I is
B = 0 IR²/2(x² + R²)³/₂
For x>>R, we get
B = 0 IR²/2x³
The area of the loop is R².
B = 0 IA/2x³
B = 0 m/2x³ (Magnetic moment m = IA)
B = 0 2 m/4x³
The Magnetic Dipole Moment of a Revolving Electron
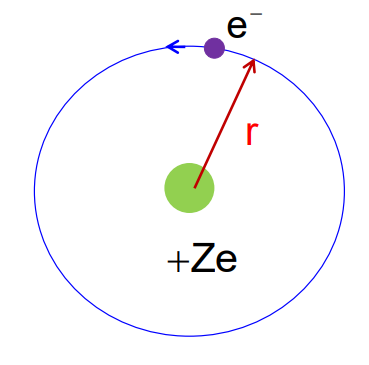
Let us consider an electron, revolving around a heavy stationary nucleus in a uniform circular motion. This gives rise to current, I = e/T.
The time period of a revolution is given by: T = 2r/v.
There will be a magnetic moment generally denoted by I.
Its magnitude is given by: I = e x I/2me
where, l = mvr = angular momentum
Vectorially, I = – e x I/2me

The negative sign indicates that the angular momentum of an electron acts in the opposite direction to the magnetic moment.
Moving Coil Galvanometer

It works on the principle that when a current-carrying coil is suspended in a magnetic field, then it experiences a torque.
The deflection of the needle can be used to measure the current and voltage of an electrical circuit.
Construction
- It consists of a coil with many turns.
- It is free to rotate about a fixed axis in a uniform radial magnetic field.

Working
When a current flows through the coil, the torque acts on it, which is given by:
τ = NIAB
N: number of turns of the coil
A: area of the circular loop
B: magnetic field
I: current flowing through the circular loop.
The given torque tends to rotate the coil and in turn, the spring provides the counter torque, which balances the torque due to the current loop.
τ = k
Where k is the torsional constant of the spring.
In the equilibrium position, k = NIAB
= NIAB/k
Therefore, = (NAB/k)I
NAB = Galvanometer constant
Galvanometer as an Ammeter
- A galvanometer can be used to measure the current in a circuit.
- For it, the galvanometer is shunt with a low resistance resistor Rs. It is connected in series with the circuit.
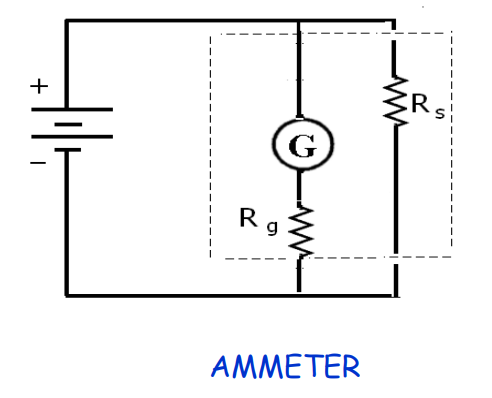
Current Sensitivity
- The current sensitivity of the galvanometer is defined as the deflection per unit current.
- It is equal to /I = (NAB/k)
- To increase the sensitivity, we can increase the number of turns N.
Galvanometer as an Voltmeter
- A galvanometer can be used as a voltmeter.
- For it, the galvanometer is connected in series with a high resistance resistor R.
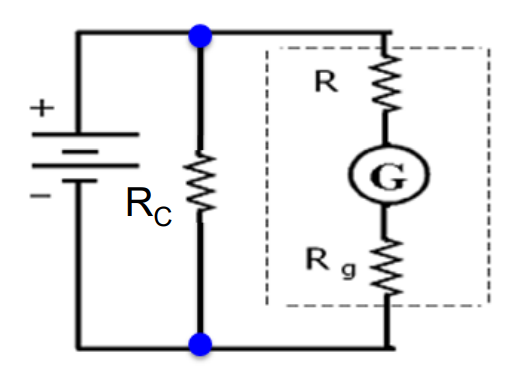
- The whole arrangement is then connected in parallel across a given section of the circuit.
Voltage Sensitivity
- The voltage sensitivity of the galvanometer is defined as the deflection per unit voltage.
- It is equal to /V = (NAB/k) x 1/R (R = V/I)
Conclusion
This chapter offers a comprehensive understanding of the interplay between moving charges and magnetism, introducing key concepts that form the foundation of electromagnetism. Through practical applications like the cyclotron, solenoids, and galvanometers, students can appreciate how these principles are applied in everyday technologies.
Practice questions on Chapter 4 - Moving Charges and Magnetism
Get your free Chapter 4 - Moving Charges and Magnetism practice quiz of 20+ questions & detailed solutions
Practice Now








