Linear Equations In One Variable – Complete Guide For Class 8 Math Chapter 2

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Linear Equations in One Variable in Mathematics Class 8th chapter 2 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.

The chapter “Linear Equations in One Variable” for Class 8 Mathematics introduces students to equations that involve only one variable and a single solution. It covers methods to solve equations, including transposing terms and balancing, and applies these concepts to real-life problems, enhancing logical thinking and problem-solving skills.

Linear Equations in One Variable

In the chapter Linear Equations in One Variable for class 8, we delve into the fascinating world of linear equations in one variable. These equations, which involve variables with the highest power of one, are fundamental to understanding algebra.

Linear Equation

Now we will learn about Linear equations in One Variable for class 8 chapter 2 in mathematics.

A linear equation is an equation of the first order, meaning its highest power is one. It typically takes the form:

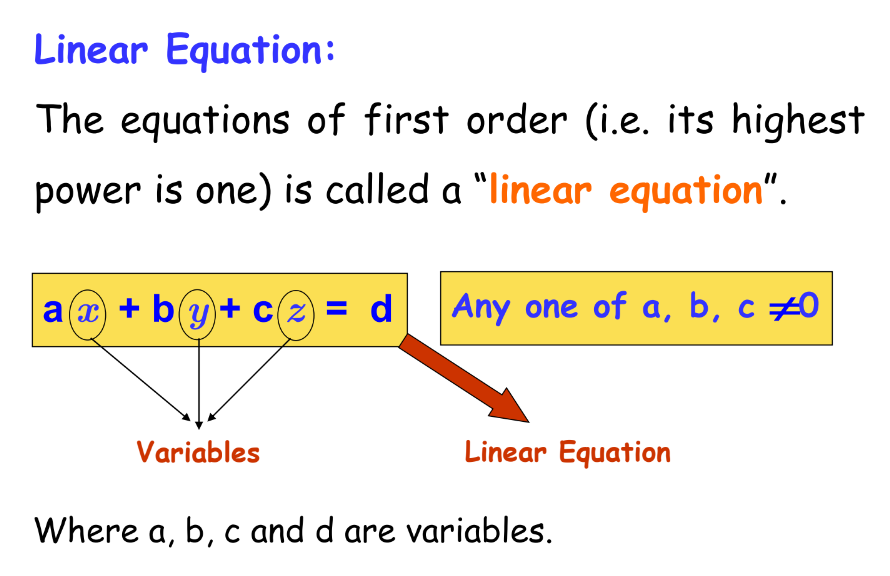
ax + by + cz = d 

where at least one of a, b, or c is non-zero.

Linear Equation in One Variable:

When a linear equation involves only one variable, it is termed a “linear equation in one variable.” The general form is:

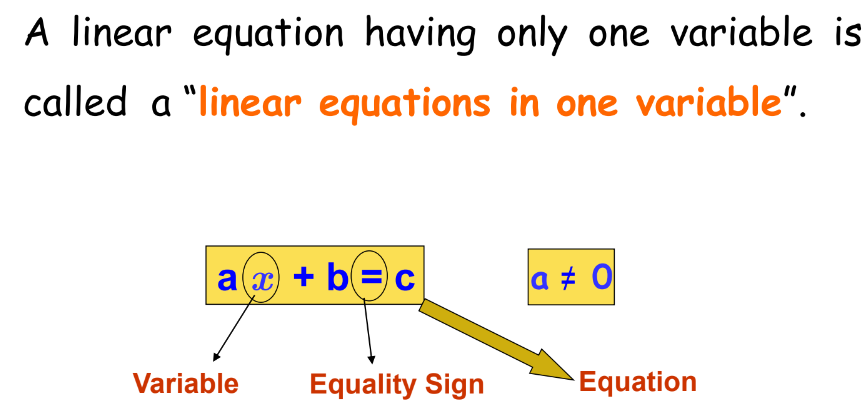
ax + b = c (a ≠ 0)

Here, a, b, and c are constants and x is the variable. Examples include:

1. 2x+5 = 8, where x is the variable.
2. 7a+10 = 0, where a is the variable.
3. 11y+1= 23, where y is the variable.

Solution of Linear Equations

We have already learned about linear equations in one variable. Now, we will learn how to solve these linear equations.

A solution of a linear equation is a value of the variable that satisfies the equation, making the left-hand side (LHS) equal to the right-hand side (RHS). For instance:

Example:

Solve the equation 4x − 11 = 5

Solution:

1. Rearrange the equation: 4x = 5+11
2. Simplify: 4x = 16
3. Solve for x: x=16/4 = 4

Thus, x = 4 is the solution.

Types of Linear Equations in One Variable

There are three types of linear equations in one variable in class 8

Let us discuss them one by one.

1. Equations with the Variable on One Side:
   • For these equations, we isolate the variable on one side and the constants on the other before solving.

Example:

Solve:  2x − 7 = 5

Solution:

1. Rearrange: 2x = 5+7
2. Simplify: 2x = 12
3. Solve for x: x=6

2.  Equations with Variables on Both Sides:

Here, variables appear on both sides of the equation.

Example:

Solve 3z + 7 = 5z − 11

Solution:

1. Rearrange: 7+11 = 5z−3z
2. Simplify: 18 = 2
3. Solve for z: z = 9

3. Equations Involving Fractions:

Equations with fractions require simplification into linear form before solving.

Example:

Solve: 3/2x + 11 = 3/4

Solution:

1. Rearrange: 3/2x = 3/4 − 11
2. Cross-multiply and solve for x.

Applications of Linear Equations in One Variable

After learning about the solution of linear equations in one variable we will see how we are going to use these linear equations in one variable in our daily life.

Example 1:

Problem: The sum of three consecutive even integers is 270. Find the integers.

Solution: Let the first even integer be x, the second x+2x, and the third x+4.

1. Set up the equation: x + (x+2) + (x+4) = 270
2. Simplify: 3x + 6 = 270.
3. Solve for x: x=88

Thus, the integers are 88, 90, and 92.

Example 2:

Problem: Srishti has a total of ₹780 in notes of ₹10, ₹20 and ₹50. The ratio of the number of ₹50 notes to ₹10 notes is 3:2. If she has 32 notes in total, find the count of each note.

Solution:

1. Let the number of ₹50 notes be 3x and ₹10 notes be 2x. Then, ₹ 20 notes will be 32−5x.
2. Set up the equation based on the total amount: 150x + 20(32−5x) + 20x = 780.
3. Solve for x.

She has 6 – ₹50 notes, 4 – ₹10 notes and 22 – ₹20 notes.

This chapter’s exploration of linear equations in one variable provides a crucial foundation for more advanced algebraic concepts. Through practice and application, students can develop strong problem-solving skills essential for mathematical proficiency.