Complete Guide For Class 9 Math Chapter 3 – Coordinate Geometry

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Coordinate Geometry in Mathematics Class 9th chapter 3 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.

Chapter 3 of Class 9 Coordinate Geometry introduces the Cartesian plane, coordinates, and plotting points. It covers the distance formula, section formula, and midpoint formula, helping students understand the geometric representation of algebraic equations and the relationships between points in a plane.

In Chapter 3 of Class 9 Mathematics, we delve into the fascinating world of Coordinate Geometry. This chapter introduces students to the concept of locating points on a plane using a system of coordinates. The Cartesian plane, named after the mathematician René Descartes, forms the foundation of this system. By understanding the Cartesian system, students learn how to plot points using two perpendicular axes, the x-axis and the y-axis, and the concept of coordinates (x, y).

Activity: Locating Points

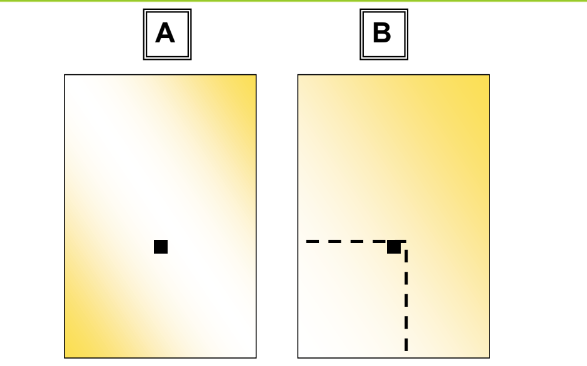
Suppose we are given two charts labeled A and B, each with a dot. How can we find the location of the dot? To locate its position, we draw two perpendicular lines – one vertical and the other horizontal – on the plane and measure its distance from these lines. This simple exercise demonstrates that to locate a point in a plane, at least two pieces of information are needed: the distances from the point to the horizontal and vertical lines.

Seating Arrangement Example

Consider the seating arrangement in a classroom, with rows and columns labeled for easy reference:

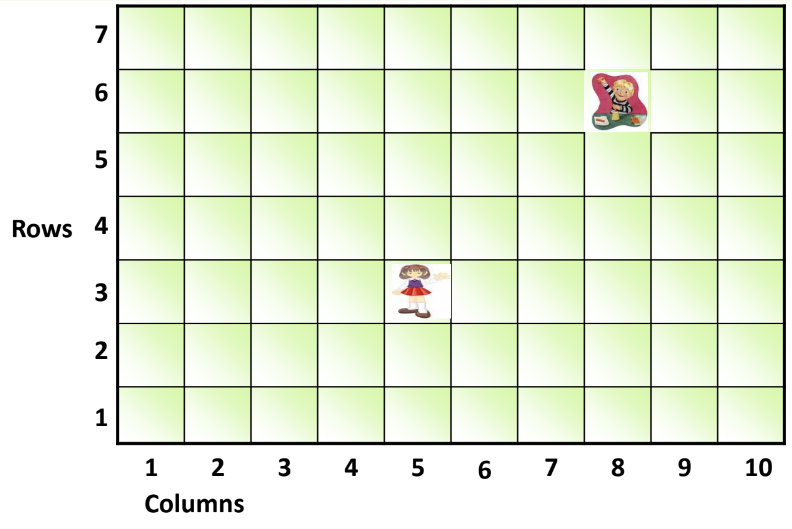
For example, the position of a girl might be in the 5th column and 3rd row, while a boy might be seated in the 8th column and 5th row. This practical example helps illustrate the concept of locating points using coordinates.

The Origin of Coordinate Geometry

The concept of coordinate geometry originated with René Descartes, a great French mathematician of the seventeenth century. Descartes enjoyed lying in bed and thinking, and one day, he devised a method for describing the position of a point in a plane. His approach built upon the older idea of latitude and longitude. In his honor, the system is also known as the Cartesian system.

Understanding the Cartesian System

In the Cartesian system, a plane is divided into four quadrants by two perpendicular lines: the x-axis (horizontal) and the y-axis (vertical). The point where these axes intersect is called the origin, denoted as ‘O’. The coordinates of any point on the plane are represented as (x, y), where ‘x’ is the abscissa (distance along the x-axis) and ‘y’ is the ordinate (distance along the y-axis).

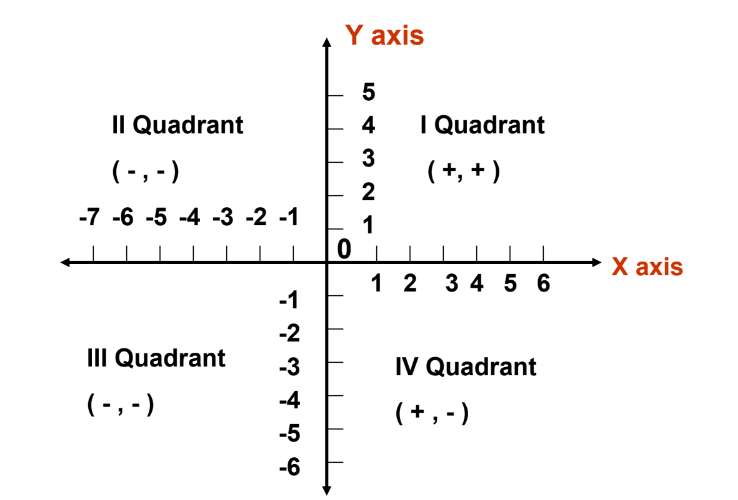
The four quadrants are defined as:

1. First Quadrant: Both x and y coordinates are positive.
2. Second Quadrant: x is negative, y is positive.
3. Third Quadrant: Both x and y coordinates are negative.
4. Fourth Quadrant: x is positive, y is negative.

Example of Plotting Points

To plot a point such as (2, 2), start from the origin and move 2 units along the positive x-axis and then 2 units along the positive y-axis. This point lies in the first quadrant. Similarly, (3, -4) lies in the fourth quadrant, and (-4, 3) lies in the second quadrant.

Coordinate Representation

The coordinates for specific points on a graph might be:

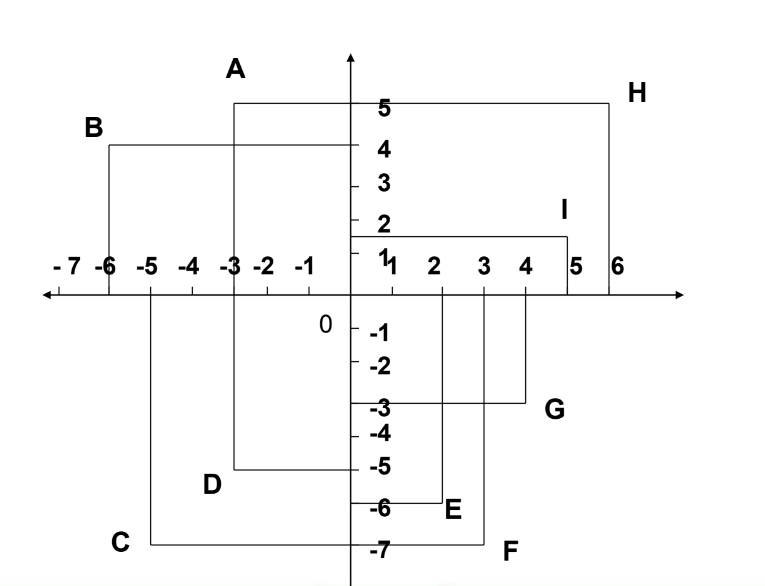
• ‘A’ ( -3, 5 )
• ‘B’ ( -6, 4 )
• ‘C’ ( -5, -7 )
• ‘D’ ( -3, -5)
• ‘E’ (2, -6)
• ‘F’ (3, -7 )
• ‘G’ (4, -3)
• ‘H’ (6, 5)
• ‘I’ (5, 1.5)

Algorithm for Plotting Points

To plot a point on a Cartesian plane, follow these steps:

1. Draw two mutually perpendicular lines on the graph paper: one horizontal (x-axis) and one vertical (y-axis). Mark their intersection as the Origin ‘O’.
2. Choose a suitable scale for the axes and mark the units.
3. Obtain the coordinates of the point to be plotted, P(a, b). Start from the origin, move |a| units along the x-axis, then |b| units along the y-axis, depending on whether the values are positive or negative.

This systematic approach helps in accurately plotting points and understanding their positions on the Cartesian plane.

In conclusion, Class 9 Math Chapter 3 – Coordinate Geometry is a fundamental topic that introduces students to the Cartesian system, which plays a crucial role in understanding the geometric representation of algebraic equations. By mastering concepts such as the distance formula, section formula, and midpoint formula, students can effectively plot points and analyze their relationships on a plane. Through the study of Chapter 3 – Coordinate Geometry, learners will develop a solid foundation in spatial reasoning, which is essential for more advanced mathematical concepts in the future.

With iPrep’s engaging resources, including animated videos, practice questions, and detailed notes, students can confidently navigate Chapter 3 – Coordinate Geometry. These tools ensure a clear understanding of the Cartesian plane and its applications, making learning more interactive and enjoyable. Whether it’s preparing for exams or reinforcing concepts, iPrep’s comprehensive approach ensures success in mastering Class 9 Math Chapter 3 – Coordinate Geometry.