Complete Guide For Class 9 Math Chapter 10 – Heron’s Formula
Our learning resources for Mathematics Class 9 Heron’s Formula are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions, and notes offer you the best of integrated learning with interesting explanations and examples.
Our comprehensive approach ensures that you have access to everything you need to have an in-depth understanding of the chapter Heron’s Formula. From detailed notes to interactive exercises, our materials are tailored to meet your learning needs and help you excel in your studies. Get ready to dive into an enriching educational experience that will make mastering this chapter a breeze.
Chapter 10: Heron’s Formula in Class 9 Mathematics introduces a method to calculate the area of a triangle when the lengths of all three sides are known. This formula, attributed to the ancient mathematician Heron of Alexandria, provides a practical approach to solving real-world problems involving triangular plots of land and various geometric applications.
We all know the area of a triangle can be calculated if we know the base and altitude. However, there may be cases when the height is not known. Let us analyze various conditions.
Calculation of Area of a Right-Angled Triangle
According to the chapter heron’s formula, in any right-angled triangle, we can calculate its area if the base and the perpendicular are known.
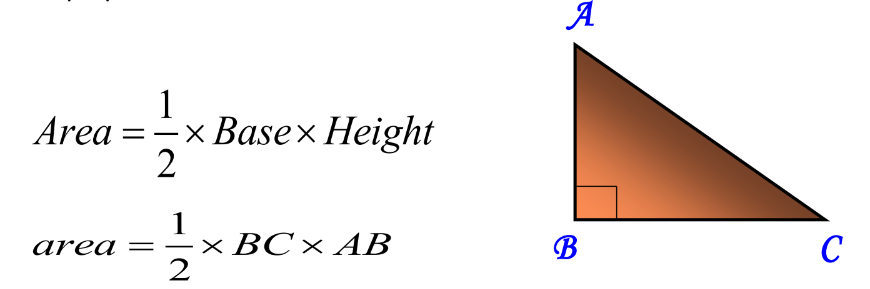
Area = 1/2 × Base × Height
Example: Area = 1/2 × BC × AB
Note: We can take the base as BC or AB; that will not make any difference in the calculation of its area.
Calculation of Area of an Equilateral Triangle
We draw the perpendicular from vertex A of the triangle. In an equilateral triangle, the perpendicular bisects the opposite side of the triangle.
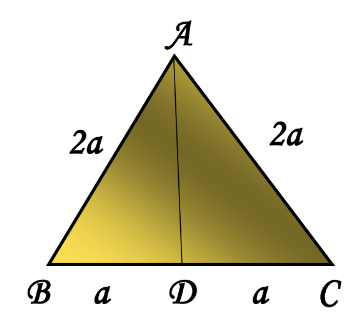
AD = √AB² − BD²
AD = √(2a)² − (a)²
AD = √4a² − a²
AD = √3a² = a√3
Calculation of the area of an equilateral triangle:
Area = 1/2 × Base × Height
Area = 1/2 × BC × AD
Area = 1/2 × 2a × a√3
Area = a²√3
Calculation of the Area of an Isosceles Triangle
In an isosceles triangle, two of the sides are equal. Let us take an example of a triangle XYZ where the equal sides are 5 cm each and the unequal side is 8 cm.
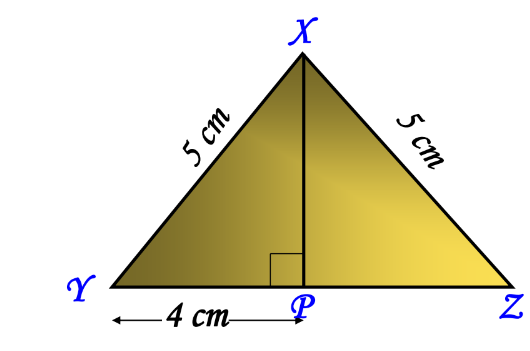
The perpendicular XP divides the base YZ of the triangle into two equal parts.
Therefore, YP = PZ = 1/2YZ = 4 cm
To find the altitude by applying Pythagoras Theorem:
XP = √XY²−YP²
XP = √5² − 4²
XP = √25 − 16 = √9 = 3 cm
Now, the area of the triangle:
Area = 1/2 × Base (YZ) × Height (XP)
Area = 1/2 × 8 × 3 = 12 cm²
Heron’s Formula
In a triangle ABC, let the side opposite to vertex A be denoted by a (side BC), the side opposite to vertex B be denoted by b (side AC), and the side opposite to vertex C be denoted by c (side AB).

The semi-perimeter (s) of ΔABC is given by:
S = a + b + c/2
The area of the triangle using Heron’s Formula:
Area = √s(s−a)(s−b)(s−c)
Example 1
Find the area of a triangle whose sides are respectively 9 cm, 12 cm, and 15 cm.
Solution:
The semi-perimeter s = 9+12+15/2 = 18
Area = √18(18−9)(18−12)(18−15)
Area = √18×9×6×3
Area = √2916 = 54 cm²
Example 2
In a triangle ABC, AB = 15 cm, BC = 13 cm, and AC = 14 cm. Find the area of the triangle.
Solution:
The semi-perimeter S = 15+13+14/2 = 21
Area = √21(21−15)(21−13)(21−14)
Area = √21×6×8×7
Area = √7056 = 84 cm²
Example 3
The lengths of the sides of a triangle are in the ratio 3:4:5 and its perimeter is 144 cm. Find the area of the triangle and the height corresponding to the longest side.
Solution:
Let the sides be denoted by 3x, 4x, and 5x.
144 = 3x + 4x + 5x
144 = 12x
x = 12
Thus, the sides are 36 cm, 48 cm, and 60 cm.
The semi-perimeter S = 144/2 = 72
Area = √72(72−36)(72−48)(72−60)
Area = √72×36×24×12 = 864 cm²
Now we need to find the altitude to the longest side:
Area = 1/2 × Base × Height
864 = 1/2 × 60 × Height
Height = 864 × 2/60 = 28.8 cm
Example 4
A triangular park ABC has sides 120 m, 80 m, and 50 m. The owner wants to put a fence all around it and also plant grass inside. How much area does he need to plant? Find the cost of fencing it with barbed wire at the rate of Rs. 50 per meter, leaving a space 3m wide for a gate on one side.
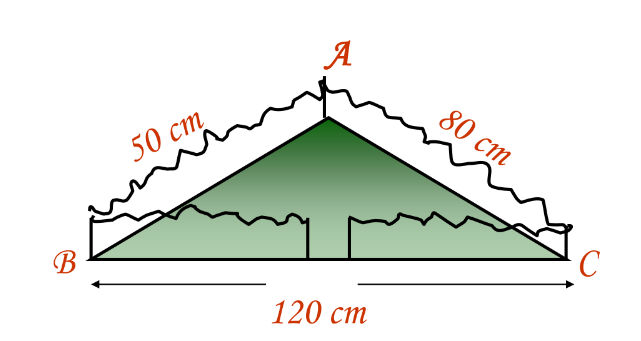
Solution:
Computation of area:
The semi-perimeter S = 120+80+50/2 = 125
Area = √125(125−120)(125−80)(125−50)
Area = √125×5×45×75 = 3750 m²
Length of wire needed for fencing:
Perimeter of the park − Width of the gate = 250m − 3m = 247m
Cost of fencing:
Cost = Rs.50×247 = Rs.12,350
By understanding Heron’s Formula and its applications, students can easily calculate the area of various triangles and quadrilaterals, enhancing their problem-solving skills in geometry.
In summary, Chapter 10 – Heron’s Formula is a pivotal part of Class 9 Mathematics, offering a powerful tool for calculating the area of a triangle when all three side lengths are known. By mastering Heron’s Formula, you gain the ability to tackle a variety of geometric problems, from practical applications in real-world scenarios to solving complex exercises in your textbook. Our resources for Class 9 Math Chapter 10 – Heron’s Formula provides a thorough understanding through detailed notes, animated videos, and ample practice questions. With these tools, you can confidently approach any problem involving triangles and apply Heron’s Formula effectively. Embrace this chapter’s concepts to enhance your mathematical skills and excel in your studies.
Practice questions on Chapter 10 - Heron's Formula
Get your free Chapter 10 - Heron's Formula practice quiz of 20+ questions & detailed solutions
Practice Now








