Complete Guide For Class 9 Math Chapter 1 – Number Systems

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Number Systems in Mathematics Class 9th Chapter 1 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.

This chapter introduces the real number systems, including rational and irrational numbers. It explores properties like closure, commutativity, associativity, and distributivity. The chapter “Number Systems” also covers the representation of real numbers on the number line, decimal expansions, and the concept of irrational numbers, including √2 and π.

Welcome to our comprehensive guide on “Number Systems,” the foundational chapter in Class 9 Mathematics. This chapter delves into the essential concepts of real numbers, exploring the different types, their properties, and how they interact. Here’s an overview of the topics covered in this chapter:

In this chapter, students will learn about:

• The basic concepts of number systems.
• Rational numbers and their decimal representations.
• Irrational numbers and their unique properties.
• Problems related to both rational and irrational numbers.
• The representation of rational and irrational numbers on the number line.

Basics of Real Numbers

In the chapter “Number systems” of class 9 we will first learn about some basics of real numbers which are given below.

Natural Numbers: The counting numbers, starting from 1 (1, 2, 3, …), are known as natural numbers.

Whole Numbers: All natural numbers, along with zero, form the set of whole numbers (0, 1, 2, …).

Integers: This includes all positive and negative whole numbers, along with zero (-3, -2, -1, 0, 1, 2, …).

Now that we understand, the basics of real numbers, let us understand another very important aspect of the chapter – Number Systems, called Rational Numbers.

Introduction to Rational Numbers

According to the chapter Number Systems, Rational numbers are numbers that can be expressed in the form p/q, where p and q are integers, and q ≠ 0. Examples include 0, -3, 4/7, and -8/15.

• Note: All natural numbers, whole numbers, and integers are considered rational numbers.

Representation of Rational Numbers

As stated in the chapter Number Systems, Rational numbers can be classified based on their decimal representation into:

• Terminating Decimals: These decimals come to an end (e.g., 0.5, 0.28).
• Non-Terminating, Repeating Decimals: These decimals repeat a pattern indefinitely (e.g., 0.333…, 2.142857…).

Irrational Numbers

Irrational numbers cannot be expressed in the form of p/q. Their decimal representations are non-terminating and non-repeating. Examples include √2 (1.414…) and √3 (1.73…).

Real Numbers

The set of all rational and irrational numbers forms the set of real numbers. Examples include 0, -3/2, 7, 2, 3, -1/5, 3/4, 1, and 8/7.

Problems With Rational Numbers

Students learn to:

• Insert rational numbers between any two given rational numbers.
• Represent decimal expressions as rational numbers.

For example, to insert five rational numbers between 2 and 3:

• Convert the numbers to a common denominator and then select the numbers in between.

Representing decimal expressions as rational numbers.

In mathematics, any repeating or terminating decimal can be expressed as a fraction, which is a form of a rational number. Here’s how to convert various types of decimal expressions into their equivalent rational numbers:

1. Terminating Decimals

A terminating decimal is one that comes to an end. To convert a terminating decimal into a fraction:

1. Write the decimal number over a power of 10 (depending on the number of decimal places).
2. Simplify the fraction if necessary.

Example: Convert 0.75 into a fraction.

• 0.75 = 75/100
• Simplify: 75/100 = 3/4

2. Non-Terminating, Repeating Decimals

A repeating decimal has one or more repeating digits after the decimal point. To convert a repeating decimal into a fraction:

1. Let x be the repeating decimal.
2. Multiply x by a power of 10 such that the decimal part after the point repeats.
3. Subtract the original x from this new expression to eliminate the repeating part.
4. Solve for x.

Example: Convert 0.333… (where 3 repeats) into a fraction.

• Let x = 0.333…
• Multiply by 10: 10x=3.333…
• Subtract the original equation: 10x − x = 3.333…−0.333…
• 9x = 3 
• x = 3/9 = 1/3

3. Non-Terminating, Non-Repeating Decimals

Non-terminating, non-repeating decimals cannot be represented as a fraction of integers, and thus are considered irrational numbers.

Representation of Irrational Numbers on the Number Line

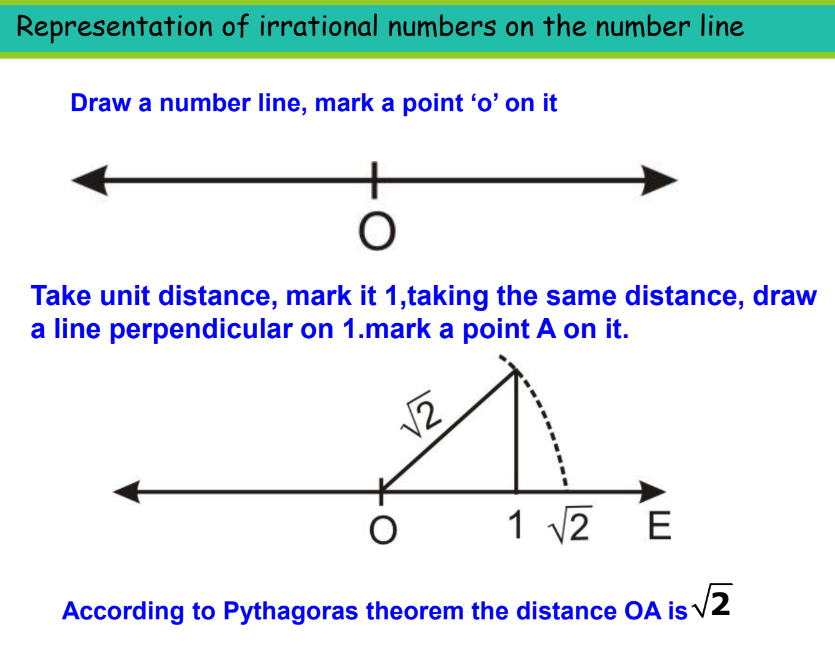
This section teaches how to locate irrational numbers, like √2, on the number line using geometric constructions based on the Pythagorean theorem.

Rationalization of Given Expressions

In the chapter Number Systems of class 9, this section involves removing irrational numbers from the denominator, making the expression rational.

Rationalize the expression: 3/√5−2

Multiply the numerator and the denominator by the conjugate:

 = 3/√5−2 × (√5+ 2/√5+2)

= 3(√5 + 2)/ (√5−2) × (√5+ 2)

= 3√5 + 6/1

= 3√5 + 6

Problems Related to Irrational Numbers

Students practice inserting irrational numbers between given numbers and representing them on the number line using successive magnification.

Insert irrational numbers between √2 and √3.

Solution: You know that the decimal representation of √2 and √3 are 1.414… and 1.732…respectively.

Now you can find any number greater than 1.414… and 1.73… such that it is non-terminating and non-repeating.

For example, we can take 1.50500500050000…

Hint: take any number of your choice whose value lies between the two given values.

Representing numbers by successive magnification methods

Represent 8.66 on the number line.

Solution: Step 1: Draw a number line

Step 2: 8.66 will lie between 8 and 9. So create 10 subdivisions between 8 and 9, locate 8.6

Step 3: 8.66 will lie between 8.6 and 8.7. Create 10 subdivisions between 8.6 and 8.7.

Step 3: 8.66 will lie between 8.6 and 8.7. Create 10 subdivisions between 8.6 and 8.7.

Step 4. The 6th division is 8.66

Exponents

Understanding positive and negative exponents, along with the laws governing integral exponents, is crucial in this chapter.

• Positive Exponents: Indicate how many times to multiply the base by itself.
• Negative Exponents: Represent the reciprocal of the base raised to the corresponding positive exponent.

Laws of Integral Exponents

According to the chapter Number Systems, Key laws of integral exponents include:

• Multiplication: aᵐ × aⁿ = aᵐ ⁺ ⁿ
• Division: aᵐ / aⁿ = aᵐ ⁻ ⁿ 
• Power of a Power: (aᵐ)ⁿ = a ᵐ ˣ ⁿ

Powers with Negative Exponents

Can exponents be negative? Yes, they can! For example:

• 5¹ = 5 
• 5² = 5×5 = 25
• 5⁰ = 1 (any number to the power of zero is 1)
• 5⁻¹ = 1/5.  ​Negative exponents indicate reciprocals. Thus, 5⁻¹ means 1/5.

Properties of Irrational Numbers

The properties include:

• The sum or difference between a rational and an irrational number is irrational.
• The sum or difference of two irrational numbers can be either rational or irrational.
• The product or quotient of two irrational numbers can also be rational or irrational.

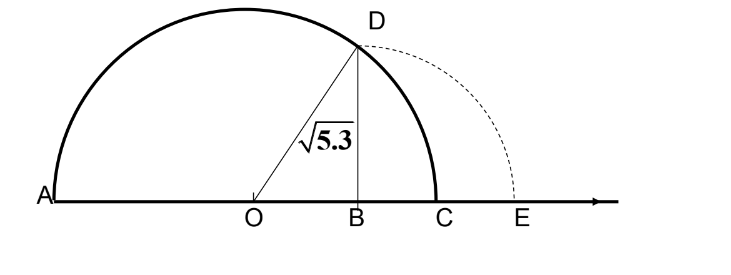
Locating a Square Root on the Number Line

Using geometric methods, students learn to locate square roots of positive real numbers on the number line, such as finding the position of √5.3.

Step1: Draw a line and mark a point A on it.

Step 2: Mark a point B such that AB=5.3 cm.

Step 3: Mark a point C on AB produced such that BC=1 unit.

Step 4. Find the midpoint of AC. Let the midpoint be O.

Step 5. Taking O as the center, and radius OC=OA as the radius, draw a semi-circle. Also, draw a line passing through B perpendicular to OB. Suppose it touches the semi-circle at D.

Step 6. Taking B as center and BD as radius cut an arc on at point E.

This chapter lays a strong foundation for understanding different types of numbers and their properties, essential for further studies in mathematics. Dive into the exercises and examples to gain a deeper understanding of the number systems!

In conclusion, the Class 9 Math Chapter 1 “Number Systems” serves as a crucial building block in your mathematical journey. Through this chapter, you’ve explored the diverse realms of rational and irrational numbers, their properties, and their representation on the number line. By mastering these concepts, you’ll not only strengthen your foundational understanding but also be well-prepared for more advanced topics in mathematics.

The chapter “Number Systems” intricately covers everything from the basics of real numbers to the complexities of irrational numbers and their rationalization. By engaging with the chapter’s content, including its problems and examples, you will develop a robust comprehension of how numbers function and interact. Remember, whether it’s understanding rational numbers, representing them on the number line, or dealing with irrational numbers, this chapter provides you with the essential tools needed for future mathematical challenges.

Dive deep into the chapter “Number Systems,” practice diligently, and use the resources available to you. Embrace the fascinating world of numbers and let this chapter be a stepping stone towards excelling in your math studies. Happy learning!