Complete Guide Class 9 Math Chapter 2 – Polynomials

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Polynomials in Mathematics Class 9th chapter 2 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.

Chapter 2, “Polynomials,” in Class 9 Mathematics explores algebraic expressions consisting of variables and coefficients. It covers definitions, types, degrees, and zeros of polynomials, along with algebraic identities and their applications. The chapter provides foundational knowledge for understanding polynomial equations and their properties.

Polynomials are an integral part of algebra, offering a foundation for various mathematical concepts. This chapter explores the fascinating world of polynomials, breaking down complex ideas into digestible pieces for Class 9 students.

Basic Concepts: Building Blocks of Polynomials

Constants: These are fixed numerical values, such as 5, 100, or 3.

Variables: Unlike constants, variables can take different values. Common examples include x, y, z, a, b, and c.

Algebraic Expressions: These are combinations of variables and constants separated by addition or subtraction. For instance, the area of a square with side x is represented as x², an algebraic expression.

Understanding Polynomials

A polynomial is a specific type of algebraic expression with non-negative exponential powers. For example, x² – 2xy + y² is a polynomial with two variables, x and y. Similarly, x⁴ + 4x³ – 2x² + 7x – 5 is a polynomial with the variable x.

Terms and Coefficients: In the polynomial f(x) = aₙxⁿ + aₙ₋₁xⁿ⁻¹ + … + a₀, each part like aₙxⁿ is a term, and the numerical factors like aₙ are coefficients.

Classifying Polynomials by Terms

Monomials: Polynomials with a single term, such as x² or 2x.

Binomials: Polynomials with two terms, like x² + 3 or 2xy – 8.

Trinomials: Polynomials with three terms, such as y³ – 3y² + 8.

Determining the Degree of a Polynomial

The degree of a polynomial is the highest power of its variable. For example, the polynomial y⁴ – 3y³ + 7x + 8 has a degree of 4. Based on their degree, polynomials can be classified as:

Linear: Degree 1 (e.g., x + 4) 

Quadratic: Degree 2 (e.g., x² + 2x + 1) 

Cubic: Degree 3 (e.g., x³ – 3x² + 2x + 5)

Value and Zeros of Polynomials

The value of a polynomial at a specific point can be found by substituting the variable with the given number. For example, f(x) = 2x³ – 3x² + 4x – 2 at x = 2 gives f(2) = 10.

Zeros of a Polynomial: A zero (or root) of a polynomial is a value that makes the polynomial equal to zero. For instance, in the polynomial x³ – 6x² + 11x – 6, x = 2 is a root because f(2) = 0.

Finding Zeros of Polynomials

For a linear equation like ax + b = 0, the zero is -b/a. For quadratic equations, roots can be found using methods such as splitting the middle term.

Classification Of Polynomials

Now, with the help of the image given below, we will see the classification of polynomials for class 9 Mathematics Chapter 2

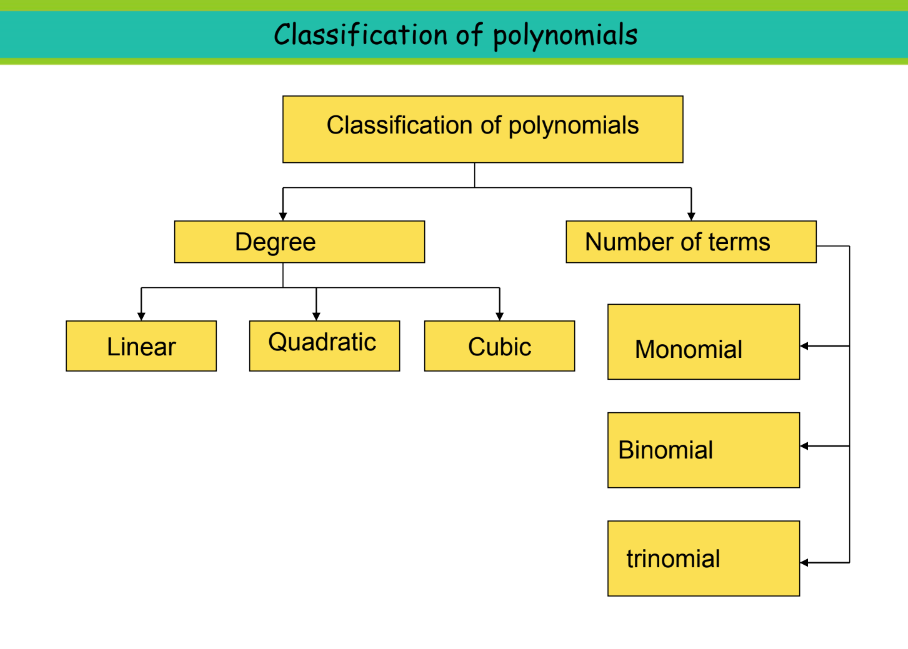
Division of Polynomials

Just like integers, polynomials can be divided, yielding a quotient and a remainder. For example, dividing y³ + y² + 2y + 3 by y + 2 gives a quotient of y² – y + 4 and a remainder of -5.

Remainder Theorem: This theorem states that the remainder of a polynomial f(x) when divided by x – a is f(a).

Factor Theorem: This theorem links the zeros of a polynomial to its factors. It states that (x – a) is a factor of a polynomial f(x) if and only if f(a) = 0.

Factorization Techniques Of Polynomials

Polynomials can be factorized in various ways:

1. Common Factors: Identifying common factors in each term.
2. Grouping: Combining like terms for factorization.
3. Perfect Squares: Utilizing identities like (a + b)² = a² + 2ab + b².
4. Difference of Squares: Applying a² – b² = (a – b)(a + b).
5. Splitting the Middle Term: Commonly used for quadratic polynomials.
6. Cubic Identities: Utilizing formulas like (a + b)³ = a³ + 3a²b + 3ab² + b³.

Conclusion

This chapter provides a solid foundation for understanding and working with polynomials, from basic definitions to complex factorization techniques. As you delve into these concepts, you’ll gain a deeper appreciation for the beauty and utility of algebraic expressions in mathematics.

For more detailed explanations and examples, feel free to explore our resources and join our community discussions!