Coordinate Geometry – Complete Guide For Class 10 Math Chapter 7

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Coordinate Geometry in Mathematics for Class 10th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Coordinate Geometry introduces students to the system of locating points on a plane using coordinates. By understanding the coordinate plane, which is formed by the intersection of the x-axis and y-axis, students learn to plot points and determine their exact positions using ordered pairs (x, y). The chapter covers the distance formula, enabling students to calculate the distance between two points on the plane. Additionally, students explore the section formula to find the coordinates of a point dividing a line segment in a given ratio, and the area of a triangle using vertex coordinates. Mastery of these concepts aids in solving geometrical problems and enhancing spatial reasoning and analytical skills.

Coordinate Geometry

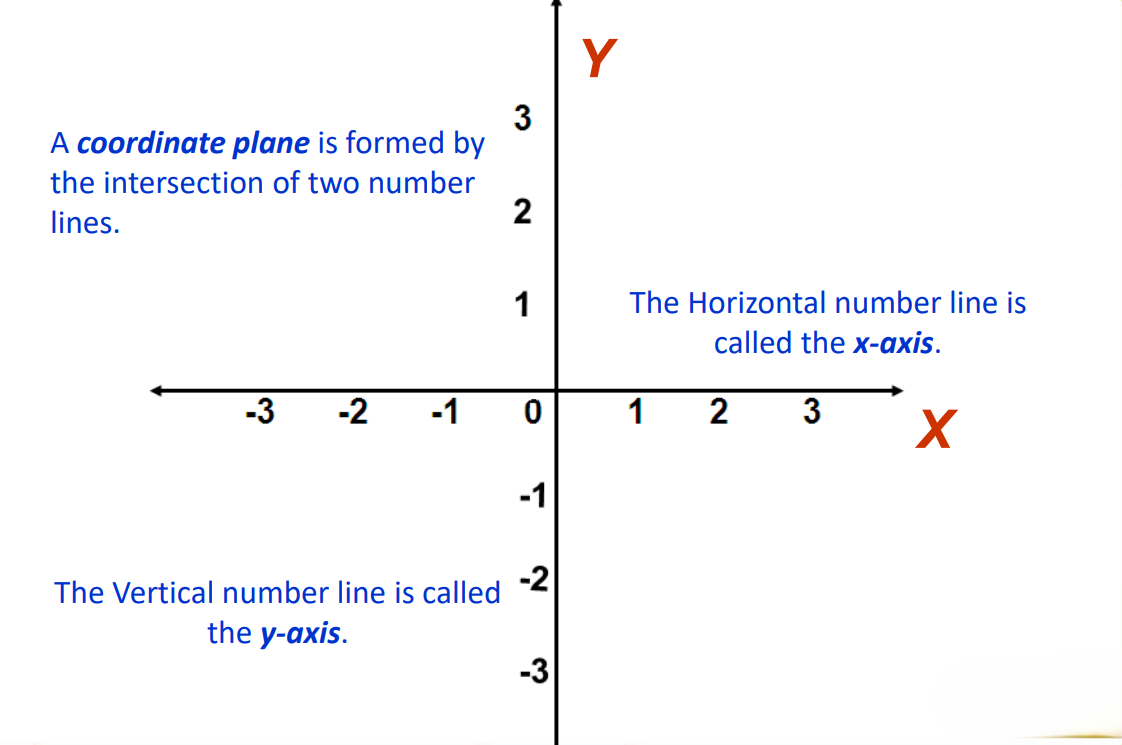
Coordinate Geometry focuses on the relationship between algebra and geometry through the use of coordinate planes. By placing two number lines perpendicular to each other, we can locate points on a plane and refer to these points using their coordinates.

Coordinate Plane

A coordinate plane is formed by the intersection of two number lines:

• X-axis: The horizontal number line.
• Y-axis: The vertical number line.

The point where these two axes intersect is known as the origin, usually denoted by ‘O’. The coordinate plane is divided into four regions called quadrants.

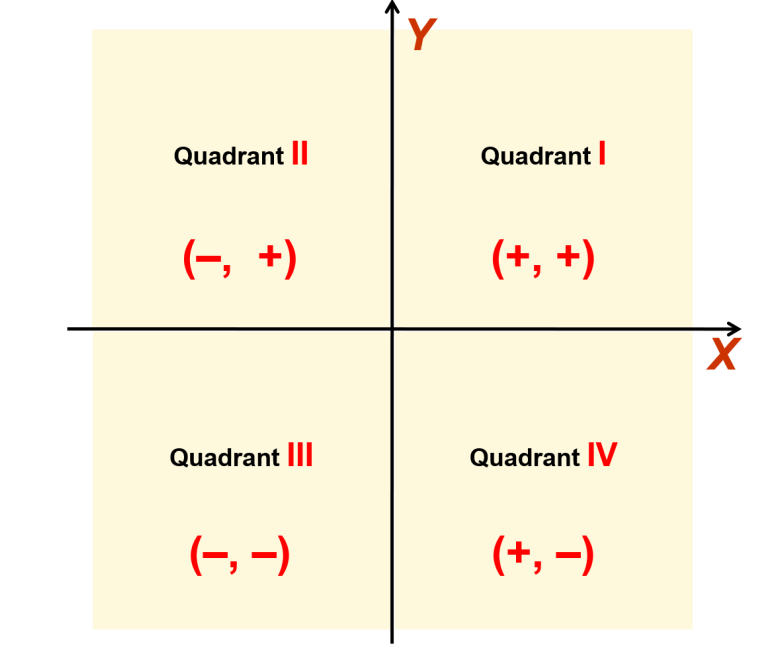
Quadrants and Their Signs

Each of the four quadrants has its specific sign for coordinates:

• First Quadrant: (+, +)
• Second Quadrant: (-, +)
• Third Quadrant: (-, -)
• Fourth Quadrant: (+, -)

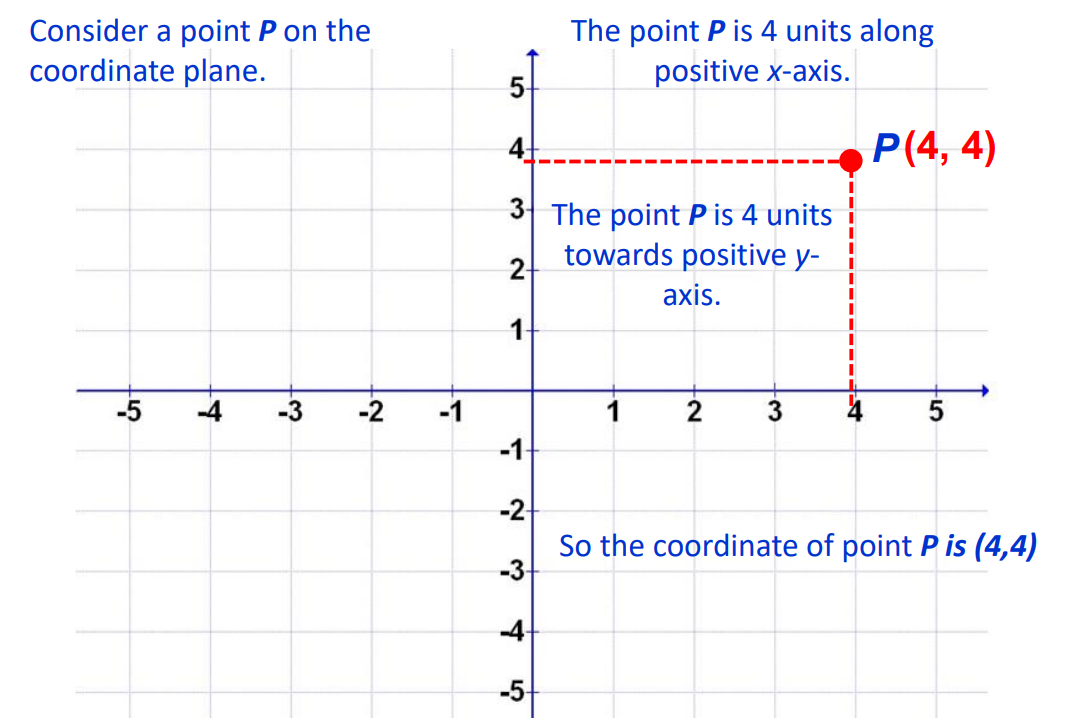
Coordinates are always written in parentheses, with the x-value first, followed by the y-value. For instance, in points (4, 4), the x-coordinate is 4 units along the positive x-axis, and the y-coordinate is 4 units along the positive y-axis.

Distance Between Two Points

The distance between two points on the coordinate plane can be calculated depending on their position:

1. Points on the X-axis: The distance is simply the difference between their x-coordinates.
2. Points on the Y-axis: The distance is the difference between their y-coordinates.
3. Points on the coordinate axes: Use the Pythagoras theorem to find the distance between the two points.
4. Points not on the coordinate axes: The distance can be found by drawing perpendiculars from the points to the axes and then applying the Pythagoras theorem.

Thus we can say,

• Coordinates are a set of values that show an exact position. 
• On maps and graphs, it is common to have a pair of numbers to show where a point is; the first number shows the distance along and the second number shows the distance up or down.

Distance Formula

Let us now find the distance between any two points P(x₁, y₁) and Q(x₂, y₂).

Draw PR and QS perpendicular to the x-axis. A perpendicular is drawn from point P on QS to meet it at the point T.

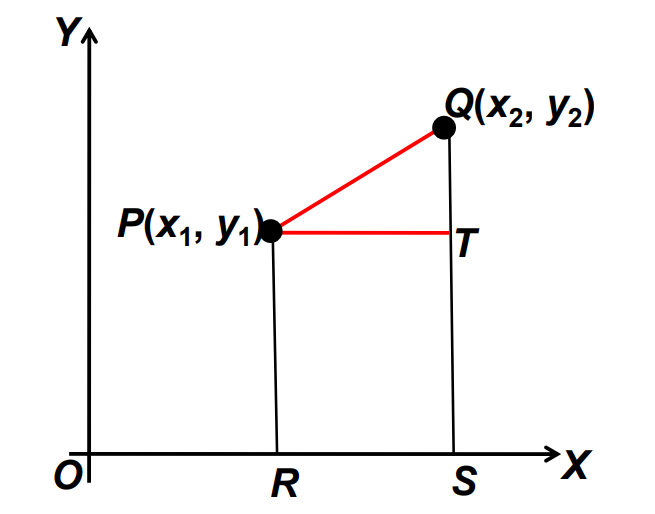
Then, (from the figure) 

OR = x₁ and OS = x₂ 

So, RS = PT = x₂ – x₁ …(1)

Also, SQ = y₂ ST = PR = y₁

So, TQ = y₂ – y₁ …(2)

Now, applying Pythagoras Theorem in ∆PTQ, we have

PQ² = PT² + TQ² 

Using (1) and (2), we get PQ² = (x₂ – x₁)² + (y₂ – y₁)²  

PQ = ± √(x₂−x₁)² + (y₂−y₁)²​

Since distance is always non-negative, we take only the positive value.So, the distance between any two points P(x₁,y₁) and Q(x₂,y₂) can be calculated as:

PQ = √(x₂−x₁)² + (y₂−y₁)²​

Example:

Find a point on the X-axis equidistant from A(2, -5) and B(-2, 9).

Solution: The coordinates of any point on the X-axis are of the form (x, 0). So, let P(x, 0) be the point which is equidistant from A(2, -5) and B(-2, 9). Then, PA = PB. 

⇒ √(x − 2)² + (0 + 5)²​ = √(x + 2)² + (0 – 9)²​

⇒  (x – 2)² + 25 = (x + 2)² + 81

⇒ x²  + 4 – 4x + 25 = x²  + 4 + 4x + 81

⇒ on solving, we get – 8x = 56

⇒ x = -7

Hence, the required point is (-7, 0). 

Section Formula

Consider any two points A(x₁, y₁) and B(x₂, y₂).

Assume that P(x, y) divides AB internally in the ratio m₁ : m₂, i.e.,

PA / PB = m₂ / m₁

Draw AR, PS, and BT perpendicular to the X-axis.  

Draw AQ and PC parallel to the X-axis.

Then, in ∆PAQ and ∆BPC,

∠PAQ = ∠BPC {corresponding Angles}

∠PQA = ∠BCP {each equal to 90⁰}

Therefore, ∆PAQ ∼ ∆BPC, {By AA similarity criterion}

Therefore, we have

PA / BP = AQ / PC = PQ / BC…..(1)

AQ = RS = OS = OR = x – x₁

PC = ST = OT – OS = x₂ – x 

PQ = PS – QS

      = PS – AR = y – y₁ 

BC = BT – CT 

      = BT – PS = y₂ – y

Putting these values in eq (1), we get 

m₁ / m₂ = x – x₁ / x₂ – x  = y – y₁ / y₂ – y

On solving for x and y, we get

x = m₁x + m₂x / m₁ + m₂ and y = m₁y +m₂y / m₁ + m₂

Thus, the coordinates of the point P(x, y) which divides the line segment joining the points A(x₁, y₁) and B(x₂, y₂), internally, in the ratio m₁ : m₂ are

(m₁x + m₂x / m₁ + m₂,  m₁y +m₂y / m₁ + m₂)

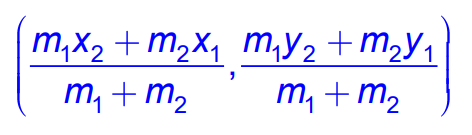
This is known as the Section Formula.

Example: 

Find the coordinates of the point that divides the line segment joining the points (6, 3) and (-4, 5) in the ratio 3: 2 internally.

Let P(x, y) be the required point.

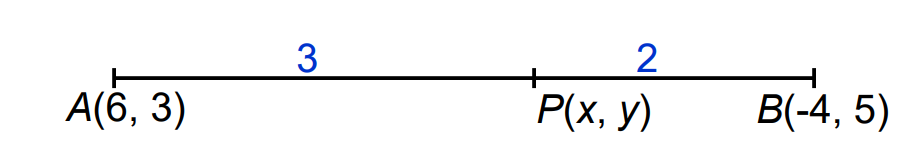
Then, on applying the section formula, we have

x = 3 x – 4 + 2 x 6 / 3 + 2 = 0 and 

Y = 3 x 5 + 2 x 3 / 3 + 2 = 21/5 

Hence, the required point is P(0, 21/5).

Let’s Conclude

In conclusion, Chapter 7 of CBSE Class 10th Math, Coordinate Geometry, is a vital segment that bridges the gap between algebra and geometry. Understanding this chapter equips students with essential skills for plotting points, calculating distances, and determining specific coordinates on the coordinate plane. By mastering concepts like the distance formula and the section formula, students can solve complex geometrical problems with greater ease and accuracy.

As we’ve explored in this blog post, the chapter on Coordinate Geometry provides a solid foundation for using coordinates to locate points and solve real-world problems. By practicing the exercises and examples given, you’ll gain proficiency in applying these concepts to various scenarios. Whether you’re preparing for exams or aiming to strengthen your mathematical understanding, the chapter on Coordinate Geometry is an indispensable part of your learning journey.

Remember, the key to excelling in Coordinate Geometry is practice and familiarity with the formulas and their applications. So, dive into the exercises and utilize resources effectively to master this chapter.