How Many Squares? – Complete Guide For Class 5 Math Chapter 3
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, How Many Squares? in Mathematics for Class 5th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
The chapter How Many Squares? Engages students in exploring the concepts of area and perimeter through hands-on activities. Students will learn to measure the side of a square and use it to create various shapes, such as rectangles, using a fixed number of squares. By counting the squares within shapes and understanding how different configurations can share the same area but have different perimeters, students develop a deeper understanding of these geometric properties. The chapter includes practical exercises like measuring the area of stamps, comparing areas of different objects, and tracing hands to calculate their areas. Through these activities, students will gain skills in estimating, measuring, and calculating areas and perimeters, making geometry both fun and educational.
How Many Squares?
Welcome to an engaging exploration of geometry with the chapter “How Many Squares?” This chapter takes students on a journey through the world of squares, rectangles, and various shapes, emphasizing hands-on activities and practical applications.
Understanding Perimeter and Area
Perimeter – The total length around the edges of a shape.
Area – The measurement of the space inside a shape.
In this chapter, through various activities, we will learn how to calculate the perimeter and area of different shapes.
Measuring the Side of a Square
Further in the chapter “How Many Squares?” We start by measuring the side of a square on a dotted sheet. This simple yet fundamental task lays the groundwork for understanding the properties of squares and other geometric shapes.
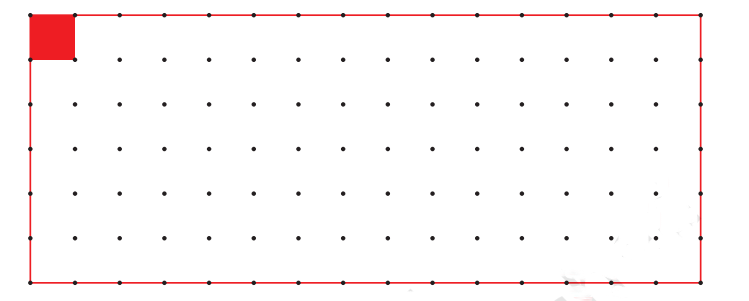
Creating Rectangles with Squares
An exciting activity involves using 12 squares to form as many rectangles as possible.

Each rectangle is made out of 12 equal squares, so all have the same area, but the length of the boundary will be different.
The length of the boundary is called a perimeter.
This activity teaches us that while each rectangle covers the same area, its perimeters can vary.
This exercise demonstrates the relationship between area and perimeter in a tangible way.
Measuring Stamps
One more activity from the chapter “How Many Squares?” is that by using interesting stamps, we can calculate the area covered by each stamp.
Look at these interesting stamps:

Place these stamps on the square grid and find their area and perimeter.
We can determine which stamp has the largest area, compare different stamps, and find the area differences between the largest and smallest stamps.
This activity integrates practical measurement skills with engaging visual aids.
Guessing and Estimating Areas
The chapter “How many Squares” states that sometimes we are challenged to estimate areas through everyday comparisons, such as determining
(a) Which has the bigger area — one of your footprints or the page of this book?
(b) Which has the smaller area — two five-rupee notes together or a hundred-rupee note?
(c) Look at a 10 rupee note. Is its area more than a hundred square cm?
(d) Is the area of the blue shape more than the area of the yellow shape? Why?

(e) Is the perimeter of the yellow shape more than the perimeter of the blue shape? Why?
These exercises help develop intuitive understanding and estimation skills.
How Big is My Hand?
Through this activity from the chapter How Many Squares, we trace our hands on a squared sheet and calculate the area, comparing the results with our friends.
- How will you decide whose hand is bigger — your hand or your friend’s hand?
- What is the area of your hand? _______ square cm.
- What is the area of your friend’s hand? _______ square cm.
This activity brings a personal touch to learning about the area and encourages collaborative discussion.
My Footprints

- Whose footprint is larger — yours or your friend’s?
- How will you decide? Discuss.
- Is the area of both your footprints the same?
Animal Footprints
As discussed in the chapter How Many squares, by exploring animal footprints of actual sizes, we can guess the areas and compare them to human footprints.
.
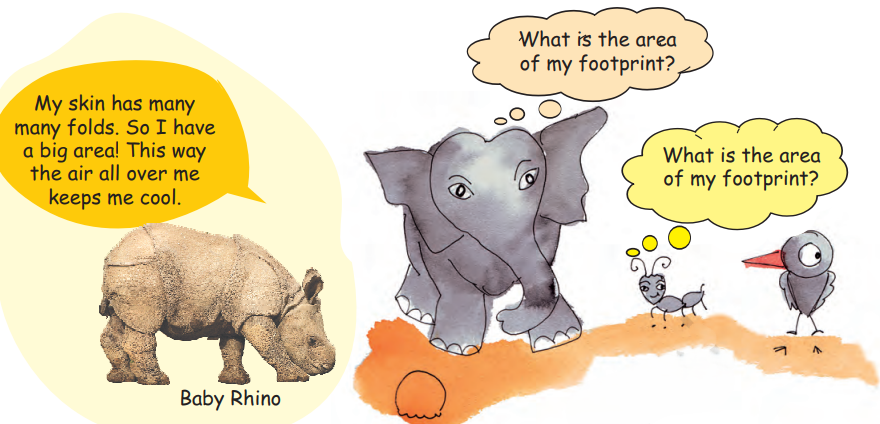
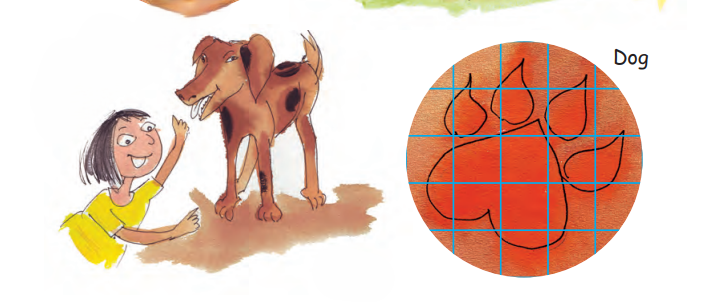
Here are some footprints of animals — in actual sizes. Guess the area of their footprints.
How to find the Area faster
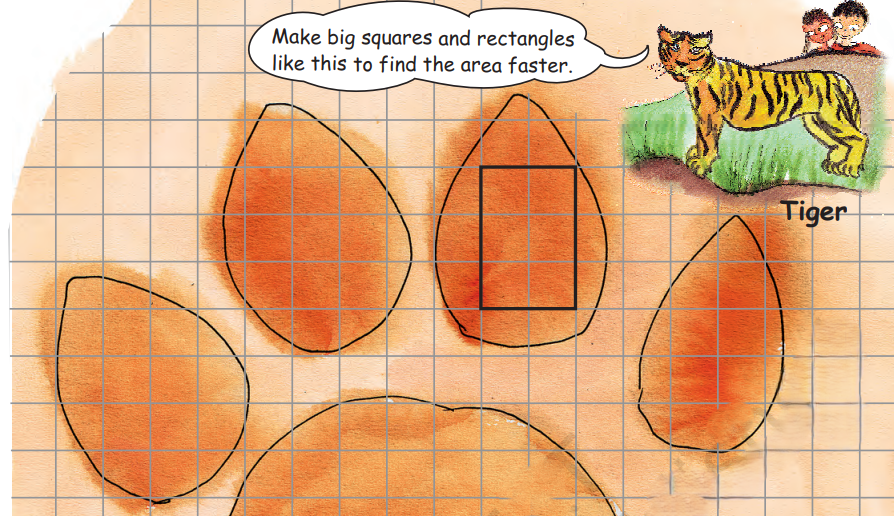
With this activity, we need not count each square.
They can identify the largest squares and rectangles within a footprint to know the area and then count small squares for irregular shapes.
Calculating Areas of Shapes

We can calculate the area of various shapes, including triangles and rectangles, using different methods.
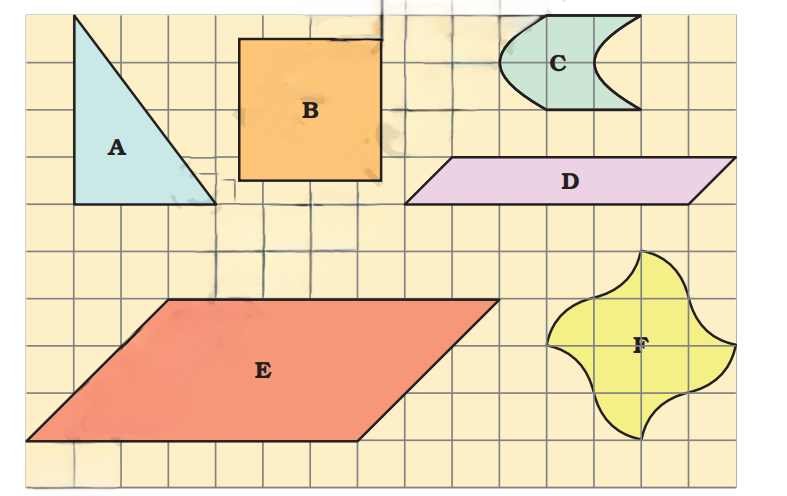
We learn to recognize geometric symmetry and develop strategies for finding areas.
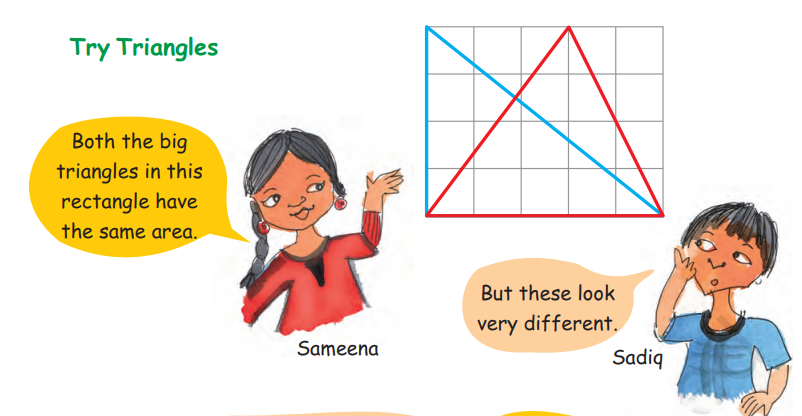
Triangles
Further in the chapter – How Many Squares, we learn the concept of Triangles.
Complete the Shape
Let’s understand this by an example
Suruchi drew two sides of a shape. She asked Asif to complete the shape with two more sides so that its area is 10 square cm.

He completed the shape like this.
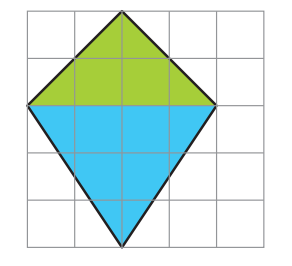
We can find out the area by completing it in different ways.
So, we can complete partially drawn shapes to reach a specified area.
This exercise encourages creativity and problem-solving as they explore multiple ways to achieve the same area.
So, we learned to calculate the area through many activities.
Steps to calculate the area by counting squares in a grid.
- Identify the Shape: Determine the shape whose area you need to calculate (e.g., a rectangle, triangle, irregular shape).
- Use a Grid: Place the shape on a grid where each square represents a unit area (e.g., 1 square centimeter).
- Count the Full Squares: Count all the fully occupied squares within the shape. Each full square counts as one unit of area.
- Estimate Partial Squares: For squares that are partially occupied by the shape, estimate their coverage. For simplicity, you can combine partially filled squares to make full squares. For example, if two half-squares are occupied, count them as one full square.
- Add Up the Squares: Add the number of full squares and the equivalent full squares made from partial squares to get the total area.
Puzzles with Five Squares
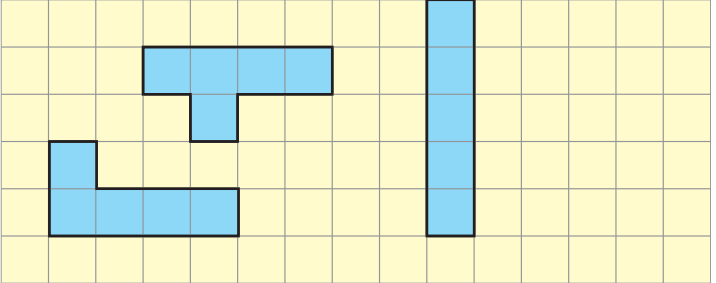
Using five squares, we can create different shapes and measure their perimeters and areas.
One more example of using 5 squares to create so many shapes
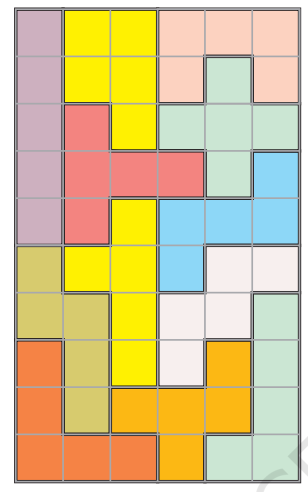
All 12 shapes are arranged here to make a rectangle. This is a 10 X 6 rectangle as there are 10 rows and 6 columns.
Here is a surprise that there are more than 2000 ways in which these shapes make a 10 X 6 rectangle.
This puzzle activity fosters spatial awareness and geometric reasoning.
Game Time
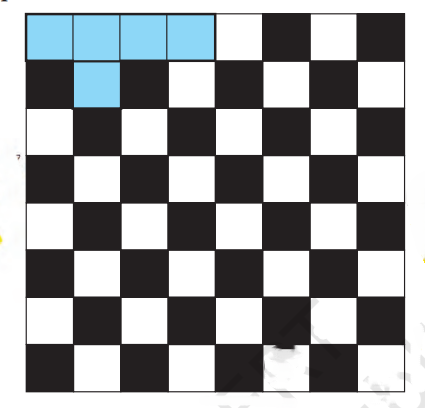
Playing a game with a chessboard and a set of 12 shapes, here we take turns placing shapes on the board.
The first player picks one shape from the set and puts it on the board covering any five squares.
The other player picks another shape and puts it on the board, but it must not overlap the first shape.
This game reinforces our understanding of area and perimeter while adding an element of fun and competition.
Make Your Own Tile
Look at some floor patterns


Steps to Create Own Tiles
Step 1: Take a piece of cardboard or thick paper. Draw a square of side 3 cm on it.
Step 2: Draw a triangle on any one of the sides of this square.
Step 3: Draw another triangle of the same size on another side of the square. But this time draw it inside the square.
Step 4: Cut this shape from the cardboard. Your tile is ready!

Make a pattern using your tile. Trace the shape to repeat it on a page, but remember there must be no gaps between them.
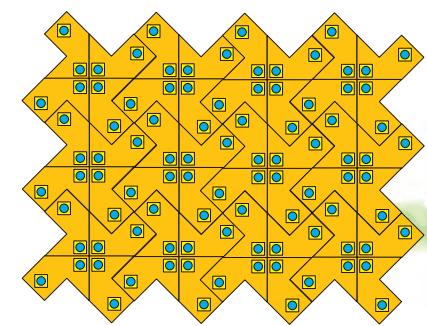
This hands-on activity nurtures their creativity and understanding of tiling patterns.
Practice Time
Finally, students apply their knowledge by making patterns using their tiles, ensuring no gaps between shapes.
This activity consolidates their learning and encourages them to experiment with new designs.
Example:

Now children can modify basic shapes to create different tiling shapes.
Through these activities, “How Many Squares?” transforms the abstract concepts of geometry into engaging, practical exercises.
By connecting mathematical ideas to real-world scenarios and hands-on projects, this chapter makes learning geometry both enjoyable and meaningful for students.
Let’s Conclude
In conclusion, CBSE Class 5th Math, Chapter 3 – How Many Squares? Offers an exciting and interactive way for students to understand the foundational concepts of area and perimeter. Through hands-on activities like creating shapes with squares, measuring stamps, and estimating the size of footprints and handprints, students gain a practical understanding of geometry. The chapter, How Many Squares? Encourages creativity, problem-solving, and collaboration, making the learning experience both fun and educational. By the end of How Many Squares? Students are well-equipped to tackle geometric challenges with confidence.
Practice questions on Chapter 3 - How Many Squares
Get your free Chapter 3 - How Many Squares practice quiz of 20+ questions & detailed solutions
Practice Now








