Parts and Wholes – Complete Guide For Class 5 Math Chapter 4
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Parts, and Wholes in Mathematics for Class 5th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
The chapter Parts and Wholes introduces students to the concept of fractions through engaging activities and practical examples. The chapter begins with drawing and dividing shapes, like rectangles, into equal parts to represent sections of a flag, helping students visualize fractions. It then explores real-world applications, such as sharing chocolate bars and analyzing flags from different countries to understand the proportions of various colors.
Creative activities, like making a magic top and coloring hats, further reinforce the idea of dividing items into equal parts. The chapter also delves into practical scenarios, such as managing a vegetable field, to illustrate how fractions are used in everyday life. Through stories and real-life applications, students learn to recognize and work with fractions, enhancing their understanding of parts and wholes in a fun and interactive manner.
Parts and Wholes
This chapter on parts and wholes focuses on grasping the concept of parts and wholes by utilizing fractions, coloring exercises, and engaging activities.
Let’s understand Parts and Wholes through some examples.
Example – Our Flag
Have you ever wondered how to draw the flag of our country India?
Here’s a simple way to do it:
- Draw a rectangle with a length of 9 cm and a width of 6 cm.
Fraction Concept: Understanding the whole (a rectangle) before dividing it.
- Divide this rectangle into three equal parts.
Fraction Concept: Dividing a whole into equal parts.
- Color the top one-third of the flag saffron (or orange).
- The middle one-third is white with the Ashoka Chakra in the center.
- The bottom one-third is green.
Fraction Concept: Identifying and working with fractions of a whole.

Exploring Other Flags
Let’s take a look at the flag of Myanmar, our neighboring country:
- Is blue more than one-fourth of the flag or less?
- Guess how much of the flag is red. Is it more than three-fourths?

Collect as many flags as you can. How many of them have three colors? Are the colored parts equal in these flags?
Here’s another flag, this time from the Math Club in a school in Kerala:
- What part of the flag is red?
- What part is green?

From these activities, we are understanding the whole (a rectangle) before dividing it. Then dividing a whole into equal parts and Identifying and working with fractions of a whole.
These activities are helping us to understand the concept of parts and wholes, which is fundamentally about understanding fractions.
Creative Activities
Going further in the chapter Parts and wholes, we’ll learn the concepts through some creative activities. These involve-
Magic Top

Let’s make a magic top:
- Take a piece of cardboard.
- Draw a circle with a radius of 3 cm and cut it out.
- Divide the circle into 8 equal parts.
- Color each part differently (red, orange, yellow, etc.).
- Push a matchstick through the center of the circle.
Spin it fast and observe the colors. Write down what you see in your notebook.
Addition and Subtraction
Addition and subtraction can also be used to better understand the concepts of parts and wholes. Here’s how.
Practice Time
A) Chocolate Bar Manju had a chocolate. She gave:
- One-fourth to Raji i.e 1/4
- One-third to Sugatha i.e. 1/3
- One-sixth to Sheela i.e. 1/6
She ate the remaining part.
How many pieces did each get? What part of the chocolate did Manju eat?
Let’s solve it
Let’s convert each fraction to have a denominator of 12:
- One-fourth (1/4) to a fraction with denominator 12: 1 x 3/4 x 3 = 3/12
- One-third (1/3) to a fraction with denominator 12: 1 x 4/3 x 4 = 4/12
- One-sixth (1/6) to a fraction with denominator 12: 1 x 2/6 x 2 = 2/12
Now, we add these fractions: 3/12 + 4/12 + 2/12 = 9/12 = 3/4
So, Manju gave away 3/4 of the chocolate.
Now, we subtract this from the whole chocolate to find out how much she had left: 1 – 3/4 = 4/4 – 3/4 = 1/4
Therefore, Manju had 1/4 of the chocolate left.
There are many more examples of Addition and Subtraction
B) Coloring Hats
Coloring can be a great way to visualize fractions. For example, if you color three-fifths of a set of hats blue and the rest red, you can easily see the parts that are colored and those that remain uncolored. As you can see
- Color three-fifths of the hats blue.
- How many hats did you color red?
- How many hats did you color blue?
- What part of the hats are not colored?

Understand Equal Fractions through these examples
A) Equal Parts of a Triangle
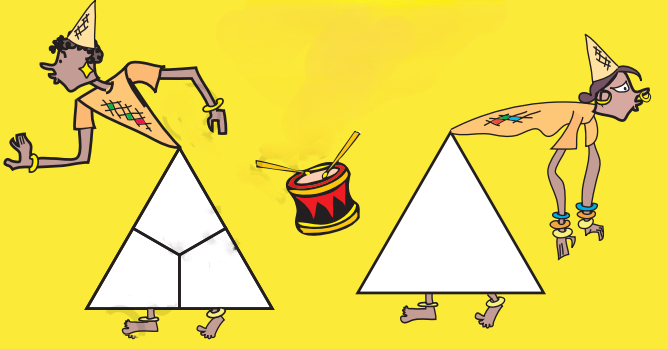
- The white triangle is divided into three equal parts. Fill each one-third part with a different color.
- Try making three equal parts of the triangle differently with one-third with a different color.
B) Six Parts of a Rectangle
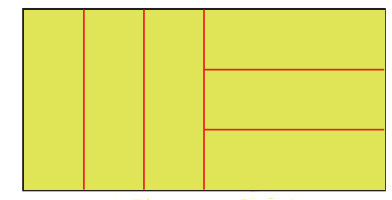
- Rani divided a green rectangle into six equal parts.
- Now you divide each of these rectangles into six equal parts in a different way.
- Discuss how to check that each part is one-sithe rectangle.
- Can you say that one-sixth of the green rectangle is bigger than one-sixth of the blue rectangle?
Greedy Gatekeepers – A story
Now in the chapter PArts and Wholes, let’s take a break from shapes and talk about a story involving Birbal, a clever minister in King Akbar’s court. When faced with greedy gatekeepers demanding fractions of his prize, Birbal’s wit shines through. This story illustrates the practical application of fractions in a fun narrative.
Story – Remember Birbal, the clever minister of King Akbar? Here’s a story about how he became a minister:
Birbal, a young poet, set off for the city to show his poems to King Akbar. At the palace gates, a gatekeeper stopped him and demanded a share of any prize he might receive. Birbal agreed and entered. At the next gate, another gatekeeper made the same demand. Birbal agreed again.
Both gatekeepers thought they would get a share of any gold coins Birbal received. If Birbal received 100 gold coins, the first gatekeeper would get half, and the second would get one-fourth of what remained.
Through this story, we can learn what part each one received.
Patterns in Parts
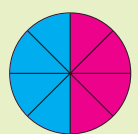
Let’s go further in the chapter Parts and Wholes and understand the patterns in Parts. Look at grid A – Is it colored: a) blue, white b) blue, white c) blue, white d) blue, white?
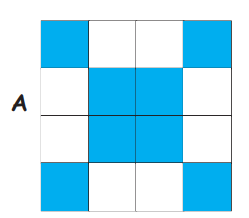
We get 8/1.6 blue and 8/16 white
We can draw grids of 16 squares and make patterns with:
- 2/8 Red, 1/2 yellow, 1/4 green
- 3/16 Blue, 5/16 red, 1/2 yellow
Ramu’s Vegetable Field
Now we’ll further learn the concepts of Parts and Wholes with the example of Ramu’s Vegetable Field. Ramu’s vegetable field is divided into nine equal parts. What vegetables does he grow, and how much of each? This exercise can lead to questions about distribution and fractions in agriculture.

Ramu’s vegetable field has 9 equal parts:
- Which vegetable grows in the biggest part of his field? What part?
- What part of the field is used to grow potatoes?
- What part is used for spinach? For brinjals?
Ramu gave some vegetables to his friends:
Aboobacker got one-fifth of the tomatoes and one-fourth of the potatoes.
Tomatoes: One-fifth of 1/3 : 1/5 x 1/3 = 1/15
Potatoes: One-fourth of 1/4: 1/4 x 2/9 = 2/36 = 1/18
Srija got one-third of the tomatoes and one-sixth of the potatoes
Tomatoes: One-third of 1/3 : 1/3 x 1/3 = 1/9
Potatoes: One-sixth of 2/9: 1/6 x 2/9 = 2/54 = 1/27.
Nancy got the rest.
Tomatoes: Total part given to Aboobacker and Srija: 1/15 + 1/9 = 3/45 + 5/45 = 8/45
Remaining part: 1/3 − 8/45 = 15/45 − 8/45 = 7/45
Potatoes: Total part given to Aboobacker and Srija: 1/18 + 1/27 = 3/54 + 2/54 = 5/54
Remaining part: 2/9 − 5/54 = 12/54 − 5/54 = 7/54
Fun with Fractions (Comparison of Fractions)
Games like “Who Colors the Circle First?” from the chapter “Parts and Wholes” can make learning fractions exciting. Players take turns coloring parts of a circle based on drawn tokens, reinforcing the concept of fractions through play.
This game is to be played in groups of 4. Each player makes a circle and 15 tokens on slips of paper, writing fractions like:
1/2, 1/3, 1/4, 1/6, 1/12, 2/12, 3/12, …… etc
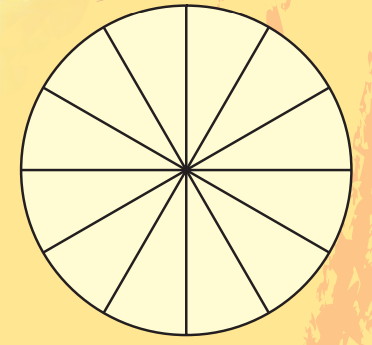
Shuffle the tokens and place them in the middle. Each player takes a token, colors that part of the circle, and puts the token back. The winner is the one who colors the circle completely first.
Guess and Check – Completing Shapes
Now to further understand the concepts of Parts And Wholes let’s play the game of completing shapes.
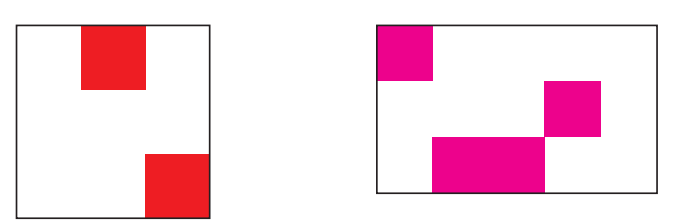
Colored Parts

1/2 = 2/4 = 3/6 = 4/8
Parts of the Strip (1/2 and 1/4)
How many one-fourths will make a half?
How many 1/8 will make 1/4?
How many 1/8 are in 1/2?
Patterns
There are various patterns you can observe in the outcomes of this activity of Parts and Wholes.

Puzzle: Is it Equal?
Ammini says half of half and one-third of three-quarters are equal. Do you agree? How will you show this?
The use of concrete things (such as matchsticks, bottle caps, etc.) will help children make sense of equivalent fractions such as 1/2 = 2/4 = 3/6 =5/10. Children must make their fraction PS using papers of different sizes. Encourage them to compare the strips by coloring them into different fractions.
From a Part to the Whole
Now further in the chapter PArts and Wholes, let’s understand the concept of Part to the whole. Let’s use some examples for the same
Examples:
- This shows the petals of a flower.
Complete the flower by drawing the other petals.

- The picture shows one-third of the blades of a fan. Complete the picture by drawing the other blades.

- Half of the blades of another fan are shown here. Complete the picture by drawing the other half. How many blades have you drawn?

Understanding fractions is not just about numbers; it’s about applying them in real life. For example, how many coins make a rupee? What part of a day do we spend sleeping, studying, or playing? These questions encourage students to relate math to their daily lives.
Rupees and Paise
Rupees and Paise are one of the best ways to understand the concepts of parts and wholes.

- How many 50 paise coins make one rupee?
- Is 50 paise half of one rupee?
- How many 25 paise coins make one rupee?
- How many 20 paise coins make one rupee?
- How many 10 paise coins make one rupee?
Story- An Old Woman’s Will (Addition and subtraction of fractions from the Whole Numbers)

An old woman had 19 camels and three daughters. Her will stated:
- The eldest daughter gets half (1/2) of the camels.
- The second daughter gets one-fourth (1/4) of the camels.
- The third daughter gets one-fifth (1/5) of the camels.
The daughters were puzzled. Their aunt solved the problem by lending them her camel, making 20 camels. The eldest daughter took 10 camels, the second took 5 camels, and the third took 4 camels. The aunt then took back her camel.
Arun’s Timetable
Use different colors to show Arun’s timetable.
Arun’s daily schedule illustrates how to break down a day into parts, showing how much time is spent sleeping, studying, and playing. This helps students understand the practical applications of fractions in daily life.
Keerti’s Shopping List
Keerti’s shopping list introduces real-world math, where students calculate costs based on weight and price per kilogram, reinforcing their understanding of fractions in financial contexts.

The chapter “Parts and Wholes” is a rich tapestry of activities, stories, and practical applications that make learning about fractions engaging and relevant. By participating in these exercises, students not only grasp the concept of fractions but also see their importance in everyday life.
Conclusion
In conclusion, CBSE Class 5th Math, Chapter 4 – Parts and Wholes is a fascinating journey into the world of fractions. Through a mix of engaging activities, real-world examples, and interactive stories, this chapter helps students visualize and understand the concept of dividing objects and quantities into equal parts. By the end of Parts and Wholes, students will be able to confidently work with fractions and see how they apply to everyday scenarios, from flags and chocolate bars to vegetable fields and shopping lists. Whether it’s solving puzzles or analyzing patterns, Parts, and Wholes encourages critical thinking and makes learning math a fun and hands-on experience!
Practice questions on Chapter 4 - Parts And Wholes
Get your free Chapter 4 - Parts And Wholes practice quiz of 20+ questions & detailed solutions
Practice Now








