Complete Guide For Class 10 Math Chapter 11 – Areas Related to Circles

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Areas Related to Circle in Mathematics for Class 10th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Areas Related to Circles explores the concepts related to the measurement of areas in circular figures. Students learn to calculate the area of a circle using the formula πr², where r is the radius. The chapter also covers the concepts of the circumference of a circle, sectors, and segments. Key formulas like the area of a sector (θ/360 × πr²) and the area of a segment are introduced. These concepts are crucial for solving problems related to circular regions, including real-life applications like finding the area of land, designing circular objects, and more. Mastery of these topics enhances students’ ability to handle complex geometrical problems involving circles and their parts.

Areas Related to Circles

A circle is a basic shape in Euclidean geometry consisting of points on a plane that are equidistant from a fixed point (the center). The distance from the center to any point on the circle is called the radius.

Radius and Circumference of a Circle

The circumference of a circle is the distance covered by traveling once around the circle. It is the perimeter of the circle, and this distance bears a constant ratio with the diameter of the circle. This constant ratio is denoted by π (pi), which approximately equals 22/7 or 3.14.

The formula for the circumference of a circle is given by: Circumference = 2πr,  where r is the radius of the circle.

Area of a Circle

To understand the area of a circle, imagine cutting a circular object (like a sheet) into several sectors and rearranging them. This arrangement forms a shape that resembles a rectangle. The area of the circle is equal to the area of this rectangle.

The formula for the area of a circle is Area = πr²,  where r is the radius of the circle.

Sector and Segment of a Circle

• A sector of a circle is the portion enclosed by two radii and the corresponding arc. 
• A segment of a circle is the portion enclosed between a chord and the corresponding arc.

Related Terms For Areas Related To Circles

• Minor Sector: The smaller part of the circle when it is divided by a chord.
• Major Sector: The larger part of the circle when it is divided by a chord.
• Minor Segment: The area enclosed by the chord and the minor arc.
• Major Segment: The area enclosed by the chord and the major arc.

When we refer to a “segment” or “sector,” we usually mean the “minor segment” or “minor sector.”

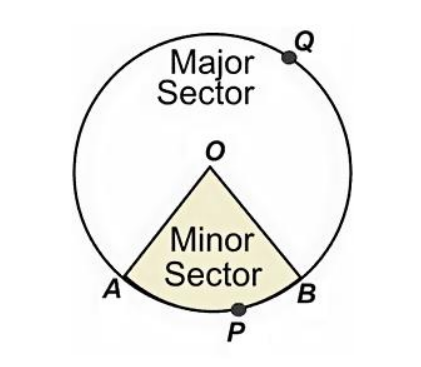
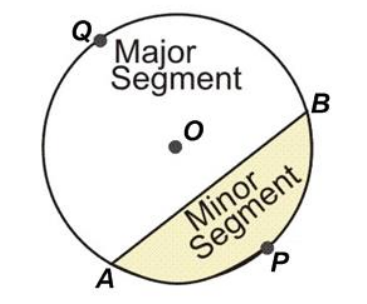
Area of a Sector

The area of a sector can be calculated using the formula: Area of Sector = θ / 360 × πr², where θ is the angle subtended by the sector at the center of the circle.

When the degree measure of the angle at the center is 360, 

area of the sector = πr²

So, when the degree measure of the angle at the center is 1,

area of the sector = πr²/360

Therefore, when the degree measure of the angle is θ

area of the sector = πr²/360 x θ

                                     = θ / 360 x πr²

Thus, the area of the sector of angle θ = θ / 360 x πr²

Length of an Arc

The length of an arc of a sector with angle θ can be calculated using: Length of Arc = θ /360 × 2πr

Example:

Find the length of the arc of a circle of radius 21 cm, if the angle subtended by the arc at the center is 60°.

Length of the arc = 2πr x θ/ 360 = 2 x 22 x 21 x 60 / 7 x 360 = 22 cm

Area of Segments of a Circle

The area of a segment can be calculated as Area of Segment = Area of Sector − Area of Triangle

Area of segment APB = Area of the sector OAPB – Area of ∆OAB

                                         = πr²θ/ 360 – Area of ∆OAB

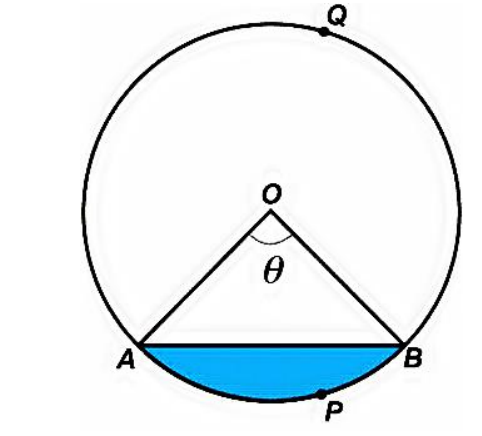
Area of major sector OAQB = πr² – Area of minor sector OAPB

Area of major sector AQB = πr² – Area of minor sector APB

Example: Given a chord of a circle with a radius of 15 cm that subtends an angle of 60° at the center, find areas of corresponding minor sectors and segments of a circle.

Area of the sector = πr²θ/ 360

Area of the segment = πr²θ/ 360 – Area of ∆OAB

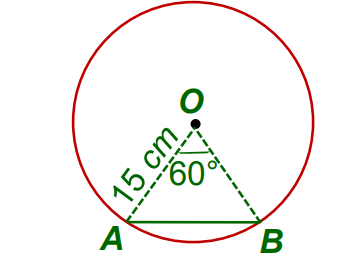
In ∆OAB, ∠AOB = 60⁰ (given)

OA = OB (Raddi of the same circle)

⇒ ∠OAB = ∠OBA = 180⁰- 60⁰ /2 = 60⁰ each

⇒ ∆OAB is an equiangular.

⇒ ∆OAB is an equilateral triangle.

We have, θ = 60⁰

Hence, are of the sector = π(15²) 60 / 360 = π(15²)/6 = 117.86 cm²

Given ∆OAB is an equilateral triangle whose side is equal to the radius of the circle.

So, area of the segment =  π(15²) 60 / 360 – √3 /4  (15²)

                                      =  (15²) (π/6 – √3 /4) = 20.42 cm²

Let’s Conclude

In conclusion, CBSE Class 10th Math, Chapter 11 – Areas Related to Circles, provides a thorough understanding of key concepts related to circular figures. From calculating the area of a circle using πr² to understanding sectors, segments, and arcs, this chapter equips students with essential geometry skills. Mastering these concepts is not only crucial for academic success but also for solving real-world problems involving circular shapes. With iPrep’s comprehensive learning resources, including animated videos, practice questions, and detailed notes, students can confidently excel in Areas Related to Circles. Explore our engaging content to enhance your learning and solidify your understanding of Areas Related to Circles.