Complete Guide For Class 10 Math Chapter 5 – Arithmetic Progressions

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Arithmetic Progressions in Mathematics for Class 10th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Arithmetic Progression (AP) introduces students to sequences where the difference between consecutive terms is constant. This difference, known as the common difference, is key to understanding and analyzing these sequences. Students learn to identify and construct APs and calculate the nth term using the formula aₙ= a+(n−1)d, where aaa is the first term and ddd is a common difference. The chapter also covers the sum of the first nnn terms of an AP, a critical concept for solving problems involving series. Mastery of these concepts enables students to solve various mathematical and real-life problems involving linear sequences, enhancing their analytical skills.

Arithmetic Progression

In our daily lives, we often encounter number patterns, which play a significant role in mathematics. This chapter delves into the concept of arithmetic progressions (AP), where numbers follow a specific pattern, making them predictable and useful in various mathematical applications.

Understanding Patterns in Arithmetic Progression

1. Basic Number Patterns:
   • Consider the pattern formed by the number of squares:
     • 1st row: 1 square
     • 2nd row: 2 squares
     • 3rd row: 3 squares, and so on.
   • This pattern is represented as 1, 2, 3, 4, 5, …

2. Ladder Rung Lengths:
   • Observe the decreasing lengths of ladder rungs:
     • 1st rung: 30 cm
     • 2nd rung: 28 cm
     • 3rd rung: 26 cm, and so on.
   • This pattern is 30, 28, 26, 24, 22, 20, …

3. Square Area Patterns:
   • If a unit square represents 1 square unit, then:
     • The pattern of areas can be 1², 2², 3², 4², which translates to 1, 4, 9, 16, …

Each of these patterns illustrates how arithmetic progressions can be identified and utilized.

Definition of Arithmetic Progression

An arithmetic progression (AP) is a sequence of numbers in which each term after the first is obtained by adding a constant value, known as the common difference, to the preceding term. The terms are typically denoted by a₁​, a₂​, a₃, …, aₙ.

Key Points:

• The successive terms are usually denoted by a₁​, a₂​, a₃, …, aₙ.
• Such a number pattern is said to form an arithmetic progression (AP)

Terms

Let’s understand the Terms

• Let a₁​, a₂​, a₃, …, aₙ be an AP.
• The numbers occurring in an arithmetic progression are called terms.
• Here, a₁​, a₂​, a₃, …, aₙ are the first, second, … and nth terms respectively.

Key Points:

• The first term is denoted by ‘a’ 
• The common difference by ‘d’
• Then, the standard form of an arithmetic progression would be a, a + d, a + 2d, a + 3d, …
• Thus, each succeeding term is obtained by adding d to the preceding term

Classification of AP based on the number of Terms 

• Finite AP

Examples: 

• 13, 9, 5, 1, -3, -7, …, -31
• -5, -2, 1, 4, 7, 10, …, 88

• Infinite AP

Examples: 

• 13, 9, 5, 1, -3, -7,…
• -5, -2, 1, 4, 7, 10,…

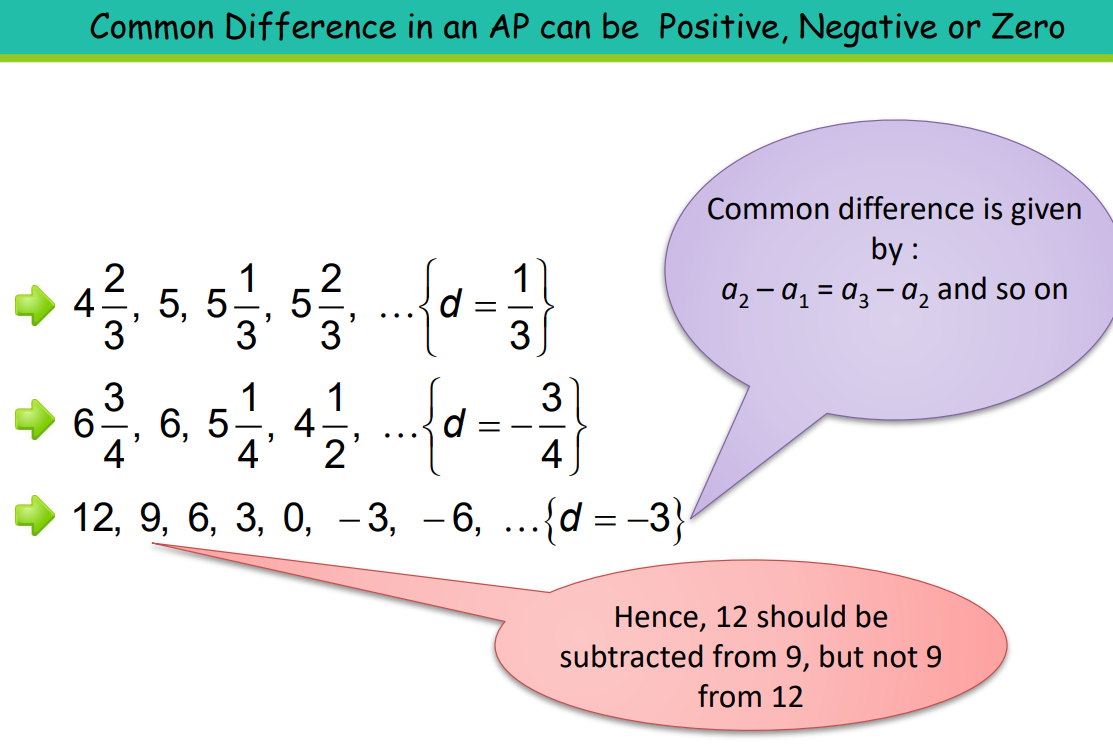
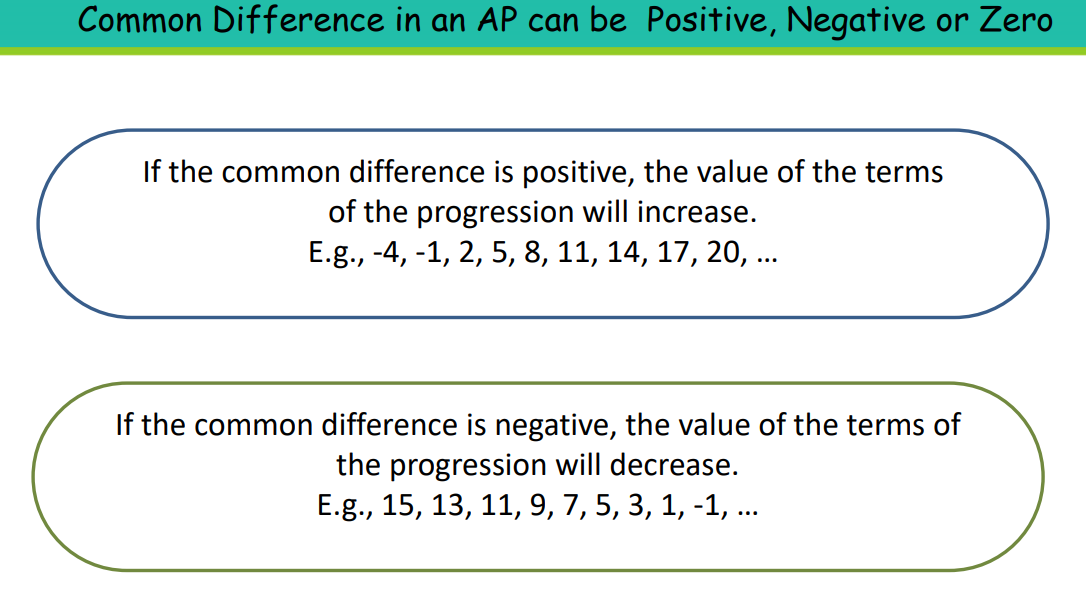
Common Differences in Arithmetic Progression

The common difference, denoted by ddd, is the fixed number that is added to each term to get the next term in the sequence. It can be positive, negative, or zero. The formula for the common difference is:

                     d = a₂−a₁= a₃−a₂ =………= aₙ−aₙ₋₁​

Examples of Arithmetic Progression

• Positive common difference: 1,3,5,7,…
• Negative common difference: 12,9,6,3,0,−3,…
• Zero common difference: 4,4,4,4,…

General Term or nth Term of an Arithmetic Progression

The nth term of an AP can be determined using the formula:

                      aₙ= a+(n−1)d

Where:

• a is the first term,
• d is the common difference,
• n is the term number.
• aₙ is also called the general term of the AP.

For instance, if the first term is 500 and the common difference is 100, the 15th term can be calculated as:

𝑎₁₅ = 500 + (15−1) × 100 = 500 + 1400 = 1900

The sum of the First n Terms of an Arithmetic Progression

The sum 𝑆ₙ of the first n terms of an AP is given by:

                          𝑆ₙ = 𝑛[2a+(n−1)d]/2

Alternatively, if the nth term (or the last term) is known, the sum can be calculated using:

                                     𝑆ₙ = 𝑛(a+𝑙)/2

Where 𝑙 is the last term of the AP.

Let’s understand it this way.

Terms of an AP are given by : 

a, a + d, a + 2d, a + 3d, …a + (n – 1)d.

Let Sn denote the sum of the first n terms of the AP.

We can write, Sn = a + (a + d) + … + {a + (n – 2)d} + {a + (n – 1)d} …(1).

On writing the terms in reverse order, we get Sn = {a + (n – 1)d} + {a + (n – 2)d} +… + (a + d) + a …(2).

On adding the corresponding terms of equation(i) and equation (2),

 we get 2Sn = {2a + (n – 1)d} + {2a + (n – 1)d} + …+ {2a + (n – 1)d}

                       = {2a + (n – 1)d} x n 

            So,  𝑆ₙ = 𝑛[2a+(n−1)d]/2

Hence this denotes the general formula for the sum of the first n terms of the AP. 

It can also be written as: 

       𝑆ₙ = 𝑛[a+a+(n−1)d]/2   

      𝑆ₙ = 𝑛 [a+aₙ]/2 (Therefore aₙ = (n−1)d/2)

Sometimes, the nth term is named as the last term and is denoted by the letter ‘l’. So,  𝑆ₙ = 𝑛(a+𝑙)/2

Example:

If you want to find out how much money will be collected by Nishi’s daughter by her 18th birthday, given that the first term is Rs. 500 and the common difference is Rs. 100, the total sum would be:

𝑆₁₈ = [2×500+(18−1)×100]=9×[1000+1700]=Rs.24,300

Let’s Conclude

In conclusion, Chapter 5 – Arithmetic Progressions for CBSE Class 10th Math offers a comprehensive understanding of sequences with a constant difference between terms. By mastering the concepts of the common difference, general term, and the sum of terms, students can effectively tackle problems involving arithmetic progressions. Our resources at iPrep are designed to support your learning journey through engaging animated videos, practice questions, and clear notes. This chapter not only enhances your mathematical skills but also prepares you for practical applications of sequences in various contexts. Embrace the power of arithmetic progressions, and let iPrep guide you toward academic success with clarity and confidence.