Surface Areas and Volumes – Complete Guide For Class 10 Math Chapter 12

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Surface Areas and Volumes in Mathematics for Class 10th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Surface Areas and Volumes focuses on calculating the surface area and volume of various three-dimensional shapes, such as cubes, cuboids, cylinders, cones, spheres, and hemispheres. Students learn to apply formulas for the lateral and total surface areas, as well as the volumes of these shapes. The chapter also explores problems involving the combination of different shapes and real-life applications, such as finding the capacity of containers or the amount of material required for construction. Mastery of these concepts enhances students’ ability to solve practical problems related to geometry, fostering a deeper understanding of spatial relationships.

Surface Areas and Volumes

Consider a cylindrical container that is hemispherical from the inside, or a frustum, which is the portion of a cone where the top has been removed. These are examples of how basic shapes can be modified or combined to create new forms.

Combinations of Solids

Surface Area of Combinations of Solids

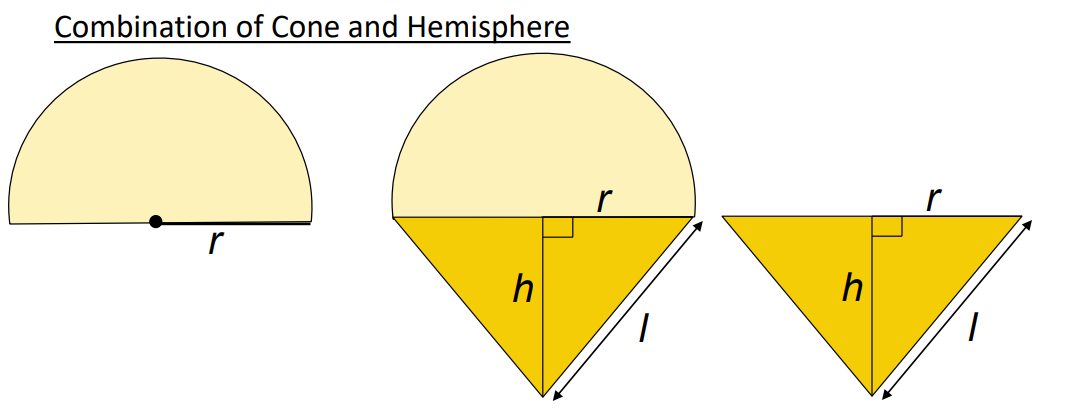
When dealing with a combination of solids like a cone and a hemisphere, the total surface area can be calculated by summing the surface areas of the individual shapes.

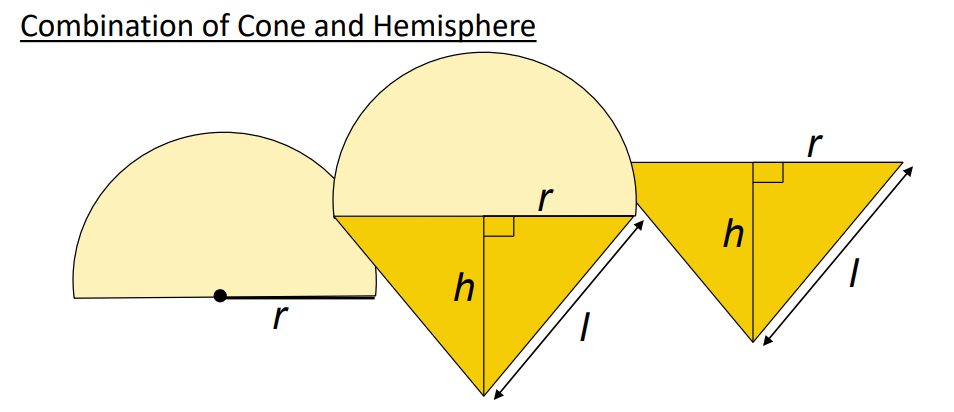
The curved surface area of the hemisphere = 2πr²

The curved surface area of the cone = πrl

The total surface area of the new solid = Curved surface area of the hemisphere + Curved surface area of the cone

                                              = 2πr² + πrl

Example: 

From a solid circular cylinder with a height of 10 cm and a radius of base of 6 cm, a right circular cone of the same height and same base, as that of the cylinder has been removed. Find the whole surface area of the new solid remained.

Way of Calculation

• The curved surface area of the hemisphere: 2πr²
• The curved surface area of the cone: πrl
• Total surface area: 2πr²+ πrl

Solution: 

Let r be the base radius, l the slant height, and h the height of the cone. 

So, the slant height of the cone (l)  = √ r² + h²

                                       = √ 6² + 10² = √ 36 +100 = 2 √ 34 cm

Now,  Whole surface area = 2πr²+ πr²+ πrl = πr (2h + r+ l)

                                              = 3.14 x 6 (2 x 10 + 6 + 2 √ 34)

                                              = 18.84 (20 + 6 + 2 √ 34)

                                              = 18.84 (26 + 2 √ 34)

                                              =  489.84 +37.68 √ 34 = 709.51 cm²

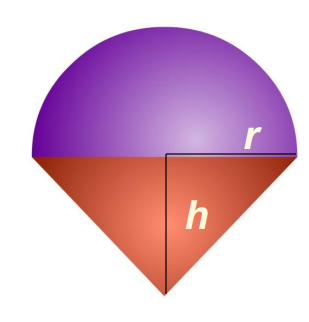
Volume of Combinations of Solids

When combining shapes, the total volume is the sum of the volumes of the individual components.

Volume of the hemisphere = 2/3 πr³

Volume of the cone = (1/3)πr²h

Total volume of the new solid = Volume of the hemisphere + Volume of the cone

                                              = 2/3 πr³ + (1/3)πr²h

Volume of the container shown = Volume of the cylinder + Volume of both hemisphere

The volume of the cylinder = πr²h

Volume of the hemisphere = 2 x (2/3)πr³

                                                    =   πr²h + 2/3 πr³ + (2/3)πr³

                                                    = πr²h + 4/3 πr³

Example:  A solid toy is in the form of a hemisphere surmounted on a right circular cone. The height of the cone is 4 cm and the diameter of its base is 6 cm. Determine the volume of the toy. 

Way of Calculation

• The volume of the hemisphere: 2/3πr³
• Volume of the cone: 1/3πr²h
• Total volume: 2/3πr³+  1/3πr²h

Solution: Height of the cone (h) = 4 cm

The radius of the base of the cone (r)= 3 cm

Also, the radius of the hemisphere (r)= 3 cm

Now, Volume of the solid toy = Volume of the hemispherical part + volume of the conical part

So, the volume of the solid toy: 2/3πr³+  1/3πr²h

                                      = 1/3 πr² (2r + h)

                                     = 1/3  x 3.14 x (3)² x (2 x 3 +4) cm³ = 94.2 cm³

Let’s Conclude

In conclusion, mastering the concepts in CBSE Class 10th Math, Chapter 12 – Surface Areas and Volumes is essential for solving real-world problems involving three-dimensional shapes. This chapter not only equips you with the knowledge to calculate surface areas and volumes of simple solids like cubes, cones, and cylinders but also teaches you how to handle combinations of these solids in practical situations. By thoroughly understanding Surface Areas and Volumes, you’ll be able to apply these formulas in various contexts, such as construction, container design, and more. With iPrep’s engaging videos, practice exercises, and notes, grasping these concepts will become a seamless and enjoyable experience. Dive deep into Surface Areas and Volumes and excel in your exams with confidence!