Polynomials – Complete Guide For Class 10 Math Chapter 2

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Polynomials in Mathematics for Class 10th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter on Polynomials delves into the essential concepts of polynomial functions, providing a thorough understanding of their structure and properties. Students explore different types of polynomials, including linear, quadratic, and cubic polynomials, each characterized by their degree and the number of roots it possesses. Key topics include polynomial operations such as addition, subtraction, multiplication, and division, as well as factorization techniques like the division algorithm and synthetic division.

The chapter also examines the geometric interpretation of polynomial roots through their graphical representations, including the behavior of polynomial functions and the relationship between their roots and coefficients. Mastery of these concepts not only forms the foundation for solving polynomial equations but also enhances students’ problem-solving skills, preparing them for more advanced mathematical challenges and real-world applications.

Introduction to Polynomials

Polynomials are fundamental algebraic expressions that play a crucial role in various branches of mathematics, including algebra, calculus, and engineering. A polynomial in one variable x is an expression of the form p(x) = aₙ xⁿ + aₙ₋₁ xⁿ⁻¹ + ⋯ + a₁ xⁿ + a₀, where aₙ, aₙ₋₁,….,a₀ are constants known as coefficients, and n is a non-negative integer representing the degree of the polynomial. The degree of a polynomial indicates the highest power of the variable x and determines the polynomial’s general shape and behavior. Polynomials can be classified based on their degree: a linear polynomial has a degree of 1, a quadratic polynomial has a degree of 2, and a cubic polynomial has a degree of 3. 

Graphs of Polynomial Functions

The graph of a polynomial f(x) is the collection of all points (x, y) on a coordinate plane where y = f(x). We will see the graphs of linear, quadratic, and cubic functions.

• Linear Functions: The simplest polynomial function is linear, represented as 

f(x)=ax+b. Its graph is a straight line. For instance, the polynomial 5x−3 has a straight-line graph that intersects the x-axis at one point.

• Quadratic Functions: A quadratic polynomial is of the form ax² + bx + c, where a ≠ 0.  Its graph is a parabola, which can open upwards or downwards depending on the sign of a. For example, the polynomial x²− 5x + 4 represents a parabola that intersects the x-axis at two distinct points, x = 1 and x = 4.

• Cubic Functions: Cubic polynomials, such as x³ −3x² − x + 3, have graphs that can exhibit more complex behavior, including multiple peaks and troughs. These graphs can intersect the x-axis at up to three points, depending on the nature of the polynomial.

Geometrical meaning of  Zeroes of Polynomials

The zeroes or roots of a polynomial function are the x-values where the graph intersects the x-axis. Let’s delve into the geometric meaning of zeroes for linear, quadratic, and cubic polynomials:

• Linear Polynomials: For a linear polynomial ax+b, there is exactly one zero. For example, the polynomial 5x − 3 has a zero at x = 3/5, which is where the line intersects the x-axis.

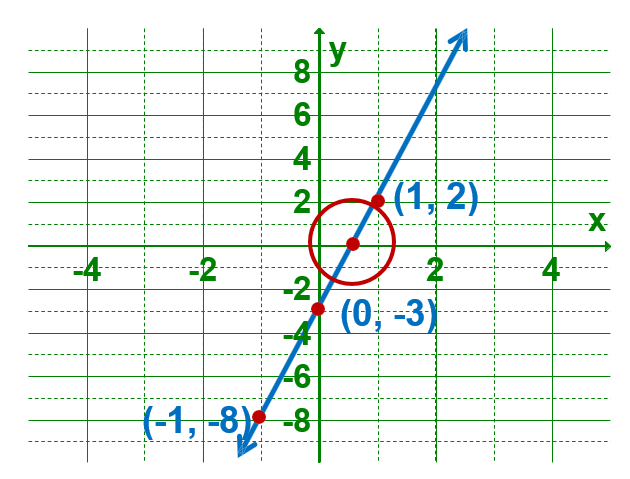
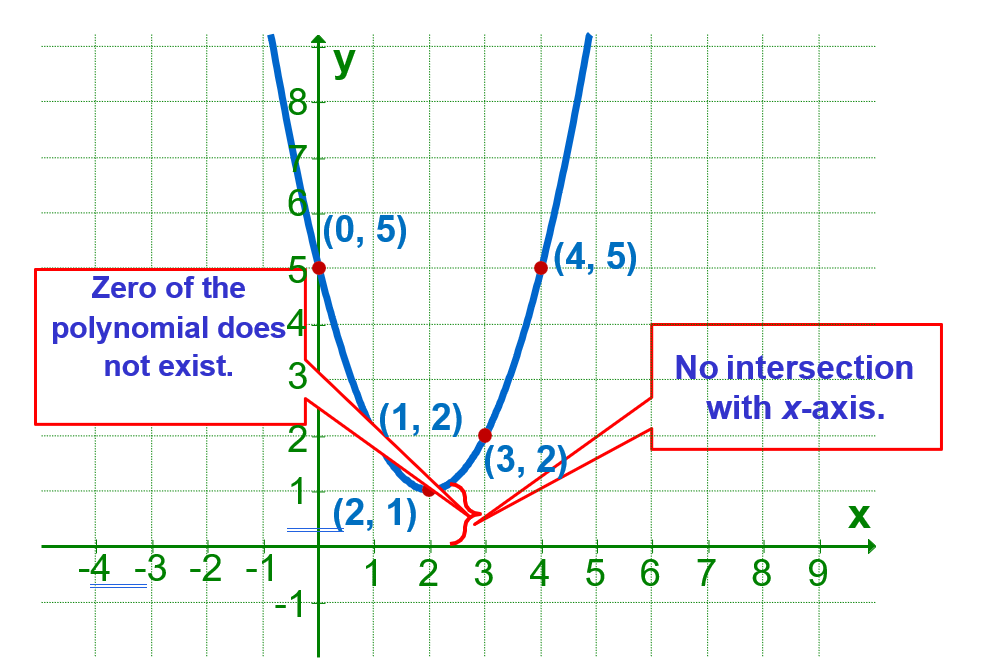
• Quadratic Polynomials: A quadratic polynomial ax²+ bx + c can have zero, one, or two zeroes:

1.  Two Distinct Zeroes: If the parabola intersects the x-axis at two points, such as x = 1 and x = 4 for x²− 5x + 4.
2. One Repeated Zero: If the parabola touches the x-axis at exactly one point, like x = 3 for x²− 6x + 9.
3. No Zeroes: If the parabola does not intersect the x-axis, as seen in x²− 4x + 5.

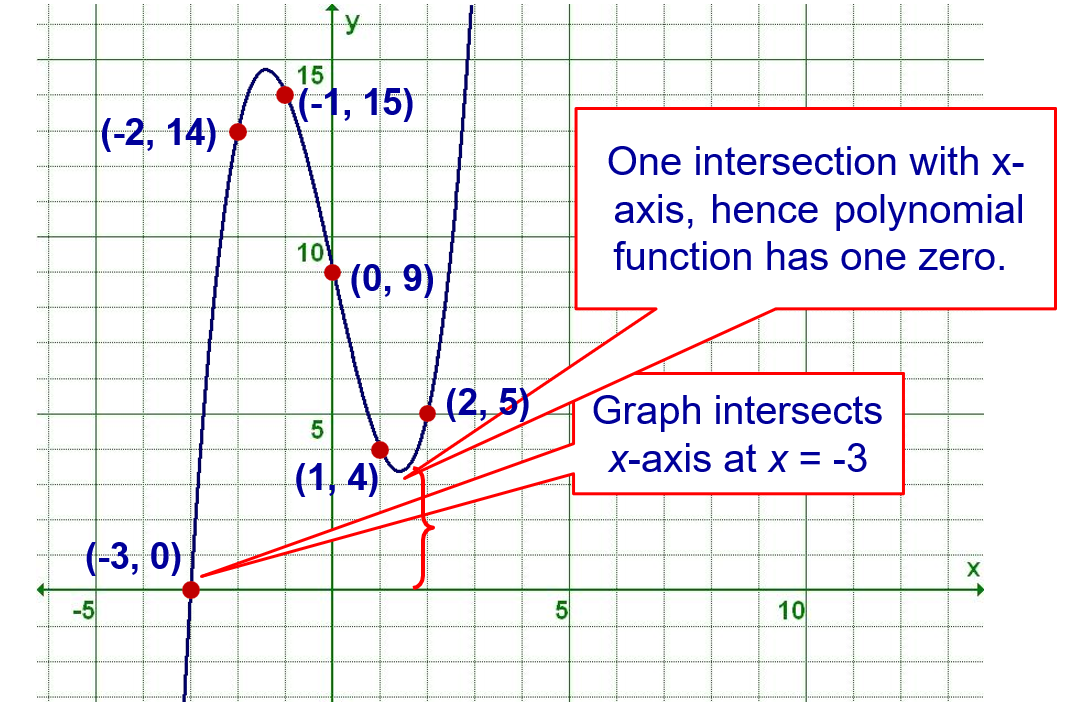
• Cubic Polynomials: A cubic polynomial ax³ + bx²+ cx + d can have up to three zeroes. For example, x³−3x−2 has two zeroes, while x³−6x+9 has one zero.

           Let’s see for x³−6x+9

Relation Between Zeroes and Coefficients

For polynomial functions, there are specific relationships between the zeroes and the coefficients:

• Quadratic Polynomials: For a quadratic polynomial ax² + bx + c, if the zeroes are α and β: 

1. Sum of Zeroes: α + β = − b / a
2. Product of Zeroes: α β =  c / a

Example: Consider the polynomial x² – 3x + 2. We will find the zeroes of this polynomial. Then, we will see whether there exists some relation between its zeroes and its coefficients.

•  We have quadratic polynomial x² – 3x + 2.  By the method of splitting the middle term, we have x² – 3x + 2 

         = x² – x – 2x + 2 (splitting middle term)

 = x(x – 1) – 2(x – 1) = (x – 1)(x – 2). 

If x² – 3x + 2 = 0, then , x – 1 = 0 , or x – 2 = 0 Hence, we have x = 1 or x = 2.

• sum of roots = 1 + 2 = 3/1 = -(coefficient of x)/(coefficient of x²).
• product of roots = 1 x 2 = 2 = (constant term)/(coefficient of x²).

Based on this example, we can generalize the relation between the zeroes and coefficients of a quadratic polynomial in subsequent slides.

•  Cubic Polynomials: For a cubic polynomial ax³ + bx²+ cx + d, if the zeroes are α,β,γ:

1. Sum of Zeroes: α + β + γ = − b / a 
2. Sum of Products of Zeroes Taken Two at a Time: αβ + βγ + γα = c / a
3. Product of Zeroes: α β γ = − d / a

Example:

Considering the cubic polynomial x³ – 3x² – 4x, let us find the zeroes of this polynomial. See whether there exists some relation between its zeroes and its coefficients.

We have cubic polynomial x³ – 3x² – 4x. Factorizing the polynomial as

x³ – 3x² – 4x = x (x² – 3x – 4x) = x (x +1)(x – 4) 

If x³ – 3x² – 4x = 0, then x = 0 or x – 4 = 0 or x + 1 = 0.

Hence, we have x = 0 or x = -1 or x = 4

Therefore, the zeroes of x³ – 3x² – 4x are a = 0, b = -1 and c = 4.

Now, sum of zeroes = a + b + c = 0 + (-1) + 4 = 4 – 1 = 3 = 3/1

= -(Coefficient of x²)/Coefficient of x³

sum of products of zeroes taken two at a time = ab + bc + ca = 0 + (-4) + 0 = -4 = (Coefficient of x)/Coefficient of x³

product of zeroes = a.b.c = 4.(- 1).0 = 0

= (Constant term)/Coefficient of x³

Division Algorithm for Polynomials

To find all zeroes of a polynomial, especially cubic ones, we can use the Division Algorithm. If a polynomial p(x) is divided by a factor (x−k), the quotient can be further analyzed to find other factors. For instance, dividing 2x³ + x² − 5x + 2 by (x−1) helps us determine the other factors and zeroes.

After solving the question, we have,

Divided = 2x³ + x² – 5x + 2, Divisor = x – 1, Quotient = 2x² + 3x – 2,  and Remainder = 0.

Let us check the basic rule of division: 

Dividend = divisor x quotient + remainder

Here, we have

2x³ + x² – 5x + 2 = (x – 1) * (2x² + 3x – 2) + 0

We can generalize this rule for any general polynomial of degree n. This rule is known as the division algorithm.

The division algorithm states that for any polynomial p(x) and any non-zero polynomial g(x), there exists unique polynomials q(x) and r(x) such that

p(x) = g(x) ´ q(x) + r (x)

Where, r(x) = 0 or degree of r(x) < degree of g(x).

Let’s Conclude

In conclusion, mastering CBSE Class 10th Math, Chapter 2 – Polynomials is essential for building a strong foundation in algebra. Through this chapter, you explore polynomial functions, their properties, and their graphical representations. By understanding concepts such as the relationship between zeroes and coefficients, factorization, and the division algorithm, you enhance your problem-solving abilities. With the resources provided by iPrep, your learning experience in Chapter 2 – Polynomials will be engaging and effective, preparing you for both exams and real-world applications. So, dive into Chapter 2 – Polynomials, and strengthen your mathematical skills with confidence!