Complete Guide For Class 10 Math Chapter 3 – Pair of Linear Equations in Two Variables

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Pair of Linear Equations in Two Variables in Mathematics for Class 10th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter on Pair of Linear Equations in Two Variables introduces students to various methods for solving systems of two linear equations. It begins with the graphical method, teaching students how to represent and solve a pair of linear equations on a coordinate plane. The chapter then delves into algebraic methods, such as substitution and elimination, equipping students with effective tools for solving linear equations.

The concept of consistency and inconsistency of solutions is also covered, enabling students to determine when a system of equations has a unique solution, no solution, or infinitely many solutions. By mastering these techniques, students can address real-world problems involving linear relationships, thereby enhancing their problem-solving and analytical abilities. This chapter lays a critical foundation for understanding more advanced algebraic concepts and their practical applications across various fields.

Pair of Linear Equations in Two Variables

In algebra, a pair of linear equations in two variables represents a system of equations where each equation is a straight line when graphed on a coordinate plane. These equations involve two variables, typically denoted as xxx and yyy, and are written in the general form:

• a₁x + b₁y = c₁
• ​a₂x + b₂y = c₂​

where a₁, b₁, c₁​, a₂, b₂, and c₂​ are constants.

Pair of Linear Equations

Let’s understand with examples:

2x + 3y = 4 and 5x – y = 8

These represent a system of linear equations in two variables.

So, a pair of linear equations in two variables is said to form a system of linear equations.

Solution of a Pair of Linear Equations in Two Variables

A pair of values of the variables x and y, which satisfies each of the given equations in terms of x and y, is called its solution.

Example :

Check if x = 4 and y = 4 is a solution of the pair of linear equations.

x + 2y = 12…..(a)

2x – y = 4…..(b)

Substituting x = 4 and y = 4 in (a), we get:

LHS = 4 + 2(4) = 4 + 8 = 12 = RHS.

Substituting x = 4 and y = 4 in (b), we get:

LHS = 2(4) – 4 = 4 = RHS

Thus, x = 4 and y = 4 satisfy both the equations.

Hence, x = 4 and y = 4 is the solution of the pair of linear equations.

Graphical Representation of a Pair of Linear Equations

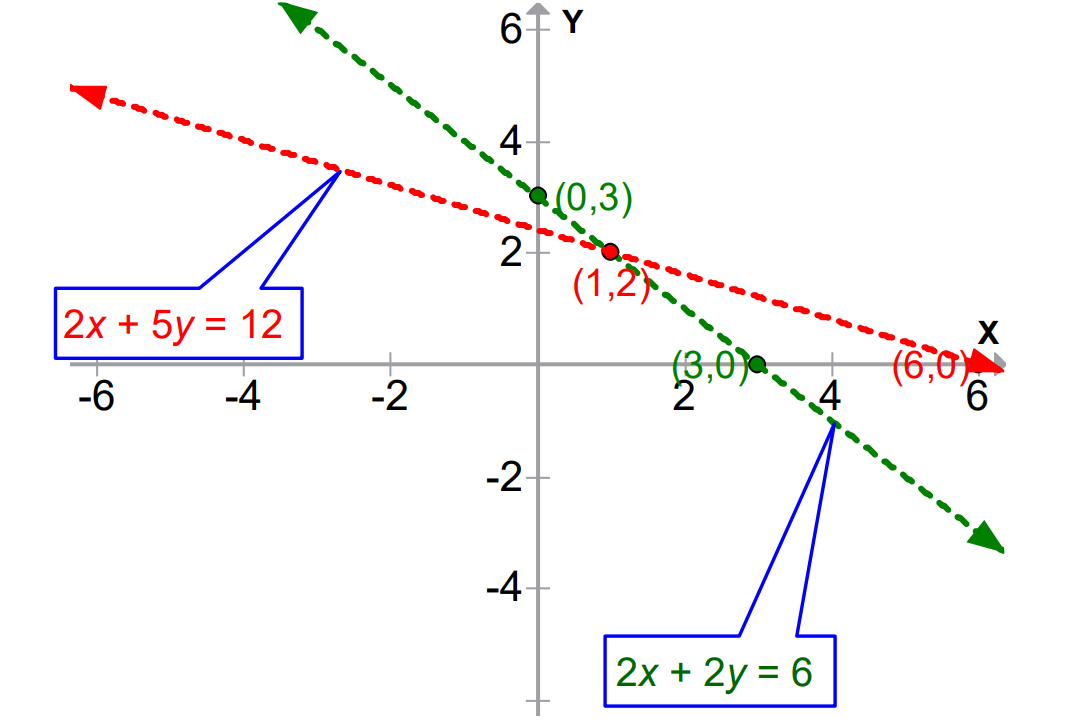
Consider the following Example: 

2 pens and 2 pencils together cost Rs 6 and 2 pens and 5 pencils cost Rs

12. Represent the situation algebraically and graphically.

Solution: Let the cost of a pen be Rs x and the cost of a pencil be Rs y. The cost of 2 pens and 2 pencils is Rs 6.

Therefore, 2x + 2y = 6. Also, 2 pens and 5 pencils cost Rs 12. Therefore, 2x + 5y = 12

Thus, the algebraic representation of the given situation is

2x + 2y = 6

2x + 5y = 12

To obtain a graphical representation of the given situation, find two points on the line representing each equation.

i.e., find two solutions to each equation.

2x + 2y = 6……(a)

2x + 5y = 12…..(b)

For 2x + 2y = 6

Put x = 0, then 2 x 0 + 2y = 6 ⇒ 2y = 6 ⇒ y = 3

When y = 0, then 2x + 2 x 0 = 6 ⇒ 2x = 6 ⇒ x = 3

Thus, two solutions of the first equation are:

x	0	3
y	3	0

 Now, Consider the second equation, 2x + 5y = 12

 When y = 0, then 2x + 5 x 0  = 12  ⇒ 2x = 6 ⇒ x = 3

When y = 2,  then 2x + 5 x 2  = 12  ⇒ 2x = 12-10 ⇒ 2x = 2 ⇒ x = 1

Thus, two solutions of the second equation are:

x	6	1
y	0	2

Now, plot the points (0, 3) and (3, 0) and draw a line passing through these

points to represent the equation, 2x + 2y = 6.

Represent the second equation, 2x + 5y = 12 by plotting the points (6, 0) and (1, 2).

Graphical Method for Solving a Pair of Linear Equations

Let’s understand the following steps

STEP 1: 

Obtain the given pair of linear equations in terms of x and y. Let the pair of equations be,

 a₁x + b₁y + c₁= 0

​a₂x + b₂y + c₂= 0​ 

STEP 2:

Draw the graphs of the equation (a) and (b) in STEP1.

Let the lines l₁ and l₂ represent the graphs of (a) and (b) respectively.

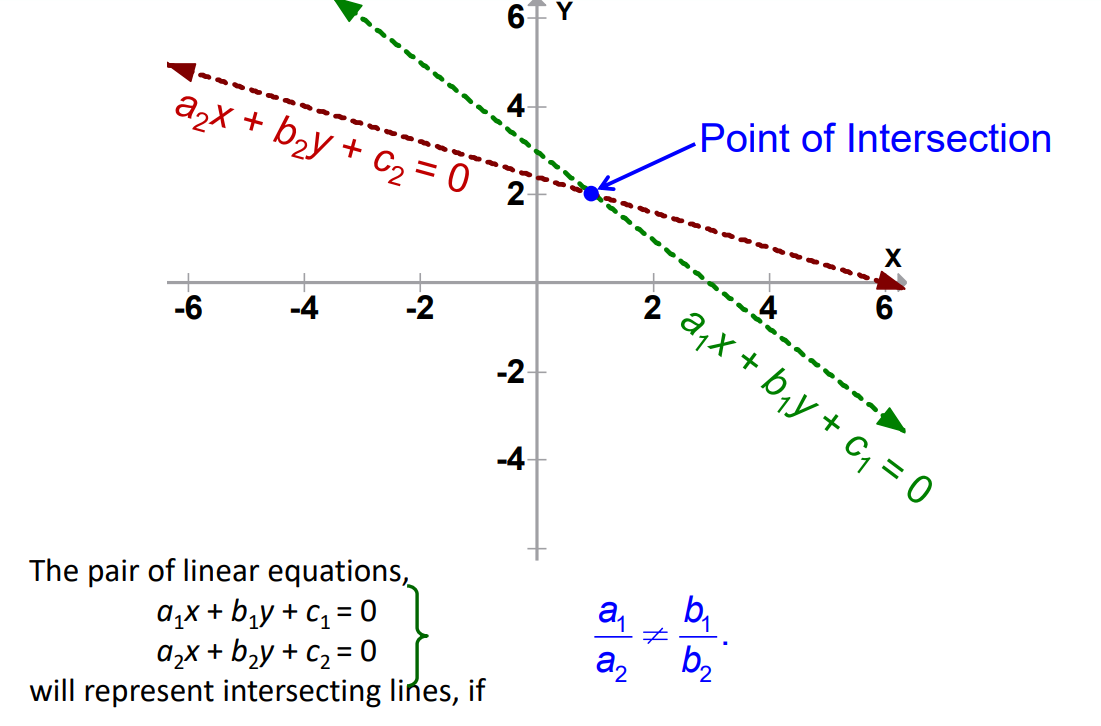
STEP 3:

If the lines l₁ and l₂ intersect at a point and (p, q) are the coordinates of this point, then the given pair of equations has a unique solution, given by x = p, y = q, else go to STEP 4.

STEP 4:

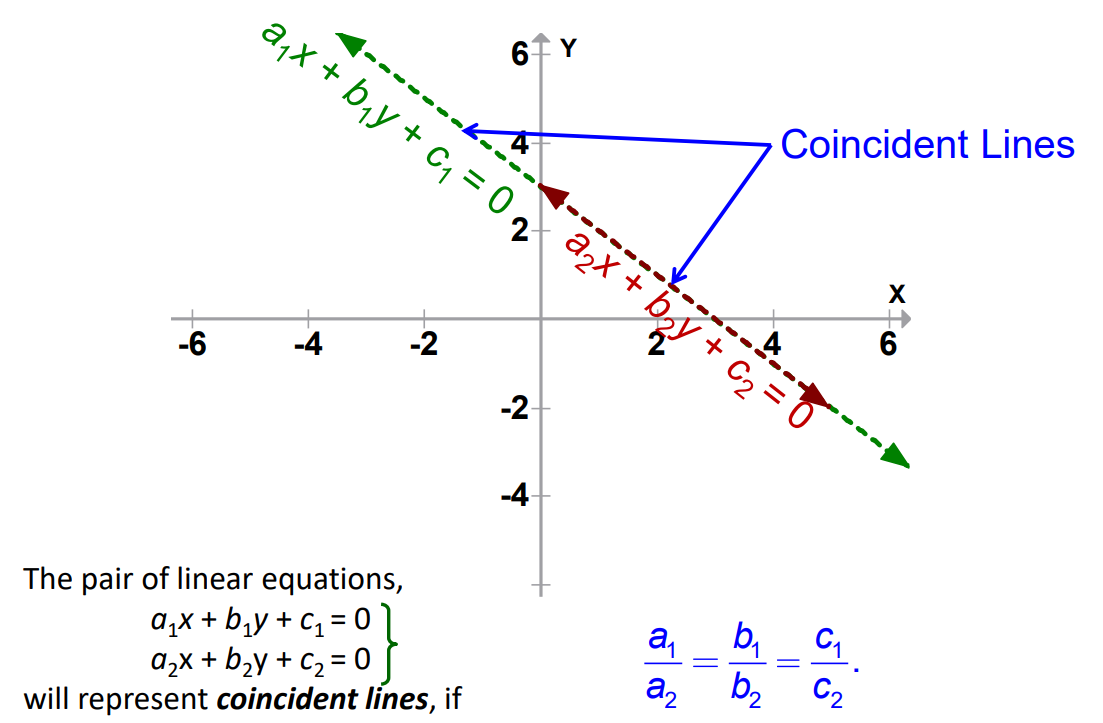
If the lines l₁ and l₂ are coincident, then the pair of equations is consistent and has infinitely many solutions. In this case, each solution of one of the equations is a solution of the system, else go to STEP 5.

STEP 5:

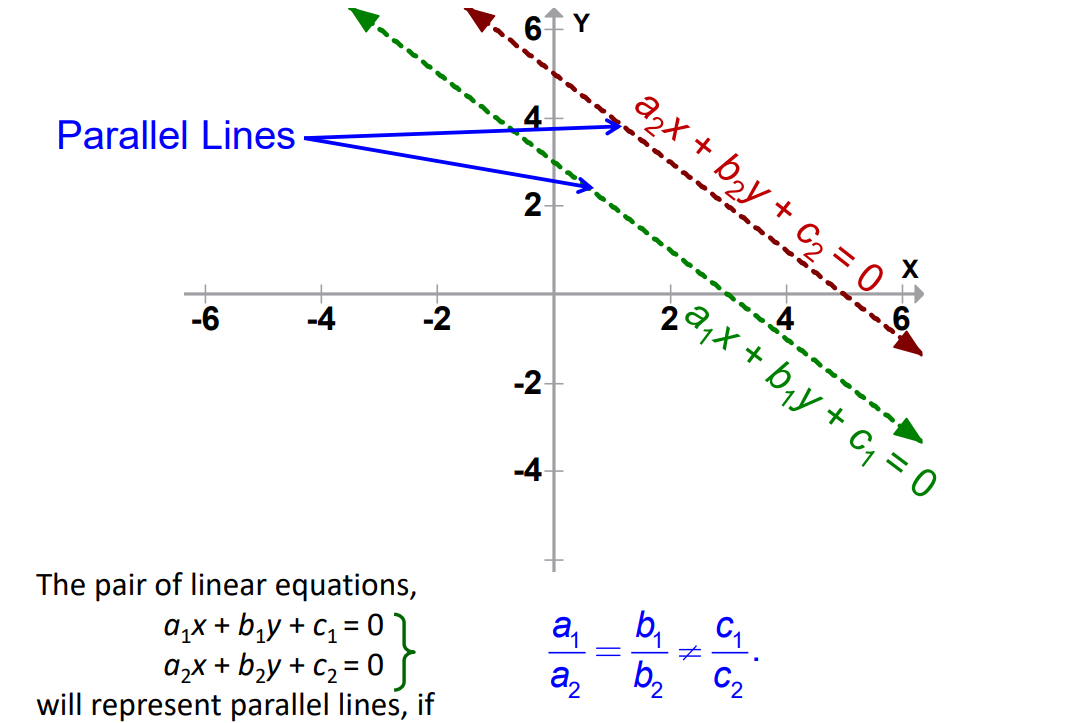
If the lines l₁ and l₂ are parallel, then the given pair of equations is

inconsistent, i.e., it has no solution.

Unique Solution

Example – Unique Solution

Determine the value of k for which the following pair of linear equations has a unique solution.

2x + 3y – 5 = 0

kx – 6y – 8 = 0

For the equations to have a unique solution, we should have

a₁ /a₂ ≠ b₁ /b₂

Substituting a₁ = 2, a₂ = k, b₁ = 3 and b₂ = -6, we get

2/k = 1/-2 ⇒ k = -4

Hence, the pair of linear equations will have a unique solution for all values

of k other than –4.

Infinitely Many Solutions

Example – Infinite Solutions

Determine the value of k for which the given pair of linear equations will have infinitely many solutions.

kx + 3y = k – 3

12x + ky = k

Rewrite the given pair of equations as:

kx + 3y –(k – 3) = 0,

12x + ky – k = 0

For the equations to have infinitely many solutions, we should have

a₁ /a₂ = b₁ /b₂ = c₁ /c₂

Substitute a₁ = k, a₂ = 12, b₁ = 3, b₂ = k, c₁ = – (k – 3) and c₂ = – k

Thus, we get k /12 = 3/k = -(k -3) /-k

1st and 2nd expression ⇒ k /12 = 3/k ⇒ k = ± 6 and 2nd and 3rd expression 3/k = -(k -3) /-k ⇒ k = 0 or k = 6.    

k = 6 is a common value. Hence, the pair of linear equations will have infinitely many solutions at k = 6.

No Solutions

Example – No Solution

For what value of k will the following pair of linear equations have no solution?

3x + y = 1 (2k –1)x + (k – 1)y = 2k + 1

Rewrite the given pair of equations as

3x + y –1 = 0, (2k –1)x + (k – 1)y – (2k + 1) = 0

For the equations to have no solution, we should have

a₁ /a₂ = b₁ /b₂ ≠ c₁ /c₂

Substitute a₁ = 3, a₂ = 2k – 1, b₁ = 1, b₂ = k – 1,

c₁ = -1 and c₂ = -(2k + 1)

We get, 3/2k-1 = 1/k-1 ≠ -1/-(2k+1) 

         ⇒ 3/2k-1 = 1/k-1 and 1/k-1 ≠ -1/-(2k+1)

Now,  3/2k-1 = 1/k-1⇒ 3 (k-1) = (2k-1) ⇒ 3k-3 = 2k-1 ⇒ k = 2

Also, for k = 2, 1/k-1 ≠ 1 /2k+1

Hence, for k = 2, the given pair of equations will have no solution.

Now let’s understand the different pairs of Linear Equations.

Different Pair Of Linear Equations

• Consistent Pair of Linear Equations 

A pair of linear equations in two variables that has a solution is called a consistent pair of linear equations.

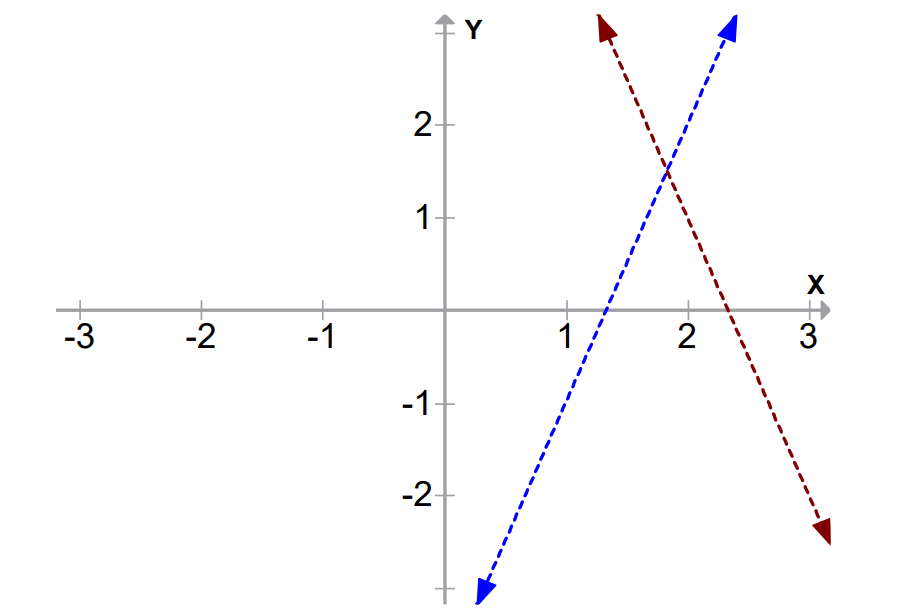
• Inconsistent Pair of Linear Equations 

A pair of linear equations that has no solution is called an inconsistent pair of linear equations.

• Dependent Pair of Linear Equations 

A pair of linear equations that are equivalent and have infinitely many distinct common solutions is called a dependent pair of linear equations in two variables. 

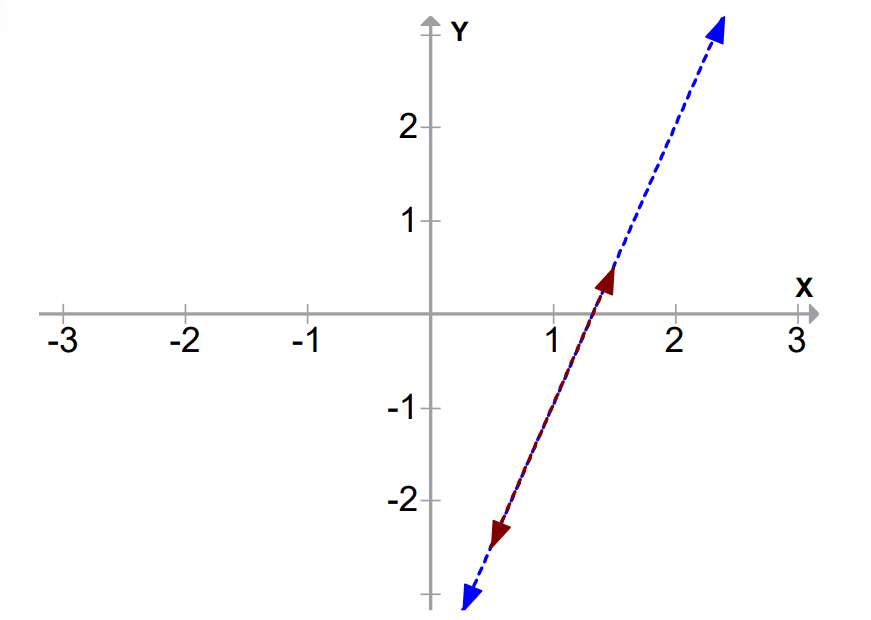
Algebraic Method of Solving Pair of Linear Equations

• Substitution Method

Let’s understand the following steps 

STEP 1:

Choose any one of the equations and find the value of one variable in terms of the other variable.

Consider the following pair of equations:

3x + y = 3…..(a)

3x – y = 3…..(b)

Let us take the equation (a) 3x + y = 3, y = 3 – 3x

 STEP 2:

3x – y = 3 

3x – (3 – 3x ) = 3 

3x – 3 + 3x = 3 

6x = 6

 x = 1

STEP 3:

Substitute the calculated value of the variable in STEP 1 into

equation (b) and solve for the second variable.

3x – y = 3 3x – (3 – 3x ) = 3

3x – 3 + 3x = 3

6x = 6

x = 1

Substitute the solution from STEP 2 into equation (a) and solve for

the variable in the equation.

3x + y = 3 3(1)+ y = 3

3 + y = 3

y = 0

• Elimination Method

 STEP 1:

 Multiply both equations by a non-zero constant such that the variable to be eliminated has equal coefficients.

Consider the following pair of equations: 3x + 4y = 10 …(a)

x – y = 1…(b)

Multiply equation (a) by 1 and equation (b) by 4 to make the coefficients of y equal.

3x + 4y = 10…(c)

4x – 4y = 4 …(d)

STEP 2:

Add or subtract the equations obtained in STEP 1, so that the variable whose coefficients are equal gets cancelled out.

Add equations (c) and (d)

 (3x + 4 y = 10) + (4x – 4y = 4) 

7x = 14 ⇒ x = 14/7 = 2 

 STEP 3:

 Solve the equation in one variable obtained in STEP 2.

7x = 14 ⇒ x = 2

STEP 4:

Substitute the value obtained in STEP 3 in either of the original

equation to obtain the value of the other variable.

3x + 4y = 10

3(2) + 4y = 10

6 + 4y = 10

4y = 4

y = 1

Let’s Conclude

In conclusion, mastering the concepts of “Pair of Linear Equations in Two Variables” is crucial for building a solid foundation in algebra and solving real-world problems efficiently. By understanding and applying both graphical and algebraic methods, such as substitution and elimination, students can effectively tackle linear equations and interpret their solutions. The chapter not only provides essential techniques for solving systems of equations but also emphasizes the importance of consistency and inconsistency in solutions.

Whether through graphical representation or algebraic manipulation, the ability to solve a pair of linear equations in two variables is a vital skill that extends beyond the classroom, aiding in various practical and academic scenarios. As you continue exploring the “Pair of Linear Equations in Two Variables,” remember that practice and application are key to mastering this fundamental concept in mathematics.