Area and Its Boundary – Complete Guide For Class 2 Math Chapter 11

Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Area and Its Boundary in Mathematics for Class 5th are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples. 

The chapter Area and Its Boundary introduces students to the fundamental concepts of area and perimeter. Through engaging activities and real-life examples, students learn how to measure the space an object occupies (area) and the distance around it (perimeter). The chapter begins with a practical discussion of comparing areas, followed by exercises that involve counting squares to measure areas of shapes like rectangles and squares. 

Students in the chapter area and its boundary, also explore the relationship between area and perimeter by experimenting with different shapes that have the same perimeter but varying areas. Practical examples, such as covering a floor with tiles or fencing a garden, help students apply these concepts to real-world situations. The chapter encourages critical thinking and problem-solving as students learn to measure, calculate, and compare different areas and perimeters in everyday contexts.

Area and Its Boundary

In the chapter, Area and Its Boundary, students dive into the concepts of area and perimeter, which are essential in understanding the size of objects and the space they occupy. 

The chapter begins with a practical discussion, where Parth and Gini compare the sizes of their aam papad slices, raising questions about how to measure and compare areas.

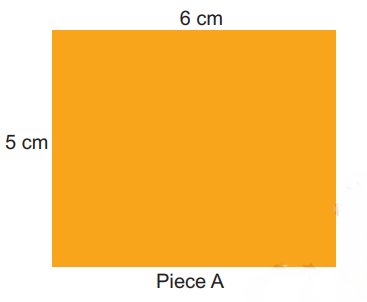
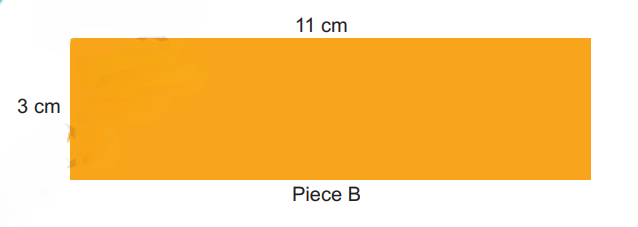
Understanding Area through Real-Life Examples

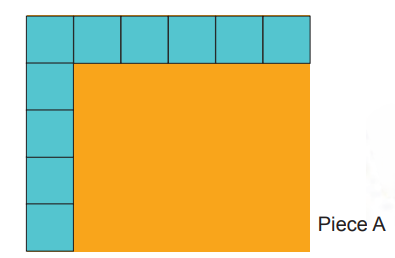
The chapter Area and Its Boundary introduces the idea of the area by asking students to count squares. This approach makes it easier to visualize how much space an object covers. 

For example, if an object covers 10 squares, its area is expressed as “10 square units.” Students learn to measure the area of simple shapes like squares and rectangles by multiplying the length and width. 

By placing the square on the aam papad slices and counting the squares, we can determine which slice is larger than the other.

Formulas for Measuring The Area and Perimeter

Here are several formulas from the chapter Area and Its Boundary for measuring the area and perimeter of different shapes like Rectangles and Squares.

Definitions:

     Area of a Rectangle:

• The area of a rectangle is calculated by multiplying its length and breadth.
• Formula: Area = Length × Breadth

       Area of a Square:

• The area of a square is found by multiplying the length of one side by itself.
• Formula: Area = Side × Side

Using Stamps, Tiles, and More to Measure the Area

One engaging activity from the chapter Area and Its Boundary involves covering objects with stamps to estimate their area, helping students grasp the concept of square units playfully. 

If the stamp has an area of 4 square cm, we can calculate the area of the rectangle by determining how many of these stamps are needed to fully cover the larger rectangle.

Another scenario asks students to calculate how many tiles would be required to cover a kitchen floor, providing a clear connection between area measurement and practical applications in real life.

Perimeter – Measuring the Boundary

In addition to area, the chapter Area and Its Boundary explores the concept of perimeter, which refers to the distance around a shape. By measuring the sides of squares and rectangles, students learn to calculate the perimeter by adding up all the sides. This skill is useful in many day-to-day situations, such as determining the length of fencing needed for a garden.

Definitions:

   The perimeter of a Rectangle:

• The perimeter is the total distance around the rectangle, calculated by adding all its sides.
• Formula: Perimeter = 2(Length + Breadth)

   The perimeter of a Square:

• The perimeter is calculated by multiplying the length of one side by 4.
• Formula: Perimeter = 4 × Side

Activity:

My Belt is the Longest!

Take a thick paper sheet measuring 14 cm in length and 9 cm in width, or you can use an old postcard instead.

1. Calculate its area and perimeter.
2. Cut strips of equal size from the paper.
3. Using tape, join the strips end to end to make a belt.

After measuring, we can find the length and perimeter of the belt and compare it with the lengths of other belts.

The belt activity aims to understand that things with the same area can take different forms and also have very different perimeters. While measuring sides, lengths in mm can be rounded off for this activity.

Activity:

Puzzle: Pass through a Postcard

Look! I can pass through a postcard. I made a loop without cutting the strips.

This belt is for the elephant.

The belt activity and Postcard activity from the chapter Area and Its Boundary, aim to understand that things with the same area can take different forms and also have very different perimeters. While measuring sides, lengths in mm can be rounded off for this activity.

The chapter Area and Its Boundary also covers the fascinating relationship between area and perimeter. 

Students discover that different shapes can have the same area but different perimeters. Conversely, shapes with the same perimeter can have varying areas. These insights are reinforced through practical problems and exercises.

Activity: People People Everywhere

Objective:
To help students understand and measure spatial dimensions through a fun, interactive game.

Materials Needed:

• Chalk or tape
• Measuring tape or ruler

Instructions:

Create two Square Metre Grids:
Mark two squares on the floor, each measuring 1 square meter, using chalk or tape. These squares will be used as the reference for the activity.

Divide into Teams:
Split the class into two teams. Each team will take turns completing the challenges below.

Challenges:

• Challenge 1: How many students from each team can sit within the 1 square meter area? Record the number.
• Challenge 2: How many students from each team can stand within the same 1 square meter? Record the number.
• Discussion: After the challenges, discuss which team could fit more students sitting and standing in the square and why.

Classroom Measurement:

• Measure the length of your classroom: Length: _______ m
• Measure the width of your classroom: Width: _______ m
• Calculate the area of your classroom: Area: _______ square meters

Estimating Occupancy:

• How many students are in your class? _______
• How many students can fit within 1 square meter based on the challenge? _______
• If you want space to move around, estimate how many students should comfortably occupy 1 square meter: _______

Understanding Large Areas

The chapter Area and its boundary also covers the understanding of large areas. 

• Fun Fact: Imagine how big a square of side 1 kilometer would be. Its area is ______ square kilometers.
• Real-Life Connection: For example, in West Bengal, around 900 people live in 1 square kilometer, while in Arunachal Pradesh, less than 15 people live in the same area.

Equally Dividing Land – A Real-Life Scenario

They are tasked with figuring out how to equally distribute a piece of land, considering both area and perimeter constraints.

By calculating the area and perimeter, we can determine if the land distribution has been done accurately or not.

Exploring the Relationship Between Area and Perimeter 

The chapter Area and Its Boundary also covers the fascinating relationship between area and perimeter. 

Students discover that different shapes can have the same area but different perimeters. Conversely, shapes with the same perimeter can have varying areas. These insights are reinforced through practical problems and exercises.

Thread Play – Activity

Take a 15 cm long thread. Make different shapes by joining their ends on this sheet. 

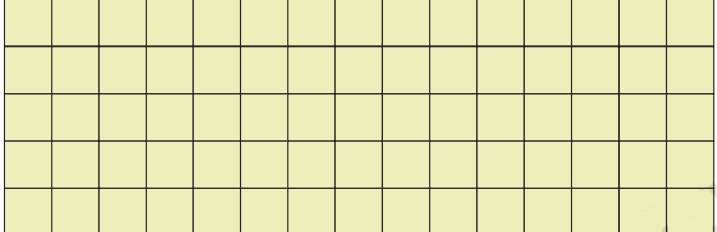
By comparing the area of different shapes, we can determine which one is larger or smaller.

We can also form different shapes like triangles, squares, rectangles, and circles, and compare them to determine which shape has the largest and smallest area.

Save the Bird

Another very fun topic covered in the chapter area and its boundary is Save the Bird. 

Understanding Scale Drawings: We learn how to use a scale to convert measurements from a drawing into actual distances. In this case, the scale is 1 cm = 1 km, helping us visualize and calculate the real-world size of the lakes.

Measuring Boundaries: By measuring the boundary of irregular shapes (like lakes) with tools such as a thread, we are learning how to apply practical methods to calculate the perimeter in real-world scenarios.

King’s Story 

From this story given in the chapter Area and Its Boundary, we learn about the relationship between area and perimeter. The king offers Cheggu land based on a fixed perimeter (100 meters of wire), and Cheggu experiments with different rectangles to maximize the area he can enclose. This teaches us that for a given perimeter, different shapes can have different areas.

On the other hand, Anar is given a fixed area (800 square meters) and is asked to create a boundary for it. By trying different rectangle dimensions, Anar discovers that the perimeter can vary greatly even if the area remains the same. For example, a rectangle that is 1 cm wide and 80,000 m long will have a massive perimeter, despite the area being the same as a more balanced rectangle like 40 m x 20 m. This illustrates how shapes with the same area can have very different perimeters.

The story also highlights why a circle might be a better choice than a rectangle for enclosing a maximum area with a given perimeter, as Cheggu’s wife suggested. This is a practical lesson in optimization: choosing dimensions and shapes wisely to maximize area or minimize boundary length, depending on the requirement.

Conclusion

In conclusion, CBSE Class 5th Math, Chapter 11 – Area and Its Boundary equips students with a thorough understanding of the key concepts of area and perimeter. Through interactive activities and practical examples, such as measuring objects with stamps or calculating fencing requirements for gardens, students learn to apply these mathematical concepts to real-life scenarios. The chapter Area and Its Boundary also introduces the relationship between area and perimeter, helping students explore how different shapes can have the same perimeter but different areas, or vice versa.

By the end of CBSE Class 5th Math, Chapter 11 – Area and Its Boundary, students are prepared to measure, calculate, and compare areas and perimeters, skills they will continue to build upon in higher classes. With iPrep’s engaging learning resources, mastering these concepts becomes easier and more enjoyable for Class 5th students.