Lines and Angles – Complete Guide For Class 9 Math Chapter 6
Welcome to iPrep, your Learning Super App. Our learning resources for the chapter, Lines and Angles in Mathematics Class 9th chapter 6 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions and notes offer you the best of integrated learning with interesting explanations and examples.
Chapter 6 introduces fundamental geometric concepts. It covers types of lines (parallel, perpendicular), angles (acute, obtuse, right, complementary, supplementary, linear pair, adjacent, vertically opposite), and their properties. The key focus is on parallel lines and transversals, exploring angle relationships like corresponding, alternate interior, and interior angles on the same side of the transversal.
Angle: Definition and Components
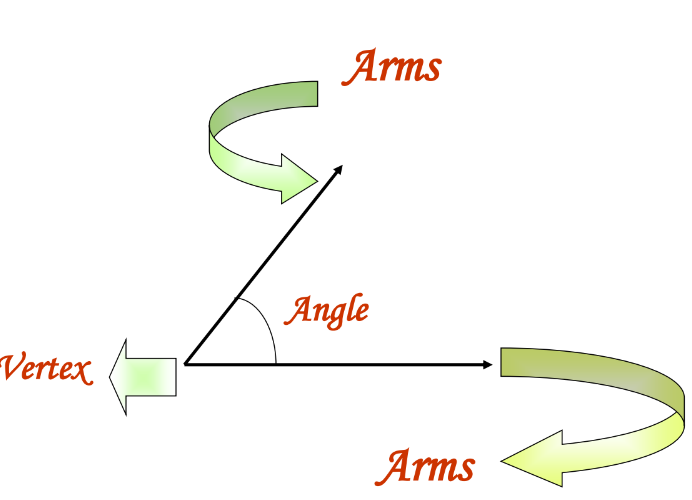
According to the chapter Lines and angles, an angle is formed by two rays meeting at a common point. The parts of an angle include:
- Arms: The rays forming the angle.
- Vertex: The common endpoint where the rays meet.
Types of Angles
There are various types of angles covered in the chapter lines and angles. these include-
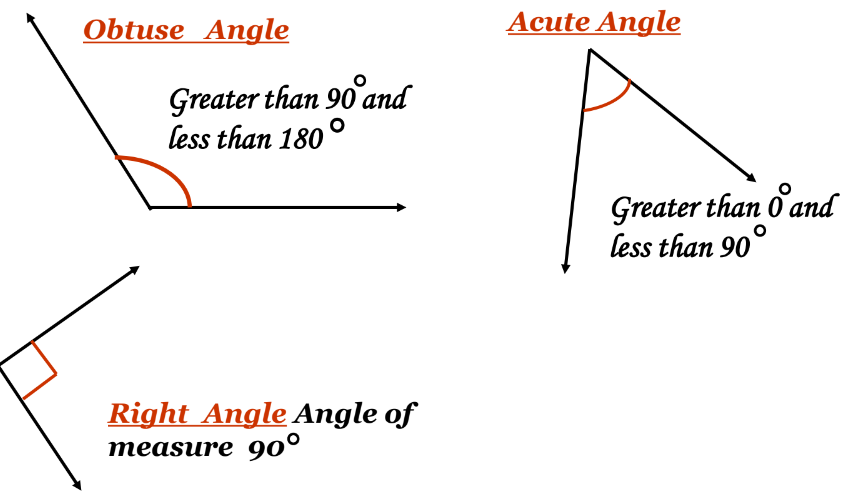
1. Acute Angle
- Greater than 0° and less than 90°.
2. Right Angle
- Measures exactly 90°.
3. Obtuse Angle
- Greater than 90° and less than 180°.
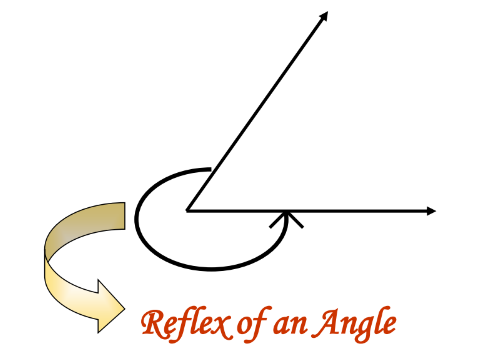
4. Reflex Angle
- Greater than 180° and less than 360°.
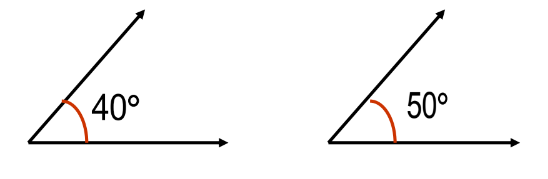
5. Complementary Angles
- Two angles whose sum is 90°. Example: 40° and 50°.
6. Supplementary Angles
- Two angles whose sum is 180°. Example: 120° and 60°.
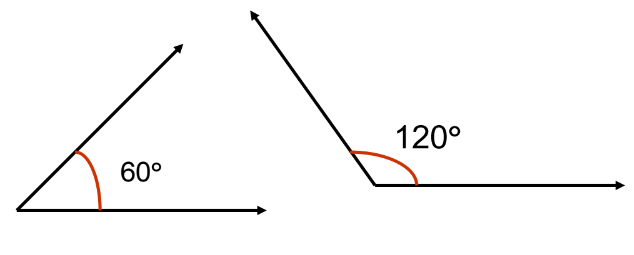
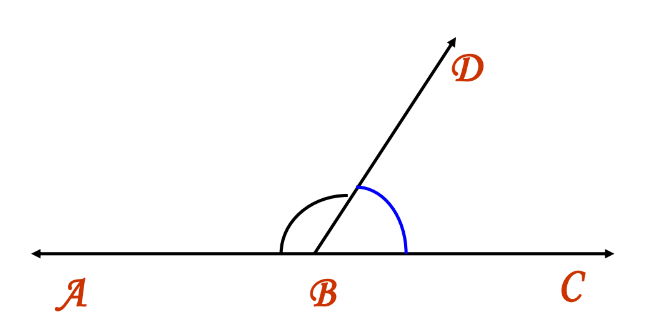
7. Linear Pair of Angles
- Two adjacent angles whose non-common arms form a straight line. Example: ∠CBD and ∠ABD form a linear pair.
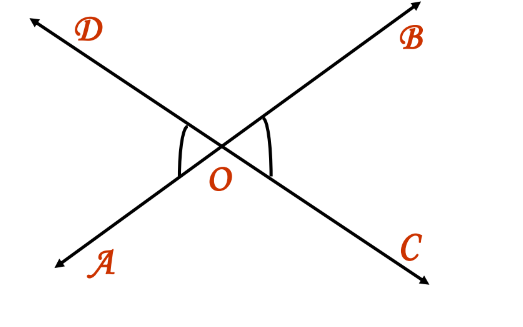
8. Vertically Opposite Angles
- When two lines intersect, the opposite angles are equal. Example: ∠AOD = ∠BOC and ∠DOB = ∠COA.
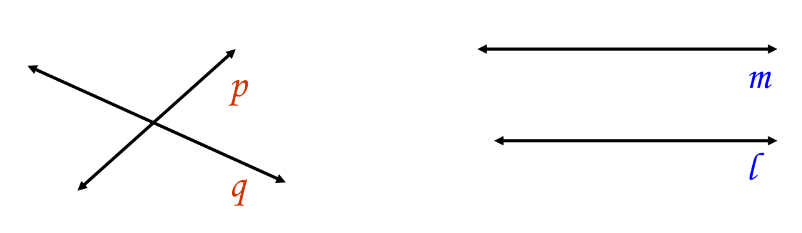
Intersecting and Non-Intersecting Lines
- Lines can either intersect or be parallel.
Theorems and Applications
The chapter lines and angles from class 9 math cover multiple theorems and applications. These include-
Vertically Opposite Angles Theorem
- If two lines intersect, their vertically opposite angles are equal.
- Proof: Given lines intersect at point O:
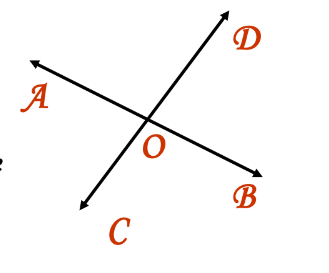
∠AOC + ∠AOD = 180° and ∠AOD + ∠DOB = 180°
By equating, ∠AOC = ∠DOB.
Hence Proved
Example Problems
- Problem 1:
- Given: Ray OS stands on line POQ, and rays OR and OT are angle bisectors of ∠POS and ∠SOQ, respectively. If ∠POS = x, find ∠ROT.
Solution:

∠POR + ∠ROS + ∠SOT + ∠TOQ = 180°
Since POR = ROS and SOT = TOQ:
2∠ROS + 2∠SOT = 180°
∠ROT = 90°
- Problem 2:
- Given: Lines PQ and RS intersect at point O. If ∠POR:∠ROQ = 5:7, find all angles.
Solution:
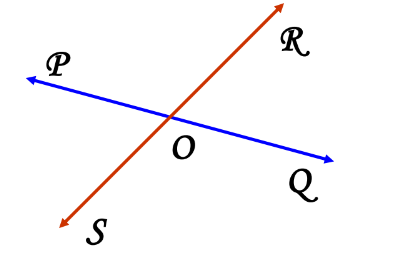
Let ∠POR = 5x and ∠ROQ = 7x.
5x + 7x = 180° (Linear pair property)
x = 15°
∠POR = 75° and ∠ROQ = 105°.
- Problem 3:
- Given: Lines AB and CD intersect at O. If ∠AOC + ∠BOE = 70° and ∠BOD = 40°, find ∠BOE and the reflex of ∠COE.
Solution:

∠AOC + ∠COE + ∠EOD = 180°
70° + ∠COE = 180°
∠COE = 110°
∠COE + ∠EOB + ∠BOD = 180°
110° + ∠BOE + 40° = 180°
∠BOE = 30°
Reflex of ∠COE = 360° – 110° = 250°.
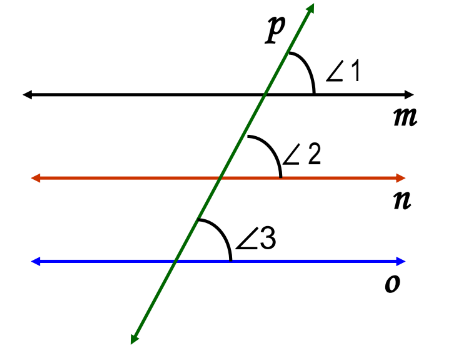
Parallel Lines and a Transversal

Corresponding Angles
- Angles in the same relative position at each intersection.
- ∠1 & ∠5, ∠2 & ∠6, ∠3 & ∠7, ∠4 & ∠8.
Alternate Interior Angles
- Angles on opposite sides of the transversal but inside the parallel lines.
- ∠3 & ∠5, ∠4 & ∠6.
Interior Angles on the Same Side
- Angles on the same side of the transversal.
- ∠3 + ∠6 = 180°, ∠4 + ∠5 = 180°.
Theorem: Lines Parallel to the Same Line
- Given: Lines m and n are parallel to line o.
m || o and n || o
So, m || n.
Example Problems on Parallel Lines
- Problem 1:
- Given: AB || CD, find x and y.
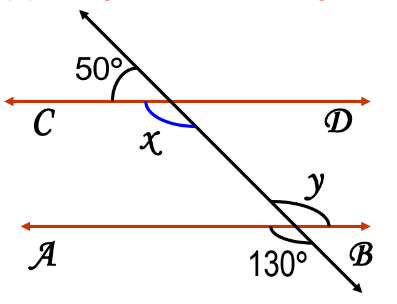
Solution:
x = 180° – 50° = 130°
x = y = 130° (Corresponding angles).
- Problem 2:
- Given: RS || PQ, ∠PAB = 70° and ∠ACS = 100°, determine ∠ABC, ∠BAC, and ∠CAQ.
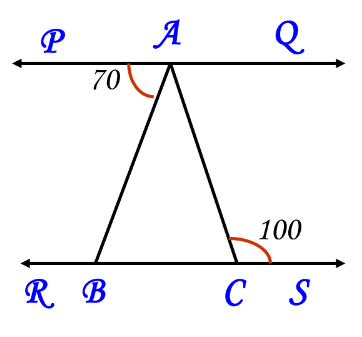
Solution:
∠ACS = ∠PAB + ∠BAC
100° = 70° + ∠BAC
∠BAC = 30°.
- Problem 3:
- Given: AB || CD, find the reflex of ∠BXC.

Solution:
∠ABX = 45°, ∠DCX = 50°
∠BXC = 45° + 50° = 95°
Reflex of ∠BXC = 360° – 95° = 265°.
Angle Sum Property of a Triangle
Theorem: Sum of Angles in a Triangle
- The sum of the angles in a triangle is 180°.
Proof: Draw a line l parallel to BC, then
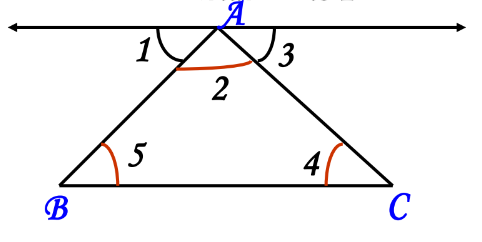
∠1 = ∠5 and ∠3 = ∠4
Also, ∠1 + ∠2 + ∠3 = 180°
On replacing values we get
∠5 + ∠2 + ∠4 = 180°
Hence proved that the sum of the angles of a triangle is 180°.
Theorem: Exterior Angle
- The exterior angle of a triangle is equal to the sum of the opposite interior angles.
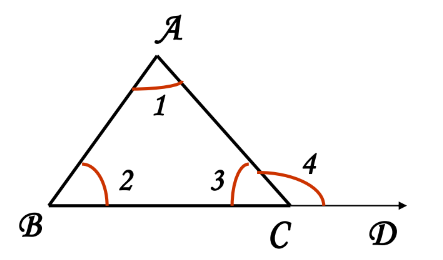
∠ACD + ∠CAB = ∠ABC
Example Problems on Triangles
- Problem 1:
- Given: QT ⊥ PR, ∠TQR = 40°, and ∠SPR = 30°, find x and y.
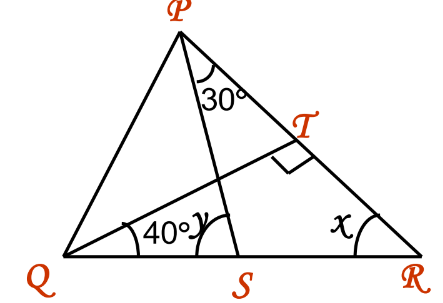
Solution: In the triangle TQR, 40° + 90° + x = 180°
x = 50° (Using angle sum property)
Also, y = ∠SPR + 50°
y = 80° (Exterior angle property).
These concepts and examples form the foundation of understanding lines and angles, crucial for higher-level geometry studies.
Let’s Conclude
In conclusion, CBSE Class 9 Math Chapter 6 – Lines and Angles serves as an essential foundation for understanding key geometric principles. Mastering the concepts in this chapter, such as the properties of different types of angles and lines, prepares you for more advanced topics in geometry. With a strong grasp of Class 9 Math Chapter 6 – Lines and Angles, you’ll be able to confidently tackle problems involving transversals, corresponding angles, and the angle sum property of triangles.
The practice problems and theorems discussed in Class 9 Math Chapter 6 – Lines and Angles help solidify these concepts and enhance your problem-solving skills. By using the resources provided in the iPrep Learning Super App, you can further strengthen your understanding of Class 9 Math Chapter 6 – Lines and Angles, setting the stage for success in your mathematics journey.
Practice questions on Chapter 6 - Lines And Angles
Get your free Chapter 6 - Lines And Angles practice quiz of 20+ questions & detailed solutions
Practice Now








