Binomial Theorem – Complete Guide For Class 11 Math Chapter 7
Our learning resources for Mathematics Class 11 ‘Binomial Theorem’ chapter 7 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions, and notes offer you the best of integrated learning with interesting explanations and examples.
Our comprehensive approach ensures that you have access to everything you need to have an in-depth understanding of the chapter Binomial Theorem. From detailed notes to interactive exercises, our materials are tailored to meet your learning needs and help you excel in your studies. Get ready to dive into an enriching educational experience that will make mastering this chapter a breeze.
What Is The Binomial Theorem?
The Binomial Theorem is a key concept in Class 11 Mathematics that provides a way to expand expressions raised to any positive integer power. Specifically, it applies to expressions of the form (a+b)ⁿ, where n is a non-negative integer. The theorem gives a formula for the expansion using binomial coefficients, which are organized in Pascal’s Triangle. The Binomial Theorem is essential for simplifying complex algebraic expressions and is widely applied in probability, calculus, and higher-level mathematics. It also introduces concepts like combinations, factorial notation, and general term formulas for series expansion.
We know:
- 99¹ = 99
- 99² = 9801
- 99³ = 970299
- 99⁴ = 96059601
- 99⁵ = ?
As the power increases, calculations become difficult. To ease this, we use the Binomial Theorem.
What is a Binomial?
The word “Binomial” comes from BI meaning two, and NOMIAL meaning terms.
Thus, a binomial expression has two terms connected by operators ‘+’ or ‘–’.
Examples: x−y, a+3b, etc., are binomials.
Expansion of Binomials
Let’s expand binomials:
- (a+b)² = a² + 2ab + b²
- (a−b)² = a² − 2ab + b²
- (a+b)³ = a³ + 3a²b + 3ab² + b³
- (a−b)³ = a³ − 3a²b + 3ab² − b³
For higher powers like (a+b)⁶ or (a−b)⁸, expansions become complex. This is where the Binomial Theorem comes in handy.
Some Basic Expansions
Observe these identities:
- (a+b)⁰ = 1
- (a+b)¹ = a + b
- (a+b)² = a² + 2ab + b²
- (a+b)³ = a³ + 3a²b + 3ab² + b³
- (a+b)⁴ = a⁴ + 4a³b + 6a²b² + 4ab³ + b⁴
Observations:
- The number of terms in the expansion is one more than the power.
- The powers of a decrease by 1, while those of b increase by 1 in successive terms.
- In each term of expansion, the sum of the indices of a and b is the same and equal to the index of (a + b).
- The coefficients follow a particular pattern.
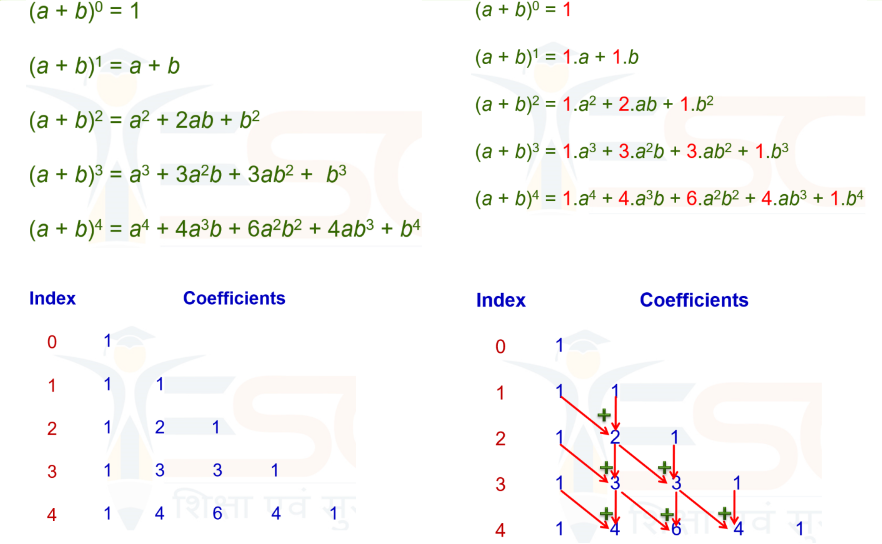
Pascal’s Triangle
Pascal’s Triangle helps in understanding the coefficients in binomial expansions. Here is how it looks:

Each number, except on the edges (which are 1), is the sum of the two numbers directly above it. This pattern can continue infinitely.
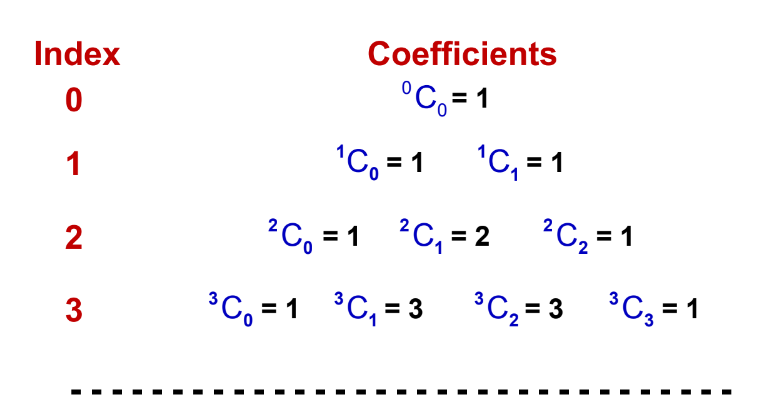
Pascal’s Triangle with Combinations
We can represent Pascal’s Triangle using combinations. The number of ways of choosing r objects from n distinct objects is given by:
ⁿCᵣ = n!/r!(n−r)!, where 0 ≤ r ≤ n.
Now, Pascal’s Triangle using combinations looks like this:
The Binomial Theorem
The Binomial Theorem helps expand any power of a binomial expression. For any positive integer n, the expansion of (a+b)ⁿ is given by:
(a + b)ⁿ = ⁿC₀aⁿb⁰ + ⁿC₁aⁿ⁻¹b¹ + ⁿC₂aⁿ⁻²b² + ………… + ⁿCₙa⁰bⁿ
where n is the power or index.
Binomial Coefficients
The coefficients of the terms in a binomial expansion are called binomial coefficients. For the expansion:
(a + b)ⁿ = ⁿC₀aⁿb⁰ + ⁿC₁aⁿ⁻¹b¹ + ⁿC₂aⁿ⁻²b² + ………… + ⁿCₙa⁰bⁿ
The coefficients ⁿCᵣ are known as binomial coefficients.
Note: The coefficients equidistant from the start and end are equal in binomial expansions.
General Term
The (r+1)th term in the expansion of (a+b)ⁿ is known as the general term, denoted by Tᵣ₊₁
Tᵣ₊₁ = ⁿCᵣ aⁿ⁻ʳ bʳ, where 0 ≤ r ≤ n.
Example
Find the general term in the expansion of (x – 1/x)⁵.
Solution:
Using the general term formula: Tᵣ₊₁ = ⁿCᵣ aⁿ⁻ʳ bʳ, where 0 ≤ r ≤ n.
Substitute the values n = 5, a = x and b = -1/x in the above equation to get
Tᵣ₊₁ = ⁵Cᵣ x⁵⁻ʳ (-1/x)ʳ, where 0 ≤ r ≤ 5.
Tᵣ₊₁ = -1ʳ ⁵Cᵣ x⁵⁻²ʳ, where 0 ≤ r ≤ 5.
Middle Term
Observation:
- If the index n is even, there is one middle term.
- If the index n is odd, there are two middle terms.
Case 1: n is even
The middle term is given by:
T₍ₙ/₂₊₁₎
For example, in (a+b)⁶, the middle term is T₄.
Case 2: n is odd
There are two middle terms, given:
T₍ₙ₊₁/₂₎ and T₍ₙ₊₁/₂₊₁₎
For example, in (a+b)⁹, the middle terms are T₅ and T₆.
Particular Term
Sometimes, a particular term is required from the binomial expansion. For example, the term independent of x in the expansion of (x+1/x)⁷ is a specific term.
Let’s Conclude
In conclusion, Chapter 7: Binomial Theorem is an essential topic in CBSE Class 11 Mathematics, offering a powerful tool to simplify and expand binomial expressions raised to any power. With the help of this theorem, students can solve complex algebraic equations and understand key mathematical concepts like binomial coefficients, combinations, and series expansions. Our interactive videos, practice exercises, and detailed notes are designed to make Chapter 7: Binomial Theorem easy to grasp, ensuring you gain a strong command over the topic. Mastering the Binomial Theorem will not only prepare you for exams but also help in advanced studies, making it a crucial part of your mathematical journey.
Practice questions on Chapter 7 - Binomial Theorem
Get your free Chapter 7 - Binomial Theorem practice quiz of 20+ questions & detailed solutions
Practice Now








