Conic Sections – Complete Guide For Class 11 Math Chapter 10
Our learning resources for Mathematics Class 11 ‘Conic Sections’ chapter 10 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions, and notes offer you the best of integrated learning with interesting explanations and examples.
Our comprehensive approach ensures that you have access to everything you need to have an in-depth understanding of the chapter Conic Sections. From detailed notes to interactive exercises, our materials are tailored to meet your learning needs and help you excel in your studies. Get ready to dive into an enriching educational experience that will make mastering this chapter a breeze.
What Are Conic Sections?
Conic sections are curves obtained by intersecting a plane with a double-napped cone. The main types of conic sections are circles, ellipses, parabolas, and hyperbolas, each defined by the angle and position of the intersecting plane. This chapter introduces the standard equations of these curves and their geometric properties, including eccentricity, foci, and directrices. Understanding conic sections is fundamental in various fields such as physics, engineering, and astronomy, as these curves describe the paths of planets, projectiles, and light rays. The study of conic sections provides essential insights into analytical geometry.
Fixed Line (l)
Imagine a figure generated by rotating the line l around a fixed line in such a way that the angle
α remains constant.

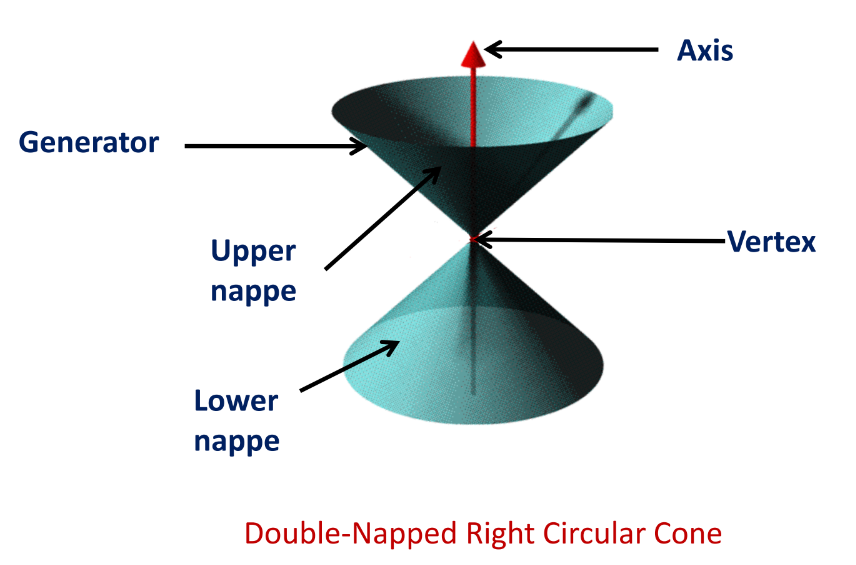
Components of the Cone
- Axis
- Generator
- Upper Vertex
- Lower Nappe
- Double-Napped Right Circular Cone
Conic Sections
- Conic sections are plane curves that can be obtained by intersecting a cone with a plane.
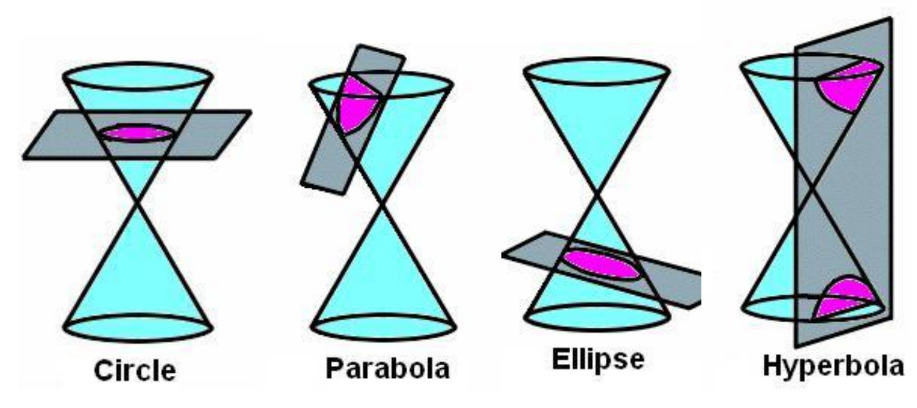
The intersection of a Double-Napped Right Circular Cone With a Plane
When a Plane Does Not Pass Through the Vertex
Let β be the angle between the plane and the vertical axis of the cone.
- If β = 90°, the section is a circle.

If β = α
- When the plane is parallel to the generator, the section is a parabola.

If α < β < 90°
- The section is an ellipse.
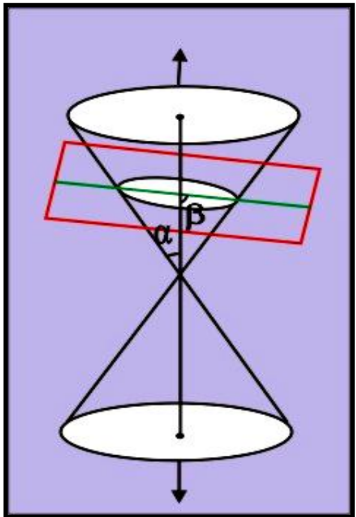
If 0 ≤ β < α
- The section is a hyperbola.
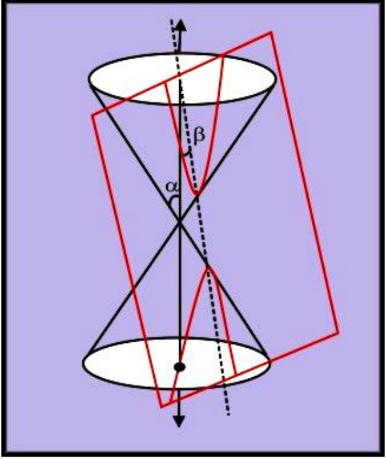
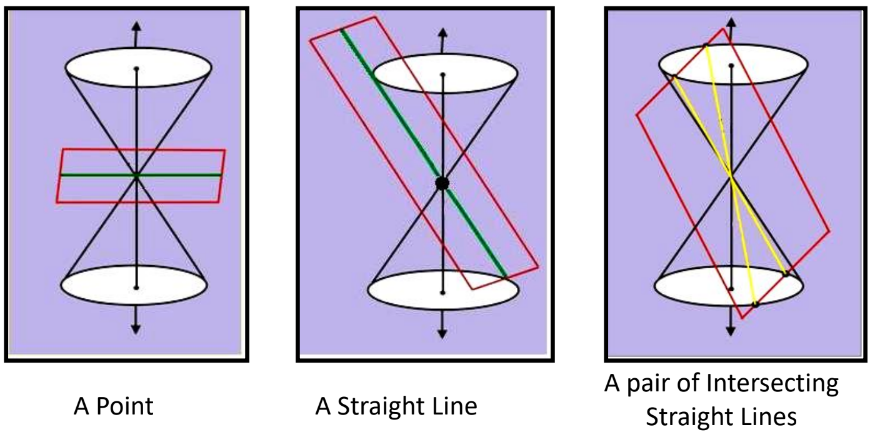
Degenerated Conic Sections
- When the plane passes through the vertex of a cone, the resulting conic is called a degenerated conic.
The degenerated conic can be:
- A point
- A straight line
- A pair of intersecting straight lines
Circle
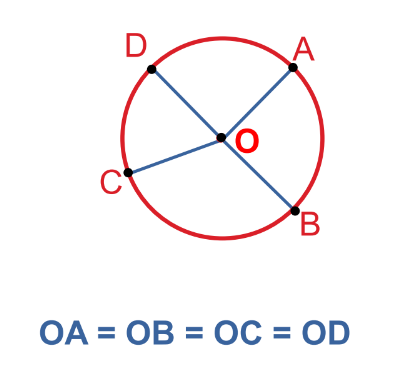
- A circle is the set of all points in a plane that are equidistant from a fixed point in the plane.
Equation of a Circle
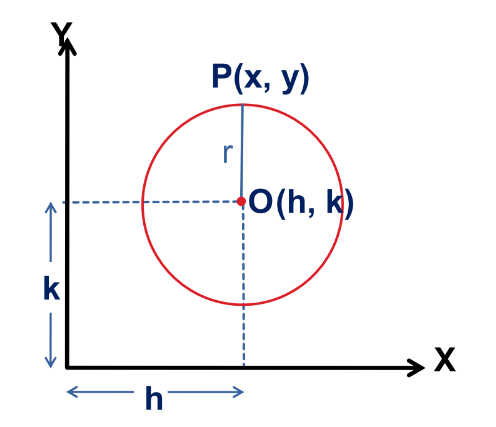
(x–h)² + (y–k)² = r²
Where (h,k) is the center and r is the radius.
Example:
Find the equation of the circle with center (2,3) and radius 2.
Solution:
Here h = 2, k = 3 and r = 2.
Therefore, the equation of the circle is:
(x–2)² + (y–3)² = 4
Parabola
- The path described by a ball is a parabola.
Definition of a Parabola
- A parabola is the set of all points in a plane that are equidistant from a fixed line and a fixed point (not on the line) in the plane.
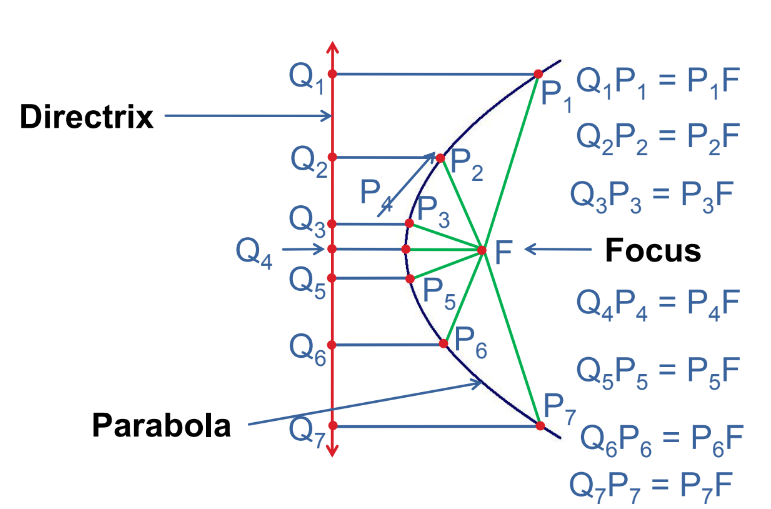
Components of a Parabola
- The line through the focus and perpendicular to the directrix is called the axis of the parabola.
- The point where the parabola meets its axis is called the vertex of the parabola.

Standard Equations of a Parabola
- y² = 4ax, a > 0
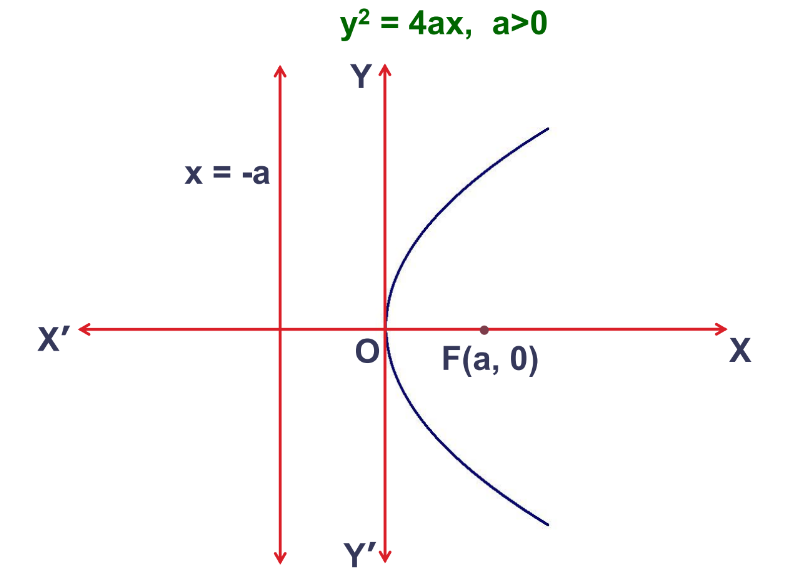
- y² = −4ax, a > 0

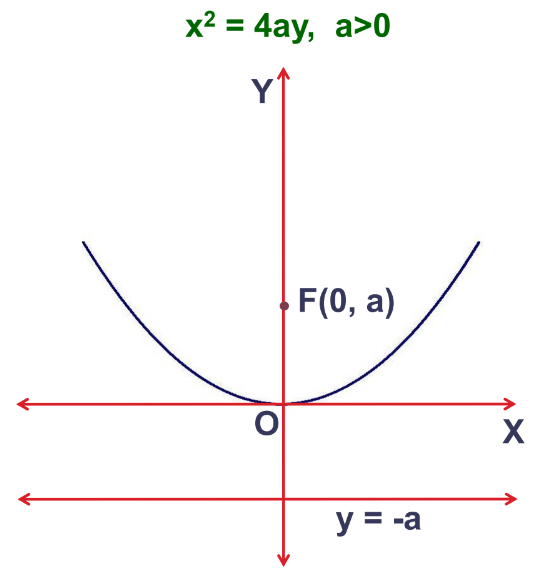
- x² = 4ay ,a > 0
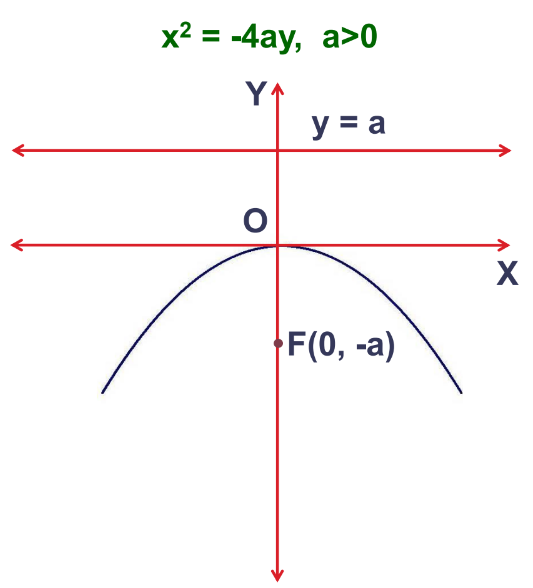
- x² = −4ay ,a > 0
Latus Rectum of a Parabola
- The latus rectum is a line segment through the focus, perpendicular to the axis of the parabola, and its endpoints lie on the parabola.
- The length of the latus rectum for the parabola y² = 4ax is 4a.
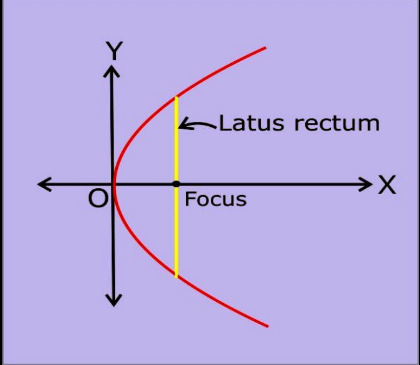
Example:
Find the axis, coordinates of the focus, the equation of the directrix, and the latus rectum of the parabola y² = 12x.
Solution:
The parabola is of the form y² = 4ax, with a positive coefficient of x.
Comparing y² = 12x with y² = 4ax, we get a = 3a.
Thus, the focus is (3,0), the directrix is x = −3, and the length of the latus rectum is 4a = 12.
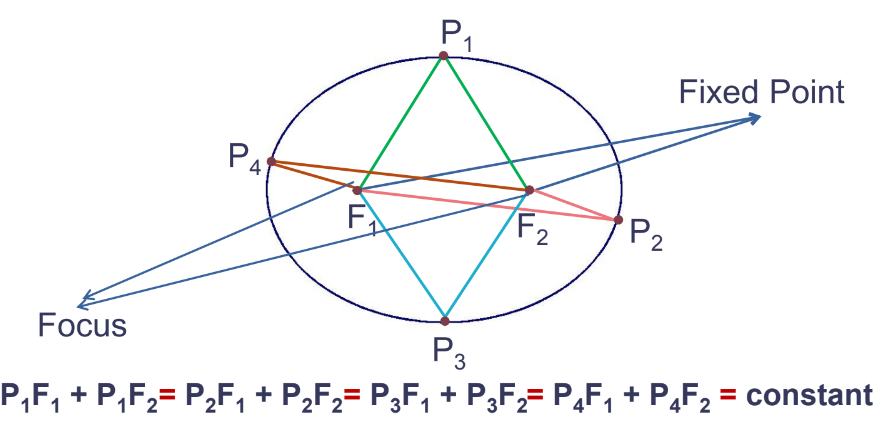
Ellipse
- An ellipse is a set of all points in a plane, the sum of whose distances from two fixed points in the plane is a constant.
This stadium is in the shape of an ellipse.

Components of an Ellipse
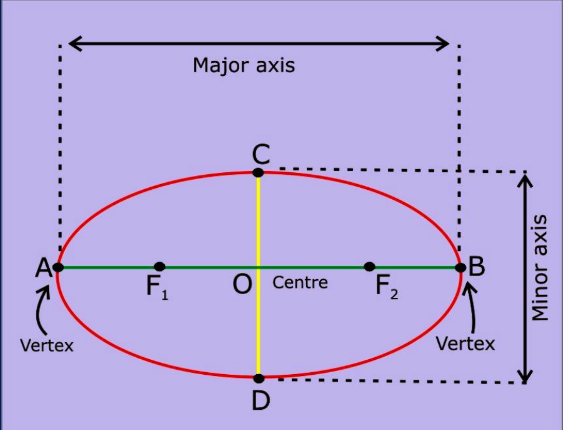
- Length of the major axis: 2a
- Length of the minor axis: 2b
- Distance between the foci: 2c
- The relationship between a, b, and c is given by:
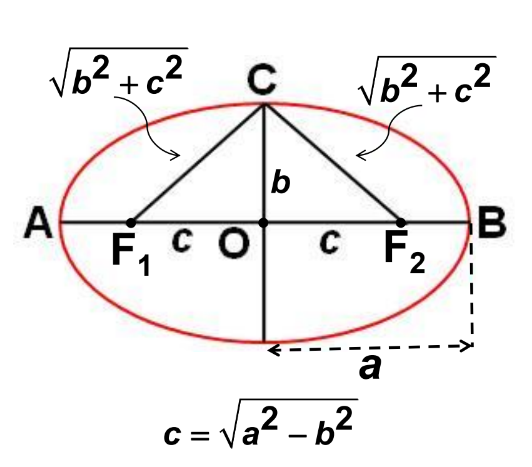
Eccentricity of an Ellipse
- The eccentricity of an ellipse is given by e = c/a.

Standard Equations of an Ellipse
In standard equations of an ellipse, the center is at the origin and the major and minor axis are the coordinate axes.
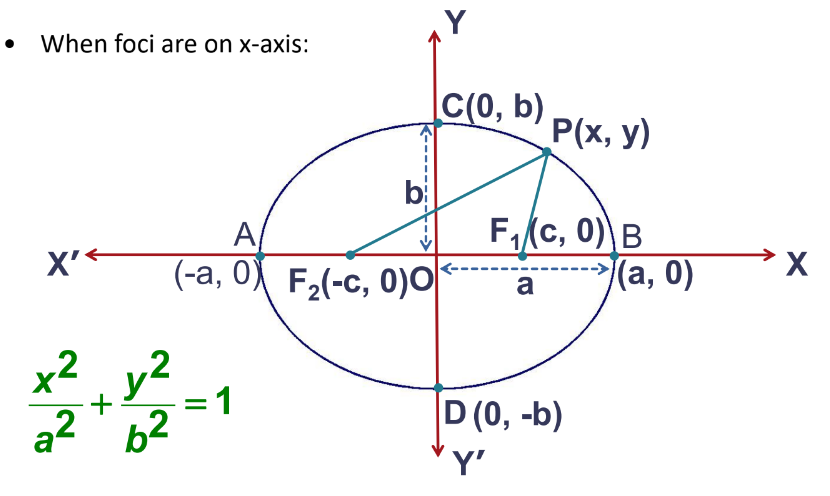
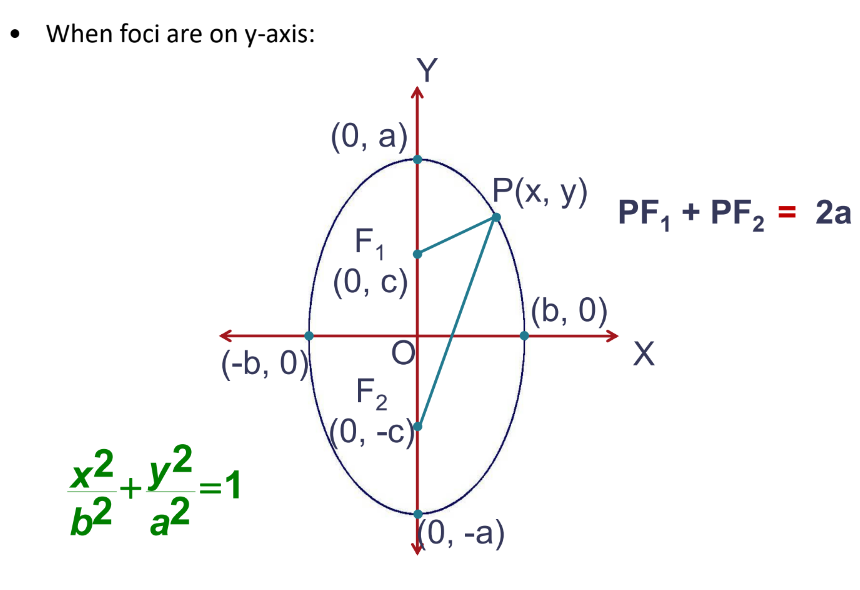
Latus Rectum of an Ellipse
- The latus rectum of an ellipse is a line perpendicular to the major axis passing through any of the foci whose endpoints lie on the ellipse.

The length of the latus rectum is 2b²/a.
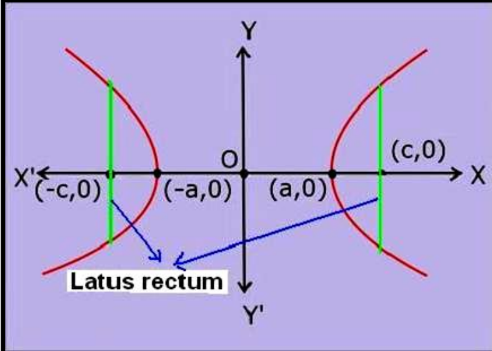
Hyperbola
The hourglass demonstrates the characteristics of a hyperbola.

- A hyperbola is the set of all points in a plane, the difference of whose distances from two fixed points in the plane is constant.
Components of a Hyperbola
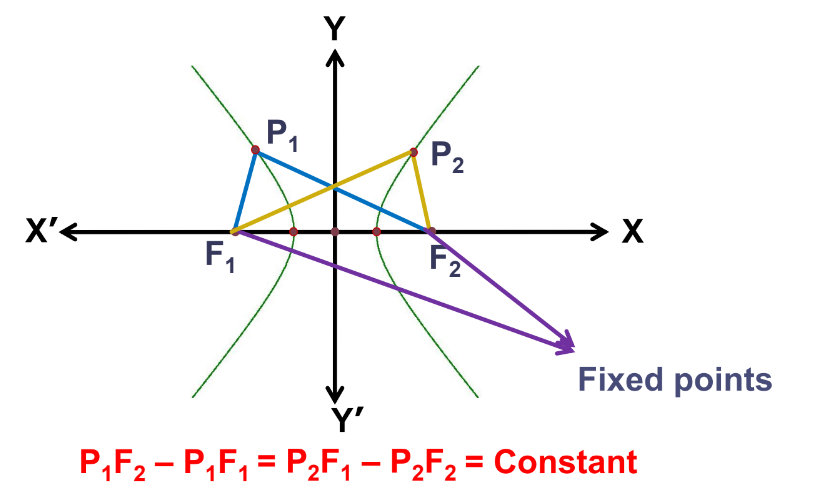
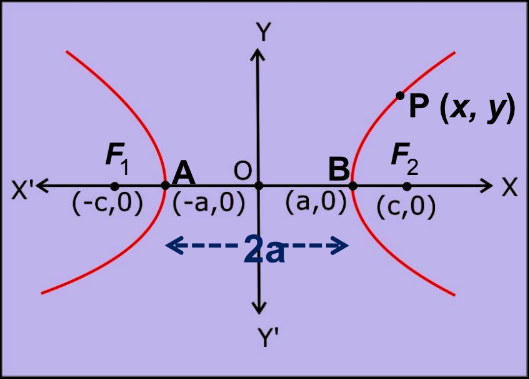
- The two fixed points are called the foci (plural of focus).
- The distance between two foci is denoted by 2c.
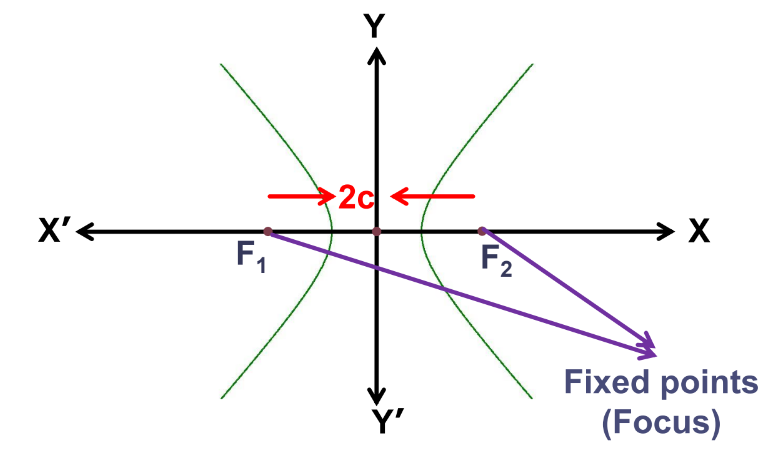
- Vertices: The points where the curve meets the line joining the foci are called the vertices. We denote the distance between two vertices by 2a.
- Center: The mid-point of the line segment joining the vertices is called the center of the hyperbola.
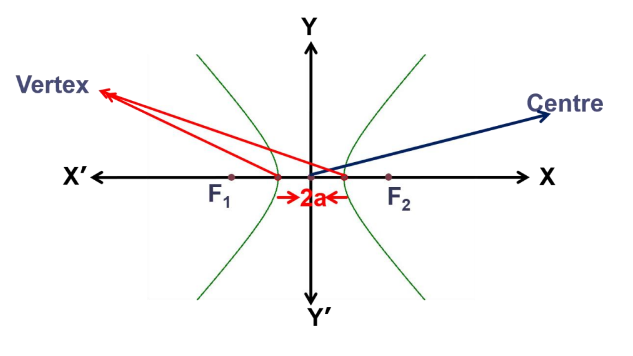
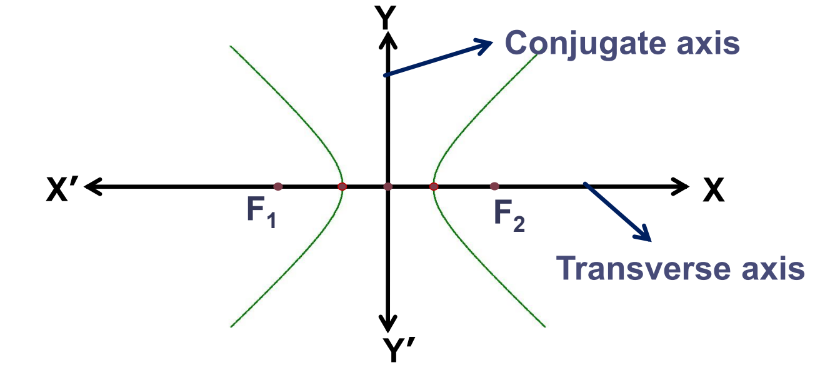
- Transverse axis: The line joining the vertices is called the transverse axis. Its length is generally taken as 2a, i.e., the distance between two vertices.
- Conjugate axis: The line through the center and perpendicular to the transverse axis is called the conjugate axis. We define 2b as the length of the conjugate axis, such that b² = c².
An important result:
If P(x, y) is any point on the hyperbola and F₁ and F₂ are two foci. Then, PF₁ – PF₂ = constant = 2a.
Eccentricity of a Hyperbola
The eccentricity of a hyperbola is given by e = c/a

Standard Equations of a Hyperbola
- Equation with foci on the x-axis: x²/a² − y²/b² = 1.
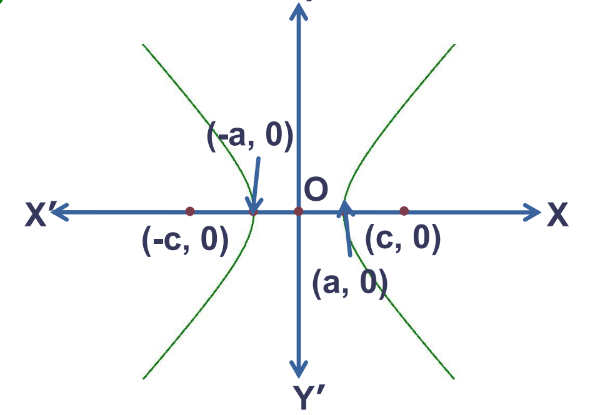
- Equation with foci on the y-axis: y²/a² − x²/b²=1.

Latus Rectum of a Hyperbola
- The latus rectum of a hyperbola is a line segment perpendicular to the transverse axis passing through any of the foci. Its length is 2b²/a.
Example: Find the coordinates of the foci, the vertices, the eccentricity, and the length of the latus rectum of the hyperbola.
x²/4 − y²/9 = 1.
Solution: On comparing the given equation with x²/a² − y²/b² = 1.
We get a = 2 and b = 3
c = √a² + b² = √13
Therefore, the coordinates of the foci are:
(-√13,0) and (√13,0)
Vertices are (-2, 0) and (2, 0)
The eccentricity, e = c/a = √13/2
Length of the latus rectum = 2b²/a = 18/2 = 9.
This concludes Chapter 10: Conic Sections from Class 11 Mathematics, covering all key concepts including circles, parabolas, ellipses, and hyperbolas, with relevant equations and examples.
Let’s Conclude
In conclusion, the Conic Sections chapter from CBSE Class 11 Mathematics serves as a foundational topic in analytical geometry. It explores different types of curves, including circles, parabolas, ellipses, and hyperbolas, providing insights into their geometric properties and equations. Mastering Conic Sections not only enhances your understanding of these curves but also builds a strong base for applications in fields like physics, engineering, and astronomy. By thoroughly engaging with this chapter, you will be well-prepared to tackle more advanced topics. Keep revising and practicing the concepts in Conic Sections to ensure a clear grasp and excel in your studies.
Practice questions on Chapter 10 - Conic Sections
Get your free Chapter 10 - Conic Sections practice quiz of 20+ questions & detailed solutions
Practice Now








