Limits and Derivatives – Complete Guide For Class 11 Math Chapter 12

Our learning resources for Mathematics Class 11 ‘Limits and Derivatives’ chapter 12 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions, and notes offer you the best of integrated learning with interesting explanations and examples.

Our comprehensive approach ensures that you have access to everything you need to have an in-depth understanding of the chapter Limits and Derivatives. From detailed notes to interactive exercises, our materials are tailored to meet your learning needs and help you excel in your studies. Get ready to dive into an enriching educational experience that will make mastering this chapter a breeze.

What Are Limits and Derivatives?

A very simple definition of limits and derivatives is that Limits define the behavior of a function as it approaches a particular point, while derivatives represent the rate of change of a function at that point. Chapter 12 introduces the foundational concepts of limits and derivatives, essential in calculus. The chapter starts by defining limits, and exploring how a function behaves as the input approaches a particular value. Students learn methods for calculating limits and the idea of continuity.

The second part of the chapter focuses on derivatives, which measure the rate of change of a function. Basic differentiation rules are covered, along with practical applications such as finding the slope of a curve at a point. These concepts form the basis for more advanced calculus topics in later studies.

In this chapter- Limits and Derivatives, we will explore the concept of limits and derivatives, focusing on how the dependent variable (output) of a function behaves as the independent variable (input) approaches a particular value. The key idea here is the notion of “approaching” a value rather than reaching it.

Understanding Limits

When learning the chapter limits and derivatives, it is crucial to properly understand the concept of limits. Here is a detailed understanding:

• A limit is the value that a function approaches as the independent variable gets closer to a specific value.
• The important word here is “approaches,” as the function doesn’t reach the value but gets infinitely close to it.

Let’s explore this idea using an example.

Example: Function g(x) = 1/x​

In this example, we will gradually increase the value of x, and observe what happens to g(x):

As we can see, as x increases towards infinity, the value of g(x) approaches zero. However, g(x) never actually reaches zero.

Types of Limits

The chapter on limits and derivatives also covers the various types of limits. There are two main types of limits, based on the direction from which we approach a given value:

1. Right-Hand Limit
   lim⁡x→a⁺f(x): This represents the limit of f(x) as x approaches a from the right side (i.e., x>a).
2. Left-Hand Limit
   lim⁡x→a⁻f(x): This represents the limit of f(x) as x approaches aaa from the left side (i.e., x<a).

Right-Hand Limit

• As x→a⁺, we denote this as lim⁡x→a⁺f(x) = L, where L is the limiting value.

Left-Hand Limit

• Similarly, as x→a⁻, we denote this as lim⁡x→a⁻f(x) = L.

A Limit That Does Not Exist

Sometimes, a function does not approach a specific limit as x approaches a particular value. In such cases, we say the limit does not exist.

Example

Consider the function:

g(x) = x² − 10/x−3​

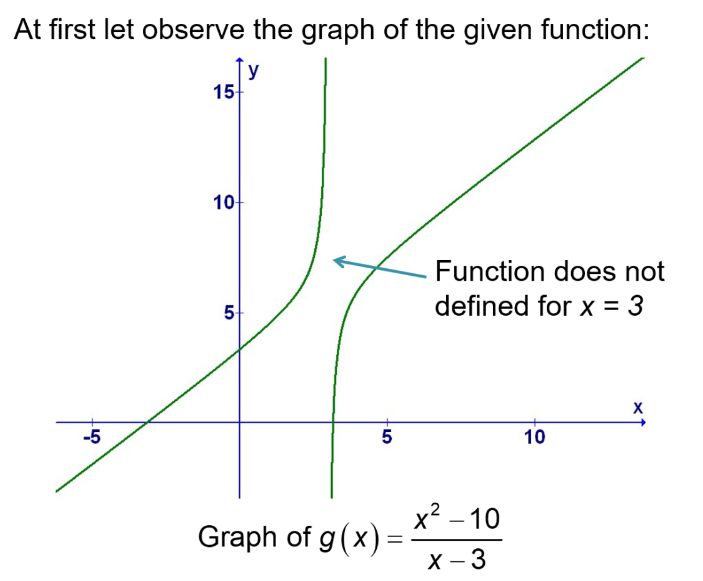
The following table illustrates how g(x) behaves as x approaches 3 from the left and right:

As x gets closer to 3, the value of g(x) increases without bounds on both sides, meaning the limit does not exist.

Algebra of Limits

Within the chapter – Limits and Derivatives, the Algebra of Limits refers to the set of rules and operations that govern the behavior of limits when combined through various arithmetic operations. These rules make it easier to calculate the limits of complex expressions by breaking them down into simpler components.

Here are the key algebraic properties or rules of limits:

1. Limit of a Sum

If Lim⁡x→c f(x) = L and lim⁡x→c g(x)=M, then the limit of their sum is:

Lim⁡x→c [f(x)+g(x)] = lim⁡x→cf(x) + lim⁡x→c g(x) = L + M

2. Limit of a Difference

Similarly, the limit of the difference between the two functions is:

If Lim⁡x→c f(x) = L and lim⁡x→c g(x)=M, then the limit of their sum is:

Lim⁡x→c [f(x)-g(x)] = lim⁡x→cf(x) – lim⁡x→c g(x) = L – M

3. Limit of a Product

If the limits of two functions exist, then the limit of their product is:

If Lim⁡x→c f(x) = L and lim⁡x→c g(x)=M, then the limit of their sum is:

Lim⁡x→c [f(x).g(x)] = lim⁡x→cf(x) . Lim⁡x→c g(x) = L . M

4. Limit of a Quotient

If Lim⁡x→c g(x) ≠ 0, the limit of the quotient of two functions is:

lim⁡x→c[f(x)/g(x)] = lim⁡x→c f(x)/lim⁡x→c g(x) = L/M​

Note that the denominator should not be zero, i.e., M ≠ 0.

5. Limit of a Constant Multiple

If k is a constant and lim⁡x→c f(x) =L, then the limit of a constant multiple of a function is:

Lim⁡x→c [k⋅f(x)] = k⋅lim⁡x→c f(x) = k⋅L

6. Limit of a Power

For any positive integer n, if lim⁡x→c f(x) = L, then the limit of the power of a function is:

Lim⁡x→c [f(x)]ⁿ = [lim⁡x→cf(x)]ⁿ = Lⁿ

Limits of Polynomial Functions

The chapter limits and Derivatives thoroughly covers the limits of polynomial functions with an example. A polynomial function can be expressed as:

f(x) = a₀ + a₁x + a₂x² + ⋯ + aₙxⁿ

The limit of a polynomial function as x approaches a value a is simply the value of the polynomial at x = a:

Lim⁡x→a f(x) = f(a)

Example:

Evaluate the limit of the polynomial function:

Lim⁡x→1 (5x² − 3x + 4)

Using the addition and product rules, we get:

Lim⁡x→1 (5x² − 3x + 4) = 5 Lim⁡x→1 (x² ) – 3 Lim⁡x→1(X) + 4 = 5(1)2 − 3(1) + 4 = 6

Limits of Rational Functions

As stated in the chapter – Limits and Derivatives, a rational function is a function of the form:

f(x) = P(x)/Q(x)​, where P(x) and Q(x) are polynomial functions, and Q(x) ≠ 0.

To calculate the limit of a rational function as x→c, we apply the algebra of limits. The key is to check the behavior of both the numerator and the denominator as x→c.

There are three possible cases when evaluating the limit of a rational function:

Case 1: Limit Exists (No Indeterminate Form)

If both the numerator and denominator are finite at x = c, and the denominator Q(x) ≠ 0 at x = c, the limit can be directly computed using:

Lim⁡ x→c f(x) = lim⁡ x→c P(x)/lim⁡ x→c Q(x)​

Example:

Lim⁡x→2 (x²−3x+2)/x−1​

1. Evaluate the numerator: lim⁡ x→2 (x²−3x+2) = (2²−3(2)+2) = 4 − 6 + 2 = 0
2. Evaluate the denominator: lim ⁡x→2 (x−1) = 2 − 1 = 1.

Thus:

Lim⁡ x→2 x²−3x+2/x−1 = 0/1 = 0

Case 2: Indeterminate Form 0/0​

If both the numerator and denominator tend to zero as x→c, this results in an indeterminate form 0/0​. In this case, further simplification (such as factoring or using L’Hôpital’s Rule) is required.

Example:

Lim⁡x→1 x²−1/x−1​

1. The numerator x² − 1 = (x−1)(x+1), so the expression becomes:

Lim⁡ x→1 (x−1)(x+1)/x−1​

2. Cancel out the common factor (x−1) (provided x ≠ 1):

lim⁡x→1(x+1) = 1 + 1 = 2

Thus: lim ⁡x→1 x² − 1/x−1 = 2

Case 3: Limit at Infinity

When x→∞, we consider the degree of the numerator and denominator.

1. If the degree of the numerator is less than the degree of the denominator:
   Lim⁡x→∞ f(x) = 0
2. If the degree of the numerator is equal to the degree of the denominator:
   Lim⁡x→∞ f(x) = leading coefficient of P(x)/leading coefficient of Q(x)
3. If the degree of the numerator is greater than the degree of the denominator:
   Lim ⁡x→∞ f(x) = ∞ (or −∞ depending on the signs)

Now that we understand the limits of rational numbers, let’s go deeper into the chapter limits and derivatives and understand the important results and theorems.

Important Results and Theorems

There are various important results and theorems introduced in the chapter limits and derivatives. These include:

For any positive integer n,

Lim⁡ x→a (xⁿ – aⁿ)/ (x – a) = naⁿ⁻¹

Limits of Trigonometric Functions

The following results are useful for evaluating limits involving trigonometric functions:

• Lim⁡ x→0 sin⁡x/x = 1
• Lim⁡ x→0 1 − cos⁡x/x = 0​

Sandwich Theorem

If f(x) ≤ g(x) ≤ h(x) for all x in a certain interval around a, and if:

Lim⁡ x→a f(x) = lim ⁡x→a h(x) = L

Then:

Lim⁡ x→a g(x) = L

Derivatives 

Consider a bicyclist riding a bicycle. Suppose we know the position of a bicycle at various time intervals. Is it possible to find out the rate at which its position is changing?

The answer to the above question is yes; as by the concept of limits, we can find the rate by making time intervals smaller and smaller.

Suppose f is a real-valued function and ‘a’ is a point in its domain of definition. The derivative of f at ‘a’ is defined by: 

Lim⁡ h→0 f( a + h) – f(a)/ h

Provided this limit exists. The derivative of f(x) at ‘a’ is denoted by f’(a).

Note that f’(a) represents the numerical change in f(x) at a with respect to x.

Definition Of Derivatives?

As per the chapter – Limits and Derivatives, a derivative of a function measures how the function’s output changes as its input changes. It is a fundamental concept in calculus that deals with the rate of change of a quantity. For Class 11 Mathematics, the derivative can be understood as a measure of the slope of the tangent to the curve at a given point.

1. Geometrical Meaning of Derivative

The derivative of a function at a point gives the slope of the tangent line to the curve at that point. This slope indicates how steep the function is increasing or decreasing at that specific point.

• If the slope is positive, the function is increasing.
• If the slope is negative, the function is decreasing.
• If the slope is zero, the function has a horizontal tangent, indicating a local maximum, minimum, or point of inflection.

2. Definition of Derivative

Let f(x) be a function, and suppose we want to find the derivative at x = a. The derivative is defined as:

f′(a) = lim⁡ h→0 f(a+h) − f(a)/h​

This formula represents the rate of change of the function f(x) as x approaches a. The derivative exists if the limit exists.

3. Derivative as a Rate of Change

The derivative also represents the instantaneous rate of change of a function. For example, if f(x) represents the position of an object at time x, then the derivative f′(x) represents the velocity of the object at that specific time.

4. Notation for Derivatives

There are several ways to denote the derivative of a function:

• f′(x) or f′(a)
• dy/dx (when y=f(x), this notation is used to represent the derivative of y concerning x).
• d/dx[f(x)]

5. Example: Derivative of a Polynomial Function

Let’s consider a simple function f(x) = x². To find its derivative using the definition, we proceed as follows:

f′(x) = lim ⁡h→0 (x+h)²− x²/h​

1. Expand (x+h)²:

(x+h)² = x² + 2xh +h²

2. Subtract x² from both sides:

f′(x) = lim ⁡h→0 x² + 2xh + h² − x²/h = lim ⁡h→0 2xh + h²

3. Simplify:

f′(x) = lim ⁡h→0 (2x+h) 

4. As h→0h \to 0h→0, the term hhh vanishes, leaving:

f′(x) = 2x

Thus, the derivative of x² is 2x.

6. Basic Rules of Differentiation

Some basic rules make finding derivatives easier:

• Constant Rule: The derivative of a constant is zero.
  d/dx(c) = 0
• Power Rule: The derivative of xⁿ is n⋅xⁿ⁻¹

d/dx (xⁿ) = n⋅xⁿ⁻¹

• Sum Rule: The derivative of the sum of two functions is the sum of their derivatives.
  d/dx [f(x) + g(x)] = f′(x) + g′(x)
• Product Rule: The derivative of the product of two functions is:
  d/dx [f(x) ⋅g(x)] = f′(x).g(x) + f(x).g′(x)
• Quotient Rule: The derivative of the quotient of two functions is:
  d/dx [f(x)/g(x)] = f′(x)g(x) − f(x)g′(x)/g(x)²​

7. Derivative of Trigonometric Functions

For Class 11, you should also know the derivatives of basic trigonometric functions:

• d/dx (sin⁡x) = cos⁡x
• d/dx (cos⁡x) = −sin⁡x
• d/dx (tan⁡x) = sec⁡²x

8. Applications of Derivatives

• The slope of a Curve: Derivatives help in finding the slope of a curve at any point.
• Rate of Change: It helps in determining how a quantity changes concerning time or any other variable.
• Optimization: Derivatives are used to find local maxima and minima (important in optimization problems).
• Kinematics: In physics, the derivative of position concerning time gives velocity, and the derivative of velocity gives acceleration.

Conclusion

Limits are essential in understanding the behavior of functions as inputs approach specific values. The concept of limits leads to the derivative, which is fundamental to calculus and allows us to measure rates of change.

The concept of a derivative is all about understanding how a function changes at any given point. It can be interpreted geometrically (as the slope of a tangent line) or physically (as a rate of change). Mastering the derivative rules and understanding their applications are key skills in Class 11 Mathematics.

In conclusion, CBSE Math Chapter 12: Limits and Derivatives serves as a pivotal foundation in your journey through calculus. By grasping the concept of limits, you begin to understand how functions behave as they approach specific values. This leads seamlessly into the derivative, a crucial tool that measures the rate of change of a function at any point.

Mastering these concepts is not just about passing exams; it’s about developing a deeper understanding of mathematical principles that will be essential in advanced studies. With our comprehensive resources tailored for Limits and Derivatives, you will find engaging materials that make these complex ideas accessible and enjoyable. Embrace the challenge, and let Chapter 12: Limits and Derivatives guide you toward excellence in mathematics!