Relations and Functions – Complete Guide For Class 11 Math Chapter 2

Welcome to iPrep, a one-stop learning super app. Our learning resources for Mathematics Class 11 ‘Relations and Functions’ chapter 2 are designed to ensure that you grasp this concept with clarity and perfection. Whether you’re studying for an upcoming exam or strengthening your concepts, our engaging animated videos, practice questions, and notes offer you the best of integrated learning with interesting explanations and examples.

Our comprehensive approach ensures that you have access to everything you need to have an in-depth understanding of the chapter Relations and Functions. From detailed notes to interactive exercises, our materials are tailored to meet your learning needs and help you excel in your studies. Get ready to dive into an enriching educational experience that will make mastering this chapter a breeze.

Introduction To Relations And Functions

This chapter introduces the concepts of relations and functions, essential in understanding mathematical relationships. A relation is a rule that pairs elements from one set to another, often represented as ordered pairs. A function is a special type of relation where each element in the domain is related to exactly one element in the codomain. The chapter covers different types of relations, functions (one-to-one, onto, bijective), the domain, codomain, and range, as well as graphical representations of functions. Mastering these concepts is crucial for advanced topics in calculus and algebra.

In this chapter, we delve into the fundamental concepts of relations and functions. From understanding ordered pairs to working with Cartesian products, relations, and different types of functions, the chapter builds a strong foundation for higher-level mathematical concepts.

In daily life, we encounter various relationships such as teacher and student, or parent and child. Similarly, mathematics deals with relations like:

• Parallel and Perpendicular lines
• Subsets and Similarities between triangles

Ordered Pair

An ordered pair consists of two objects or elements arranged in a fixed order. It is written as:

(a,b)

Where a is the first component and b is the second component. Examples include:

• (Ram, Sita)
• (Krishna, Radha)
• (Shiva, Gauri)

Note: Two ordered pairs (a, b) and (x, y) are equal only if a = x and b = y.

Cartesian Product

The Cartesian product of two non-empty sets A and B is the set of all ordered pairs (a,b), where a ∈ A and b ∈ B. It is denoted by A×B.

A×B = {(a,b): a ∈ A and b ∈ B}

Example:

If A = {a,b} and B = {1,2,3}, then:

A×B = {(a,1),(a,2),(a,3),(b,1),(b,2),(b,3)}

Note: If set A has p elements and set B has q elements, then A×B will contain p×q elements.

Graphical Representation of Cartesian Product

To graphically represent the Cartesian product of sets A and B:

1. Draw two perpendicular lines (horizontal and vertical).
2. Mark the elements of A on the horizontal line and the elements of B on the vertical line.
3. For each ordered pair (a,b), draw vertical and horizontal lines intersecting at a point representing (a,b).

Example:

Let A = {1,2} and B = {1,2,3}, the Cartesian product A×B is:

A×B = {(1,1),(1,2),(1,3),(2,1),(2,2),(2,3)}

Relations

A relation from set A to set B is a subset of A×B. The subset is formed by describing a relationship between the first and second elements of the ordered pairs.

If (a,b) ∈ R, we say that A is related to B, and we write a R b.

Total Number of Relations

If n(A) = p and n(B) = q, then the total number of possible relations from A to B is 2pq. 

Representation of Relations

A relation R can be represented in the following forms:

1. Roster Form

The relation is represented by listing all ordered pairs.
Example:
Let R be the relation from A = {2,1,0,−1,−2} to B = {0,1,4,9}, defined by the relation x² = y.
Then R = {(0,0),(1,1),(−1,1),(2,4),(−2,4)}.

2. Set-Builder Form

A relation can be described in set-builder notation.
Example:
Let A = {1,2,3,4,5,6} and B = {1,3,5}, and R is defined by:

R = {(a,b): a ∈ A, b ∈ B, a < b}

3. Arrow Diagram

In an arrow diagram, draw arrows from each element of set A to the related elements of set B.

Domain, Range, and Codomain

• Domain: The set of all first components of the ordered pairs in a relation is called the domain.
• Range: The set of all second components is called the range.
• Codomain: The entire set B is called the codomain.

Example:

Let R = {(1,3),(1,5),(2,3),(2,5),(3,5),(4,5)},

• Domain of R = {1, 2, 3, 4}
• Range of R = {3, 5}
• Codomain of R = {1, 3, 5}

Inverse Relation

An inverse relation reverses the pairs of a given relation. If a relation R contains the pair (a,b), the inverse relation R contains (b, a). For example, if R⁻¹ = {(1,2),(3,4)}, then R⁻¹ = {(2,1),(4,3)}.

Functions

A function is a special type of relation from set A to set B where each element of A is related to a unique element of B.

Domain, Codomain, and Range of a Function:

If f : A → B,

• Domain: Set A
• Codomain: Set B
• Range: The set of all f-images of elements of A.

Real-Valued and Real Functions

• Real-Valued Function: A function where the range is a subset of real numbers R.
• Real Function: A function where both the domain and range are subsets of real numbers R.

Types of Functions

In the chapter Relations and Functions, there are various types of functions mentioned. These include-

1.  Identity Function

A function f : R → R is called an identity function if f(x) = x for all x ∈ R.

2.  Constant Function

A function f : R → R is a constant function if f(x) = a for all x ∈ R, where A is a fixed real number.

3.  Polynomial Function

A polynomial function f(x) is defined as:

f(x) = a₀ + a₁x + a₂x² + ⋯ + aₙxⁿ

4. Rational function

A rational function is a function that can be expressed as the ratio of two polynomials, i.e., f(x)=P(x)/Q(x)​, where P(x) and Q(x) are polynomials, and Q(x) ≠ 0. The domain of a rational function excludes values of x that make Q(x) = 0.

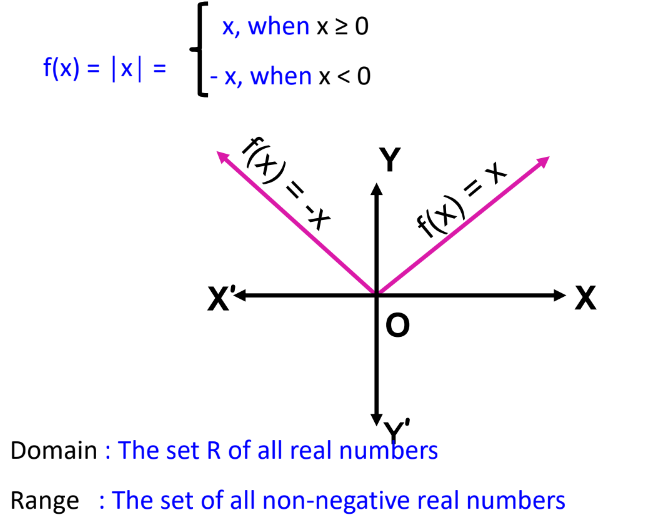
5.  Modulus Function

The modulus function is defined as:

f(x) = ∣x∣ = {x, if x ≥ 0 and -x, if x < 0}​

6.  Signum Function

The Signum function is defined as:

f(x) = {1, if x > 0, 0 if x = 0 and -1 if x < 0}

​

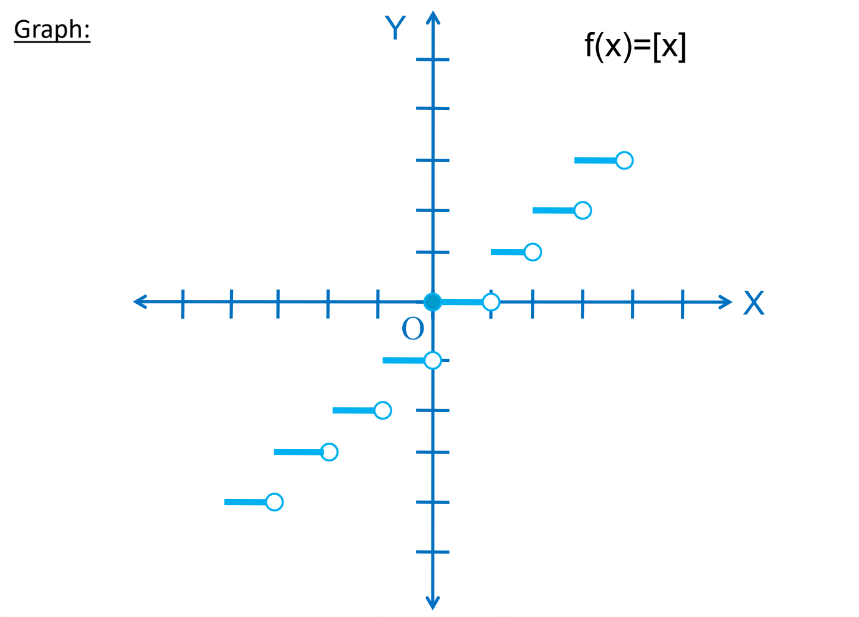
7. Greatest Integer Function

The greatest integer function, denoted as f(x) = ⌊x⌋, assigns to each real number x the largest integer less than or equal to x. For example, ⌊3.7⌋ = 3 and ⌊−2.3⌋ = −3. It is a step function with discontinuities at integer points.

Algebra of Real Functions

Real functions can be combined through addition, subtraction, multiplication, and division:

1. Addition: (f+g)(x) = f(x) + g(x)
2. Subtraction: (f−g)(x) = f(x) − g(x)
3. Multiplication: (f⋅g)(x) = f(x)⋅g(x)
4. Division: (f/g)(x) = f(x)/g(x), where g(x)≠0

This concludes the key points of the chapter “Relations and Functions” for Class 11 Mathematics. Understanding these foundational concepts will help you excel in advanced mathematical topics.

Let’s Conclude

In conclusion, the chapter “Relations and Functions” in CBSE Class 11 Mathematics lays a crucial foundation for your mathematical journey. By thoroughly engaging with the concepts of relations, functions, Cartesian products, and their graphical representations, you will gain a deeper understanding of how mathematical relationships are structured and analyzed. Whether you’re dealing with ordered pairs, domains, codomains, or various types of functions, mastering this chapter will equip you with essential skills that apply to more advanced topics in algebra and calculus.

Our comprehensive resources, including animated videos, practice exercises, and detailed notes, are designed to help you navigate this chapter with confidence. The chapter “Relations and Functions” is not just about memorizing definitions but about developing a conceptual framework that will support your learning in future mathematics courses. By engaging with these materials and applying your knowledge, you’ll be well-prepared to tackle more complex mathematical challenges.

Embrace the learning experience provided by our resources and take full advantage of the insights offered in the chapter “Relations and Functions.” With consistent practice and a clear understanding of the foundational concepts, you’ll find that mastering this chapter is a rewarding and achievable goal.